23. Quantum Mechanics V: Particle in a Box
TLDRThe video script is a detailed lecture on quantum mechanics, focusing on the particle in a box problem and the concept of energy quantization. The professor begins by discussing the wave function and its relationship with energy measurement, emphasizing the probabilistic nature of quantum mechanics. The main example used is that of a particle confined within an infinitely deep potential well, or 'box'. The lecture explores the mathematical formulation of the problem, resulting in quantized energy levels and corresponding wave functions that must vanish at the boundaries of the well. The script also delves into the physical interpretation of these wave functions and the implications for the particle's momentum and position. Furthermore, the professor touches upon the Heisenberg Uncertainty Principle and its role in the particle's behavior within the box. The lecture concludes with a discussion on scattering in quantum mechanics, contrasting the classical and quantum behaviors of particles encountering potential barriers. The script provides a comprehensive understanding of fundamental quantum mechanical principles through a clear and pedagogical approach.
Takeaways
- 📌 The process of measuring energy in quantum mechanics involves writing the wave function as a sum over functions associated with definite energy states.
- 📌 The probability of obtaining a certain energy E is given by the absolute value squared of the coefficient of the corresponding energy state function.
- 📌 Functions of definite energy, ψ_E(x), depend on the specific problem's potential energy and are found by solving the Schrödinger equation for that potential.
- 📌 In classical mechanics, energy depends on momentum and potential energy, which in turn depends on position, leading to different energy formulas for different problems.
- 📌 For a free particle (no potential), the Schrödinger equation solutions are exponential functions e^(ikx) and e^(-ikx), where k is related to the momentum p by p = ℏk.
- 📌 The energy of a particle in a one-dimensional box is quantized, with allowed energy levels given by E_n = (n^2 * π^2 * ℏ^2)/(2m * L^2), where n is a positive integer, m is the mass, and L is the length of the box.
- 📌 The wave function for a particle in a box that is confined by infinite potential walls must vanish at the walls, leading to quantized momentum and energy levels.
- 📌 The normalization condition ψ^(2)dx = 1 determines the coefficient B in the wave function, which is √(2/L) for a particle in a box.
- 📌 In quantum mechanics, particles cannot exist in a state of absolute rest (n=0) within a potential well due to the Heisenberg uncertainty principle.
- 📌 Quantum scattering involves solving the Schrödinger equation in different regions and applying boundary conditions to find the reflection and transmission coefficients.
- 📌 Even if a particle's energy is greater than a potential barrier, there is a non-zero probability of reflection due to the wave nature of particles in quantum mechanics.
- 📌 Quantum tunneling allows particles to have a non-zero probability of being found on the other side of an energy barrier that they classically could not surmount.
Q & A
What is the main concept being discussed in the transcript?
-The main concept discussed is quantum mechanics, specifically focusing on the behavior of particles in a potential well, the quantization of energy levels, and the wave function solutions to the Schrödinger equation.
What is the significance of the wave function ψ(x) in quantum mechanics?
-The wave function ψ(x) represents the state of a quantum particle. It is used to calculate the probability of finding a particle in a particular location and is central to understanding quantum phenomena such as energy level quantization.
How does the energy of a particle in a quantum system relate to its wave function?
-The energy of a particle in a quantum system is related to the square of the wave function's coefficient when the wave function is expressed as a sum over functions associated with definite energy states.
What is the Schrödinger equation, and what role does it play in determining the allowed energy states of a quantum system?
-The Schrödinger equation is a fundamental equation in quantum mechanics that describes how the quantum state of a physical system changes with time. It is used to determine the allowed energy states of a system by solving for the wave functions that correspond to definite energy levels.
What does it mean for energy levels to be quantized in a quantum system?
-Energy level quantization means that the energy states a quantum system can occupy are discrete and separate from one another, rather than continuous. This leads to the phenomenon where a system can only exist in certain well-defined energy states.
How does the potential energy function V(x) influence the solutions to the Schrödinger equation?
-The potential energy function V(x) is a key part of the Schrödinger equation. The specific form of V(x) determines the allowed wave functions and energy levels of the system. Different potentials lead to different sets of quantum states and energy spectra.
What is the relationship between the momentum of a particle and its energy in the context of a free particle (no potential)?
-For a free particle (no potential), the energy E is directly related to the momentum p of the particle by the equation E = p^2 / (2m), where m is the mass of the particle. This shows that the energy is a function of the square of the momentum.
What is the condition for the wave function of a particle in a one-dimensional box to be normalized, and why is this important?
-The wave function must be normalized so that the integral of the probability density (the square of the wave function) over all space is equal to 1. This ensures that the total probability of finding the particle somewhere in the box is 100%, which is a requirement in probability theory.
Why are negative integer values for n not allowed in the context of a particle in a box?
-Negative integer values for n are not allowed because they would result in the same wave function as their positive counterparts, just multiplied by -1, which does not represent a different physical state. Additionally, n=0 results in a wave function that identically vanishes, which is not a valid solution for a particle in a box.
What is the physical interpretation of the wave function's nodes in the context of a particle in a box?
-The nodes of the wave function, which are the points where the function equals zero, represent points of zero probability of finding the particle. In the context of a particle in a box, the nodes occur at the walls of the box, ensuring that the wave function vanishes at the boundaries as required by the physical conditions of the system.
How does the height of the potential barrier affect the transmission and reflection probabilities in quantum mechanics?
-The height of the potential barrier affects the transmission and reflection probabilities by altering the momentum of the particle within the barrier region. A higher barrier reduces the transmission probability (the likelihood of the particle passing through the barrier) and increases the reflection probability (the likelihood of the particle being reflected back).
Outlines
😀 Introduction to Quantum Measurement and Energy States
The professor begins by revisiting an incomplete topic from the previous class, emphasizing the importance of questions from students to understand their doubts. The main focus is on quantum measurement, specifically the process of measuring energy in a quantum system. The explanation involves wave functions and the necessity to find functions ψ_E(x) that represent states of definite energy. The probability of measuring a certain energy E is given by the absolute value squared of the corresponding coefficient. The process is analogous to measuring momentum, with the integral A_E representing the expectation value of the energy. The challenge is to determine these functions ψ_E(x), which depend on the potential energy and position in classical mechanics. Each problem has a unique formula for energy, and the Schrödinger equation must be solved for each potential to find the allowed energy states.
📚 Solving the Schrödinger Equation for Energy States
The professor elaborates on the process of solving the Schrödinger equation to find the functions of definite energy. The solutions are not straightforward and require mathematical solutions for each potential. The professor also discusses the example of a free particle with no potential, leading to the equation d^(2)ψ/dx^(2) = k^(2)ψ = 0, where k^(2) is derived from the energy. The solutions to this equation are exponential functions e^(ikx) and e^(-ikx), which are related to the momentum of the particle. The professor also connects the quantum mechanical energy to the classical mechanics concept, highlighting that the energy in quantum mechanics is quantized and depends on the momentum squared divided by twice the mass.
🔍 Quantum Mechanics and the Particle in a Ring
The professor explores the quantum mechanics of a particle in a ring, explaining how the particle's momentum is quantized and related to the energy. The allowed values of momentum are restricted to be multiples of 2Π/L, leading to quantized energy levels. This quantization results in the system absorbing and emitting light only at certain frequencies, which can be used to probe the energy differences in the system. The professor also discusses the hydrogen 21-centimeter line as an example of how atomic spectra can be used to understand the universe, including the motion of galaxies through redshift and blueshift observations.
🤔 Quantum Superposition and the 'Particle in a Box'
The professor delves into the concept of superposition in quantum mechanics, using the example of a particle in a ring that can exist in a state of both clockwise and counterclockwise momentum simultaneously. This is contrasted with classical mechanics, where the particle would have a definite momentum in only one direction. The professor also discusses the 'particle in a box' model, where the particle has a definite energy but an uncertain momentum, leading to the particle being in a state of 'limbo'. The probability of finding the particle moving in a particular direction is proportional to the square of the corresponding coefficient in the wave function.
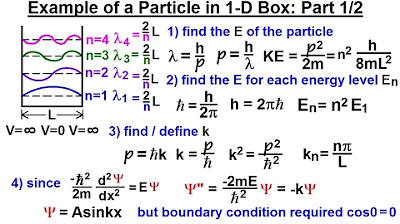
🧲 The Quantum Mechanics of a Particle in a One-Dimensional Box
The professor discusses the quantum mechanics of a particle confined to a one-dimensional box with infinitely high walls. The particle's wave function must be zero at the walls, leading to quantized energy levels and associated wave functions that are sine functions. The professor explains that the wave function must vanish at the boundaries, which quantizes the allowed values of the wave number k and, consequently, the energy levels. The energy levels are given by the formula ℏ^(2)Π^(2)/(2mL^(2)) times n^(2), where n is a positive integer, and the wave functions are given by Bsin(nΠx/L), with B being a normalization constant. The professor also addresses why the n=0 state is not allowed, as it would result in a trivial wave function that is identically zero.
🚧 The Behavior of Quantum Particles in a Finite Potential Well
The professor explores the behavior of quantum particles in a finite potential well, contrasting it with classical mechanics. In the classical view, a particle with energy less than the potential barrier would be confined, but in quantum mechanics, there is a non-zero probability of the particle tunneling through the barrier due to its wave-like nature. This phenomenon, known as barrier penetration, means that no barrier is completely impenetrable in quantum mechanics. The professor uses the example of alpha decay to illustrate this concept, where an alpha particle inside a nucleus can occasionally tunnel out, causing the nucleus to decay. The lecture concludes with the survival tip of repeatedly attempting to overcome a barrier, as there is always a small chance of success in quantum mechanics.
Mindmap
Keywords
💡Wave Function
💡Energy Measurement
💡Quantization
💡Schrödinger Equation
💡Potential Energy
💡Momentum
💡Particle in a Box
💡Normalization
💡Scattering
💡Complex Conjugate
💡Action of Measurement
Highlights
The wave function ψ(x) is used to predict the outcome of measuring a particle's energy in quantum mechanics.
Functions of definite energy, ψ_E(x), are states where the electron has a definite energy.
The probability of measuring a certain energy E is given by the absolute value squared of the corresponding coefficient in the sum over ψ_E(x).
The Schrödinger equation is central to finding functions of definite energy for a given potential V(x).
In classical mechanics, energy depends on momentum and potential energy, which in turn depends on position.
The functions of definite energy are not given by a simple formula and require solving the Schrödinger equation for each specific potential.
The simple example of a free particle (no potential) results in plane wave solutions representing states of definite momentum.
Quantization of energy levels is observed in systems such as an electron moving on a ring, where only certain momenta are allowed.
The quantized energy levels can be used to determine the absorption and emission spectra of a quantum system, such as the hydrogen 21 centimeter line.
The Bohr radius, a_0, is a length parameter that emerges from the Schrödinger equation for the hydrogen atom and represents the size of the orbit.
The particle in a box problem is used to illustrate the concept of energy quantization and the requirement for wave functions to vanish at the boundaries.
The wave function for a particle in a box is a sine function that vanishes at the boundaries, leading to quantized energy levels.
The normalization condition for the wave function determines the coefficient B and ensures the probability density integrates to one over space.
The Heisenberg Uncertainty Principle implies that a particle in a box cannot have a definite position and momentum simultaneously.
The concept of zero-point motion is introduced, indicating that particles in confined spaces, like nuclei, have a minimum kinetic energy.
Scattering in quantum mechanics is different from classical mechanics, with particles having a probability of being reflected even if their energy exceeds the barrier.
Quantum tunneling allows particles to penetrate barriers and be found on the other side even when their energy is insufficient to classically surmount the barrier.
The phenomenon of alpha decay is an example of quantum tunneling, where an alpha particle can escape the nucleus despite the potential barrier.
Transcripts
Browse More Related Video

22. Quantum mechanics IV: Measurement theory, states of definite energy
Physics - Ch 66 Ch 4 Quantum Mechanics: Schrodinger Eqn (19 of 92) Particle in 1-D Box: Example 1/2
21. Quantum Mechanics III

Lecture 10 | The Theoretical Minimum
20. Quantum Mechanics II

25. Quantum Mechanics VII: Summary of postulates and special topics
5.0 / 5 (0 votes)
Thanks for rating: