Standard Error of the Mean

The Organic Chemistry Tutor
20 Sept 201910:45
EducationalLearning
32 Likes 10 Comments

TLDRThis video explains how to calculate the standard error of the mean for a sample. It provides two examples, one with a small sample size of 5 students' ages, walking through the full calculation of the standard deviation and standard error. The second uses a larger university sample to demonstrate that as the sample size increases, the standard error decreases, meaning the sample mean approximates the actual population mean more accurately. It visually represents this on a graph showing a narrower, taller normal distribution. Overall it aims to provide instruction on calculating standard error and convey why a larger sample size gives more precise results.

Takeaways
  • 😀 The standard deviation measures how spread out data is from the mean
  • 📊 To find the standard error, divide the standard deviation by the square root of the sample size
  • 🍎 Calculate the mean and standard deviation of a sample to find the standard error
  • 🔢 The standard error gets smaller as the sample size increases
  • 📈 The standard error represents variability in the sampling distribution of the mean
  • 😮 A small standard error means the sample mean is likely close to the population mean
  • 🎓 Examples are shown calculating standard error for a sample of student ages
  • 📊 The standard deviation of a population is represented by sigma σ
  • 🌡️ Increasing sample size narrows the sampling distribution and decreases standard error
  • 📉 Less standard error means the sample mean approximates the population mean more accurately
Q & A

  • What is the difference between the standard deviation of a sample and the standard deviation of a population?

    -The standard deviation of a sample is represented by the symbol s and is calculated using the sample data. The standard deviation of a population is represented by the symbol σ and is calculated using data on the entire population.

  • What is the formula used to calculate the standard error of the mean?

    -The formula is: Standard Error = Standard Deviation / Square Root of Sample Size (n)

  • Why does the standard error decrease when the sample size increases?

    -Because the standard error formula includes the square root of the sample size n in the denominator. So as n increases, the denominator increases, resulting in a smaller standard error.

  • What is the difference between the standard deviation and standard error?

    -The standard deviation measures the amount of variability in a data set. The standard error measures how precisely you have estimated the true population mean from your sample data.

  • What is meant by the sampling distribution of the mean?

    -It is the distribution of means from many samples drawn from the same population. Its shape follows a normal distribution centered around the population mean.

  • How does increasing the sample size impact the sampling distribution of the mean?

    -Increasing the sample size makes the sampling distribution narrower and taller in height. This indicates there is less variability around estimating the true population mean.

  • Why does calculating the standard deviation for a large data set take a long time?

    -Because you have to find the difference between each data point and the mean, square each difference, sum them up, divide by n-1, and take the square root. More data points means more computations.

  • What are some ways to visually represent differences in standard error?

    -You can plot sampling distributions, where decreased standard error is shown through a distribution that is narrower and taller in shape.

  • What impacts whether you calculate standard error or standard deviation?

    -If your data comes from a sample, you calculate the standard deviation. If it's population data, you use the standard deviation to determine standard error.

  • How can you decrease standard error?

    -By increasing the sample size n. A larger sample leads to a more precise estimate of the true population parameter.

Outlines
00:00
😀 Calculating Standard Deviation and Standard Error

This paragraph provides an example of calculating the standard deviation and standard error for a sample of 5 student ages. The steps to calculate standard deviation are shown, resulting in a value of 3.114. Then the standard error is calculated by dividing the standard deviation by the square root of the sample size n, which is 5, giving a standard error of 1.393.

05:00
😀 Calculating Standard Error Using Population Parameters

This paragraph provides an example of calculating standard error using the known population standard deviation rather than a sample standard deviation. With a population SD of 0.8 and sample sizes of 25 and 100 students, the standard error is calculated as the population SD divided by the square root of n. This shows how increasing sample size decreases standard error.

10:02
😀 Visualizing the Effect of Sample Size on Standard Error

This paragraph provides a visual graph to represent the effect of sample size on standard error and the sampling distribution. As sample size increases, the graph becomes narrower and taller, representing the decrease in standard error and more accurate approximation of the population mean.

Mindmap
Keywords
💡standard deviation
The standard deviation is a measure of how spread out a set of numbers are from their average value. It quantifies the amount of variation in the sample. In the video, standard deviation is used to calculate the standard error which determines the accuracy of the sample mean as an estimate of the population mean.
💡standard error
The standard error is a measure of how precisely the sample represents the overall population. It decreases as the sample size increases, meaning larger sample sizes can estimate the population mean more accurately. Calculating the standard error is a key focus of the video.
💡sample size
The sample size refers to the number of observations that are included in a sample from a larger population. The video demonstrates that as the sample size increases, the standard error decreases, meaning there is less variability around estimating the true population parameter.
💡mean
The mean refers to the average value of a dataset. The video focuses on using sample means to estimate population means and how the standard error determines the precision of this estimation.
💡population
A population includes all possible members of a group while a sample only includes some members selected from the larger population. The video discusses estimating overall population values (like the mean) based on statistics calculated from a smaller sample.
💡sampling distribution
The sampling distribution depicts the distribution of a statistic (like the mean) if we took many samples from the same population. The video visually shows how this distribution changes with larger sample sizes, becoming narrower around the true population parameter.
💡variance
Variance refers to how far each number in a distribution lies from the mean value. A higher variance means the values are more spread out. The standard error and standard deviation quantify this variance in different ways.
💡precision
Precision refers to how close an estimate is expected to be to the true value of the quantity being estimated. The video discussion centers around precision in estimating the population mean based on the standard error of a sample mean.
💡hypothesis testing
While not explicitly mentioned, conceptually the video relates to hypothesis testing because the standard error determines how precisely the sample mean estimates the assumed population mean value.
💡confidence interval
A confidence interval provides a range of likely values for a population parameter based on a sample statistic. Though not mentioned directly, the video relates to confidence intervals around a sample mean using the standard error.
Highlights

Calculate the standard deviation of sample ages to find the standard error

Standard error formula: Standard deviation of sample divided by square root of sample size n

As sample size increases, standard error decreases, giving more accurate mean approximation

Population standard deviation used to calculate standard error if known, vs sample standard deviation

Visualize standard error decreasing in sampling distribution graphs as sample size rises

Square root relationship between sample size and standard error causes inverse proportionality

Finding differences from mean, squaring, and summing is done to calculate sample standard deviation

Smaller sample used due to computational complexity of sample standard deviation formula

Mean of ages calculated first before finding sample standard deviation

Each age value substituted in full formula shown for sample standard deviation calculation

Population vs sample standard deviation used correctly in respective formulae

Standard error gives interval estimate of how precisely sample mean estimates population mean

Decreasing standard error improves accuracy of conclusions drawn from sample statistics

Smaller standard error corresponds to narrower sampling distribution centered on true mean

Standard error of 0.16 with sample size 25 decreased to 0.08 with sample size 100

Quadrupling sample size approximately halves the standard error value due to inverse relationship

Transcripts

Browse More Related Video

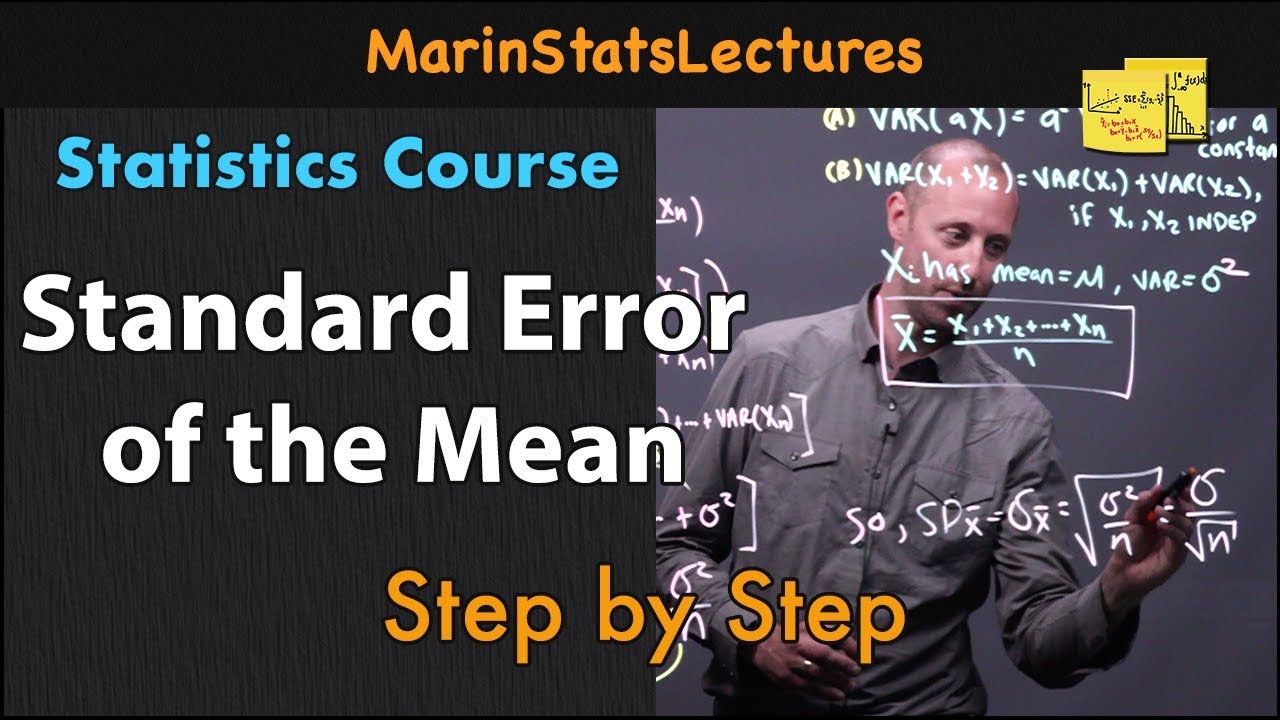
Standard Error of the Mean: Concept and Formula | Statistics Tutorial #6 | MarinStatsLectures

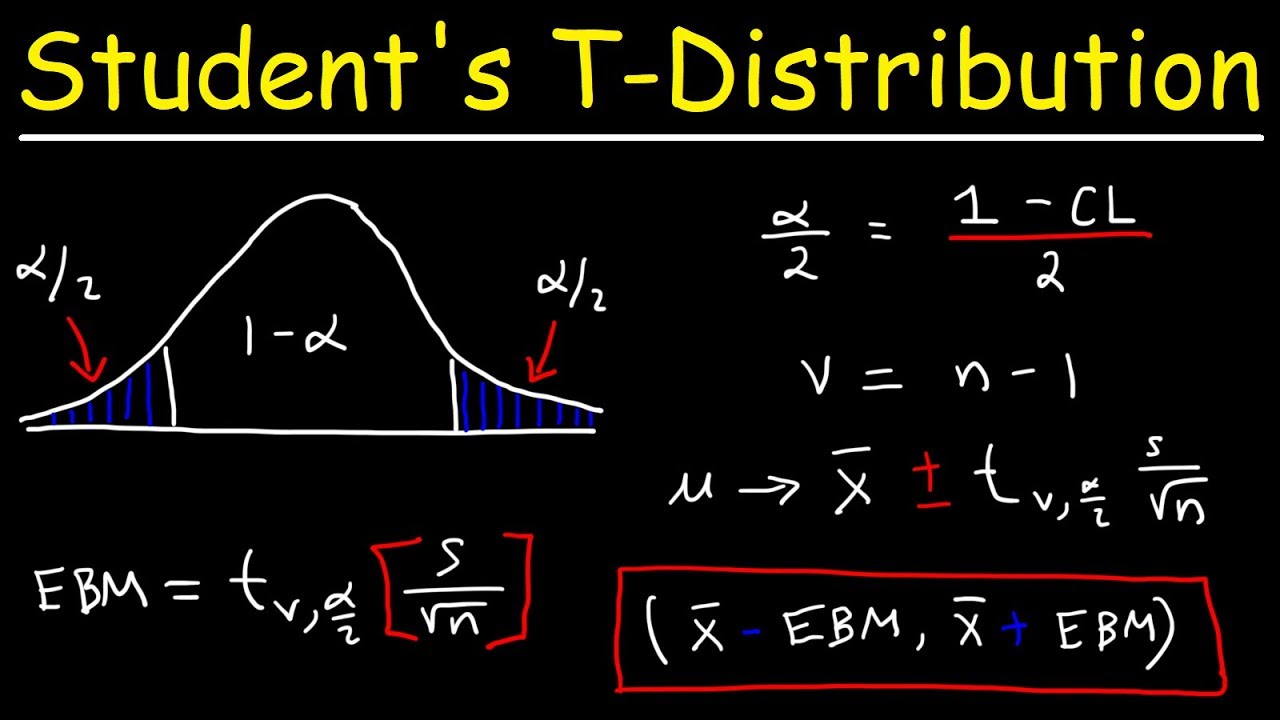
Student's T Distribution - Confidence Intervals & Margin of Error

Sampling distribution of the sample mean 2 | Probability and Statistics | Khan Academy

Standard Error (of the sample mean) | Sampling | Confidence Intervals | Proportions

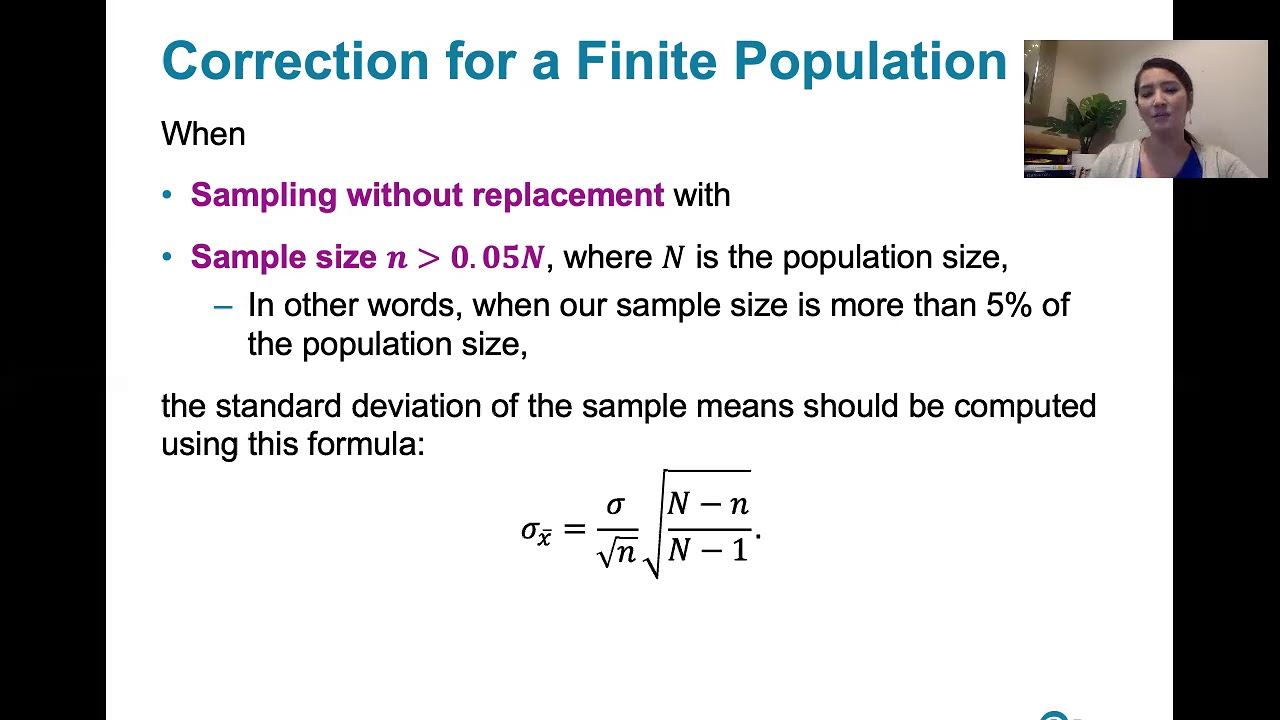
6.4.4 The Central Limit Theorem - Finite Population Correction Factor

Standard error of the mean | Inferential statistics | Probability and Statistics | Khan Academy

Rate This

5.0 / 5 (0 votes)

Thanks for rating: