Physics - Ch 66 Ch 4 Quantum Mechanics: Schrodinger Eqn (19 of 92) Particle in 1-D Box: Example 1/2

Michel van Biezen
15 Feb 201710:24
EducationalLearning
32 Likes 10 Comments

TLDRThe lecture delves into the quantum mechanics concept of a particle within a one-dimensional box, exploring its energy levels and wave functions. It explains how the wavelength of the particle is related to its energy levels and the box's length, leading to the quantization of energy. The de Broglie wave equation is used to derive the relationship between momentum, wavelength, and energy. The energy levels are expressed as a function of the quantum number 'n', and the wave number 'K' is defined. The lecture concludes with the development of a wave equation for the particle and the identification of the correct wave function that satisfies the boundary conditions, setting the stage for understanding particle probability distributions within the box.

Takeaways
  • 📝 The lecture focuses on deriving the equation for a particle within a one-dimensional box, specifically addressing the first four energy levels (N=1, 2, 3, 4).
  • 🌊 The concept of standing waves on a string is used as an analogy to explain the wavelengths (λ1, λ2, λ3, λ4) corresponding to each energy level.
  • 🔢 Wavelengths are calculated as λ = 2L/N, where L is the length of the box and N is the quantum number representing the energy level.
  • 💡 The kinetic energy of the particle is quantized and depends on the square of the quantum number (N^2), the mass, and the box's length (L).
  • 🌐 The de Broglie wavelength equation is used to express the particle's wavelength in terms of its momentum and Planck's constant (h).
  • 🔄 The kinetic energy equation is derived by replacing momentum (p) with h/λ and is expressed as (h^2/2mL^2) * N^2.
  • 🏷 The energy levels are defined as E_n = N^2 * (h^2/8mL^2), where E_n represents the energy for each quantum level n.
  • 📈 The wave number (K) for the particle is determined as K = Nπ/L, based on the energy level and the box's length.
  • 🌊 The wave function for the particle in a one-dimensional box is represented by a sine function of Kx, due to boundary conditions requiring the wave function to be zero at x=0 and x=L.
  • 🌌 The probability function, which describes the likelihood of finding the particle at different points within the box, is derived from the wave function through normalization.
  • 🔍 The entire process must be re-evaluated for different physical situations, as the constants and variables are specific to the one-dimensional box scenario discussed.
Q & A

  • What is the main topic of the lecture?

    -The main topic of the lecture is the derivation and explanation of the equation describing a particle within a one-dimensional box, focusing on the first four energy levels.

  • How are the wavelengths lambda related to the length of the box (L) for the first four energy levels?

    -For the first four energy levels, the wavelengths lambda are related to the length of the box (L) as follows: lambda 1 = 2L, lambda 2 = L, lambda 3 = (2/3)L, and lambda 4 = (1/2)L.

  • What is the general formula for the wavelength in terms of the quantum number n and the length of the box (L)?

    -The general formula for the wavelength in terms of the quantum number n and the length of the box (L) is lambda = (2/n) * L.

  • How is the kinetic energy of the particle inside the box quantized?

    -The kinetic energy of the particle is quantized and depends on n^2, where n is the quantum number representing the energy level of the box.

  • What is the expression for the energy of a particle at a particular energy level?

    -The expression for the energy of a particle at a particular energy level is E_n = (n^2 * pi^2 * ħ^2) / (2m * L^2), where n is the energy level, ħ is the reduced Planck constant, m is the mass of the particle, and L is the length of the box.

  • How is the wave number (K) defined in the context of the one-dimensional box?

    -The wave number (K) is defined as K = n * pi / L, where n is the quantum number representing the energy level.

  • What is the condition that the wave function must satisfy at the boundaries of the box?

    -The wave function must satisfy the boundary condition of being zero at the boundaries of the box, which means it equals zero when x = 0 or x = L.

  • Why can't the cosine function be used to represent the wave function for a particle in a one-dimensional box?

    -The cosine function cannot be used to represent the wave function because it does not satisfy the boundary condition of the wave function being zero at the boundaries (x = 0 or x = L), as the cosine of zero is not zero.

  • What type of function is suggested to represent the wave function for a particle in a one-dimensional box?

    -The sine function of KX is suggested to represent the wave function for a particle in a one-dimensional box, as it satisfies the required boundary conditions.

  • What is the next step after finding the wave function for the particle in the box?

    -The next step after finding the wave function is to determine the value of the normalization constant 'a' using the normalization condition, which ensures the total probability of finding the particle within the box is equal to one.

  • How is the probability function derived from the wave function?

    -The probability function is derived from the wave function by taking the square of the wave function's magnitude, which gives the probability density of finding the particle at a particular point within the one-dimensional box.

Outlines
00:00
📚 Quantum Mechanics: Particle in a One-Dimensional Box

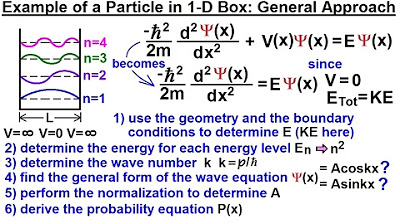
This paragraph introduces the concept of a particle within a one-dimensional box and discusses the first four energy levels (N=1, 2, 3, 4). It explains how the wavelength (lambda) can be calculated for each energy level and how it relates to the length of the box. The de Broglie wave equation is used to express the particle's momentum and kinetic energy, leading to the quantization of energy levels inside the box. The energy of the particle is found to depend on the square of the quantum number (N) and the box's length (L), with specific formulas provided for each energy level.

05:02
🌐 Wave Number and Wave Equation for a One-Dimensional Box

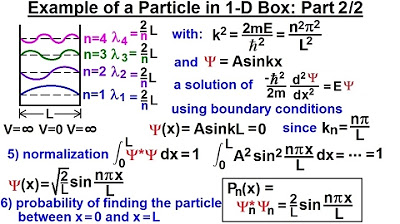
The second paragraph delves into the calculation of the wave number (K) and its relationship with the energy levels. It starts by defining K using the momentum of a photon and then derives an expression for K based on the energy levels of the box. The paragraph then moves on to describe the wave equation for a particle in a one-dimensional box, highlighting the connection between the second derivative of the wave function and the wave number squared. Two potential wave functions (sine and cosine of KX) are considered, but the cosine function is ruled out due to boundary condition requirements. The sine function is identified as the viable solution, and the process of normalization to find the value of 'a' is mentioned, leading to the probability function that describes the location of the particle within the box.

10:02
🎓 Summary of Quantum Mechanics Concepts for a One-Dimensional Box

In this concluding paragraph, the focus is on summarizing the process of utilizing the Schrödinger equation to derive the wave equation and the probability function for a particle in a one-dimensional box. The emphasis is on the step-by-step approach to understanding quantum mechanics within this specific context, encouraging the application of the outlined steps to gain a comprehensive understanding of the particle's behavior in relation to its energy levels and wave functions.

Mindmap
Keywords
💡Particle in a one-dimensional box
This concept refers to a quantum mechanical model where a particle is confined within a one-dimensional box, also known as an infinite potential well. It is used to illustrate the principles of quantum mechanics, such as quantization of energy levels and wave-particle duality. In the video, the speaker discusses the mathematical representation of this system, including the calculation of energy levels and wave functions for a particle confined within such a box.
💡Quantum number (n)
In quantum mechanics, the quantum number is a value that defines the unique quantum state of a particle in a system. In the context of the one-dimensional box, the quantum number (n) represents the energy level of the particle, with higher values of n corresponding to higher energy states. The speaker explains how the wavelength and energy of the particle are dependent on the quantum number.
💡Wavelength (lambda)
Wavelength is the spatial period of a wave, and in the context of the one-dimensional box model, it represents the distance over which the particle's wave function repeats. The wavelength is directly related to the energy level of the particle, with higher energy levels corresponding to longer wavelengths. The speaker calculates the wavelength for different energy levels and explains how it is determined by the quantum number and the box's length.
💡Energy levels
In quantum mechanics, energy levels are the discrete values of energy that a quantum system can have. For a particle in a one-dimensional box, the energy levels are quantized and depend on the square of the quantum number (n). The speaker explains how to calculate these energy levels and how they relate to the particle's wave function.
💡de Broglie wave equation
The de Broglie wave equation relates the wavelength of a particle's wave to its momentum, stating that the wavelength (lambda) is equal to Planck's constant (h) divided by the particle's momentum (p). This equation is fundamental in understanding the wave-particle duality of matter and is used in the video to derive the relationship between the particle's momentum and the box's dimensions.
💡Kinetic energy
Kinetic energy is the energy a particle possesses due to its motion. In the context of the one-dimensional box model, the kinetic energy is the only form of energy considered, as there is no potential energy within the box. The speaker calculates the kinetic energy of the particle using the relationship between momentum and wavelength, and further relates it to the energy levels.
💡Schrodinger equation
The Schrodinger equation is a fundamental equation in quantum mechanics that describes how the quantum state of a physical system changes with time. In the video, the time-independent form of the Schrodinger equation is used to derive the wave function of the particle in the one-dimensional box, assuming that the potential energy is zero inside the box.
💡Wave function
A wave function is a mathematical function that describes the quantum state of a particle. It provides information about the probability of finding the particle in a particular location. In the video, the speaker derives the wave function for a particle in a one-dimensional box, which must satisfy boundary conditions and the Schrodinger equation.
💡Normalization
Normalization is the process of adjusting a function so that its integral over all space equals one. In quantum mechanics, this ensures that the probability of finding the particle anywhere in the allowed space is 100%. The speaker mentions normalization in the context of finding the constant 'a' in the wave function for the particle in the box.
💡Probability function
The probability function, also known as the probability density function, gives the probability distribution of finding a particle in a particular state or location. In the video, the speaker aims to find this function for a particle in a one-dimensional box, which will describe where the particle is most likely to be found based on its energy level.
💡Boundary conditions
Boundary conditions are the constraints applied to the solution of a differential equation at the edges of the domain. In the context of the one-dimensional box, the wave function must be zero at the boundaries (the walls of the box). The speaker discusses how the choice of wave function is influenced by these conditions, ruling out the cosine function because it does not satisfy the requirement that the wave function be zero at the boundaries.
Highlights

The lecture introduces the concept of a particle within a one-dimensional box and its corresponding energy levels.

Four specific energy levels (N=1, N=2, N=3, N=4) are discussed with their respective wavelengths (λ1, λ2, λ3, λ4) related to the box's length.

The wavelength λ is derived to be 2/n times the length of the box, L, for each energy level.

The kinetic energy of the particle is quantized and depends on the square of the quantum number n.

The energy levels are defined as E_sub_n, with a formula provided for calculating the energy of each level.

The wave number K is defined and related to the momentum of a photon, with a formula for K in terms of the energy level.

The wave number K is expressed as nπ/L, linking it directly to the energy level of the one-dimensional box.

A wave equation for the particle in the one-dimensional box is derived, relating the second derivative of the wave function to -K^2 times the wave function.

The sine and cosine functions of KX are considered as potential solutions for the wave function, but the cosine function is dismissed due to boundary condition requirements.

The lecture concludes that the sine function of KX is the viable solution for representing the particle's wave function within the one-dimensional box.

Normalization is mentioned as a method to determine the value of a in the wave function.

The probability function, which describes the likelihood of finding the particle at different points within the box, is discussed as the final step in the analysis.

The process of deriving the wave equation and probability function for a particle in a one-dimensional box is outlined as a series of steps.

The lecture emphasizes the uniqueness of these calculations for the specific case of a one-dimensional box and notes that different situations require re-evaluation.

The de Broglie wave equation is used as a starting point to solve for the particle's momentum and subsequently its kinetic energy.

The potential energy inside the box is zero, and the kinetic energy is the total energy of the particle in the box.

The energy levels are directly related to the square of the quantum number n, indicating a quantization of energy.

Transcripts

Browse More Related Video

Physics - Ch 66 Ch 4 Quantum Mechanics: Schrodinger Eqn (20 of 92) Particle in 1-D Box: Example 2/2

Physics - Ch 66 Ch 4 Quantum Mechanics: Schrodinger Eqn (18 of 92) Particle in 1-D Box: Gen. Appr.

22. Quantum mechanics IV: Measurement theory, states of definite energy

23. Quantum Mechanics V: Particle in a Box

EASIEST question on the International Physics Olympiad?

21. Quantum Mechanics III

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
QuantumMechanicsParticlePhysicsOneDimensionalBoxEnergyLevelsWaveFunctionsDeBroglieEquationSchrodingerEquationQuantizationProbabilityDensityPhysicsLecture