Vectors - Basic Introduction - Physics
TLDRThis video script delves into the concept of vectors, distinguishing them from scalars by their possession of both magnitude and direction. It explains how displacement, velocity, and acceleration are vectors, while mass and temperature are scalars. The video also covers how to calculate the x and y components of a force vector using trigonometry, providing a step-by-step guide on applying sine and cosine functions to find these components. The use of unit vectors to express the force vector in component form is also introduced, offering a comprehensive understanding of vector analysis.
Takeaways
- 📌 Vectors are quantities with both magnitude and direction, unlike scalars which have only magnitude.
- 📐 Examples of vectors include displacement, velocity, acceleration, and force, while examples of scalars include temperature and mass.
- 🏃♂️ Distance is a scalar, but displacement is a vector when direction is specified, such as running 45 meters east.
- 🔢 Speed is a scalar quantity that indicates how fast an object is moving, whereas velocity is a vector that includes direction.
- 🚀 Acceleration is a vector that describes the rate of change of velocity, including changes in direction.
- 📐 To find the components of a force vector, use trigonometric functions like sine and cosine based on the angle from the positive x-axis.
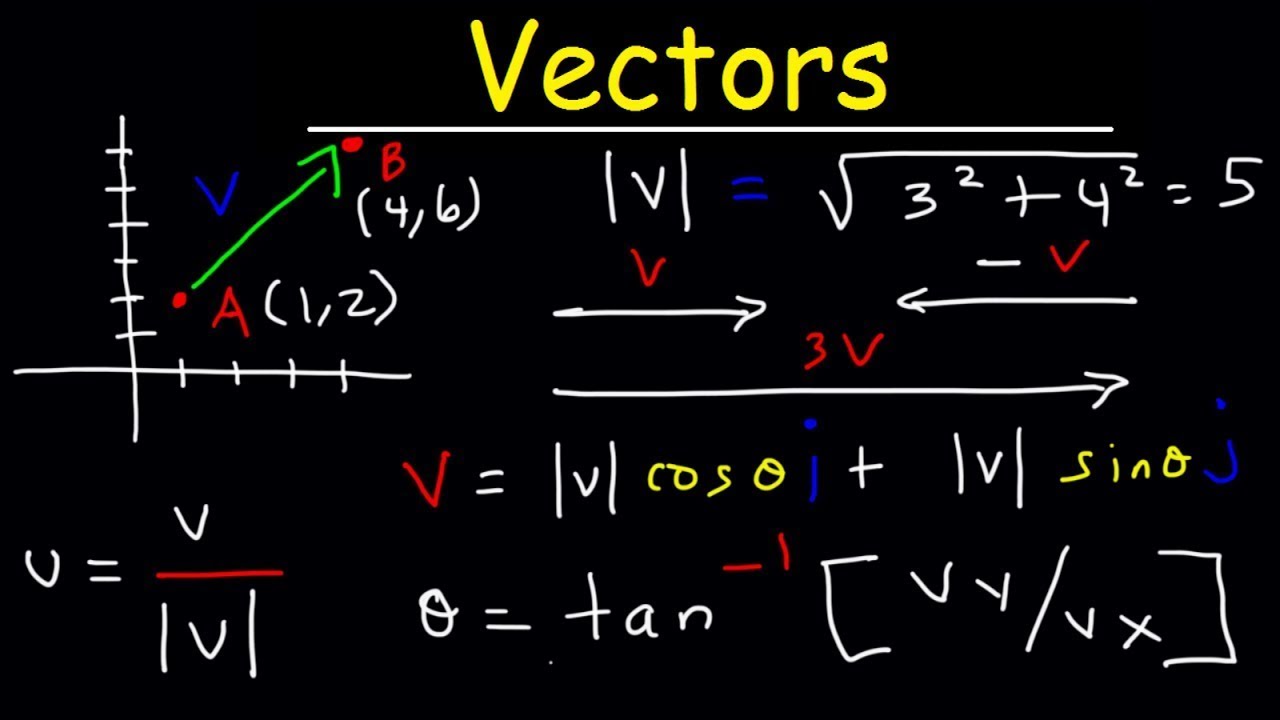
- 🧭 The sine of an angle (θ) gives the y-component (opposite side) of a vector, and the cosine of an angle gives the x-component (adjacent side).
- 🔄 The tangent of an angle (θ) can be used to find the angle when given the x and y components of a vector.
- 📐 The magnitude of a vector can be calculated using the Pythagorean theorem: √(x-component² + y-component²).
- 📈 When expressing vectors, unit vectors (i, j, k) can be used to represent the x, y, and z components respectively.
- 🔍 The script provides a method for calculating the x and y components of a force vector with a given magnitude and direction.
Q & A
What is the main topic of the video?
-The main topic of the video is vectors, specifically distinguishing between scalar and vector quantities.
What is a scalar quantity?
-A scalar quantity is something that has magnitude but no direction, such as temperature.
How is a vector quantity different from a scalar quantity?
-A vector quantity has both magnitude and direction, which allows it to be described in terms of its size and the direction it points in.
Which of the given quantities in the video is not a vector?
-Mass is not a vector; it is a scalar quantity because it has magnitude but no direction.
What is the difference between distance and displacement?
-Distance is a scalar quantity that describes the length of a path without direction, while displacement is a vector quantity that includes both the length of the path and the direction.
How is velocity related to speed?
-Velocity is to speed as displacement is to distance. Velocity is speed with direction, providing information on both how fast an object is moving and the direction of its movement.
What are the components of a force vector?
-A force vector can be broken down into its x and y components, which represent the force's magnitude in the horizontal and vertical directions, respectively.
How can you calculate the x and y components of a force vector?
-You can use trigonometric functions like sine and cosine to calculate the x and y components of a force vector. The x component is the magnitude of the force times the cosine of the angle, and the y component is the magnitude times the sine of the angle.
What is a unit vector?
-A unit vector is a vector with a magnitude of one, used to represent direction in a coordinate system. The standard unit vectors are i (along the x-axis), j (along the y-axis), and k (along the z-axis).
How can you express a vector using unit vectors?
-A vector can be expressed as a linear combination of its components using the corresponding unit vectors. For example, a force vector F can be written as Fx times i plus Fy times j.
What is the Pythagorean theorem's application in vector calculations?
-The Pythagorean theorem is used to calculate the magnitude of a vector when its x and y components are known. It states that the square of the vector's magnitude is equal to the sum of the squares of its x and y components.
Outlines
📏 Understanding Scalars and Vectors
This paragraph introduces the fundamental concepts of scalar and vector quantities. Scalars, such as temperature, possess only magnitude without direction. In contrast, vectors like force include both magnitude and direction. The video emphasizes the importance of distinguishing between scalars and vectors, using displacement and velocity as examples of vector quantities because they incorporate direction along with magnitude. It also clarifies that distance and speed are scalars, while acceleration is a vector, similar to force. The main point is to recognize that a vector has both magnitude and direction, which is crucial for solving the given problem.
📐 Calculating Vector Components
This segment delves into the process of calculating the x and y components of a force vector. It begins by establishing the definitions of sine and cosine in relation to the angle θ, which is the angle the force vector makes with the x-axis. The paragraph then uses trigonometric relationships to derive the formulas for the x and y components of the force vector. It explains how to use these formulas to calculate the magnitude of the x and y components given the magnitude of the force and the angle θ. The example provided uses a force vector with a magnitude of 100 newtons at an angle of 30 degrees above the x-axis, showing how to apply the formulas to find the x and y components.
📐 Expressing Vectors with Unit Vectors
The final paragraph discusses the concept of unit vectors and how they can be used to express any vector in component form. It explains that a unit vector has a magnitude of one and is represented by i, j, and k along the x, y, and z axes, respectively. The video then demonstrates how to express the calculated x and y components of the force vector using unit vectors. By doing so, the force vector is represented as a linear combination of the unit vectors i and j, with the x and y components as coefficients. This method provides an alternative way to represent vectors, which can be particularly useful in three-dimensional space and for further vector analysis.
Mindmap
Keywords
💡vectors
💡scalar quantities
💡magnitude
💡direction
💡displacement
💡velocity
💡acceleration
💡mass
💡components
💡trigonometry
💡unit vectors
💡Pythagorean theorem
Highlights
The main topic of the video is vectors and the distinction between scalar and vector quantities.
Displacement, velocity, and acceleration are examples of vector quantities, while mass and temperature are scalar quantities.
A scalar quantity has magnitude but no direction, such as temperature, whereas a vector quantity has both magnitude and direction, like force.
Force can be described using magnitude and direction, for example, 100 newtons at a 30-degree angle above the x-axis.
Distance is a scalar quantity, but displacement is a vector when direction is specified, like running 45 meters east.
Speed is a scalar quantity, while velocity is a vector quantity because it includes direction.
Acceleration is a vector quantity that describes how quickly the velocity of an object changes.
The correct answer to the question is that mass is a scalar quantity, not a vector.
The problem involves calculating the x and y components of a force vector with a given magnitude and direction.
Trigonometry, specifically the SOHCAHTOA mnemonic, is used to calculate vector components.
The sine of an angle (θ) gives the y-component of a vector, while the cosine gives the x-component.
The tangent of an angle (θ) can be used to find the angle when the vector components are known.
The Pythagorean theorem is applied to find the magnitude of a vector when the x and y components are known.
Unit vectors i, j, and k are introduced as a way to express vectors in component form along the x, y, and z axes.
The force vector's x-component is calculated as 86.6 newtons using the cosine of the angle.
The force vector's y-component is calculated as 50 newtons using the sine of the angle.
The final expression of the force vector uses standard unit vectors i and j for the x and y components.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: