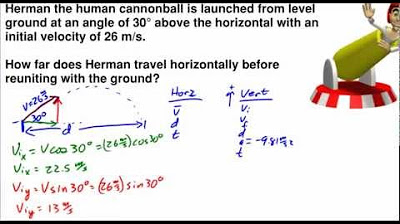
2D Kinematics Problem Solving Examples
TLDRThe video script is an in-depth walkthrough of solving 2D kinematics problems, focusing on a diver jumping off a board and a basketball being thrown. It emphasizes the importance of breaking down the problem into X and Y components, using given information effectively, and applying kinematic equations to find unknowns such as velocity and height at various points in the trajectory. The script also covers calculating the time taken to reach the highest point and the horizontal distance traveled. The process is methodical and educational, aiming to improve problem-solving skills in physics.
Takeaways
- 📚 The script is a lesson on solving 2D kinematics problems, focusing on understanding and applying problem-solving strategies.
- 🤸♀️ A diver jumping from a 4.5-meter high diving board is used as an example to illustrate the process of breaking down the problem into X and Y components.
- 📐 The initial velocity (V1) is given as 3.4 m/s at a 75-degree angle, and the task is to find the final velocity (V2) after the diver lands in the water.
- 🖌️ The讲师 emphasizes the importance of separating the given information into X and Y components to simplify the problem-solving process.
- 📈 The讲师 uses the sohcahtoa mnemonic to find the horizontal (V1x) and vertical (V1y) components of the initial velocity.
- 🚫 The acceleration in the X direction is 0 due to no horizontal force acting on the diver, indicating constant horizontal velocity throughout the jump.
- 📊 The讲师 applies kinematic equations to determine the time it takes for the diver to reach the water and the height and horizontal distance traveled by the basketball when thrown at an angle.
- 🏀 Another example involves a basketball thrown at an initial velocity and angle, with the goal of finding the time to reach the highest point, the maximum height, and the horizontal distance traveled.
- 🎯 The讲师 demonstrates how to use the Pythagorean theorem to find the magnitude of the final velocity and the angle of descent.
- 💌 A final scenario describes a note dropped from a balcony, with the讲师 working backwards to find the initial velocity and angle of the note based on the known horizontal distance and height of the fall.
- 📝 The讲师's approach involves a step-by-step breakdown of the problem, emphasizing the importance of understanding the direction and components of velocity and acceleration in 2D motion.
Q & A
What is the main focus of the transcript?
-The main focus of the transcript is to practice problem-solving strategies with 2D kinematics problems, specifically those involving diving and throwing objects.
What is the initial height of the diving board from which the diver jumps?
-The initial height of the diving board from which the diver jumps is 4.5 meters above the ground or water.
What is the initial velocity of the diver as given in the problem?
-The initial velocity of the diver is given as 3.4 meters per second.
At what angle does the diver jump from the diving board?
-The diver jumps from the diving board at an angle of 75 degrees.
How is the given information split for problem-solving in 2D kinematics?
-The given information is split into X and Y components, which simplifies the problem-solving process by separating the horizontal and vertical aspects of the motion.
What is the acceleration in the X-direction for the diver?
-The acceleration in the X-direction for the diver is 0, as there is no horizontal force acting on the diver during the jump.
How is the final velocity (V2) of the diver in the X-direction determined?
-The final velocity (V2) in the X-direction is determined to be equal to the initial velocity (V1) since there is no acceleration in the X-direction.
What is the formula used to find the time it takes for the basketball to reach its highest point?
-The formula used to find the time to reach the highest point is V2y = V1y + a*(ΔT), where V2y is 0 (at the highest point), V1y is the initial vertical velocity, a is the acceleration due to gravity, and ΔT is the time.
What is the horizontal distance the note travels before reaching the ground?
-The horizontal distance the note travels before reaching the ground is 3.4 meters.
How long does it take for the note to fall from the balcony to the ground?
-It takes 1.2 seconds for the note to fall from the balcony to the ground.
What is the initial velocity of the note in the Y-direction?
-The initial velocity of the note in the Y-direction is approximately -3.45 m/s, indicating that the note was thrown downward.
What is the total initial velocity of the note and the angle of its trajectory?
-The total initial velocity of the note is approximately 4.5 m/s, and the angle of its trajectory is about 39 degrees from the horizontal.
Outlines
🤔 Problem-Solving Strategies for 2D Kinematics
The paragraph introduces the topic of 2D kinematics problems, focusing on a specific scenario where a diver jumps from a 4.5-meter high diving board. The speaker emphasizes the importance of breaking down the problem into X and Y components to simplify the process. The given information includes the diver's initial velocity (3.4 m/s at a 75-degree angle) and the need to find the final velocity (V2). The speaker begins by analyzing the X-component of the initial velocity (V1x) using trigonometric relationships and the acceleration due to gravity (9.8 m/s²), which is directed downwards and affects only the Y-component.
📐 Solving for Final Velocity in 2D Kinematics
This paragraph continues the discussion on solving 2D kinematics problems, specifically focusing on finding the final velocity (V2) for the diver's jump. The speaker explains the process of using kinematic equations to determine the X and Y components of the final velocity. The X-component (V2x) remains constant throughout the jump as there is no acceleration in the horizontal direction. For the Y-component (V2y), the speaker uses the kinematic equation involving initial velocity, acceleration, and displacement to calculate the final velocity. The process involves squaring and taking the square root of the calculated values, resulting in a positive or negative value that corresponds to the direction of motion.
🏀 Basketball Trajectory and Time to Peak
The paragraph shifts focus to a basketball thrown at an initial velocity and angle, aiming to find the time to reach the highest point, the maximum height, and the horizontal distance traveled before reaching that height. The speaker sets up the problem by identifying the initial conditions (zero height and horizontal position) and the known variables (initial velocity and angle of projection). The speaker then uses kinematic equations to solve for the time it takes to reach the peak of the trajectory, the maximum height the ball reaches, and the horizontal distance traveled during that time.
💌 Note Dropping from a Balcony
In this paragraph, the speaker describes a scenario where a note is dropped from a balcony to reach someone below. The speaker outlines the known conditions, such as the height of the balcony and the horizontal distance the note travels before hitting the ground. The speaker uses this information to work backwards to determine the initial velocity of the note, both in the horizontal (V1x) and vertical (V1y) directions. The process involves using kinematic equations and the given time of fall to calculate the unknown initial velocity components. The speaker then combines these components to find the total initial velocity and its direction.
Mindmap
Keywords
💡2D Kinematics
💡Initial Velocity (V1)
💡Acceleration
💡Trajectory
💡SoH-CaH-TOA
💡Pythagorean Theorem
💡Vector
💡Highest Point
💡Horizontal Distance
💡Time of Flight
Highlights
The problem-solving session focuses on 2D kinematics problems, which are typically more complex than their 1D counterparts.
A diver jumps from a 4.5-meter high diving board with an initial velocity of 3.4 m/s at a 75-degree angle.
The importance of splitting given information into X and Y components for easier problem-solving in 2D kinematics.
The initial velocity is split into its horizontal (V1x) and vertical (V1y) components using trigonometric functions (cos and sin).
The acceleration in the X direction is 0 due to the absence of any horizontal force acting on the diver.
The acceleration in the Y direction is -9.8 m/s^2, representing the force of gravity acting downwards.
The final horizontal velocity (V2x) remains the same as the initial horizontal velocity (V1x) since there's no acceleration in the X direction.
The final vertical velocity (V2y) is calculated using the kinematic equation that accounts for the initial vertical velocity, acceleration, and change in height.
The total final velocity (V2) is found by applying Pythagoras' theorem to the horizontal and vertical velocity components.
The direction of the final velocity vector is determined using the inverse tangent function (arctan) and the components of the final velocity.
A basketball is thrown with an initial velocity of 4.5 m/s at a 60-degree angle, and the problem involves finding the height, time to reach the highest point, and horizontal distance traveled.
The horizontal component of the initial velocity (V1x) is found using the cosine of the throwing angle, and the vertical component (V1y) using the sine.
The time to reach the highest point is determined by the vertical motion with zero final vertical velocity and given initial vertical velocity and acceleration due to gravity.
The height at the highest point is calculated using the kinematic equation for displacement (y2) with the known initial conditions and time.
The horizontal distance traveled by the basketball is found by considering the horizontal motion with constant velocity and given time.
The problem concludes with a scenario involving Juliet dropping a note from a balcony to Romeo below, requiring the calculation of the initial velocity and its angle based on the known horizontal distance and height.
The initial horizontal velocity (V1x) of the note is determined by dividing the horizontal distance by the known time of fall.
The initial vertical velocity (V1y) is calculated by considering the change in height over time and the acceleration due to gravity.
The total initial velocity (V1) and its angle are found by combining the horizontal and vertical components using Pythagoras' theorem and the inverse tangent function.
Transcripts
Browse More Related Video
5.0 / 5 (0 votes)
Thanks for rating: