What are Vector and Scalar Quantities?

Free Animated Education

2 Feb 201904:23

EducationalLearning

32 Likes 10 Comments

TLDRThis video script offers a comprehensive overview of the fundamental concepts of vectors and scalars in physics. Scalars are physical quantities defined by a magnitude and an appropriate unit, such as mass or temperature. They follow standard algebraic rules for operations like addition, subtraction, multiplication, and division. Vectors, on the other hand, are distinguished by having both magnitude and direction, with examples including displacement and force. The script explains how vectors are represented, introduces the concept of unit vectors, and discusses equal vectors, null vectors, free vectors, and position vectors. It also touches on the resolution of vectors into their rectangular components. Additionally, the script intriguingly connects these physical concepts to vector graphics, highlighting the scalability and quality of vector-based digital images, which are composed of mathematical commands defining lines and shapes in two or three dimensions.

Takeaways

📏 Scalars are physical quantities with magnitude and units, examples include mass, distance, speed, etc.
📐 Vectors have magnitude, units, and direction, like displacement, force, and velocity.
👉 Vector representation uses a line with an arrow to show direction and length to represent magnitude.
📍 A unit vector has a magnitude of 1 and the same direction as the original vector.
🔀 Equal vectors have the same direction, magnitude, and unit.
🟢 The zero or null vector has zero magnitude and the same initial and terminal points.
🔄 Free vectors can be moved parallel to themselves without changing their effect.
📍 Position vectors represent the position of a point in space relative to the origin.
🧩 Resolution of a vector involves splitting it into rectangular components that are perpendicular to each other.
🖼️ Vector graphics are digital images made from commands that place lines and shapes in 2D or 3D space.
🔍 Vector graphics maintain quality and do not pixelate when zoomed in, unlike raster graphics.

Q & A

What are scalars in physics and how are they specified?
-Scalars are physical quantities that are completely specified by a number representing their magnitude and an appropriate unit. Examples include mass, distance, speed, density, volume, time, temperature, entropy, energy, charge, work, and potential.
How do you represent a vector in physics?
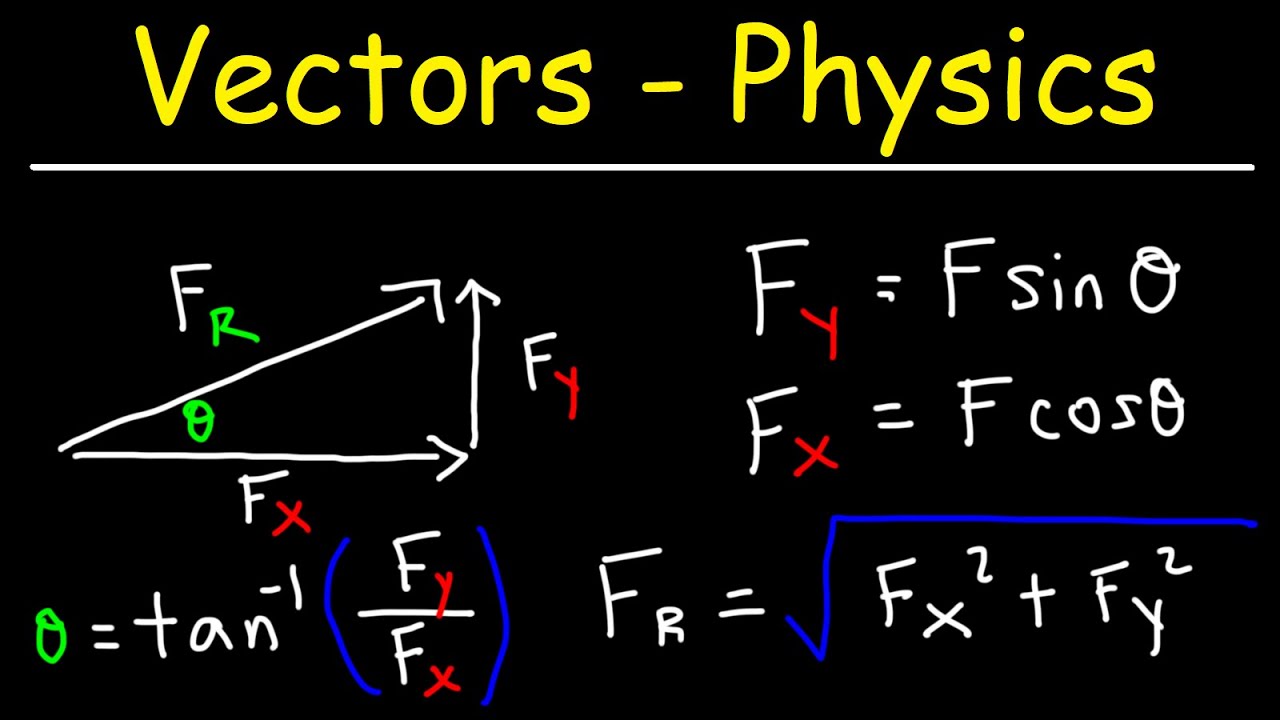
-A vector is represented by a straight line parallel to the direction of the vector. The length of the line on a certain scale specifies the magnitude of the vector, and an arrowhead at one end indicates its direction.
What is a unit vector and how is it denoted?
-A unit vector is a vector with a magnitude of 1 that is directed along the direction of a given vector. It is usually denoted by a letter with a cap over it, such as if 'r' is the given vector, then 'r̂' will be the unit vector in the direction of 'r'.
What are equal vectors and how are they defined?
-Equal vectors are two vectors that have the same direction, magnitude, and unit. They are considered identical in terms of their physical properties.
Define a null vector and how it is represented.
-A null vector, often denoted by 'O', is a vector with zero magnitude. Its initial and terminal points are the same, meaning it does not displace from its starting point.
What is a free vector and how can it be displaced?
-A free vector is one that can be displaced parallel to itself and applied at any point. It can be specified by giving its magnitude and any two of the angles between the vector and the coordinate axes.
What is the position vector and what does it represent?
-A position vector represents the position of a point in space with respect to the origin, 'O'. It indicates the distance and direction of the point from the origin.
Explain the resolution of a vector and its components.
-The resolution of a vector involves splitting up a single vector into its rectangular components, which must be at an angle of 90° to each other. This process allows for the vector to be analyzed in terms of its components along different axes.
What is vector graphics and how does it differ from other types of graphics?
-Vector graphics is the creation of digital images through a sequence of commands or mathematical statements that place lines and shapes in a given two-dimensional or three-dimensional space. Unlike raster graphics, vector graphics maintain quality and are not pixelated, even when zoomed in.
How does the quality of vector graphics compare to other image formats when zoomed in?
-The quality of vector graphics remains perfect and non-pixelated, no matter how many times you zoom in, which is a significant advantage over other image formats that may lose quality upon magnification.
What is the mathematical representation of a unit vector in terms of a given vector?
-The mathematical representation of a unit vector in the direction of a given vector 'r' is 'r̂ = r / |r|', where '|r|' represents the magnitude of the vector 'r'.
How are the projections of a vector in 3-D space related to its position and direction?
-In 3-D space, a vector's position and direction are determined by its three projections on the x, y, and z-axes. These projections provide the components of the vector along each axis.

Outlines

00:00

📏 Scalars and Vectors: Fundamentals of Physical Quantities

This paragraph introduces the concepts of scalars and vectors, which are two types of physical quantities. Scalars are quantities that can be fully described by a magnitude and an appropriate unit, such as mass, distance, and temperature. They follow standard algebraic rules for operations like addition, subtraction, multiplication, and division. Vectors, on the other hand, are characterized by a magnitude, a unit, and a specific direction, such as displacement and force. The representation of a vector is depicted by a line with an arrow indicating direction and a scale showing magnitude. The unit vector is a vector of magnitude one, parallel to the given vector, and is denoted with a cap over the letter representing it. Equal vectors are those with the same direction, magnitude, and unit. A null vector has zero magnitude and is denoted by 'O'. Free vectors can be moved parallel to themselves without changing their properties and are defined by their magnitude and the angles they make with coordinate axes. Position vectors represent the position of a point in space with respect to the origin. Vector resolution involves breaking down a vector into its rectangular components, which are perpendicular to each other. Additionally, the paragraph touches on vector graphics, which are digital images created using mathematical commands to place lines and shapes in a two or three-dimensional space, maintaining image quality even when zoomed in.

Mindmap

Keywords

💡Scalars

Scalars are physical quantities that are fully defined by their magnitude and a unit, without a need for direction. In the context of the video, scalars include examples like mass, speed, and energy. These quantities are emphasized as being governed by simple algebraic laws for operations like addition and subtraction, highlighting the fundamental properties that do not require directional consideration.

💡Vectors

Vectors are quantities that are described by magnitude, direction, and unit. The script uses examples like displacement and velocity to illustrate vectors. Vectors are central to the video's theme as they represent quantities that convey both size and the direction in which they act, making them crucial for describing physical phenomena like forces and movements.

💡Unit Vector

A unit vector is a vector whose magnitude is one and points in a specific direction. It is often used to specify direction alone. The script discusses how any vector can be normalized to a unit vector by dividing it by its magnitude. This concept is important for understanding vector operations and directions in physical spaces.

💡Zero or Null Vector

A zero or null vector is defined as having zero magnitude and no specific direction, denoted typically by 'O' in the script. It results from subtracting a vector from itself. This concept is vital for explaining the conditions under which no physical quantity is present or no movement occurs.

💡Free Vector

A free vector is described as one that can be displaced parallel to itself and applied at any point in space. The script uses this concept to illustrate vectors like forces or velocities, which can act anywhere along their line of action, emphasizing the flexibility in application of such vectors.

💡Position Vector

Position vector represents the position of a point relative to an origin, as described in the script. It involves both magnitude and direction. This concept is crucial in fields like physics and engineering to determine the location of points in space, which is fundamental to describing movement and location.

💡Resolution of a Vector

Resolution of a vector refers to breaking it down into perpendicular components, typically along the axes of a coordinate system. The script notes that these components make a 90° angle with each other. This process is essential for simplifying the analysis of vectors in physics and engineering by dealing with each component separately.

💡Vector Graphics

Vector graphics are digital images created through sequences of commands that define lines and shapes in a two- or three-dimensional space. Highlighted in the script, vector graphics maintain high quality upon zooming, unlike pixel-based graphics. This application of vectors extends beyond physics to digital art and graphic design.

💡Magnitude

Magnitude in the context of both scalars and vectors refers to the size or quantity of a physical property. The script distinguishes between scalar and vector magnitudes, emphasizing the role of magnitude in fully describing scalars and contributing to the description of vectors alongside direction.

💡Direction

Direction is crucial for defining vectors and differentiates them from scalars. As discussed in the video script, direction combined with magnitude helps in specifying vectors like velocity or force. Understanding direction is essential for predicting physical behaviors and interactions in multiple dimensions.

Highlights

Scalars are physical quantities that can be specified by a magnitude and an appropriate unit.

Scalar quantities can be manipulated using standard algebraic laws.

Examples of scalar quantities include mass, distance, speed, density, volume, time, temperature, entropy, energy, charge, work, potential, etc.

Vectors are physical quantities that require a magnitude, a unit, and a specific direction.

Vectors represent both a quantity and a direction simultaneously.

Examples of vectors include displacement, force, velocity, acceleration, and momentum.

A vector is represented by a straight line parallel to its direction with a specified magnitude and an arrowhead to indicate direction.

A unit vector has a magnitude of 1 and is directed along the direction of a given vector.

Unit vectors are denoted by a letter with a cap over it.

Equal vectors have the same direction, magnitude, and unit.

A null vector has zero magnitude and its initial and terminal points are the same.

The difference between two equal vectors is represented by a null vector.

A free vector can be displaced parallel to itself and applied at any point.

In 3-D, a free vector is determined by its three projections on the x, y, z-axes.

A position vector represents the position of a point in space with respect to the origin.

Resolution of a vector involves splitting it into its rectangular components, which are perpendicular to each other.

Vector graphics are digital images created through a sequence of commands or mathematical statements.

Vector graphics maintain perfect quality and do not pixelate when zoomed in.

Transcripts

Browse More Related Video

Vectors Physics Class 11th

Scalars, Vectors, and Vector Operations

12 - What are Vectors and Scalars?

Calculus 3 - Intro To Vectors

GCSE Physics - Scalar and Vector Quantities #41

Vectors - Basic Introduction - Physics

What are Vector and Scalar Quantities?

Takeaways

Q & A

What are scalars in physics and how are they specified?

How do you represent a vector in physics?

What is a unit vector and how is it denoted?

What are equal vectors and how are they defined?

Define a null vector and how it is represented.

What is a free vector and how can it be displaced?

What is the position vector and what does it represent?

Explain the resolution of a vector and its components.

What is vector graphics and how does it differ from other types of graphics?

How does the quality of vector graphics compare to other image formats when zoomed in?

What is the mathematical representation of a unit vector in terms of a given vector?

How are the projections of a vector in 3-D space related to its position and direction?