1D Motion & Kinematics - Physics 101 / AP Physics 1 Review with Dianna Cowern
TLDRIn this Physics Girl video, Dianna Cowern explores the principles of kinematics, the physics of motion, through engaging experiments and mathematical modeling. She demonstrates how to use graphs and equations to predict and understand motion, from the launch of a ping-pong ball to the safety of bike helmets. Key concepts like velocity, acceleration, and the equation of motion are explained with clarity and enthusiasm, making complex physics concepts accessible and fun.
Takeaways
- 📚 Kinematics is the physics of motion in general, not just human movement.
- 🚴♀️ The example of a cyclist on a flat trail is used to introduce mathematical modeling in physics.
- 📈 Graphing is a mathematical tool in physics, where the slope of a line represents velocity.
- 📐 Units are crucial in physics, and dimensional analysis is a technique to ensure consistency in equations.
- 🚀 The equation of motion (position = initial position + initial velocity * time + 0.5 * acceleration * time^2) is derived from a velocity vs. time graph.
- 🏎️ Acceleration is the change in velocity over change in time, with units of meters per second squared (m/s^2).
- 🥊 The acceleration experienced in a bike accident can be calculated using the equation of motion and is survivable but potentially concussive.
- 🛡️ A safer bike helmet can be designed by increasing the distance over which the head comes to a stop, akin to a car's crumple zone.
- 🎥 The video demonstrates how to calculate the acceleration of a ping-pong ball shot out of a vacuum-sealed PVC pipe.
- 🌌 Time can pass at different rates depending on velocity, which is a concept explored in special relativity.
- 💡 The lesson emphasizes the importance of visualizing physics problems through graphs and using average velocity for constant acceleration scenarios.
Q & A
What is the main topic of the video?
-The main topic of the video is kinematics, which is the physics of motion in general, and how it can be used to understand and potentially design a safer bike helmet.
How does the vacuum pump turn the PVC pipe with a ping-pong ball into a potential cannon?
-When the vacuum pump sucks out most of the air from the PVC pipe, it creates a pressure differential. Once the ping-pong ball's polyester covering is punctured, the atmospheric pressure outside the pipe pushes the air inside, accelerating the ping-pong ball down the pipe like a cannon.
What is the significance of the cyclist example in the video?
-The cyclist example is used to illustrate the concepts of kinematics, specifically constant velocity motion, by creating a mathematical model of her movement over time. It demonstrates how physics uses graphs and equations to predict and understand motion.
How does the speaker convert meters per second to miles per hour?
-The speaker converts meters per second to miles per hour by doubling the value in meters per second. So, 10 meters per second is approximately equal to 20 miles per hour.
What is a mathematical model in the context of physics?
-A mathematical model in physics is a simplified representation of a real-world situation, which allows for the prediction and understanding of physical phenomena. It often involves graphs, equations, and assumptions to focus on the most important aspects of the situation.
What is the equation of motion derived in the video?
-The equation of motion derived in the video is: position = initial position + initial velocity * time + 0.5 * acceleration * time^2. This equation describes the relationship between an object's position, its initial conditions, and the effects of constant acceleration.
How does the video demonstrate the concept of acceleration?
-The video demonstrates acceleration by discussing the change in velocity of the cyclist over time and by calculating the acceleration of the ping-pong ball in the PVC pipe, which was found to be an incredibly high 47,000 meters per second squared for the first 10 centimeters of travel.
What is the practical application of the concepts discussed in the video?
-The practical application of the concepts discussed is to design safer bike helmets. By understanding the acceleration experienced during a bike accident and how it can be distributed over a greater distance, one can design helmets with better impact absorption properties.
How does the video relate the concept of acceleration to everyday experiences?
-The video relates the concept of acceleration to everyday experiences by comparing the acceleration felt during a bike accident to the acceleration due to gravity, as well as the acceleration experienced by someone in a car accelerating from zero to 60 miles per hour in three seconds.
What is the significance of the mantis shrimp's claw acceleration in the video?
-The mantis shrimp's claw acceleration, which is around 3,000 meters per second squared, is used in the video as an extreme example to illustrate the concept of very high acceleration, showing that the acceleration experienced in the ping-pong ball experiment is not as extreme as the mantis shrimp's claw.
What is the final advice given by the speaker regarding learning physics?
-The final advice given by the speaker is to encourage viewers to stick with physics as it provides a deeper understanding of the world through the lens of mathematical models and equations. The speaker also encourages viewers to work through the problems presented in the video to truly grasp the concepts of kinematics.
Outlines
🚀 Introduction to Kinematics and the Physics of Motion
The video begins with a demonstration involving a PVC pipe, a vacuum pump, and a ping-pong ball to illustrate the concept of atmospheric pressure and motion. The host, Dianna Cowern, introduces herself and the topic of kinematics, which is the study of motion without considering the forces causing the motion. She emphasizes the importance of kinematics in ensuring safety in motion. The lesson focuses on the concept of designing a safer bike helmet, introducing mathematical modeling as a tool to achieve this. Dianna explains the conversion of units from meters per second to miles per hour and uses a mountain biking scenario to illustrate the creation of a mathematical model through graphing position versus time.
📈 Understanding Velocity and Mathematical Models
This paragraph delves into the concept of velocity and how it can be represented graphically. Dianna discusses the mathematical representation of the cyclist's motion, explaining how changes in velocity can be depicted as changes in the steepness of the line on the graph. She introduces the concept of slope as a measure of velocity and explains the use of dimensional analysis to ensure units are consistent in physics problems. The paragraph also covers the algebraic representation of motion, leading to the derivation of the equation Delta x = v * Delta t, which relates change in position to velocity and time.
🚴♀️ Modeling Motion with Constant and Variable Velocity
The host continues to explore kinematics by comparing the motion of an object with constant velocity to one with variable velocity. She uses the example of a cyclist on a highway to illustrate how acceleration affects the motion and the resulting change in position. Dianna demonstrates how to calculate the distance traveled by the cyclist using the area under the curve of a velocity versus time graph, which includes both rectangular and triangular areas. She introduces the concept of acceleration as the change in velocity over time and confirms the units for acceleration. The paragraph concludes with the formation of the equation of motion, which combines initial position, initial velocity, and acceleration to determine an object's position at any given time.
🛡️ Applying Kinematics to Real-World Scenarios
Dianna applies the concepts of kinematics to real-world scenarios, such as the acceleration experienced during a bike accident and the potential for a safer bike helmet design. She calculates the acceleration experienced by a cyclist's head during a fall and compares it to the acceleration due to gravity and other extreme examples, like the mantis shrimp's punch. The paragraph highlights the importance of understanding acceleration in the context of safety, particularly in the design of protective gear like bike helmets. Dianna encourages viewers to explore the concept of inflatable bike helmets and the role of crumple zones in reducing impact forces.
🌟 Wrapping Up the Kinematics Lesson and Encouraging Further Physics Study
In the concluding paragraph, Dianna summarizes the key takeaways from the lesson on kinematics, emphasizing the value of visualizing physics problems through graphs and the utility of average velocity in solving constant acceleration problems. She assigns homework to reinforce the concepts learned and encourages viewers to seek out additional problems for practice. The video ends with a message from Simone, a fellow YouTuber who shares her enthusiasm for physics and its ability to explain the world through mathematical models. The lesson leaves viewers with a curiosity about the deeper aspects of physics, such as time dilation in special relativity, and an invitation to continue exploring the subject.
Mindmap
Keywords
💡Kinematics
💡Ping-pong ball
💡Vacuum pump
💡Mathematical model
💡Velocity
💡Acceleration
💡Equation of motion
💡Crumple zone
💡Dimensional analysis
💡Graphing
💡Safety
Highlights
The physics concept of kinematics is introduced, which is the study of motion in general, not just human movement.
A demonstration is set up involving a PVC pipe, a vacuum pump, and a ping-pong ball to illustrate the principles of kinematics and acceleration.
The importance of kinematics in ensuring safety while moving through the world is discussed.
A mathematical model is explained as a simplified representation of real-world phenomena, using the example of a cyclist on a flat trail.
The conversion between metric and imperial units is explained, with a simple method to convert meters per second to miles per hour.
The concept of a point particle is introduced, a common simplification in physics where complex objects are reduced to a single point for the sake of calculations.
A graphical representation of the cyclist's motion is created, showing how a graph can be used to predict future positions based on constant velocity.
The definition of a mathematical model is explored, emphasizing its role in ignoring extraneous details to focus on average behavior.
The concept of slope in a graph is related to the physical concept of velocity, with the slope representing the change in position over time.
Dimensional analysis is introduced as a tool for solving physics problems, ensuring that units are consistent and making sense throughout calculations.
The algebraic equation for constant velocity motion is derived, showing that change in position equals velocity times time.
The concept of velocity versus time graphs is introduced, allowing for the calculation of displacement through the area under the curve.
The equation of motion is derived from the area under a velocity versus time graph, providing a master equation for kinematics.
The application of the equation of motion is demonstrated to solve a real-world problem, calculating the speed of a ping-pong ball being shot out of a tube.
The importance of understanding acceleration and its impact on safety is discussed, using the example of a bike helmet's crumple zone.
The video concludes with a call to action for viewers to continue exploring physics, hinting at more complex concepts like special relativity.
A guest message from Simone, a fellow physics enthusiast and YouTuber, encourages viewers to pursue their physics education for a deeper understanding of the world.
Transcripts
Browse More Related Video

HOW is the speed ZERO when rolling? Bulgarian Physics Olympiad

Rotation and Torque - Physics 101 / AP Physics 1 Review with Dianna Cowern
How to Cram Kinematics in 1 hour for AP Physics 1

Physics - Basic Introduction
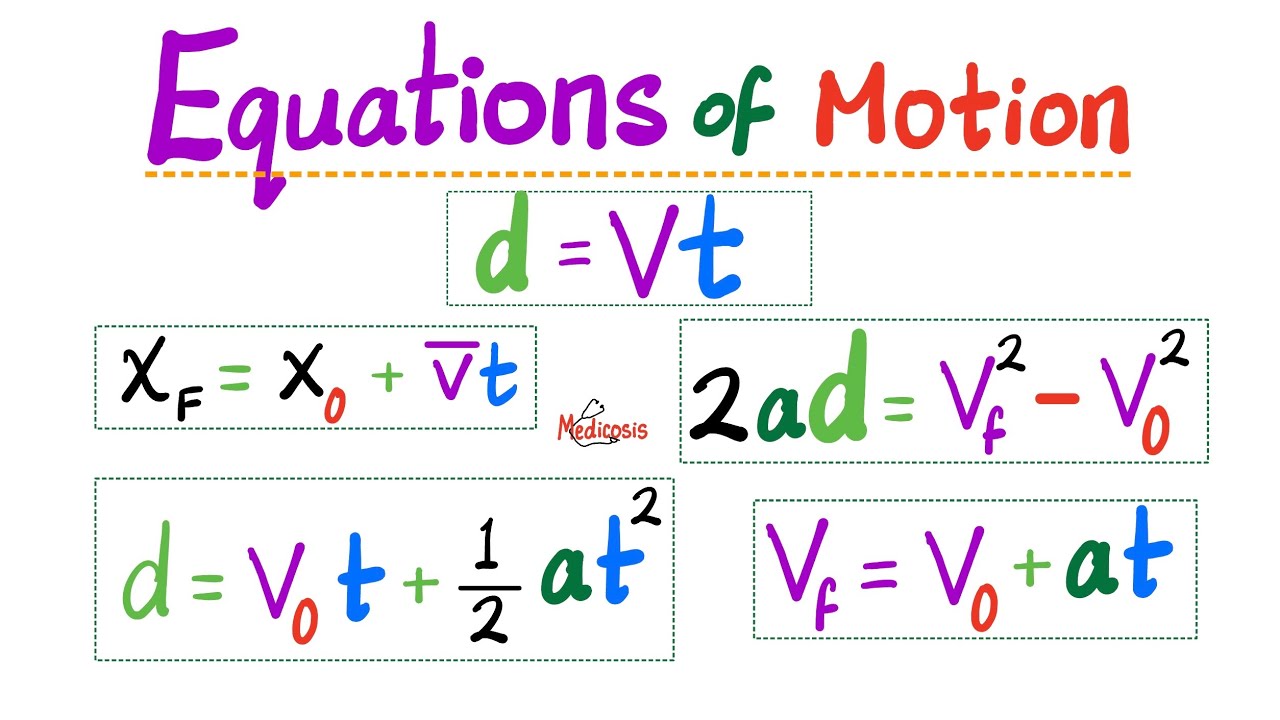
Equations of motion - Kinematics in one dimension - acceleration, velocity (initial, final) -Physics
AP Physics 1 | Unit 1: Kinematics
5.0 / 5 (0 votes)
Thanks for rating: