HOW is the speed ZERO when rolling? Bulgarian Physics Olympiad

ZPhysics
5 Mar 202310:34
EducationalLearning
32 Likes 10 Comments

TLDRThis video explores a challenging physics Olympiad problem from Bulgaria, focusing on rotational physics, thermodynamics, and relativity. The presenter walks through the solution to a problem involving a billiard ball, explaining key concepts like rolling without slipping, angular speed, and Newton's laws of motion. The video breaks down the equations and physics principles involved, making it accessible for viewers to understand complex topics. The explanation is detailed, offering insights into how to approach such problems, making it an engaging resource for physics enthusiasts.

Takeaways
  • 🔍 The video discusses a Physics Olympiad question from Bulgaria, focusing on challenging topics like rotational physics, thermodynamics, capacitors, and Lorentz transformations.
  • 🎱 The first problem involves a billiard ball that starts rolling without slipping after being hit, with initial velocity V_0 and friction coefficient K.
  • ⏰ The goal is to find the time T_0 when the ball starts rolling without slipping and its speed at that moment.
  • 🎯 The condition for rolling without slipping is that the horizontal distance traveled by the ball must equal the arc length it has covered.
  • ⚖️ Newton’s Second Law is applied to determine the deceleration of the ball due to friction, resulting in the equation V = V_0 - KgT.
  • ⚙️ The video explains angular velocity and acceleration, deriving that the angular speed ω is directly proportional to time.
  • 🔄 Using the condition for rolling without slipping, the equation V = Rω is used to find the time T_0 and the speed at that time.
  • 📉 The final speed of the ball at T_0 is calculated to be 5/7 of the initial speed V_0.
  • 🚲 A practical example is given, explaining that when a wheel rolls without slipping, the contact point with the ground momentarily has zero velocity.
  • 💡 The video concludes with an appreciation of the complexity and beauty of rotational physics problems, hinting at another problem from the International Physics Olympiad.
Q & A

  • What is the initial problem discussed in the transcript?

    -The initial problem involves a billiard ball that experiences an initial force and starts moving with an initial speed V₀. The task is to find the time at which the ball starts rolling without slipping and the speed at that precise time.

  • What does 'rolling without slipping' mean in the context of this problem?

    -'Rolling without slipping' means that the horizontal distance the ball travels (Δx) is equal to the arc length the ball covers as it rolls, which is given by the radius times the angle of rotation (RΔθ). This implies that the linear speed V is equal to the angular speed Ω multiplied by the radius R.

  • How is the linear speed V related to the angular speed Ω during rolling without slipping?

    -During rolling without slipping, the linear speed V is equal to the radius R multiplied by the angular speed Ω (V = RΩ).

  • How is the deceleration of the center of mass of the ball calculated?

    -The deceleration of the center of mass is calculated using Newton's Second Law. The frictional force acting on the ball, which is equal to the coefficient of friction (K) times the gravitational force (mg), provides the deceleration. The acceleration is then a = Kg.

  • What equation is used to describe the speed of the ball at a given time T?

    -The speed of the ball at a given time T is described by the equation V(T) = V₀ - KgT, where V₀ is the initial speed and KgT is the deceleration over time T.

  • How is the angular acceleration of the ball determined?

    -The angular acceleration (α) is determined using Newton's Second Law for rotation. The torque caused by the frictional force is equal to the moment of inertia times the angular acceleration. After simplifying, α is found to be 5Kg / 2R₀.

  • What is the relationship between the linear speed and the angular speed at the time the ball starts rolling without slipping?

    -At the time the ball starts rolling without slipping, the linear speed V is equal to the radius R₀ times the angular speed Ω, leading to the equation V₀ - KgT = 5KgT / 2.

  • How is the time T at which the ball starts rolling without slipping calculated?

    -The time T at which the ball starts rolling without slipping is calculated as T = 2V₀ / 7Kg, derived from the condition that the linear speed equals the product of the radius and angular speed.

  • What is the speed of the ball at the time it starts rolling without slipping?

    -The speed of the ball at the time it starts rolling without slipping is 5/7 of the initial speed V₀.

  • What is the significance of the contact point of a rolling ball in relation to its speed?

    -The contact point of a rolling ball, while it is rolling without slipping, momentarily has a speed of zero. This means that, at the instant of contact with the surface, the point is stationary relative to the surface, while the rest of the ball continues to rotate around it.

Outlines
00:00
🔍 Exploring a Bulgarian Physics Olympiad Problem

The video begins with an introduction to a physics Olympiad problem from Bulgaria, focusing on rotational physics, thermodynamics, parallel plate capacitors, and Lorentz transformations. The first problem to be solved involves a billiard ball experiencing an initial force, moving with speed V₀, and eventually rolling without slipping due to friction. The video explains the concept of rolling without slipping, where the ball's horizontal distance equals the arc length it travels. The condition for rolling without slipping is established by equating the linear speed V with the angular speed Rω.

05:01
⚙️ Analyzing Angular Acceleration and Rolling Conditions

This section delves deeper into the problem by analyzing the angular acceleration of the ball due to frictional force. Using Newton's Second Law for rotation, the angular acceleration (α) is derived by considering the torque caused by friction at a distance R₀ from the ball's center. The equation for angular speed ω as a function of time is obtained. Finally, the condition for rolling without slipping is applied to find the time (τ₀) at which the ball starts rolling without slipping and the corresponding speed, which is 5/7 of the initial speed V₀.

10:03
🔄 Understanding Rolling Motion in Everyday Contexts

The final paragraph provides a practical explanation of rolling motion, emphasizing the significance of the point of contact during rolling without slipping. It explains that at the contact point, the ball (or a bicycle wheel) momentarily has an instantaneous speed of zero while the rest of the wheel rotates around it. This concept is related to the everyday experience of driving, where the wheel’s contact point with the ground exhibits a brief moment of zero speed during each rotation. The paragraph concludes by highlighting the fascination of such rotational physics problems and introduces another problem from the International Physics Olympiad.

Mindmap
Keywords
💡Moment of Inertia
Moment of inertia is a measure of an object's resistance to rotational motion around a particular axis. In the video, it's a key concept in solving the rotational physics problem involving a billiard ball. The moment of inertia determines how much torque is required for a desired angular acceleration. For example, the moment of inertia of the ball with respect to its axis of rotation is essential in calculating the angular acceleration caused by friction.
💡Rolling Without Slipping
Rolling without slipping occurs when a rolling object's point of contact with the surface has zero relative velocity to the surface. This means the linear velocity of the object is equal to the product of its angular velocity and radius. In the video, the condition of rolling without slipping is central to solving the problem, where the time and speed at which the billiard ball transitions to rolling without slipping are calculated.
💡Frictional Force
Frictional force is the resistance force that acts opposite to the direction of motion when two surfaces are in contact. In this scenario, it decelerates the ball after it's struck. The video uses frictional force to calculate the deceleration of the ball's center of mass and to determine the torque that leads to its angular acceleration.
💡Angular Velocity
Angular velocity is the rate of change of an object's angular position with respect to time. It describes how quickly an object rotates or revolves around an axis. In the video, angular velocity is derived from the angular acceleration and is crucial in establishing the condition for rolling without slipping, where the linear speed of the ball equals the product of the angular velocity and radius.
💡Torque
Torque, also known as the moment of force, is a measure of the force causing an object to rotate. It is the product of the force applied and the distance from the axis of rotation. In the video, the torque caused by the frictional force at a distance from the ball’s center is used to determine the angular acceleration, which is necessary for understanding the ball's rolling motion.
💡Newton's Second Law
Newton's Second Law states that the force acting on an object is equal to the mass of the object times its acceleration (F = ma). The video applies this law to both the linear and rotational motion of the billiard ball, helping to calculate the deceleration due to friction and the resulting angular acceleration.
💡Coefficient of Friction
The coefficient of friction is a dimensionless scalar that represents the ratio of the frictional force between two bodies and the normal force pressing them together. In the video, it is denoted by 'K' and is used to calculate both the frictional force acting on the ball and the subsequent deceleration and torque, playing a key role in solving the physics problem.
💡Linear Speed
Linear speed refers to the distance traveled per unit time by an object in motion along a straight path. In the video, the linear speed of the billiard ball after being struck is analyzed to determine when it matches the speed due to angular rotation, a critical point for the rolling without slipping condition.
💡Angular Acceleration
Angular acceleration is the rate of change of angular velocity over time. It describes how quickly an object’s rotation is speeding up or slowing down. In the video, angular acceleration is calculated using the torque produced by friction, and it is essential for determining the angular velocity, which leads to the rolling without slipping condition.
💡Velocity Addition Formula
The velocity addition formula is used in relativistic physics to calculate the relative velocity of an object when considering the velocities in different reference frames. In the video, the Lorenz transformations and the velocity addition formula are mentioned in the context of a complex physics problem, illustrating the depth of knowledge required for the Physics Olympiad.
Highlights

Introduction to the problem involving a billiard ball with initial speed and the concept of rolling without slipping.

Explanation of the condition for rolling without slipping, where the horizontal distance equals the arc length traveled by the ball.

Derivation of the linear speed equation V = R * Omega, where V is the linear speed and Omega is the angular speed.

Application of Newton's Second Law to determine the deceleration of the center of mass of the ball due to friction.

Calculation of the acceleration of the center of mass as a = k * g, where k is the coefficient of friction and g is the acceleration due to gravity.

Introduction of the equation for the linear speed at time T: V = V0 - k * g * T.

Analysis of the angular velocity of the ball caused by frictional force acting at a distance R from the center.

Calculation of the angular acceleration using Newton's Second Law for rotation.

Derivation of the angular speed equation: Omega = 5 * k * g * T / (2 * R).

Application of the rolling without slipping condition to relate linear speed and angular speed.

Solution for the time when the ball starts rolling without slipping: T = 2 * V0 / (7 * k * g).

Calculation of the speed of the ball at the time it starts rolling without slipping: V = 5/7 * V0.

Discussion on how the linear speed equals the speed due to angular rotation, demonstrating the rolling without slipping condition.

Explanation of the concept that the contact point on a rolling ball momentarily has a speed of zero.

Conclusion emphasizing the significance of understanding rotational physics through problems like the one discussed.

Transcripts

Browse More Related Video

AP Physics 1 2018 Free Response Solutions

Rotational Motion: Crash Course Physics #11

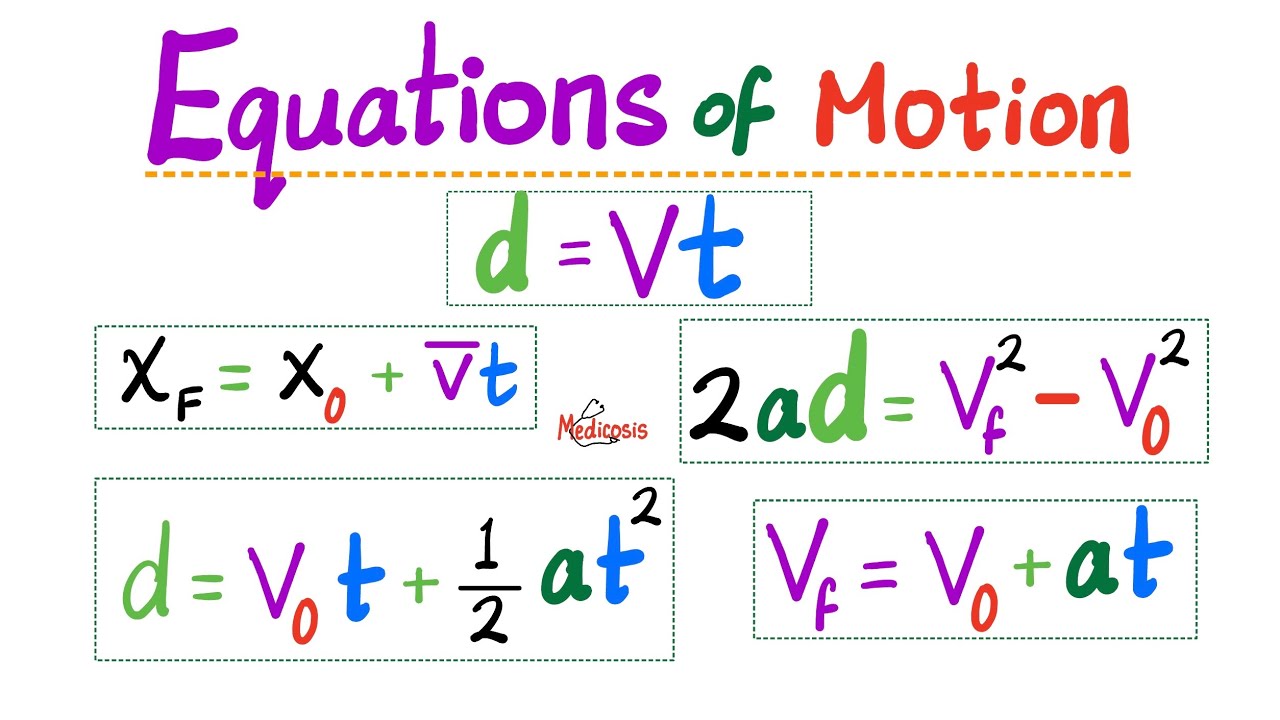
Equations of motion - Kinematics in one dimension - acceleration, velocity (initial, final) -Physics

Rotational Kinetic Energy | Rolling Without Slipping (AP Physics 1)

1D Motion & Kinematics - Physics 101 / AP Physics 1 Review with Dianna Cowern

A Simple Problem on the Hardest Physics Olympiad (IPhO)

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Physics OlympiadRotational MotionThermodynamicsLorentz TransformationsBulgarian ContestInertiaCapacitorsAngular VelocityProblem SolvingAdvanced Physics