Rotation and Torque - Physics 101 / AP Physics 1 Review with Dianna Cowern
TLDRIn this engaging physics lesson, Dianna Cowern explores the concepts of rotation and torque, using everyday examples like spinning objects and opening doors. She explains how rotational velocity is measured in radians per second and how it relates to linear velocity. Dianna also delves into the application of torque, demonstrating its effects with a screwdriver and discussing the impossibility of truly rigid objects due to special relativity. The lesson is a blend of practical examples and theoretical concepts, aiming to demystify rotational motion and its applications in our lives.
Takeaways
- 🌌 The introduction of rotational motion concepts using objects spinning and their paths on a dark background.
- 🌍 Understanding the Earth's rotation and its effect on linear and angular velocity, including how fast we spin around on Earth's surface.
- 📐 Learning the difference between linear speed (meters per second) and angular speed (radians per second).
- 🔄 Exploring the relationship between linear velocity (v), angular velocity (omega), and radius (r) in circular motion.
- 🐞 Analyzing the friction force on a bug on a spinning record and how it relates to centripetal force.
- 🌀 Explaining why we don't feel the Earth's rotation due to the balance between gravitational force and the normal force.
- 🚪 Discussing the physics behind door handles and why they are placed at the edge rather than in the middle or near the hinge.
- 💡 Introducing the concept of torque (rotational force) and its importance in causing rotation.
- 🔧 Demonstrating how a screwdriver works as a force multiplier using the principle of torque.
- 🤸♂️ Applying the concept of torque to balance a seesaw by understanding the position of the fulcrum.
- 🌐 Considering the impossibility of a truly rigid object due to special relativity and the effects of contraction at high speeds.
Q & A
What is the main topic of the lesson?
-The main topic of the lesson is 'Rotation and Torque', which focuses on understanding spinning objects and the forces that cause rotation.
How does the host demonstrate the concept of rotational motion?
-The host demonstrates rotational motion by tossing random objects with a fluorescent dot against a dark background, showing how they follow a smooth, curved path when the dot is placed at a specific point on the object.
What is the Earth's angular velocity in radians per second?
-The Earth's angular velocity is approximately 7.27 x 10^-5 radians per second.
How does the host relate linear velocity to angular velocity?
-The host relates linear velocity to angular velocity by using the formula v = ω * r, where v is the linear velocity, ω is the angular velocity, and r is the radius of rotation.
What is the purpose of the special dot placed on the objects in the demonstration?
-The special dot is placed on the objects to mark the center of mass, which is the point around which the object will naturally spin when it is freely rotating.
Why don't we feel the Earth's rotation?
-We don't feel the Earth's rotation because the centripetal force required for circular motion is balanced by the gravitational force and the normal force, resulting in a negligible change in the apparent weight of objects.
What is the relationship between torque and rotational motion?
-Torque is the rotational equivalent of linear force and is responsible for causing an object to rotate. It is calculated as the product of the force applied and the perpendicular distance from the axis of rotation to the point where the force is applied.
How does the position of the handle on a door affect the ease of opening it?
-The position of the handle affects the ease of opening the door because the further the handle is from the axis of rotation, the easier it is to apply torque and open the door.
What is the force multiplier effect of a screwdriver?
-A screwdriver acts as a force multiplier, increasing the force applied by the hand onto the screw by a factor of about 20 due to the difference in the radius of the torque applied by the hand and the radius at the screw head.
According to special relativity, why can't objects be completely solid and rigid?
-According to special relativity, objects cannot be completely solid and rigid because as they spin, different parts of the object move at different speeds, leading to different amounts of length contraction. This would cause the object to twist, preventing it from being truly rigid.
What is the significance of understanding torque in the context of everyday objects?
-Understanding torque is significant because it helps explain how everyday objects like door handles, screwdrivers, and seesaws work. It shows how the position and application of force affect the rotational motion of these objects.
Outlines
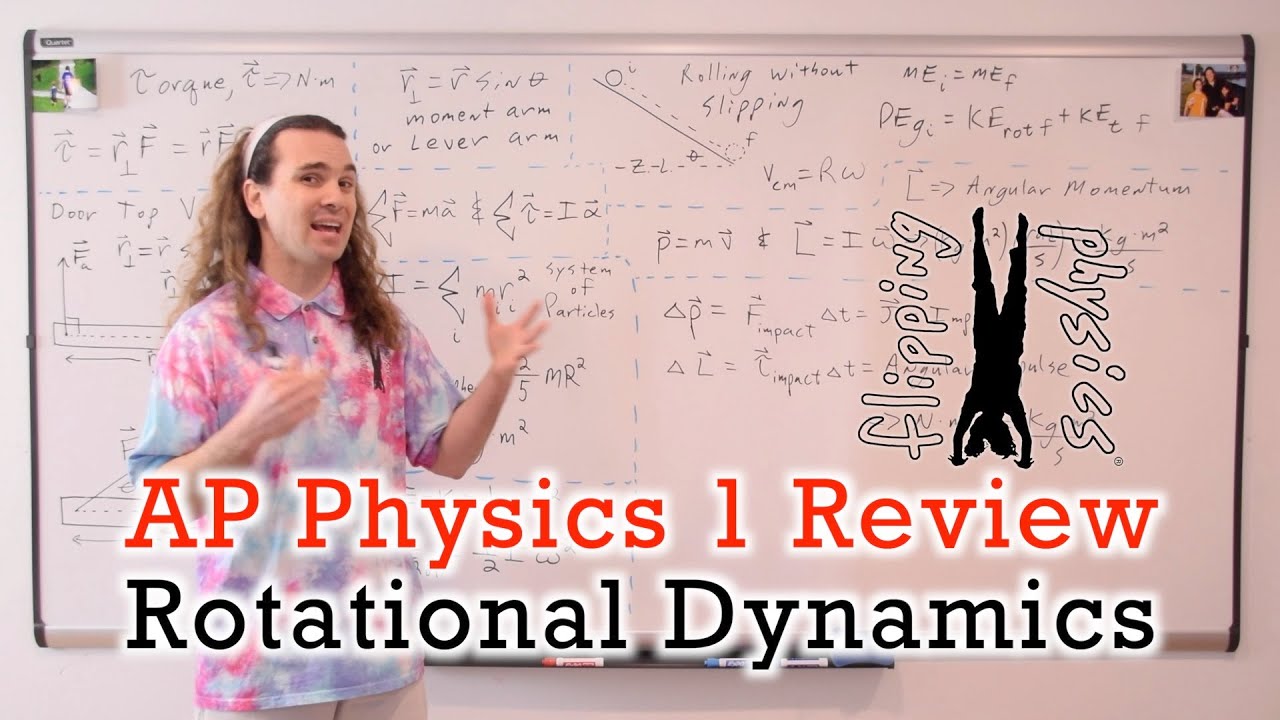
🌀 Introduction to Rotation and Torque
The video begins with a demonstration of objects' motion against a dark background, highlighting the smooth, curved paths they take when marked with a fluorescent dot. Host Dianna Cowern introduces the topic of rotation and torque, setting the stage for a lesson on understanding spinning objects and rotational motion. She discusses the concept of Earth's rotation and how it affects our perception of motion, leading into an exploration of the tools needed to describe and calculate rotational dynamics.
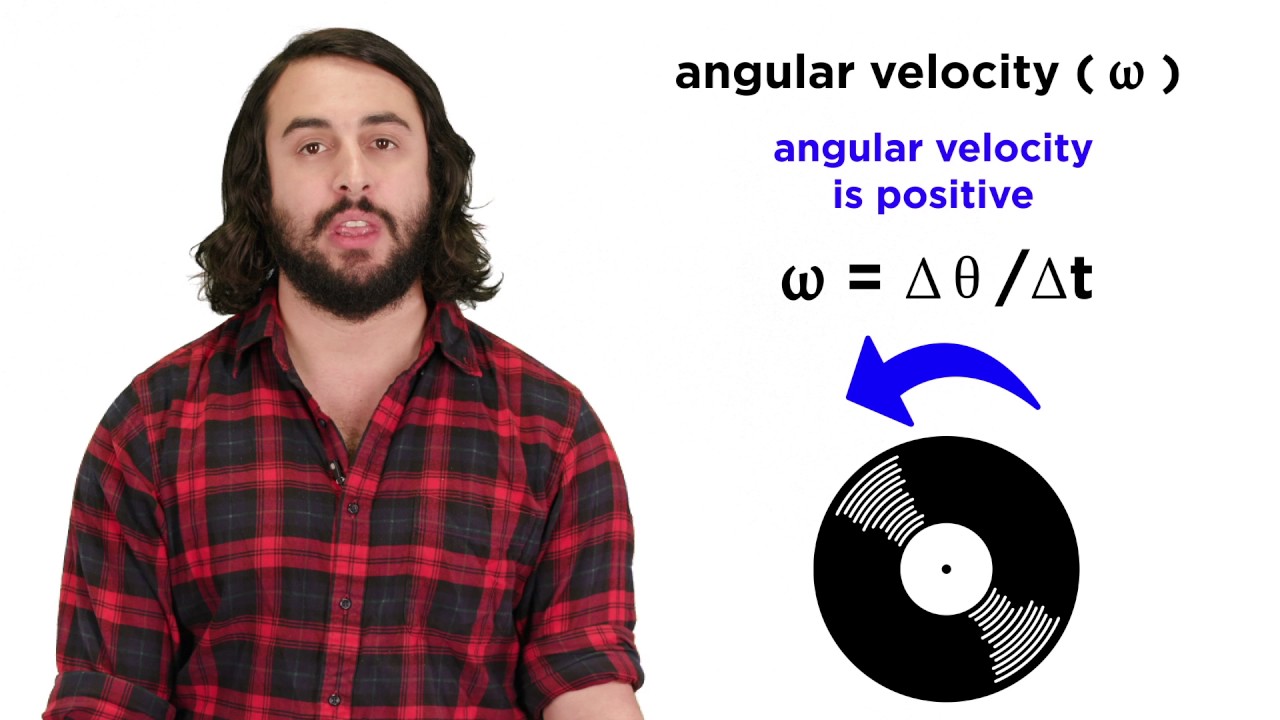
📐 Calculating Angular Velocity and Linear Speed
This segment delves into the mathematics of angular velocity and linear speed, differentiating between the two by using the example of Earth's rotation. The host calculates her own angular velocity and relates it back to her linear speed, demonstrating the conversion between the two using the relationship between angular velocity (omega) and radius (r). She also introduces the concept of centripetal force and its role in circular motion, providing a practical example involving a bug on a spinning record.
🚀 Understanding Centripetal Force and Earth's Rotation
The host uses a model of Earth to explain why we don't feel the centrifugal force while standing on a rotating Earth. She describes how centripetal force is a result of Earth's gravitational force and the normal force, and how the normal force changes due to Earth's rotation. The segment also covers the calculation of the friction force acting on a cat on a spinning record, using the centripetal force equation and the previously discussed concepts of angular velocity and radius.
🔧 The Physics of Torque and Door Knobs
This part of the video introduces the concept of torque, the rotational equivalent of linear force, and its symbol, the Greek letter tau. The host explains how torque is calculated and its relationship with the force applied and the distance from the axis of rotation. She uses the analogy of a door's handle placement to illustrate the effectiveness of torque application and discusses the center of mass in relation to spinning objects, including a demonstration with a hammer.
🛠️ The Mechanics of Screwdrivers and Teeter Totters
The host concludes the lesson by exploring the mechanics of screwdrivers, which act as force multipliers through the application of torque. She explains how the small radius at which a screwdriver applies force can amplify the force exerted by the hand. The segment also revisits the concept of torque with a teeter totter example, illustrating how the position of the fulcrum affects the balance of torques. The video ends with a brief mention of special relativity and its implications on rigid objects, encouraging viewers to continue their physics education.
Mindmap
Keywords
💡Rotation
💡Torque
💡Angular Velocity
💡Centripetal Force
💡Center of Mass
💡Linear Velocity
💡Radian
💡Gravitational Force
💡Special Relativity
💡Friction Force
Highlights
Dianna Cowern introduces the concept of rotational motion and its relevance to everyday experiences, such as the Earth's rotation and doorknobs' placement.
The lesson focuses on understanding 'Rotation and Torque' and their applications in physics.
Dianna explains the difference between linear speed and rotational velocity, using the Earth's rotation as an example.
Angular velocity, measured in radians per second, is introduced as a key concept for describing circular motion.
The relationship between linear velocity (v) and angular velocity (omega) is established through the formula v = omega * r.
Dianna demonstrates how the centripetal force acting on a bug on a spinning record can be calculated using angular velocity.
The reason why we don't feel the Earth's rotation is explained by the balance between gravitational force and the centrifugal force due to Earth's rotation.
Torque, the rotational equivalent of linear force, is defined and its role in causing objects to rotate is discussed.
The concept of center of mass and its importance in rotational motion is introduced, with objects naturally spinning around their center of mass.
Dianna shows how the position of a door's handle affects the ease of opening the door, relating it to the concept of torque.
The seesaw (teeter totter) example is used to illustrate the balance of torques and the calculation of the fulcrum's position.
The practical application of torque is demonstrated through the use of a screwdriver, explaining how it multiplies the force applied by the hand.
Dianna discusses the impossibility of a truly rigid object according to special relativity, as moving objects contract.
The lesson concludes with an encouragement to continue studying physics for its vast applications, including space travel and rocket engineering.
The importance of practicing physics problems at home is emphasized as a crucial method for learning and understanding the subject.
A special guest, Diana Alsindy, a propulsion engineer, shares her experience and encourages viewers to pursue physics for future opportunities in space exploration.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: