Physics - Ch 66 Ch 4 Quantum Mechanics: Schrodinger Eqn (77 of 92) The Barrier: An Overview

Michel van Biezen
6 May 201804:15
EducationalLearning
32 Likes 10 Comments

TLDRThe lecture delves into the quantum mechanical phenomenon of particle tunneling through barriers. It contrasts this with classical mechanics, where particles would fully reflect off a barrier if it exceeds their energy. Quantum mechanics allows for partial transmission, with the amplitude and frequency of the particle's wave changing depending on the energy and mass of the particle and the barrier's potential. The lecture introduces the concept of an exponential decay function to describe the particle's wave function as it passes through the barrier, highlighting the calculation of the wave function's amplitude on the other side of the barrier.

Takeaways
  • 🌟 In classical mechanics, particles with energy lower than a barrier's potential are fully reflected.
  • 📈 In quantum mechanics, particles can partially penetrate and even pass through barriers despite their energy being lower than the barrier's potential.
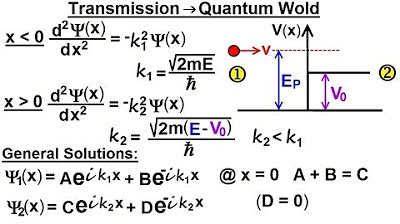
  • 🔄 The particle's behavior is described by wave functions in different regions, with separate equations for each region.
  • 🌊 There are three regions identified in the barrier scenario: Region 1 (before the barrier), Region 2 (inside the barrier), and Region 3 (after the barrier).
  • 🔄 Wave functions in each region have terms for particles moving to the right and left, except in Region 3 where there's no reflector.
  • 📉 Energy loss occurs when a particle transitions from Region 1 to Region 3, resulting in a smaller amplitude on the far side of the barrier.
  • 🌀 The wave number (k) remains the same across Regions 1 and 3, indicating that frequency and wavelength do not change, only the amplitude.
  • 📊 The barrier's potential creates an exponential decay function for the wave function within it, described by the coefficient alpha.
  • 🔄 For particles reflected back, there's an exponential increase function moving from right to left.
  • 🧮 The decay and amplitude of the wave function depend on the particle's energy, mass, and the barrier's potential energy.
  • 🚀 The lecture aims to calculate the wave function's appearance and amplitude in Region 3 compared to Region 1, providing insight into quantum tunneling.
Q & A

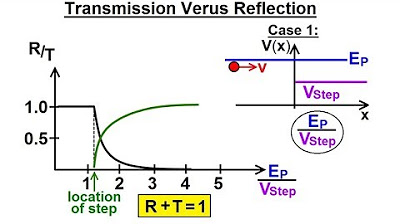
  • What happens when a particle encounters a barrier in classical mechanics?

    -In classical mechanics, when a particle encounters a barrier with a potential greater than the particle's energy, it will be 100% reflected off the barrier and move in the opposite direction.

  • How does quantum mechanics differ from classical mechanics in terms of a particle encountering a barrier?

    -In quantum mechanics, depending on the relative sizes of the particle's energy and the barrier's potential, some particles may be reflected while others can tunnel through the barrier and continue on the other side.

  • What are the three regions described in the lecture?

    -The three regions described are Region 1, where the particle initially is, Region 2, which is the barrier itself, and Region 3, which is the area beyond the barrier.

  • What do the two terms in the wave equations for Regions 1 and 2 represent?

    -The two terms in the wave equations for Regions 1 and 2 represent the particle moving to the right and the particle moving to the left, accounting for the possibility of reflection.

  • Why is the coefficient in front of the term for particles moving to the left in Region 3 equal to zero?

    -The coefficient is zero because there is nothing on the right side of Region 3 to cause reflection, so this term is not present in the wave function for Region 3.

  • What happens to the amplitude of a particle's wave function as it moves from Region 1 through the barrier to Region 3?

    -The amplitude of the particle's wave function decreases as it moves through the barrier due to energy loss, resulting in smaller oscillations in Region 3 compared to Region 1.

  • Does the frequency and wavelength of a particle change as it tunnels through the barrier?

    -No, the frequency and wavelength do not change as the particle tunnels through the barrier. Only the amplitude changes.

  • What is the equation for the wave number in Regions 1 and 3?

    -The wave number (k) in Regions 1 and 3 is given by the equation k = sqrt(2mE)/h-bar, where m is the mass of the particle, E is the energy of the particle, and h-bar is the reduced Planck constant.

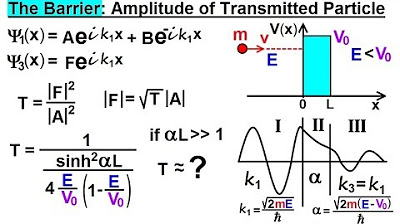
  • How does the energy of the barrier and the energy of the particle affect the exponential decay function?

    -The exponential decay function is represented by alpha, which is the square root of 2 times the mass of the particle times the difference in energy between the barrier and the particle. A higher barrier or lower particle energy results in a more significant exponential decay.

  • What is the significance of the exponential decay and increase functions in the barrier region?

    -The exponential decay function indicates the decrease in wave amplitude from the left side to the right side of the barrier, while the exponential increase function (with a positive coefficient) indicates the reflection and reversal of direction for particles that are reflected off the barrier.

  • What will be the focus of the lecture moving forward?

    -The lecture will focus on determining the function and amplitude of the wave function in Region 3 compared to Region 1, considering the energy of the particle, its mass, and the energy of the potential barrier. It will also cover how the wave function decays as it passes through the barrier.

Outlines
00:00
🌟 Quantum Mechanics and Barrier Tunneling

This paragraph introduces the concept of quantum tunneling, contrasting it with classical mechanics. In classical mechanics, particles with less energy than a barrier's potential will always reflect off it. However, in quantum mechanics, some particles can pass through the barrier, depending on their energy relative to the barrier's potential. The lecture explains the behavior of particles in three different regions when encountering a barrier, with a focus on the wave functions and energy loss during transition. It also discusses the change in amplitude and the constant frequency and wavelength as particles move through the barrier. The key equation for the wave number is introduced, highlighting its dependence on the particle's energy and the barrier's potential energy.

Mindmap
Keywords
💡Quantum Mechanics
Quantum mechanics is a fundamental theory in physics that describes the behavior of matter and energy at very small scales, such as atomic and subatomic particles. In the context of this video, quantum mechanics is contrasted with classical mechanics, highlighting that particles in quantum mechanics can pass through barriers that would be insurmountable in classical physics due to the phenomenon of quantum tunneling.
💡Barrier
A barrier in the context of the video refers to a region of space where the potential energy is higher than the energy of the particle. This concept is crucial in understanding quantum tunneling, where particles can pass through such barriers despite not having enough energy to overcome them classically.
💡Potential Energy
Potential energy is the stored energy an object possesses due to its position in a force field, such as a gravitational or electric field. In the video, the potential energy of the barrier is what creates the conditions for quantum tunneling, as it is greater than the energy of the particle in certain scenarios.
💡Quantum Tunneling
Quantum tunneling is a quantum mechanical phenomenon where a particle can pass through a potential barrier that it classically shouldn't be able to overcome. This occurs due to the wave-like nature of particles in quantum mechanics, which allows them to exist on both sides of the barrier, albeit with reduced probability.
💡Wave Functions
Wave functions in quantum mechanics are mathematical descriptions that provide information about the probability amplitude of a particle's location. They evolve over time according to the Schrödinger equation and are used to calculate the probability of finding a particle in a particular state.
💡Amplitude
Amplitude in the context of wave functions refers to the maximum value of the wave, which is related to the probability of finding a particle at a certain location. In the video, the amplitude of the wave function decreases when a particle passes through a barrier due to energy loss.
💡Wave Number
The wave number, denoted by 'k', is a measure of the spatial frequency of a wave, inversely related to the wavelength. In quantum mechanics, the wave number is used to determine the momentum of a particle and is affected by the energy of the particle and the potential landscape.
💡Exponential Decay Function
An exponential decay function describes how a quantity decreases exponentially over time or space. In the context of the video, it is used to describe the decrease in the amplitude of the wave function as a particle moves from one side of the barrier to the other in a quantum tunneling scenario.
💡Reflection
In the context of the video, reflection refers to the change in direction of a particle when it encounters a barrier. In classical mechanics, all particles are reflected off the barrier if the barrier's potential is higher than the particle's energy. However, in quantum mechanics, some particles may be transmitted through the barrier, in addition to some being reflected.
💡Energy Conservation
Energy conservation is a fundamental principle of physics stating that the total energy in an isolated system remains constant. In the video, this principle is implicit when discussing the energy of the particle relative to the potential of the barrier, and how it affects the particle's behavior, such as tunneling or reflection.
💡Schrödinger Equation
The Schrödinger equation is a fundamental equation in quantum mechanics that describes how the quantum state of a physical system changes with time. It is used to determine the wave function of a system, which in turn provides information about the probabilities of various outcomes.
Highlights

In classical mechanics, particles with energy lower than the barrier's potential are entirely reflected.

Quantum mechanics allows some particles to pass through barriers despite having less energy than the barrier's potential.

The particle's energy relative to the barrier's potential determines the reflection and transmission of particles.

If the barrier is not wide and the particle has sufficient energy, it can tunnel through the barrier.

There are three regions in the barrier problem, each with different wave equations for particle behavior.

Region 1 and 2 have terms for particles moving both to the right and left due to potential reflection.

Region 3 has no term for particles moving to the left because there's no reflector on that side.

The wave number in region 3 is the same as in region 1, indicating that frequency and wavelength remain unchanged.

Amplitude of the particle's oscillations decreases in region 3 due to energy loss during barrier transition.

The barrier's potential creates an exponential decay function, unlike the auditory function in classical mechanics.

The coefficient alpha in the exponential decay function is determined by the particle's mass and energy difference.

Reflection from the barrier results in an exponential increase function for particles moving from right to left.

The lecture aims to calculate the wave function and amplitude on the other side of the barrier.

Tunneling through a barrier is possible for particles with less energy than the barrier's potential if the barrier is not too wide.

The energy of the particle, its mass, and the potential energy of the barrier are key factors in tunneling.

The decay and amplitude of the wave function after passing through the barrier will be analyzed.

This overview provides a fundamental understanding of quantum tunneling through barriers.

Transcripts

Browse More Related Video

Physics - Ch 66 Ch 4 Quantum Mechanics: Schrodinger Eqn (65 of 92) Wave Constants B=? C=?

Physics - Ch 66 Ch 4 Quantum Mechanics: Schrodinger Eqn (64 of 92) Transmission-Reflection (Q.M.)

Physics - Ch 66 Ch 4 Quantum Mechanics: Schrodinger Eqn (76 of 92) Penetration Depth V0, E: Ex.

Physics - Ch 66 Ch 4 Quantum Mechanics: Schrodinger Eqn (78 of 92) The Barrier: Amplitude

23. Quantum Mechanics V: Particle in a Box

Physics - Ch 66 Ch 4 Quantum Mechanics: Schrodinger Eqn (62 of 92) Transmission vs Reflection

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
QuantumMechanicsTunnelingPhenomenonParticleBarrierWaveFunctionsEnergyStatesAmplitudeDecayPhysicsLectureEducationalContentScientificExplorationMathematicalModels