Physics - Ch 66 Ch 4 Quantum Mechanics: Schrodinger Eqn (76 of 92) Penetration Depth V0, E: Ex.

Michel van Biezen
5 May 201805:06
EducationalLearning
32 Likes 10 Comments

TLDRThis lecture explores the concept of quantum tunneling, where particles can penetrate a potential barrier despite having insufficient energy. It explains the penetration depth using the equation derived from the wave function, highlighting the relationship between the particle's energy and the barrier's energy. The lecture provides numerical examples to illustrate how the probability of a particle penetrating the barrier decreases with distance, emphasizing that a particle is unlikely to travel more than five atomic diameters into a potential step when the step's energy is ten times the particle's energy.

Takeaways
  • πŸ“ The lecture discusses the penetration probability of a particle into a potential step when its energy is lower than the step's energy.
  • 🧬 The probability is derived from the wave function in the region of interest, specifically the magnitude squared of the wave function.
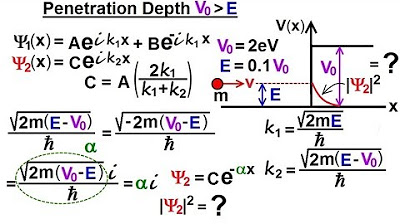
  • 🌟 The equation for penetration depth is given by e^{-2\alpha x}, where \alpha is related to the wave number squared and is a real, decaying function due to imaginary numbers.
  • πŸ”¬ The value of \alpha was determined in a previous lecture and is used to calculate the penetration depth.
  • πŸ“ The bore number, 53 times 10^-12 meters, is introduced as a comparison to the penetration depth.
  • πŸ’‘ The penetration depth is compared to the diameter of a hydrogen atom, which is approximately 0.1 nanometers or 116 picometers.
  • πŸ“Š Calculations for 2\alpha x for various values of X are pre-computed to simplify the process.
  • 🎒 The probability of the particle penetrating one-tenth of an atom's diameter is high, with a value of 0.349.
  • 🎯 At one atom's diameter (0.1 nm), the probability drops to about 25%, indicating a 1 in 4 chance.
  • 🚫 Beyond five atom diameters, the probability of penetration is almost zero, suggesting that particles do not typically penetrate much further than this distance into the potential step.
  • βš–οΈ The potential step's relative height to the particle's energy greatly affects the probability and depth of penetration.
Q & A

  • What is the main topic of the lecture?

    -The main topic of the lecture is the discussion of the probability of a particle penetrating a potential step when its energy is smaller than the energy of the step.

  • How is the penetration probability calculated?

    -The penetration probability is calculated by taking the magnitude squared of the wave function in the region of interest, which is equal to 0.4 e to the minus 2 alpha X, where alpha is related to the wave number squared (k^2) and X is the position.

  • What is the significance of the bore number?

    -The bore number, 53 times 10 to the minus 12 meters or 53 picometers, is used to compare the penetration depth of a particle with the size of a typical atom, such as a hydrogen atom.

  • How does the diameter of a hydrogen atom relate to the penetration depth?

    -The diameter of a hydrogen atom is approximately 0.1 nanometers or 116 picometers, which is used as a reference to understand the probability of a particle penetrating the potential step at various distances.

  • What happens to the probability as the particle moves further into the potential step?

    -As the particle moves further into the potential step, the probability of it continuing to penetrate decreases, becoming almost zero at five atom diameters.

  • What is the role of the potential step's energy relative to the particle's energy in the penetration probability?

    -The greater the potential step's energy relative to the particle's energy, the less probable it is for the particle to penetrate any depth into the barrier. Conversely, if the energy is almost as big as the potential, the particle is expected to go further.

  • What is the value of 2 alpha X when X is zero?

    -When X is zero, the value of 2 alpha X is 1, which results in the magnitude of the wave function squared being equal to 0.4.

  • At what distance does the probability of the particle penetrating the potential step drop to about 25%?

    -The probability drops to about 25% when the particle has penetrated a distance of one atom diameter (0.1 nanometers).

  • What is the probability of the particle penetrating five atom diameters into the potential step?

    -The probability of the particle penetrating five atom diameters into the potential step is approximately 0.00004, which is almost zero.

  • How does the penetration depth change if the potential step's energy is ten times the value of the particle's energy?

    -If the potential step's energy is ten times the value of the particle's energy, the particle is much less likely to penetrate any depth into the barrier, and the probability decreases significantly.

  • What can one infer from the calculated probabilities?

    -One can infer that the probability of a particle penetrating a potential step decreases exponentially with the distance penetrated, and it is highly dependent on the relative energies of the particle and the potential step.

Outlines
00:00
🌟 Quantum Mechanics: Particle Penetration into Potential Step

This paragraph introduces the concept of quantum mechanics, specifically focusing on the penetration of a particle into a potential step. It explains the equation that describes the probability of finding a particle within the step when its energy is lower than the step's energy. The wave function's magnitude squared is used to calculate this probability, leading to an equation involving a decaying exponential function. The penetration depth is compared to the Bohr number, with calculations provided for various values of X, demonstrating how the probability decreases as the particle moves further into the potential step.

Mindmap
Keywords
πŸ’‘potential step
A potential step refers to a region in space where the potential energy of a particle is higher than in the surrounding areas. In the context of the video, it describes a barrier that a particle with insufficient energy must overcome to penetrate. The video explains how the probability of a particle being found within this step decreases with depth, as quantified by the penetration depth.
πŸ’‘wave function
The wave function is a mathematical description of the quantum state of a particle. It provides information about the probability of finding a particle in a particular location. In the video, the wave function is used to derive the penetration probability into a potential step, with its magnitude squared representing this probability.
πŸ’‘energy
Energy, in the context of the video, refers to the quantum mechanical energy of a particle. It is a fundamental property that determines whether a particle can overcome the potential step and penetrate into it. The video discusses how the energy of the particle relative to the potential step influences the probability of penetration.
πŸ’‘penetration depth
Penetration depth is the distance into the potential step that a particle with a certain energy is likely to penetrate. It is a measure of how far a particle can get into the potential step against the energy barrier. The video provides an equation to calculate this depth and discusses how it compares to the size of an atom.
πŸ’‘alpha
Alpha is a constant that appears in the equation for the penetration depth. It is related to the decay rate of the wave function's magnitude squared within the potential step and is dependent on the energy difference between the particle and the potential step. Alpha is used to determine the rate at which the probability of finding the particle decreases as it penetrates deeper into the potential step.
πŸ’‘imaginary numbers
In the context of the video, imaginary numbers are used to convert the negative potential energy difference into a real, decaying function for the wave function. This is necessary because the energy of the particle is less than the potential step, which would otherwise result in a negative probability, which is not physically meaningful.
πŸ’‘Bohr radius
The Bohr radius is a standard unit of length in atomic physics, approximately equal to 53 picometers. It is used as a reference for atomic sizes and is the most probable distance between the proton and electron in a hydrogen atom in its ground state. The video uses the Bohr radius to compare the penetration depth and to illustrate the scale of the distances involved.
πŸ’‘probability
In quantum mechanics, probability is used to describe the likelihood of finding a particle in a particular state or location. The video focuses on the probability of a particle penetrating a potential step and how this probability changes with distance into the step.
πŸ’‘exponential decay
Exponential decay refers to a type of decay where the quantity decreases exponentially with time or distance. In the video, the exponential decay is used to describe how the probability of finding a particle within the potential step decreases rapidly as the particle penetrates deeper.
πŸ’‘atomic diameter
The atomic diameter is the distance across an atom, typically measured from the center to the outermost shell. In the video, the atomic diameter is used as a comparative measure to understand the penetration depth of particles into the potential step.
πŸ’‘quantum tunneling
Quantum tunneling is a phenomenon in quantum mechanics where a particle can pass through a potential barrier that it classically could not overcome due to lack of sufficient energy. The video's discussion of particle penetration into the potential step is an example of quantum tunneling, where the particle has a non-zero chance of getting through the barrier despite the energy mismatch.
Highlights

The lecture discusses the probability of a particle penetrating a potential step when its energy is smaller than the step's energy.

The penetration depth is calculated using the wave function squared.

The equation for penetration depth is derived from the wave function and is proportional to e^(-2Ξ±x).

The value of Ξ± is related to the wave number squared (k^2) and is a real number with imaginary parts.

The Bohr number (53 * 10^-12 meters) is introduced as a comparison for the penetration depth.

The diameter of a hydrogen atom is approximately 0.1 nanometers or 116 * 10^-12 meters.

The quantity 2Ξ±X is calculated for various X values to simplify the process.

When X is zero, the magnitude squared of the wave function equals 0.4.

At one-tenth the diameter of an atom (10 picometers), the probability of penetration is significantly high.

At one atom diameter (0.1 nanometers), the probability of a particle penetrating is about 25%.

The probability decreases as the particle penetrates further, reaching 0.0256 at an atom diameter.

At five atom diameters, the probability of penetration is almost zero.

The potential step's relative energy value greatly affects the particle's penetration probability.

If the particle's energy is close to the potential step's energy, the particle is expected to penetrate further.

The lecture provides a method to determine how far a particle will penetrate a potential step with a given energy.

Transcripts

Browse More Related Video

Physics - Ch 66 Ch 4 Quantum Mechanics: Schrodinger Eqn (75 of 92) Penetration Depth V0, E

Physics - Ch 66 Ch 4 Quantum Mechanics: Schrodinger Eqn (77 of 92) The Barrier: An Overview

Physics - Ch 66 Ch 4 Quantum Mechanics: Schrodinger Eqn (64 of 92) Transmission-Reflection (Q.M.)

Physics - Ch 66 Ch 4 Quantum Mechanics: Schrodinger Eqn (62 of 92) Transmission vs Reflection

Physics - Ch 66 Ch 4 Quantum Mechanics: Schrodinger Eqn (65 of 92) Wave Constants B=? C=?

Physics - Ch 66 Ch 4 Quantum Mechanics: Schrodinger Eqn (74 of 92) R=? T=? V0=(3/4)E,.(Ex. 4 of 4)

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
QuantumMechanicsParticlePenetrationPotentialBarriersWaveFunctionsQuantumDecayPhysicsLectureEnergyComparisonAtomicDiameterProbabilityCalculationsScientificAnalysis