Physics - Ch 66 Ch 4 Quantum Mechanics: Schrodinger Eqn (65 of 92) Wave Constants B=? C=?

Michel van Biezen
24 Apr 201807:19
EducationalLearning
32 Likes 10 Comments

TLDRIn this online lecture, the presenter discusses the quantum mechanical behavior of particles encountering a potential barrier. Despite the particle's energy exceeding the barrier's energy, a phenomenon occurs where some particles are transmitted and others are reflected, contrary to classical mechanics. The lecture focuses on deriving two key equations that describe the wave function on either side of the barrier and uses boundary conditions to solve for the constants, ultimately aiming to calculate the transmission (T) and reflection (R) probabilities of the particles.

Takeaways
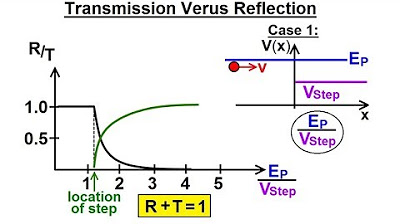
  • 🌟 In quantum mechanics, particles can be both transmitted and reflected even when their energy exceeds the potential barrier.
  • 📐 The wave function is described by two equations on either side of the boundary, with different conditions on the left (no potential barrier) and right (with potential barrier).
  • 🔢 The potential barrier in the example is smaller than the particle's energy, leading to partial reflection and transmission of particles.
  • 🎯 At the boundary (x=0), the wave function must satisfy two conditions: the function itself and its derivative must be continuous.
  • 🧠 By setting x=0, we derive the first equation relating the coefficients A, B, and C of the wave function.
  • 📚 The second equation is obtained by taking the derivative of the wave functions and ensuring the slopes are continuous at the boundary.
  • 🌐 By solving these equations, we can express the coefficients B and C in terms of the coefficient A.
  • 🔄 The process of solving for B and C allows us to eliminate these coefficients, leaving us with a single constant A in the wave functions.
  • 🔢 The wave numbers (k1 and k2) are crucial in defining the equations and are related to the particle's energy on each side of the boundary.
  • 📈 The final equations will enable us to calculate the transmission (T) and reflection (R) probabilities of the particles in future videos.
  • 🔍 The script provides a foundation for understanding quantum behavior at potential barriers and sets the stage for further analysis of particle transmission and reflection.
Q & A

  • What is the main topic of the lecture?

    -The main topic of the lecture is the behavior of wave functions in quantum mechanics, specifically when particles encounter a potential barrier.

  • What is the significance of the potential barrier being smaller than the particle's energy?

    -When the potential barrier is smaller than the particle's energy, part of the particles will be transmitted and part will be reflected, which is a phenomenon not observed in classical mechanics.

  • What are the two key equations derived in the lecture?

    -The two key equations derived in the lecture describe the wave function on both sides of the boundary where a potential barrier exists.

  • Why is it important to find the values of B and C in terms of A?

    -Finding the values of B and C in terms of A is important because it allows us to solve for the wave functions with only one constant, which is necessary for determining the transmission (T) and reflection (R) probabilities of the particles.

  • What does the continuity condition at the boundary imply?

    -The continuity condition at the boundary implies that the slope of the function on the left side of the boundary must equal the slope of the function on the right side, ensuring a smooth transition of the wave function across the boundary.

  • How are the wave numbers (k1 and k2) related to the energies of the particles?

    -The wave numbers (k1 and k2) are defined by the energies of the particles on the respective sides of the boundary, according to the laws of quantum mechanics.

  • What happens at the boundary when x equals zero?

    -When x equals zero, we use the derived equations to find the relationship between the coefficients A, B, and C of the wave functions, which helps in understanding the transmission and reflection of particles.

  • What is the significance of the equation K1*B + K2*B = K1*A - K2*A?

    -This equation is used to solve for B in terms of A, which is a crucial step in eliminating B and finding the transmission and reflection coefficients.

  • How does the cancellation of K2 in the numerator and denominator simplify the equation for C?

    -The cancellation of K2 in the numerator and denominator simplifies the equation for C, allowing us to express C in terms of A more clearly, which is necessary for further calculations.

  • What will be covered in the future videos?

    -In future videos, the lecture series will show how to use the derived equations and constants to calculate the transmission (T) and reflection (R) probabilities of particles at the boundary.

  • Why is it assumed that D is equal to zero?

    -It is assumed that D is equal to zero because once the particles pass the initial part of the barrier and enter region two, none of them will return, meaning there are no particles moving to the left past the boundary.

Outlines
00:00
🌟 Quantum Mechanics: Wave Function and Barrier

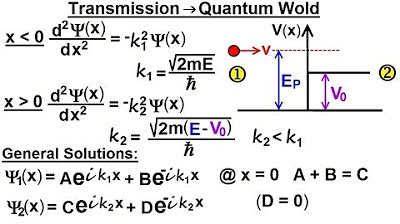
This paragraph introduces the concept of wave functions in quantum mechanics, focusing on the behavior of particles encountering a potential barrier. It explains that despite the particle's energy being greater than the barrier's energy, a portion of the particles will be reflected, which is a phenomenon not observed in classical mechanics. The speaker presents two equations that describe the wave function on both sides of the boundary and emphasizes the importance of understanding what happens at the boundary where x equals zero. The equations lead to the conclusion that the coefficients A, B, C, and D must satisfy certain conditions for continuity and slope at the boundary. The goal is to solve for B and C in terms of A to simplify the equations and ultimately determine the transmission (T) and reflection (R) probabilities of the particles.

05:03
📚 Solving for Coefficients in Quantum Barrier Scenario

The second paragraph delves into the process of solving for the coefficients B and C in terms of A, which is necessary for understanding particle transmission and reflection probabilities. The speaker guides through the algebraic manipulation of the equations, aiming to isolate B and C. By replacing B with its equivalent expression and solving the resulting equations, the speaker derives a formula for B in terms of A. Similarly, the process is repeated to express C in terms of A. The paragraph concludes with the achievement of two key equations that express B and C solely in terms of A, setting the stage for future calculations of transmission and reflection probabilities, which will be covered in subsequent videos.

Mindmap
Keywords
💡Wave Function
In quantum mechanics, the wave function is a mathematical description of the quantum state of a system. It provides information about the probability of finding a particle in a particular state. In the video, the wave function is used to describe the behavior of particles on both sides of a potential barrier, illustrating how it changes when a particle encounters a barrier with less energy than its own.
💡Potential Barrier
A potential barrier in quantum mechanics is a region in space where the potential energy is higher than the energy of a particle. It is a situation where a particle can still pass through (quantum tunneling) or be reflected, depending on the energy levels. In the video, the barrier is smaller than the particle's energy, leading to both reflection and transmission of particles.
💡Quantum Mechanics
Quantum mechanics is a fundamental theory in physics that describes the behavior of matter and energy at the atomic and subatomic scales. It differs from classical mechanics in that it incorporates the principles of wave-particle duality and the uncertainty principle. The video uses concepts from quantum mechanics to explain why particles can be reflected even when their energy exceeds the barrier's energy.
💡Particle Energy
Particle energy refers to the total energy of a particle, which can be kinetic or potential. In the context of the video, the particle's energy is compared to the potential barrier's energy to determine the particle's behavior when encountering the barrier.
💡Boundary
In the context of the video, a boundary refers to a specific location, usually defined by a change in potential energy, where the behavior of the wave function changes. The left side of the boundary has no potential barrier, while the right side does.
💡Reflection
In the context of quantum mechanics, reflection refers to the phenomenon where particles are 'bounced back' from a potential barrier. This is unexpected in classical mechanics, where particles with sufficient energy would simply pass over or through the barrier. The video explains that even when the particle's energy exceeds the barrier's energy, some particles are still reflected.
💡Transmission
Transmission in quantum mechanics refers to the process where particles pass through a potential barrier. Unlike classical mechanics, quantum mechanics allows for the possibility of transmission even when the barrier's energy is greater than the particle's energy, due to the wave-like nature of particles.
💡Constants
In the context of the video, constants are symbols used in equations to represent fixed values that do not change. The constants A, B, C, and D are used to solve for the wave function and its derivative at the boundary, which helps in determining the transmission and reflection coefficients.
💡Derivative
The derivative is a mathematical concept that represents the rate of change of a function with respect to its independent variable. In the video, taking the derivative of the wave functions is necessary to ensure continuity at the boundary, meaning the slope of the wave function on one side of the boundary must equal the slope on the other side.
💡Transmission Coefficient (T)
The transmission coefficient, denoted as T, is a measure of the probability that a particle will be transmitted through a potential barrier in quantum mechanics. It is a crucial concept in understanding the behavior of particles at boundaries and potential barriers.
💡Reflection Coefficient (R)
The reflection coefficient, denoted as R, quantifies the probability that a particle will be reflected by a potential barrier in quantum mechanics. It is an essential aspect of understanding how particles interact with barriers and is complementary to the transmission coefficient.
Highlights

The lecture discusses the behavior of particles in quantum mechanics versus classical mechanics when encountering a potential barrier.

In quantum mechanics, even when the particle's energy exceeds the barrier's energy, some particles are reflected and some are transmitted.

The potential barrier in the example is smaller than the particle's energy, leading to both reflection and transmission of particles.

Two equations are derived to describe the wave function on both sides of the boundary, one without a potential barrier and one with.

At the boundary, the wave function must satisfy two conditions: the function itself and its derivative must be continuous.

The continuity of the wave function at the boundary leads to the first equation: A + B must equal C, with the understanding that D is already zero.

The second condition for the wave function at the boundary is that the slope on the left must equal the slope on the right, leading to the equation k1*A - k1*B = k2*C.

By setting X equal to zero and taking the derivative of both functions, the equation k1*A - k1*B = k2*C is derived.

The equations are used to solve for B and C in terms of A, which simplifies the process of finding the transmission (T) and reflection (R) probabilities.

The equation B = A*(k1 - k2) / (k1 + k2) is derived to express B in terms of A, which is a key step in the analysis.

Similarly, the equation C = A*(2*k1) / (k1 + k2) is derived, which allows C to be expressed in terms of A.

With B and C expressed in terms of A, the wave functions on both sides of the boundary are now described using a single constant, A.

These derived expressions for B and C will be used in future videos to calculate the transmission and reflection probabilities.

The wave numbers k1 and k2 are associated with the energy of the particle on either side of the boundary and are crucial in the equations.

The lecture provides a detailed mathematical framework for understanding quantum tunneling and the behavior of particles at potential barriers.

The process of deriving the equations and solving for B and C demonstrates the application of boundary conditions in quantum mechanics.

The lecture is part of a series that will eventually show how to calculate the transmission and reflection probabilities, which have practical applications in understanding quantum phenomena.

Transcripts

Browse More Related Video

Physics - Ch 66 Ch 4 Quantum Mechanics: Schrodinger Eqn (64 of 92) Transmission-Reflection (Q.M.)

Physics - Ch 66 Ch 4 Quantum Mechanics: Schrodinger Eqn (62 of 92) Transmission vs Reflection

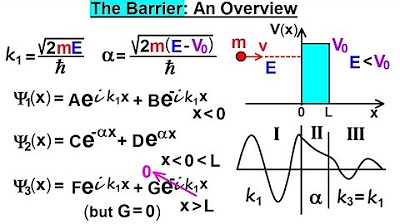
Physics - Ch 66 Ch 4 Quantum Mechanics: Schrodinger Eqn (77 of 92) The Barrier: An Overview

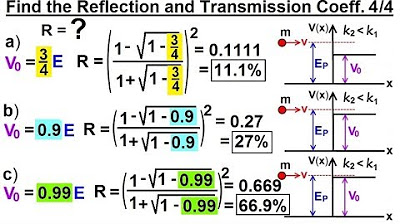
Physics - Ch 66 Ch 4 Quantum Mechanics: Schrodinger Eqn (74 of 92) R=? T=? V0=(3/4)E,.(Ex. 4 of 4)

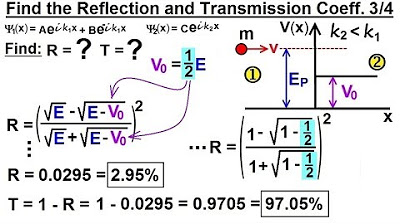
Physics - Ch 66 Ch 4 Quantum Mechanics: Schrodinger Eqn (73 of 92) R=? T=? V0=(1/2)E (Ex. 3 of 4)

Physics - Ch 66 Ch 4 Quantum Mechanics: Schrodinger Eqn (76 of 92) Penetration Depth V0, E: Ex.

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
QuantumMechanicsWaveFunctionsPotentialBarriersParticleBehaviorEnergyDynamicsQuantumReflectionPhysicsLectureOnlineEducationEducationalContentScienceExploration