Video 33 - Covariant Derivative Proof

Tensor Calculus

4 Jun 202218:26

EducationalLearning

32 Likes 10 Comments

TLDRIn this educational video, the host provides a detailed proof that the expressions derived for the covariant derivative indeed represent tensors. The demonstration involves deriving transformation equations for the covariant vector components and showing their tensorial transformation properties. By manipulating and substituting expressions with Jacobian factors, the video concludes with the proof that both the covariant and contravariant derivative expressions are tensors, solidifying the understanding of tensor calculus.

Takeaways

📚 The video is part of a series on tensor calculus, focusing on proving that derived expressions for the covariant derivative are indeed tensors.
🔍 The presenter begins by examining the covariant derivative as it relates to a covariant vector component, aiming to show it produces a second rank tensor that is twice covariant.
📉 To prove tensorial nature, the transformation equation for the covariant derivative expression is derived, demonstrating its tensor transformation.
🔗 The transformation involves finding how the expression relates to its counterpart in a different coordinate system (z prime system), expecting two Jacobian factors with appropriate indices.
📝 The script revisits previous videos, using derived expressions for the partial derivative of a covariant component and the transformation rule for the Christoffel symbol.
🔑 The transformation of the Christoffel symbol is key to building the full expression for the covariant derivative term.
🔄 The process involves renaming indices to match terms and ensuring tensor calculus syntax rules are followed, such as avoiding index collisions.
🧩 By combining terms and recognizing patterns, the presenter simplifies the expression to a form that can be directly substituted into the original equation.
🔄 The script demonstrates the cancellation of identical terms and the emergence of the covariant derivative in the z-prime system, confirming tensorial transformation.
🔍 A similar approach is taken for the covariant derivative of a contravariant vector component, using established transformation rules for partial derivatives.
🔗 The final expressions show the correct transformation rule between the z prime system and the unprimed system, proving the tensorial nature of the covariant derivative for both covariant and contravariant components.

Q & A

What is the main objective of the video?
-The main objective of the video is to provide a direct proof that the expressions derived for the covariant derivative are indeed tensors by deriving their transformation equations.
What type of tensor is the covariant derivative of a covariant vector component claimed to be?
-The covariant derivative of a covariant vector component is claimed to be a second rank tensor that is twice covariant.
What is the role of the Jacobian factors in the transformation of the covariant derivative expressions?
-The Jacobian factors are expected to affect the transformation between the original and the primed coordinate system, indicating the tensorial nature of the expressions.
How does the transformation of the partial derivative of a covariant component relate to the proof?
-The transformation of the partial derivative of a covariant component is used as a base to substitute into the covariant derivative expression, which is part of proving its tensorial nature.
What is the significance of the transformation rule for the Christoffel symbol in the video?
-The transformation rule for the Christoffel symbol is used to work on the part of the covariant derivative expression involving this symbol, which is crucial for the proof.
Why is it important to rename indices when transforming tensor expressions?
-Renaming indices is important to avoid index collisions and to ensure that the transformed expressions match the expected tensorial form, adhering to the rules of tensor calculus syntax.
What does the Kronecker delta represent in the context of the video?
-In the context of the video, the Kronecker delta represents an identity mapping between indices, which simplifies the transformed expression by absorbing certain indices.
How does the video demonstrate the cancellation of identical terms in the transformed expression?
-The video demonstrates the cancellation by showing that after renaming and regrouping terms, identical terms on opposite sides of the equation cancel each other out, simplifying the expression.
What is the final form of the covariant derivative expression in the primed system?
-The final form of the covariant derivative expression in the primed system is shown to be equivalent to the original expression, involving the covariant derivative with respect to the primed coordinates.
How does the video conclude the proof that the covariant derivative expressions are tensors?
-The video concludes the proof by showing that the transformed expressions match the expected tensorial form with the correct transformation rule involving Jacobian factors and matching free indices.

Outlines

00:00

📚 Direct Proof of Tensorial Nature in Covariant Derivatives

This paragraph introduces the objective of the video, which is to provide a direct proof that the expressions derived for the covariant derivative are indeed tensors. The focus is on the expression related to the covariant vector component and the goal is to show it transforms as a second-rank tensor with two covariant indices. The method involves deriving the transformation equation and demonstrating its tensorial transformation with the help of Jacobian factors.

05:01

🔍 Transformation Equation Derivation for Covariant Derivatives

The paragraph delves into the process of deriving the transformation equation for the covariant derivative expression. It involves finding the transformation of partial derivatives and Christoffel symbols, and combining them to form the complete transformation rule. The use of Kronecker deltas and index renaming is highlighted to simplify the expression and establish the tensorial nature of the covariant derivative.

10:01

📐 Establishing Tensorial Transformation for Contravariant Components

This section continues the exploration of tensor calculus by focusing on the covariant derivative of a contravariant vector component. The transformation rule for the partial derivative of a contravariant component is applied, and the Christoffel symbol's transformation is used to construct the full transformation expression. The paragraph explains the steps of multiplying factors, introducing Kronecker deltas, and renaming indices to simplify and prove the tensorial property of the expression.

15:02

🧩 Finalizing the Proof for Mixed Tensors in Covariant Derivatives

The final paragraph concludes the proof by examining the transformation rule for a second-rank mixed tensor. It discusses the process of factoring out common terms, renaming indices to avoid conflicts, and using the product rule for partial derivatives. The paragraph demonstrates the cancellation of identical terms and the final expression that confirms the tensorial nature of the covariant derivative in both covariant and contravariant forms.

Mindmap

Keywords

💡Tensor Calculus

Tensor calculus is a branch of mathematics that deals with tensors, which are generalizations of scalars, vectors, and higher-dimensional arrays. It is used extensively in physics, particularly in the field of general relativity. In the video, tensor calculus is the main theme, with the speaker discussing the proof that certain expressions derived for the covariant derivative are indeed tensors.

💡Covariant Derivative

The covariant derivative is a generalization of the directional derivative to curved spaces, such as those described in general relativity. It is a key concept in differential geometry and is used to define curvature. The video focuses on proving that expressions for the covariant derivative of both covariant and contravariant vector components are tensors.

💡Second Rank Tensor

A second rank tensor is a tensor with two indices, which can be thought of as a matrix in linear algebra. In the context of the video, the claim is that the expression for the covariant derivative of a covariant vector component produces a second rank tensor that is twice covariant.

💡Transformation Equation

A transformation equation is used to describe how a quantity changes under a coordinate transformation. In the video, the speaker derives the transformation equation for the covariant derivative expression to demonstrate that it transforms as a tensor, which is a necessary condition for it to be a tensor.

💡Jacobian Factors

Jacobian factors are determinants that relate to the transformation between two coordinate systems. They are crucial in tensor calculus for ensuring that the transformation of a tensor is consistent with its rank. The video discusses how two Jacobian factors with appropriate indexes affect the transformation of the covariant derivative expression.

💡Christoffel Symbol

The Christoffel symbol is a function that encodes the curvature of a manifold in the language of differential geometry. It is used in the calculation of covariant derivatives. The video script includes the transformation rule for the Christoffel symbol, which is essential for the proof of the tensorial nature of the covariant derivative.

💡Partial Derivative

A partial derivative is the derivative of a function with respect to one variable, while the other variables are held constant. In the video, the transformation of the partial derivative of a covariant component is discussed, which is a fundamental step in proving the tensorial nature of the covariant derivative.

💡Kronecker Delta

The Kronecker delta is a function that equals 1 if its two indices are equal and 0 otherwise. It is used in tensor calculus to simplify expressions and to absorb indices. In the video, the Kronecker delta is used to simplify the transformation of the covariant derivative expression.

💡Contravariant Vector Component

A contravariant vector component is a component of a vector that transforms in the opposite way to a covariant vector component under a change of coordinates. The video includes a discussion on the covariant derivative of a contravariant vector component and its transformation rule.

💡Symmetric and Antisymmetric

In the context of tensors, symmetric and antisymmetric refer to the properties of tensor components under index permutation. A symmetric tensor has components that remain the same when its indices are swapped, while an antisymmetric tensor changes sign. The video mentions the symmetry of certain terms in the covariant derivative expression, which is important for the transformation rule.

💡Product Rule

The product rule is a fundamental calculus rule that allows for the differentiation of a product of two functions. In the video, the product rule is used when taking the partial derivative of the covariant derivative expression with respect to a new variable, which is necessary for the proof.

Highlights

Introduction to a direct proof that expressions for the covariant derivative are tensors.

Starting with the covariant derivative expression related to a covariant vector component from video 32.

Claim that the expression produces a second rank tensor that is twice covariant.

Deriving the transformation equation to prove tensor nature by showing it transforms as a tensor.

Finding the transformation of expressions between the z and z prime systems.

Expectation of two Jacobian factors with appropriate indexes for transformation.

Using the transformation rule for the partial derivative of a covariant component from video 27.

Deriving a similar expression for the term involving the Christoffel symbol from video 29.

Building an expression for the whole term using the transformation of the Christoffel symbol.

Renaming indexes to match the expression's structure for tensor transformation.

Substituting covariant transformation expressions to simplify the proof.

Avoiding index collision by using new letters for tensor calculus syntax.

Simplifying the expression by factoring out common terms and using the Kronecker delta.

Proving the covariant derivative for the covariant component is a tensor through transformation.

Applying the same method to the covariant derivative of a contravariant vector component.

Using the transformation rule for the partial derivative of a contravariant vector component.

Multiplying and distributing factors to construct the transformation for the contravariant case.

Renaming indexes and using the product rule for partial derivatives in the proof.

Final expression showing the transformation rule for a second rank mixed tensor.

Conclusion that both derived expressions for the covariant derivative are indeed tensors.

Transcripts

Browse More Related Video

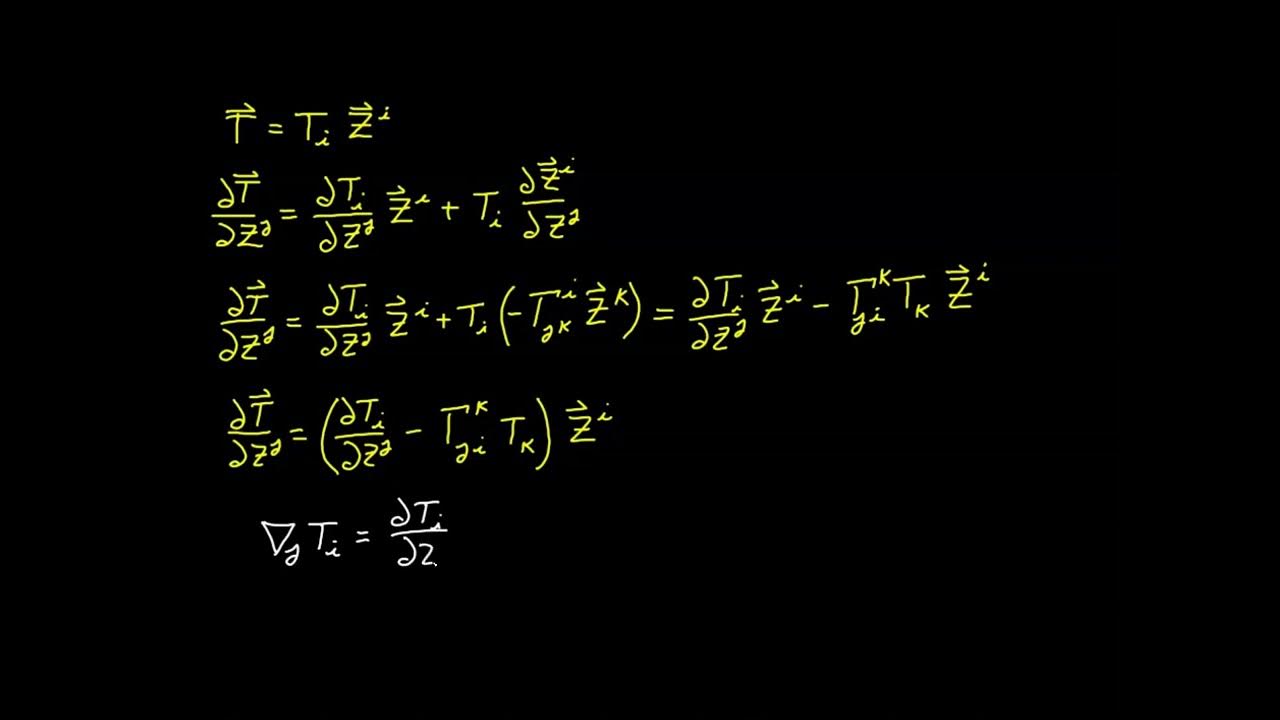
Video 32 - Covariant Derivative for Covariant Vector

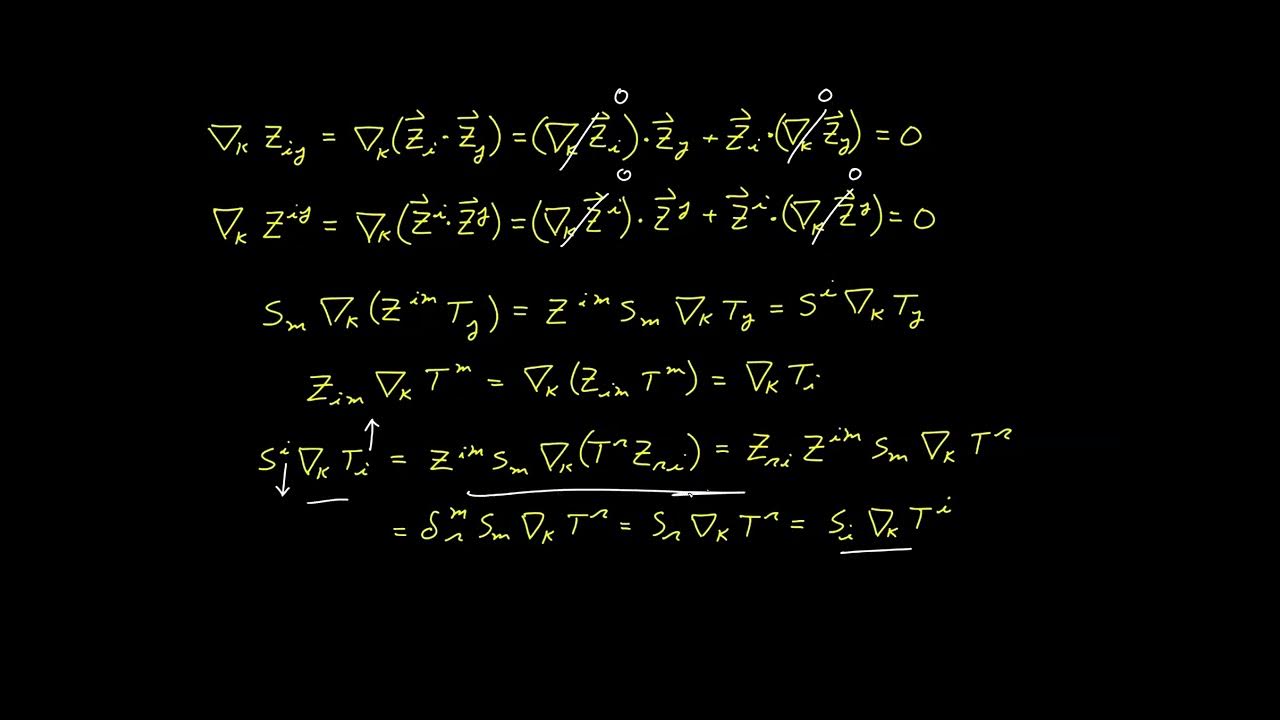
Video 38 - Metrinilic Property - Part 2

Tensor Calculus 17.5: Covariant Derivative (Component Definition) - Optional

Video 19 - Tensor Definition

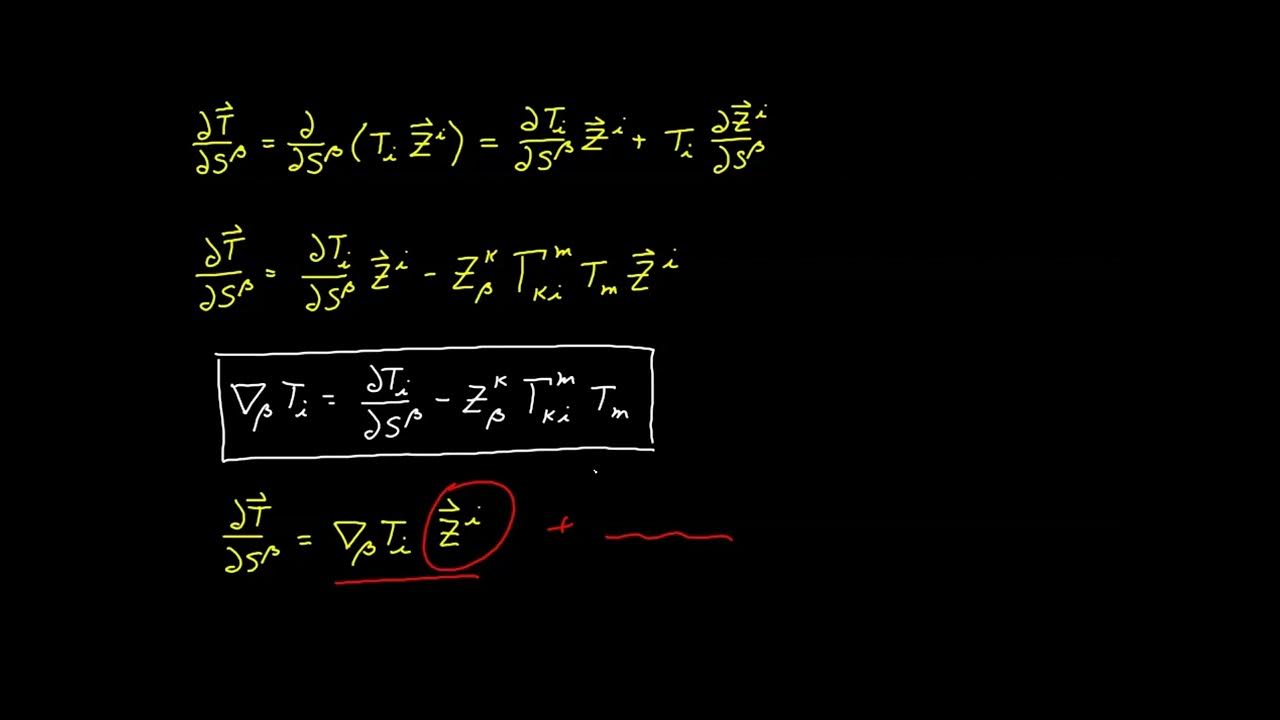
Video 72 - Surface Covariant Derivative for Non Tangent Vectors

Video 27 - Transformation of Partial Derivatives