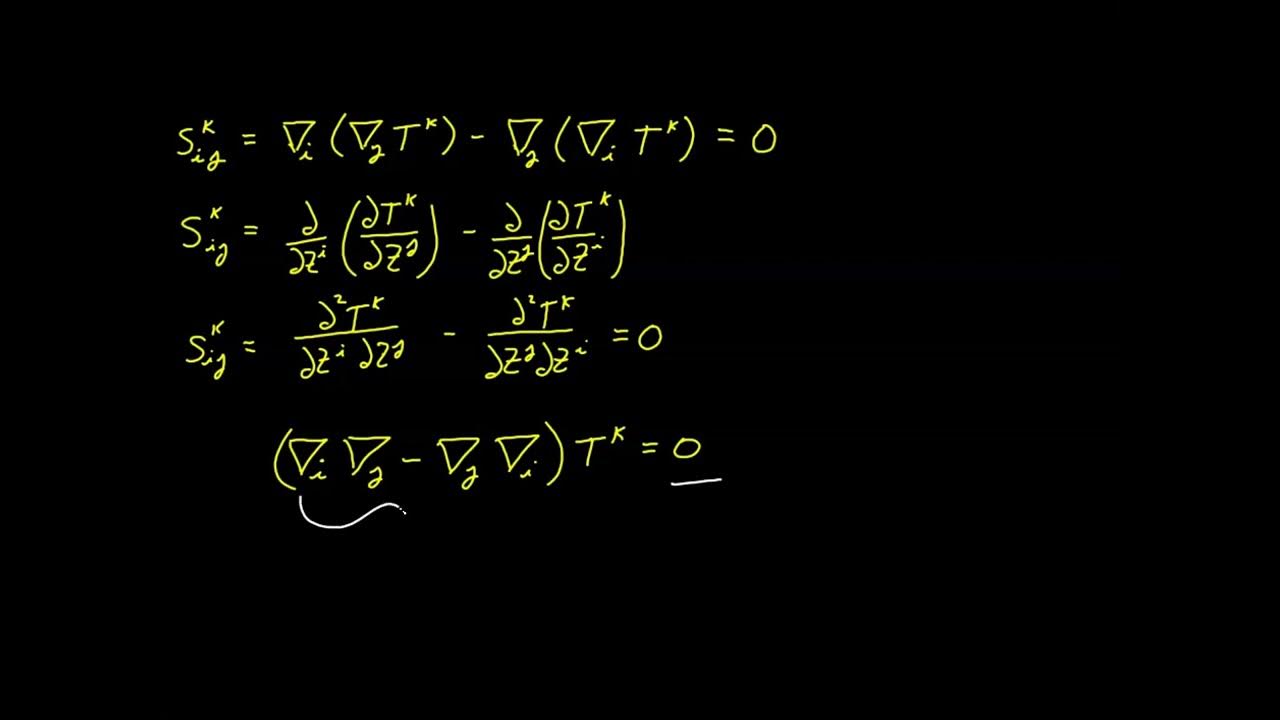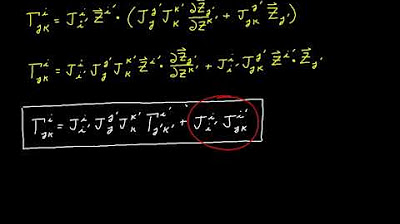Tensor Calculus 17.5: Covariant Derivative (Component Definition) - Optional

eigenchris
11 Nov 201816:50
EducationalLearning
32 Likes 10 Comments

TLDRThis video explores the concept of the covariant derivative, focusing on its component definition used in engineering-oriented classes. It clarifies the covariant derivative as both a vector field and a rank two tensor, explaining the geometric and component-based perspectives. The video also addresses the transformation of covariant derivatives between coordinate systems using Jacobian matrices and emphasizes that Christoffel symbols, despite their importance, are not tensors due to their unique transformation law.

Takeaways
  • 📚 The video discusses an engineering-oriented approach to the covariant derivative, which is a concept used in differential geometry and general relativity.
  • 🔍 Cartesian variables x and y are denoted as c1 and c2, while polar variables R and theta are denoted as p1 and p2, following the notation from a previous video.
  • 📈 The covariant derivative in flat space is explained as the ordinary derivative, with the use of product rule for differentiating both vector components and basis vectors.
  • 📘 Christoffel symbols are introduced as part of the notation for expressing the covariant derivative, which are defined in a specific mathematical way.
  • 🌐 The covariant derivative of a vector field is described as another vector field that indicates the rate of change of the original field in a given direction.
  • 🤔 A viewer's question about the covariant derivative being a rank two tensor is addressed, explaining the difference between the matrix (component) view and the vector field (geometric) view.
  • 📝 The covariant derivative is expanded in Cartesian coordinates and the transformation to polar coordinates or other systems is discussed, emphasizing the geometric consistency across different coordinate systems.
  • 📊 Engineers often use a specific notation for the components of the covariant derivative, focusing on the components rather than the full vector field.
  • 🔄 The transformation between covariant derivative components in different coordinate systems involves both contravariant and covariant transformation rules, using Jacobian and inverse Jacobian matrices.
  • 📐 Christoffel symbols are shown not to be tensors due to their unique transformation law, which includes an additional term not found in the tensor transformation law.
  • 🚫 A derivation is provided to demonstrate that Christoffel symbols do not adhere to the tensor transformation law, hence they cannot be represented as a tensor product of basis vectors and co-vectors.
Q & A

  • What is the main topic discussed in the video?

    -The main topic discussed in the video is the covariant derivative, specifically its component definition and its application in engineering-oriented classes.

  • What is the relationship between Cartesian variables and polar variables in the context of this video?

    -In the video, Cartesian variables x and y are denoted as c1 and c2, while polar variables R and theta are denoted as p1 and p2, indicating their use in different coordinate systems.

  • What is the covariant derivative in flat space as introduced in the video?

    -The covariant derivative in flat space is the ordinary derivative, used when differentiating a vector field with the product rule applied to both the vector components and the basis vectors.

  • What are Christoffel symbols and how are they used in the context of covariant derivatives?

    -Christoffel symbols are used to express the covariant derivative of a vector field. They are defined in a way that incorporates the differentiation of both the vector components and the basis vectors.

  • How does the video explain the rate of change of a vector field along a radial line?

    -The video explains that along a radial line, the rate of change of a vector field is represented by vectors pointing upward in the angular theta direction, indicating the increase in the component of the vectors in that direction.

  • Why might there be confusion about the covariant derivative being both a matrix and a vector field?

    -The confusion arises because the covariant derivative can be viewed from different perspectives: as a matrix focusing on components, often preferred by engineering students, or as a vector field focusing on geometry, preferred by pure mathematics students.

  • What is the difference between the matrix point of view and the vector field point of view when considering the covariant derivative?

    -The matrix point of view focuses on the components of the covariant derivative, treating it as a rank two tensor, while the vector field point of view emphasizes the geometrical aspect of the derivative, dealing with vectors in space.

  • How does the video explain the transformation of covariant derivative components between different coordinate systems?

    -The video explains that to transform covariant derivative components between different coordinate systems, one must use a contravariant transformation rule with the Jacobian and a covariant transformation rule with the inverse Jacobian.

  • Why are Christoffel symbols not considered tensors according to the video?

    -Christoffel symbols are not tensors because they do not transform with the expected tensor transformation law using Jacobians and inverse Jacobians; instead, they follow a more complex transformation law with an additional term.

  • What is the significance of the covariant derivative components forming a rank two tensor?

    -The significance is that the covariant derivative components, which have both contravariant and covariant indices, transform according to tensor transformation rules, allowing for a consistent description of the derivative across different coordinate systems.

  • How does the video address the transformation of Christoffel symbols between coordinate systems?

    -The video derives the transformation law for Christoffel symbols, showing that they transform with a complex law that includes an additional term beyond the standard tensor transformation, thus confirming they are not tensors.

Outlines
00:00
📚 Introduction to Covariant Derivative in Component Form

This paragraph introduces the concept of the covariant derivative from a component perspective, which is commonly used in engineering-oriented classes. It serves as a continuation of the flat space definition from a previous video. The Cartesian and polar variables are denoted as c1, c2 for Cartesian and p1, p2 for polar, respectively. The covariant derivative is explained in the context of vector fields and the use of Christoffel symbols is highlighted. An example is given to illustrate how the covariant derivative changes along a radial line in polar coordinates. The paragraph also addresses a viewer's question about the covariant derivative being a rank two tensor, explaining the difference between the matrix (component) view favored by engineers and the vector field (geometric) view preferred by mathematicians.

05:01
🔍 Deep Dive into Covariant Derivative Notation and Transformation

The second paragraph delves deeper into the notation used for the covariant derivative, particularly focusing on the engineering approach that treats it as a rank two tensor. It explains the use of semicolon notation to represent the components of the covariant derivative in different coordinate directions. The paragraph also reviews the concepts of covariant and contravariant indices, discussing how basis vectors and vector components transform under changes in the coordinate system. The transformation between covariant derivative components in Cartesian and polar coordinates is derived, showing that these components indeed form a rank two tensor, requiring both an inverse Jacobian and a Jacobian matrix for the transformation.

10:01
📐 Exploring the Geometry of Covariant Derivatives in 2D Space

This paragraph discusses the geometric implications of the covariant derivative in two-dimensional space. It explains how the covariant derivative of a vector field results in two vector fields corresponding to the main coordinate directions. The components of these vector fields are represented using the semicolon notation, and the transformation between these components using Jacobian and inverse Jacobian matrices is detailed. The paragraph emphasizes that the covariant derivative components form a tensor that follows both contravariant and covariant transformation rules.

15:02
🚫 Clarification on Christoffel Symbols Not Being Tensors

The final paragraph clarifies a common misconception by stating that Christoffel symbols are not tensors, despite their role in the transformation of vector field components. It presents a derivation of the transformation law for Christoffel symbols, showing that they do not follow the standard tensor transformation law due to an additional term in their transformation formula. The paragraph concludes by emphasizing that Christoffel symbols cannot be represented as a tensor independent of coordinates and cannot be written as a linear combination of tensor products of basis vectors and co-vectors.

Mindmap
Keywords
💡Covariant Derivative
The covariant derivative is a concept in differential geometry that generalizes the directional derivative to curved spaces. It measures how a vector field changes as one moves along a curve on a manifold. In the video, the covariant derivative is discussed in the context of flat space and its component definition, which is crucial for understanding how vector fields behave in different coordinate systems. The script explains that the covariant derivative of a vector field results in another vector field, illustrating this with the rate of change along radial and angular directions in polar coordinates.
💡Christoffel Symbols
Christoffel symbols are used in differential geometry to describe the change in basis vectors under a coordinate transformation. They are part of the language of tensor calculus and are essential in expressing the covariant derivative in a coordinate-free manner. The video script introduces Christoffel symbols in the context of the covariant derivative in flat space and discusses their role in writing the derivative using the product rule for differentiating both vector components and basis vectors.
💡Flat Space
Flat space, in the context of this video, refers to a geometric space that is not curved, such as Euclidean space. The script mentions a previous video that introduced the covariant derivative in flat space, which serves as a simpler introduction before moving on to more complex spaces. The concept is foundational as it provides a basis for understanding how derivatives work in more general, possibly curved, spaces.
💡Cartesian Coordinates
Cartesian coordinates are a coordinate system commonly used in mathematics where each point is defined by its x and y coordinates in a plane. In the script, the Cartesian variables x and y are relabeled as c1 and c2 to distinguish them when discussing the covariant derivative in different coordinate systems. The video uses Cartesian coordinates as a basis for explaining the covariant derivative and its expansion in various coordinate systems.
💡Polar Coordinates
Polar coordinates are an alternative coordinate system where each point is defined by its distance from the origin (R) and the angle it makes with the positive x-axis (theta). The video script uses polar coordinates to illustrate the covariant derivative in a more geometrically intuitive way, showing how the derivative changes when considering radial and angular directions.
💡Rank Two Tensor
A rank two tensor is a mathematical object that can be thought of as a matrix with two indices. In the video, a viewer's question about the covariant derivative being a rank two tensor is addressed, explaining that it can be viewed as such when considering its components. The script clarifies that the covariant derivative adds a covariant index to the tensor components, thus forming a rank two tensor.
💡Engineering vs. Pure Mathematics
The script contrasts the approaches of engineering and pure mathematics students to the concept of the covariant derivative. Engineering students are said to focus on the component approach, often using matrices and components, while pure mathematics students are described as favoring a more geometric approach. This distinction is important as it highlights different perspectives on the same mathematical concept.
💡Basis Vectors
Basis vectors are fundamental vectors in a vector space that can be combined to form any vector in that space. In the context of the video, basis vectors are used to express the covariant derivative in different coordinate systems. The script explains that basis vectors obey the covariant transformation rule and are written with lower indexes or covariant indexes.
💡Contravariant Components
Contravariant components are elements of a vector in a given coordinate system that transform in the opposite way to basis vectors under a change of coordinates. The video script discusses how vector components are contravariant and how they are represented with superscripts, contrasting this with the covariant transformation of basis vectors.
💡Jacobian Matrix
The Jacobian matrix is a matrix of all first-order partial derivatives of a vector-valued function. It is used to describe how a function changes as its input changes. In the script, the Jacobian matrix is used to convert between different sets of basis vectors and to transform the components of the covariant derivative from one coordinate system to another.
💡Transformation Law
A transformation law describes how quantities or components change under a transformation of coordinates. The video script derives the transformation law for the Christoffel symbols and shows that they do not follow the ordinary tensor transformation law due to an additional term, indicating that they are not tensors. This is a key point in understanding the geometric properties of the covariant derivative and its components.
Highlights

Introduction to the covariant derivative in the context of engineering-oriented classes.

Explanation of Cartesian and polar variable notation as c1/c2 and p1/p2 respectively.

Review of the covariant derivative in flat space from a previous video.

Differentiation of vector components and basis vectors using the product rule.

Introduction of Christoffel symbols in the expression of covariant derivatives.

Illustration of the covariant derivative of a vector field along a radial line.

Clarification of the covariant derivative of a vector field being both a matrix and a vector field.

Different perspectives on the covariant derivative between engineering and pure mathematics.

Geometric interpretation of the covariant derivative in the direction of a curve parameterized by lambda.

Expansion of the vector field and lambda derivative using multivariable chain rule.

Expression of the covariant derivative in Cartesian coordinates using Christoffel symbols.

Potential for expansion of the covariant derivative in various coordinate systems.

Discussion on the covariant derivative as a rank two tensor in engineering terms.

Symbolic representation of covariant derivative components with superscript and subscript notation.

Explanation of covariant and contravariant indexes in the context of basis vectors and vector components.

Conversion between covariant derivative components in different coordinate systems using Jacobian matrices.

Christoffel symbols are not tensors due to their unique transformation law.

Derivation of the transformation law for Christoffel symbols, highlighting their non-tensorial nature.

Summary of the video's content on the covariant derivative's geometric interpretation and tensorial properties.

Transcripts

Browse More Related Video

Tensor Calculus Lecture 6c: The Covariant Derivative 2

Video 19 - Tensor Definition

Video 33 - Covariant Derivative Proof

Video 36 - Covariant Derivative Properties

Video 29 - Christoffel Symbol Transformation

Video 11 - Vector Transformations

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Covariant DerivativeVector FieldChristoffel SymbolsEngineering MathPolar CoordinatesCartesian CoordinatesTensor TransformationMultivariable CalculusGeometric InterpretationDifferential Geometry