AP Physics B Kinematics Presentation #69
TLDRThe video script demonstrates a graphical method to determine the resultant vector of three displacement vectors. It involves plotting the vectors on a coordinate system, calculating their individual x and y components using trigonometric functions, and then summing these components to find the net x and y components. The resultant vector's direction is found by using the tangent inverse of the net y over x components, yielding an angle of approximately 39 degrees north of East.
Takeaways
- 📐 The task involves graphically determining the resultant of three vector displacements.
- 🔴 Vector 1 is 115 meters long at 30 degrees north of East.
- 🟢 Vector 2 is 20 meters long at 37 degrees north of East.
- 🟣 Vector 3 is 325 meters long at 45 degrees north of East (there seems to be a typo in the transcript, as it should be 25 meters instead of 325).
- 📈 The graphical representation involves drawing the vectors on a coordinate system with X and Y axes.
- 📏 The resultant vector (R) is drawn from the initial point to the final endpoint, connecting all vectors.
- 🧭 To find the direction of the resultant, calculate the net X and Y components of the vectors.
- 📉 The X component of a vector is found using the cosine of the angle it makes with the X axis.
- 📊 The Y component of a vector is found using the sine of the angle it makes with the X axis.
- 🔢 The net X and Y components are summed up for all vectors to find the resultant's components.
- 📍 The direction of the resultant vector is determined by the arctangent of the ratio of the Y component to the X component.
- 🌐 The final direction of the resultant vector is approximately 39 degrees north of East.
Q & A
What is the task described in the video script?
-The task is to graphically determine the direction of the resultant vector of three given vector displacements.
What are the three vector displacements mentioned in the script?
-The three vector displacements are 115 meters at 30 degrees north of East, 20 meters at 37 degrees north of East, and 325 meters at 45 degrees north of East.
What is the first step in solving the problem according to the script?
-The first step is to provide a graphical representation of the three vectors being added together on a coordinate system with X and Y axes.
How is the direction of the resultant vector determined?
-The direction of the resultant vector is determined by finding the net X and Y components of the three vectors and then calculating the angle these components make with the X-axis.
What mathematical function is used to find the X component of a vector?
-The cosine function (cos) is used to find the X component of a vector, as it represents the adjacent side in a right-angled triangle formed by the vector and the X-axis.
What mathematical function is used to find the Y component of a vector?
-The sine function (sin) is used to find the Y component of a vector, as it represents the opposite side in the same right-angled triangle.
How is the resultant vector's direction angle calculated?
-The resultant vector's direction angle is calculated using the tangent inverse function (tan^-1), where the tangent of the angle is the ratio of the Y component to the X component of the resultant vector.
What is the final calculated angle for the resultant vector direction?
-The final calculated angle for the resultant vector direction is approximately 39 degrees north of East.
Why is the script emphasizing the use of SOHCAHTOA in determining the direction of the resultant vector?
-SOHCAHTOA is a mnemonic for remembering the trigonometric ratios sine, cosine, and tangent, which are essential in determining the direction of the resultant vector by relating the components to the angle they form with the X-axis.
What is the significance of the graphical representation in understanding the problem?
-The graphical representation helps visualize the vectors and their components, making it easier to understand how they combine to form the resultant vector and its direction.
What is the purpose of creating a table for the X and Y components of each vector?
-The table organizes the calculated components of each vector, making it easier to sum them up and find the net components, which are necessary for determining the resultant vector's direction.
Outlines
📏 Vector Addition and Resultant Direction Calculation
This paragraph explains the graphical process of determining the resultant vector of three displacement vectors. It begins by setting up a coordinate system with X and Y axes and then illustrates the addition of three vectors with different magnitudes and angles relative to the East direction. The first vector is 115 meters at 30 degrees north of East, the second is 20 meters at 37 degrees north of East, and the third is 325 meters at 45 degrees north of East. The resultant vector, represented in purple, is the vector that connects the initial starting point to the final endpoint of the combined vectors. The paragraph details the method of finding the net X and Y components of the vectors by using trigonometric functions such as cosine and sine, and then uses these components to determine the angle of the resultant vector with respect to the X-axis.
📉 Calculating Components and Resultant Angle
The second paragraph continues the process of vector addition by calculating the X and Y components of each vector using trigonometric functions. It provides the specific calculations for the X and Y components of the second and third vectors, which are 20 meters at 37 degrees and 325 meters at 45 degrees, respectively. The paragraph then sums up the X and Y components of all three vectors to find the net components, resulting in a total X component of 47 meters and a total Y component of 38 meters. Using the tangent function and the arctangent (inverse tangent), the paragraph concludes by determining the angle of the resultant vector to be approximately 39 degrees north of East, which is the final answer to the problem presented in the script.
Mindmap
Keywords
💡Graphical Representation
💡Vector Displacements
💡Axes
💡Resultant Vector
💡Angle
💡Trigonometric Functions
💡Component
💡Net X Component
💡Net Y Component
💡Direction
💡Tangent
Highlights
Graphical representation of three vector displacements is used to determine the resultant vector.
Axes are established with X going horizontally and Y vertically.
Vector 1 is 115 meters at 30 degrees north of East, drawn in red.
Vector 2 is 20 meters at 37 degrees north of East, shown in green.
Vector 3 is 325 meters at 45 degrees north of East, represented in purple.
The resultant vector R connects the initial starting point to the final endpoint.
Net x and y components of the vectors are calculated to find the direction of the resultant.
Cosine and sine functions are used to determine the x and y components of each vector.
For Vector 1, the x component is calculated using cos(30°), resulting in approximately 13 meters.
The y component of Vector 1 is found using sin(30°), equaling 7.5 meters.
For Vector 2, the x component is calculated with cos(37°), yielding approximately 16 meters.
The y component of Vector 2 is determined using sin(37°), resulting in approximately 12 meters.
For Vector 3, both x and y components are calculated using cos(45°) and sin(45°), both equaling 18 meters.
The net x component of the resultant vector is the sum of the x components of the three vectors, totaling 47 meters.
The net y component of the resultant vector is the sum of the y components, totaling 38 meters.
The direction of the resultant vector is determined using the tangent function and inverse tangent (arctan).
The angle of the resultant vector relative to the x-axis is approximately 39 degrees north of East.
Transcripts
Browse More Related Video

AP Physics B Kinematics Presentation #68
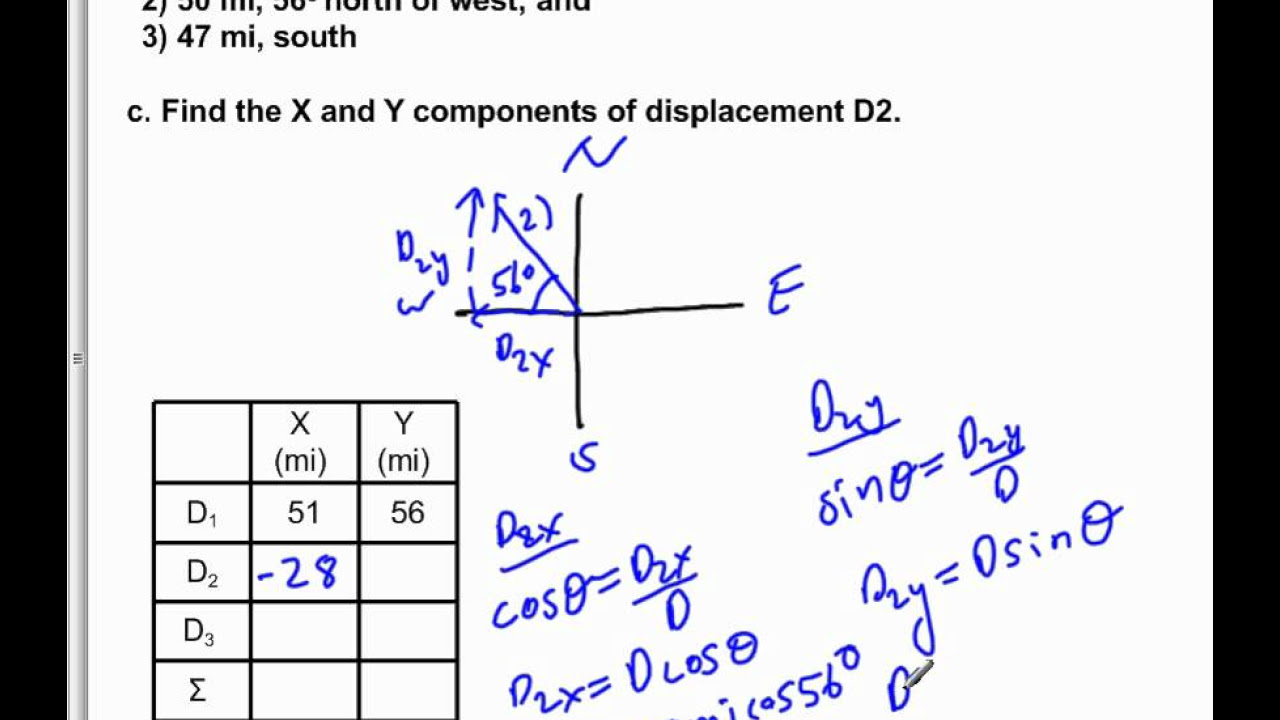
AP Physics B Kinematics Presentation General Problems #08

Adding Vector Components Example | Physics with Professor Matt Anderson | M3-11
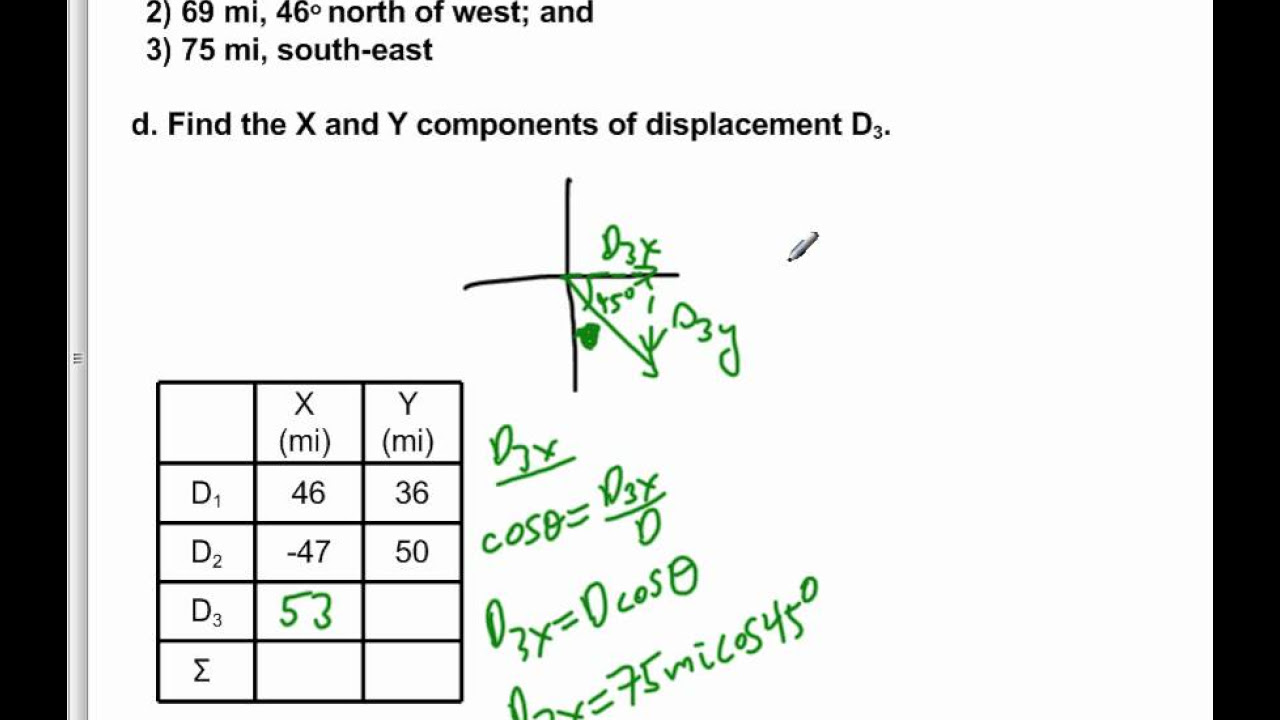
AP Physics B Kinematics Presentation General Problems #09
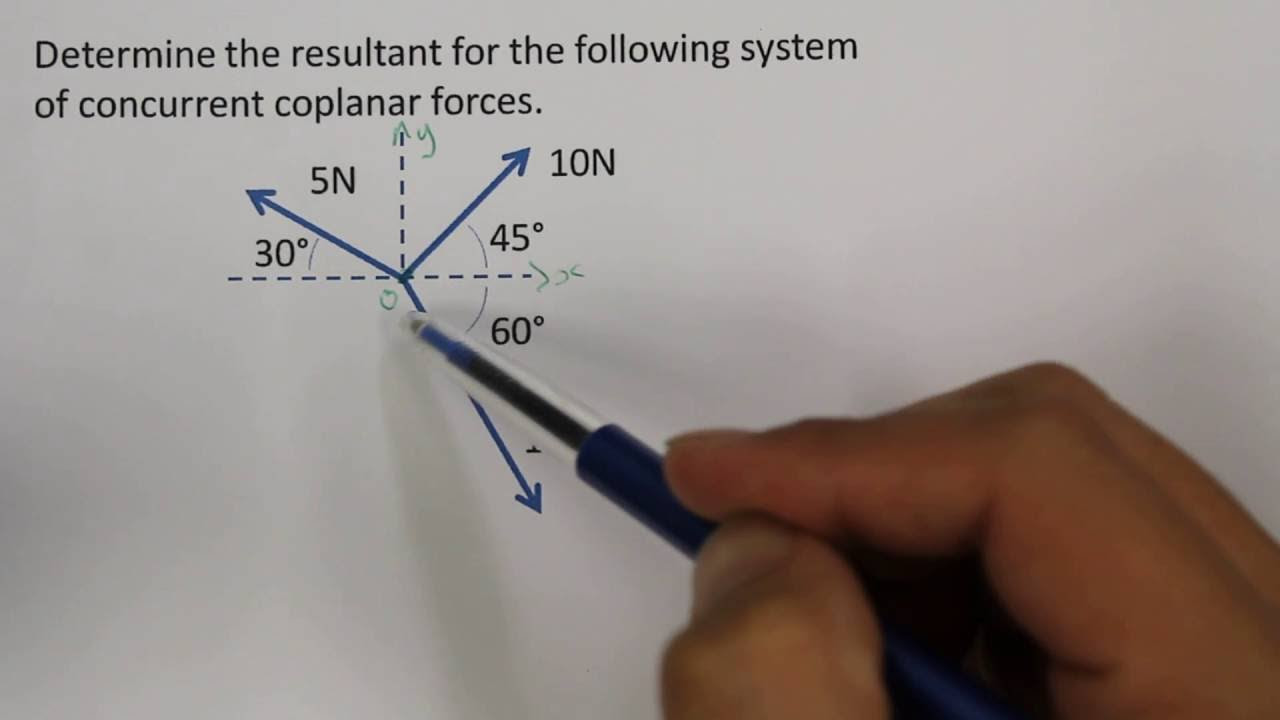
Resultant of Three Concurrent Coplanar Forces
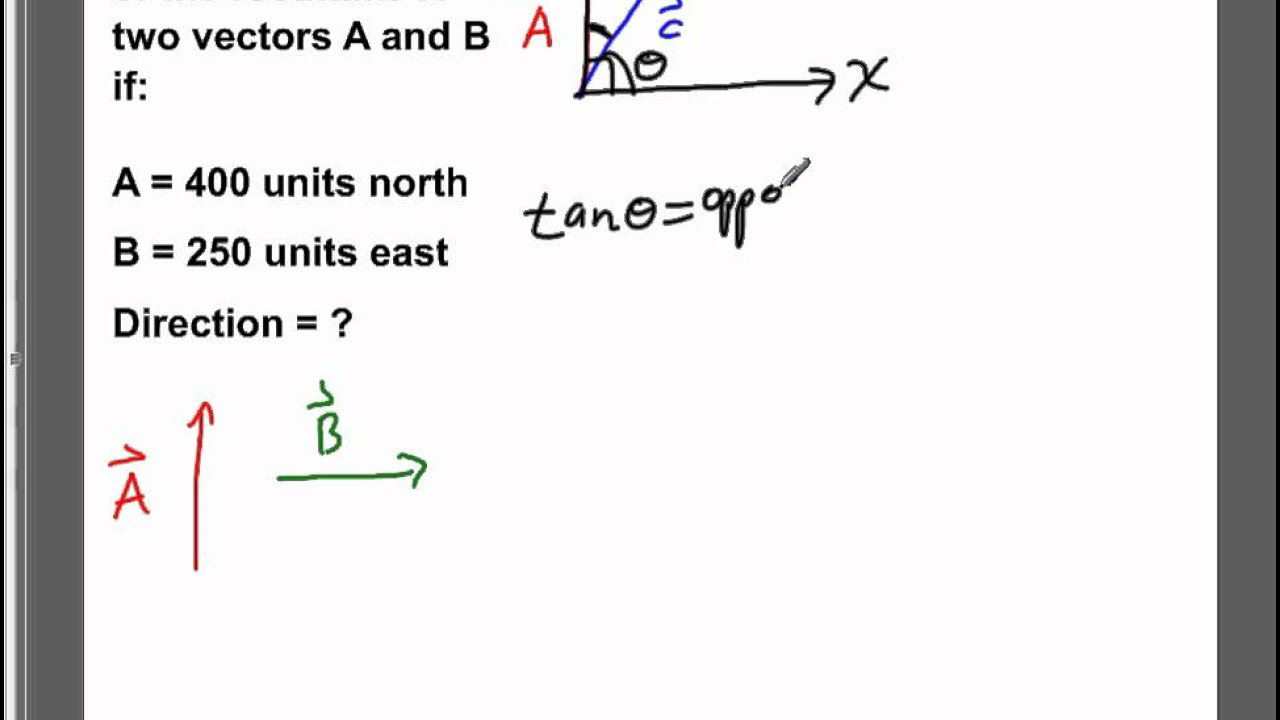
AP Physics B Kinematics Presentation #51
5.0 / 5 (0 votes)
Thanks for rating: