AP Physics B Kinematics Presentation General Problems #09
TLDRThe script provides a detailed explanation of calculating the resultant vector from three displacement vectors. It involves drawing vectors on a graph, finding their X and Y components using trigonometric functions, and applying the Pythagorean theorem to find the magnitude of the resultant. The direction of the resultant is determined using the arctan function. The process emphasizes the importance of keeping units consistent and accurate calculations for significant figures.
Takeaways
- 📚 The script describes a process of analyzing and calculating the resultant vector from three displacement vectors.
- 🚍 The bus's displacements are given in terms of direction and distance, with angles relative to the cardinal directions.
- 📏 The first displacement is 158 miles at 38 degrees north of east.
- 🔄 The second displacement is 269 miles at 46 degrees north of west.
- 🔽 The third displacement is 375 miles in the southeast direction, which is a 45-degree angle from south and east.
- 📈 A clear diagram is essential for visualizing the vectors and calculating the resultant vector.
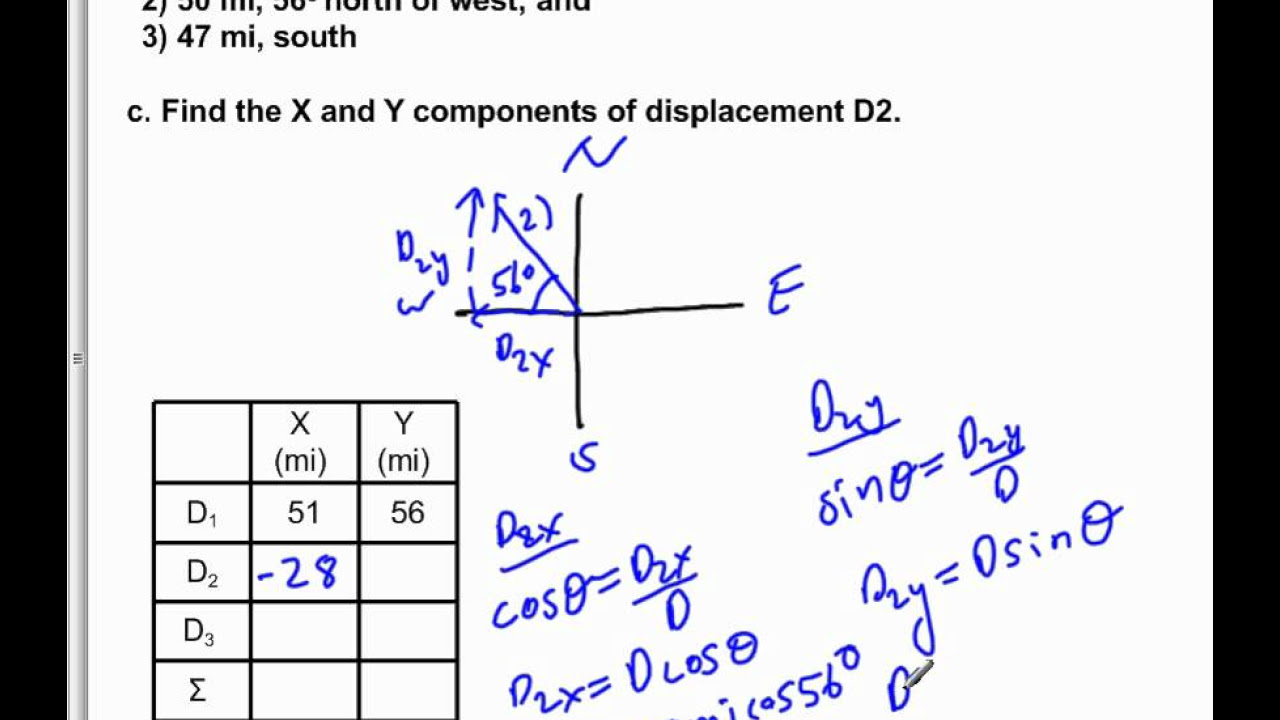
- 🧭 The use of trigonometric functions (SOHCAHTOA) is necessary to find the x and y components of each displacement vector.
- 📐 The x and y components for each vector are calculated using cosine and sine of the respective angles.
- ➕ The resultant vector is found by summing the x components and y components of the individual vectors.
- 🔢 The magnitude of the resultant vector is calculated using the Pythagorean theorem, considering the sum of the squares of the x and y components.
- 📊 The direction of the resultant vector is determined using the inverse tangent function (tan^-1) of the ratio of the y components to the x components.
- 📝 The importance of maintaining correct units and significant figures throughout the calculations is emphasized.
Q & A
What are the three displacement vectors described in the script?
-The three displacement vectors are: 158 miles at 38 degrees north of east, 269 miles at 46 degrees north of west, and 375 miles in the southeast direction.
How is the first displacement vector represented in terms of direction?
-The first displacement vector is represented as 158 miles at an angle of 38 degrees north of east.
What is the significance of the angle in the description of the second displacement vector?
-The angle in the second displacement vector, which is 46 degrees north of west, indicates the direction of the vector relative to the westward direction.
What does the term 'southeast' imply about the third displacement vector?
-The term 'southeast' implies that the third displacement vector is at a 45-degree angle with respect to both the south and east directions, pointing directly between them.
How is the resultant vector determined from the three displacement vectors?
-The resultant vector is determined by adding the endpoint of the first vector to the origin of the third vector, giving a general direction in the northeast with both positive X and Y components.
What trigonometric method is used to find the X and Y components of the displacement vectors?
-The SOHCAHTOA method is used to find the X and Y components of the displacement vectors by applying the cosine and sine functions to the angles and magnitudes of the vectors.
What is the significance of keeping track of the direction when calculating the X and Y components?
-Keeping track of the direction is crucial because it determines whether the components are positive or negative, which affects the final calculation of the resultant vector.
How are the magnitudes of the X and Y components of the first displacement vector calculated?
-The magnitudes are calculated using the cosine for the X component (46 miles) and the sine for the Y component (36 miles) of the 38-degree angle.
What is the process for finding the magnitude of the resultant vector?
-The process involves summing the X components and the Y components of all displacement vectors, then applying the Pythagorean theorem to find the magnitude of the resultant vector.
How is the direction of the resultant vector determined?
-The direction of the resultant vector is determined by calculating the arctangent (inverse tangent) of the ratio of the sum of the Y components to the sum of the X components, which gives an angle with respect to the x-axis.
What is the importance of considering significant figures in the calculations?
-Considering significant figures ensures the accuracy of the results, especially when rounding off the values to match the precision given in the original data.
Outlines
🚍 Displacement Vectors and Resultant Calculation
This paragraph describes the process of visualizing and calculating the resultant vector of a bus's displacements. The bus undergoes three movements in different directions: 158 miles at 38 degrees north of east, 269 miles at 46 degrees north of west, and 375 miles southeast. The paragraph explains how to draw these vectors on a diagram with a clear reference to north, east, south, and west. It also details the calculation of the resultant vector's direction and magnitude using the individual vector endpoints, emphasizing the need for accuracy in the diagram and calculations.
📏 Calculating Displacement Vector Components
The second paragraph focuses on the mathematical breakdown of the displacement vectors using trigonometric principles. It explains how to find the x and y components of each displacement vector by applying the SOHCAHTOA method. The paragraph provides step-by-step calculations for the x and y components of the first two vectors, taking into account the direction (positive or negative) of each component. It also highlights the importance of keeping units consistent and applying the correct trigonometric functions for each vector's angle.
🧭 Determining Components and Magnitude of the Third Displacement
This paragraph continues the theme of calculating displacement vector components but applies it to the third vector, which is 75 miles in the southeast direction at a 45-degree angle. The explanation includes using cosine and sine functions to find the x and y components of this vector, emphasizing the correct application of the 45-degree angle for both components. The paragraph also leads into the calculation of the resultant vector's magnitude by summing the x and y components of all three vectors and applying the Pythagorean theorem.
📐 Resultant Vector Magnitude and Direction Calculation
The final paragraph concludes the process by calculating the magnitude and direction of the resultant vector. It describes summing the x and y components from the previous calculations and using these sums to find the magnitude of the resultant vector using the Pythagorean theorem. The paragraph also explains how to find the direction of the resultant vector by using the inverse tangent function, taking the ratio of the summed y components to the summed x components. The importance of maintaining significant figures in the calculations is emphasized, and the final direction of the resultant vector is determined to be northeast at an angle of 32 degrees.
Mindmap
Keywords
💡Displacement
💡Vector
💡Sohcahtoa
💡Resultant Vector
💡Magnitude
💡Direction
💡Trigonometric Functions
💡Pythagorean Theorem
💡Significant Figures
💡Tangent
Highlights
A bus makes three displacements with specific directions and distances.
The first displacement is 158 miles at 38 degrees north of east.
The second displacement is 269 miles at 46 degrees north of west.
The third displacement is 375 miles in the southeast direction.
A diagram is drawn to visualize the three displacement vectors.
The resultant vector is calculated to determine the final position.
The X and Y components of each displacement are calculated using trigonometric functions.
SOHCAHTOA is applied to find the components of the first displacement.
The X component of the first displacement is found to be 46 miles.
The Y component of the first displacement is 36 miles.
The second displacement's X component is calculated as -47 miles, indicating a negative direction.
The Y component of the second displacement is 50 miles, pointing in the positive Y direction.
The third displacement's components are calculated with a 45-degree angle.
The X component of the third displacement is 53 miles, pointing in the positive X direction.
The Y component of the third displacement is -53 miles, indicating a negative Y direction.
The magnitude of the resultant vector is calculated to be approximately 62 miles.
The direction of the resultant vector is found to be 32 degrees from the x-axis.
The importance of checking significant figures in calculations is emphasized.
The process demonstrates the application of vector addition and trigonometry in real-world scenarios.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: