Gauss law of electricity | Electrostatics | Physics | Khan Academy
TLDRThis educational video script delves into the concept of electric flux, starting with a basic scenario of a positively charged sphere and its surrounding electric field. It explains how to calculate flux using the integral of the electric field vector dot product with a surface element vector, emphasizing the role of the cosine of the angle between them. The script explores how flux remains constant regardless of the sphere's size or position due to the inverse square law, leading to Gauss's law, which states that the total electric flux through a closed surface equals the enclosed charge divided by the permittivity of free space. The script concludes by highlighting the generality of Gauss's law in electrostatics.
Takeaways
- ⚡ The video aims to explore Gauss's law of electricity, starting with basic concepts and progressing to intricate details.
- 🔋 A positive charge at the center of a sphere creates an electric field, leading to the question of calculating electric flux through the sphere.
- 📐 Electric flux (Φ) is defined as the integral of the electric field (E) dot product with the area vector (dA), mathematically expressed as Φ = ∫E·dA.
- 🎓 Flux depends on the perpendicular component of the electric field to the area vector, introducing a cosine (cos θ) term in the calculation.
- 🌐 For a sphere, the electric field is constant on the surface, simplifying the flux calculation as the product of the electric field and the total surface area.
- 🔢 The flux through a sphere with a charge at the center is calculated to be Φ = Q/ε₀, where Q is the charge and ε₀ is the permittivity of free space.
- 🔄 The flux remains the same regardless of the sphere's radius due to the inverse square law of the electric field.
- 📍 Even if the charge is moved off-center within the sphere, the flux remains the same, demonstrating the independence of flux from the charge's position.
- 🕳️ The surface must be closed for the flux calculation to hold; holes in the surface would lead to inaccuracies as some electric field lines would escape.
- 🌟 Gauss's law generalizes to any closed surface, stating that the flux through the surface equals the total charge enclosed divided by ε₀, independent of the shape of the surface or the position of the charge within it.
Q & A
What is the main topic of the video?
-The main topic of the video is to explore the concept of electric flux and Gauss's Law in the context of electricity.
How is electric flux defined in the video?
-Electric flux, denoted as phi, is defined as the integral of the electric field (E) dot the differential area (dA), multiplied by the cosine of the angle (theta) between them.
What is the significance of the cosine term in the electric flux formula?
-The cosine term accounts for the perpendicular component of the area vector (dA) through which the electric field is considered to flow, as the electric field can only 'see' the area that is perpendicular to its direction.
Why is the electric field considered to be constant on the surface of a sphere with a point charge at its center?
-The electric field is considered constant because every point on the surface of the sphere is at the same distance from the point charge, resulting in the same electric field strength according to the inverse square law.
What happens to the angle theta between the electric field and the area vector on a sphere with a point charge at its center?
-The angle theta is zero everywhere on the surface of the sphere because the electric field lines are always perpendicular to the surface, aligning with the radius of the sphere.
How does the size of the sphere affect the electric flux through it when a point charge is at its center?
-The size of the sphere does not affect the electric flux. Even if the sphere's radius changes, the flux remains the same due to the inverse square law, which causes the increase in area and the decrease in electric field strength to cancel each other out.
What would be the effect on electric flux if the charge is moved off-center within the sphere?
-If the charge is moved off-center, the electric field would no longer be constant across the sphere's surface, and the angle theta would vary, making the calculation of electric flux more complex. However, the total flux through the entire surface remains the same due to the conservation of electric field lines.
What is the relationship between the electric flux through a closed surface and the total charge enclosed, according to Gauss's Law?
-According to Gauss's Law, the total electric flux through a closed surface is equal to the total charge enclosed divided by the permittivity of free space (epsilon naught).
How does the presence of multiple charges affect the electric flux through a closed surface?
-The electric flux through a closed surface is the sum of the individual contributions from each enclosed charge, divided by the permittivity of free space.
Why does a charge outside the closed surface not contribute to the electric flux through the surface?
-A charge outside the closed surface does not contribute to the electric flux because the electric field lines entering the surface from the outside charge are exactly balanced by the lines exiting the surface, resulting in no net flux.
What is the significance of Gauss's Law in electrostatics?
-Gauss's Law is significant in electrostatics as it provides a fundamental relationship between the electric field and the charge distribution. It is a general law that applies to any closed surface and any charge distribution, simplifying the calculation of electric fields in various situations.
Outlines
📚 Introduction to Electric Flux
The video begins by setting the stage for an exploration of the law of electricity, specifically focusing on the concept of electric flux. The host introduces a hypothetical scenario involving a positively charged sphere and the resulting electric field. The primary question posed is how to calculate the electric flux through the sphere. The explanation delves into the definition of flux as the integral of the electric field (E) dot product with a differential area (dA), taking into account the cosine of the angle (theta) between the field and the area vector. The significance of the angle and the perpendicular component of the area vector to the field is emphasized, leading to the understanding that the flux is calculated using the dot product of E and dA. The recap encourages viewers to revisit previous material if they are not familiar with the concept.
🔍 Calculating Flux Through a Sphere
This paragraph continues the discussion on electric flux, focusing on calculating the flux through a sphere with a point charge at its center. The host guides the viewer through the process of determining the angle between the electric field and the differential area vector (dA), concluding that the angle is zero everywhere on the sphere due to the field's alignment with the radius. This simplifies the calculation because cos(0) equals 1. The electric field is identified as constant across the sphere's surface because all points are equidistant from the charge. The integral of dA over the sphere's surface is the total surface area, which is 4πr². Using Coulomb's law, the electric field E is expressed as q/(4πε₀r²), and the total flux is found to be q/ε₀ after simplification. The host also discusses the implications of changing the sphere's size and position, highlighting the independence of flux from the sphere's size due to the inverse square law of the electric field.
🌐 Gauss's Law and Flux Independence
The host introduces Gauss's law by exploring the implications of moving the charge off-center and the effect on the electric flux through the sphere. Initially, the derivation becomes more complex due to the non-uniform electric field and varying angle theta. However, the host reveals that the flux remains unchanged, despite the charge's displacement, which is a surprising result attributed to the inverse square law. The concept is further expanded by imagining the electric field as 'bullets' being emitted by the charge, illustrating that the flux, or the number of 'bullets' passing through the sphere, remains constant regardless of the sphere's position or the charge's location. The host also touches on scenarios involving multiple charges and closed surfaces, leading to the general principle that the flux through a closed surface equals the total charge enclosed, divided by the permittivity of free space (ε₀).
🌟 Gauss's Law in Electrostatics
The final paragraph encapsulates the essence of Gauss's law in electrostatics. The host reiterates that the law, derived for a simple case of a charge at the center of a sphere, is universally applicable to any closed surface, regardless of its shape or the position of the charge. The law states that the total electric flux through a closed surface is equal to the total charge enclosed by the surface, divided by the permittivity of free space (ε₀). The host emphasizes that charges outside the surface do not affect the flux, and the law holds true even if there are multiple charges or if the surface has an irregular shape, as long as it is closed. The paragraph concludes by highlighting the generality and importance of Gauss's law in understanding electrostatic fields.
Mindmap
Keywords
💡Electric Flux
💡Coulomb's Law
💡Gauss's Law
💡Point Charge
💡Permittivity of Free Space (Epsilon Naught)
💡Inverse Square Law
💡Closed Surface
💡Vector Form of Electric Flux
💡Differential Area Vector (dA)
💡Cosine Theta (cos θ)
💡Charge Enclosure
Highlights
The video explores the concept of electric flux and its relation to the law of electricity.
Introduction of a hypothetical scenario with a positively charged sphere and its resulting electric field.
Explanation of electric flux calculation using the integral of the electric field dot product with the area vector.
Clarification of the role of cos(theta) in the flux calculation, representing the perpendicular component of the electric field.
Illustration of the dot product between the electric field and area vector to determine the flux.
The electric field's uniformity across the surface of a sphere due to the charge being at the center.
Flux simplification due to the angle theta being zero everywhere on the sphere's surface.
Demonstration that the electric field's magnitude remains constant on the sphere's surface.
The derivation of the flux formula, showing it to be independent of the sphere's size.
Explanation of why the flux remains constant even when the sphere's size changes, due to the inverse square law.
Discussion on the effect of moving the charge off-center and its impact on the electric field and flux.
Introduction of Gauss's Law and its relation to the electric flux through a closed surface.
The proof that the electric flux remains the same regardless of the surface's shape or the charge's position inside the surface.
Gauss's Law's application to scenarios with multiple charges, where the total flux is the sum of individual charges' contributions.
Clarification that external charges do not affect the flux through a closed surface, despite affecting the electric field.
The general formulation of Gauss's Law, emphasizing its applicability to any closed surface and its independence from external charges.
The philosophical and practical implications of Gauss's Law in understanding electrostatics.
Transcripts
Browse More Related Video
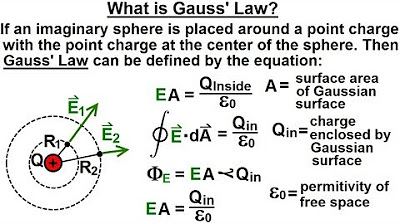
Physics 37.1 Gauss's Law Understood (3 of 29) What is Gauss' Law?
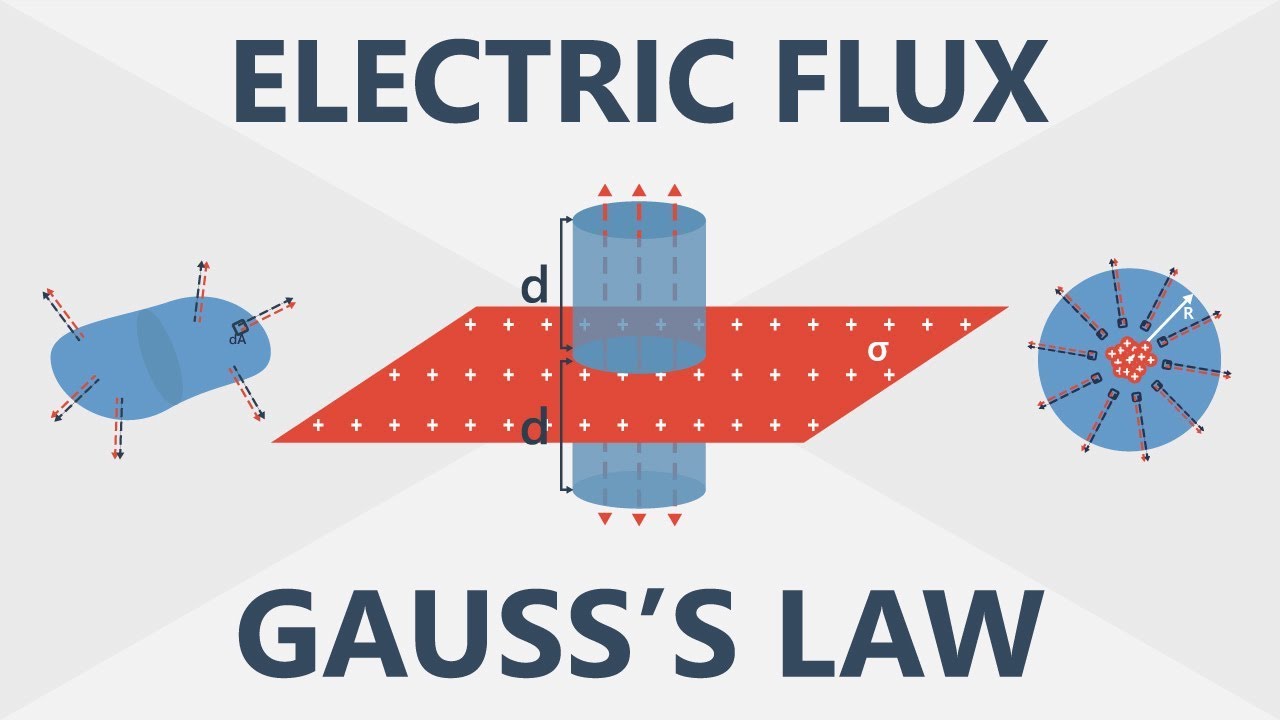
Electric Flux and Gauss’s Law | Electronics Basics #6
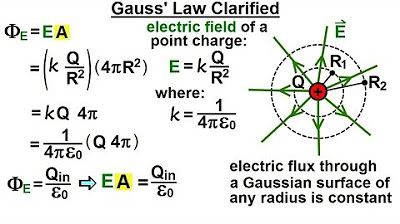
Physics 37.1 Gauss's Law Understood (4 of 29) Gauss' Law Clarified
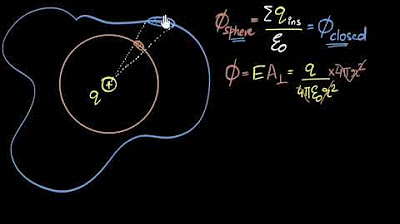
Gauss law logical proof (any closed surface) | Electric charges & fields | Physics | Khan Academy
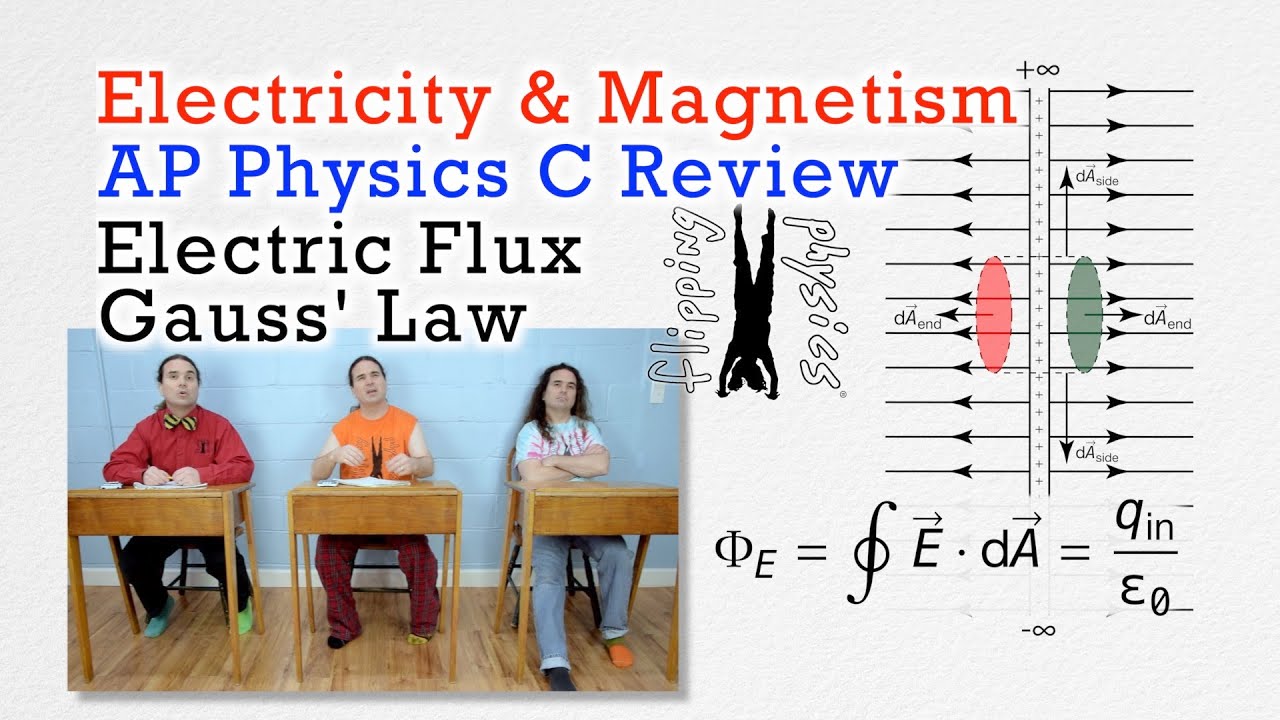
Electric Flux and Gauss' Law - Review for AP Physics C: Electricity and Magnetism
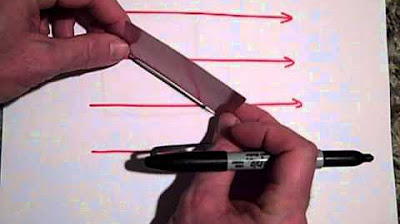
Electric Flux (part 1)
5.0 / 5 (0 votes)
Thanks for rating: