Electric Flux and Gauss' Law - Review for AP Physics C: Electricity and Magnetism
TLDRThis video script from Flipping Physics dives into the concepts of electric flux and Gauss' law within the context of AP Physics C: Electricity and Magnetism. It explains electric flux as the measure of electric field passing through a surface, using the dot product and cosine function to determine its value. The script explores Gauss' law through various examples, including a uniform electric field through a triangular box and a sphere around a point charge, demonstrating how the law relates to the enclosed charge and the permittivity of free space. It also touches on the implications of Gauss' law for different symmetrical charge distributions and concludes with a discussion on the electric field of a uniformly charged sphere, highlighting its equivalence to that of a point charge outside its surface.
Takeaways
- 🔋 Electric flux is a measure of the effect of an electric field passing through a surface, and it does not necessarily imply movement of the field itself.
- 📚 The symbol for electric flux is Φ_E, and it is calculated using the equation Φ_E = E • A cos(θ), where E is the electric field, A is the area, and θ is the angle between the field and the area vector.
- 📏 Electric flux is a scalar quantity, and its unit is newtons times square meters per coulomb (N·m²/C).
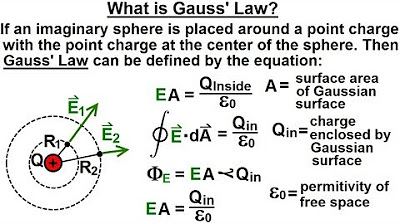
- 🌐 Gauss' law is foundational in electromagnetism and states that the net electric flux through a closed surface is equal to the charge enclosed divided by the permittivity of free space.
- 📦 When calculating electric flux through a closed surface, such as a right triangular box, the sum of the fluxes through all sides must be considered.
- 🔄 The directionality of electric flux is important; it can be positive or negative depending on whether the electric field is entering or exiting the surface.
- 🌀 For a uniform electric field, the electric field's magnitude is constant at a constant radius, which simplifies the calculation of flux through a symmetric surface like a sphere.
- 📈 Gauss' law is particularly useful for calculating electric fields in highly symmetrical situations, such as spherical, cylindrical, or planar charge distributions.
- 💡 The electric field outside a uniformly charged sphere behaves as if the sphere were a point charge, regardless of the sphere's size or the distance from it.
- 📊 The choice of Gaussian surface is crucial and should align with the symmetry of the charge distribution to simplify the calculation of electric flux.
- 🚀 The concepts of electric flux and Gauss' law are essential for understanding electromagnetism and are part of the AP Physics C: Electricity and Magnetism curriculum.
Q & A
What is the term 'flux' defined as in the context of physics?
-In physics, 'flux' refers to any effect that appears to pass or travel through a surface or substance, with the understanding that the effect does not necessarily need to actually move.
What is the symbol for electric flux and how is it related to the electric field?
-The symbol for electric flux is an uppercase Phi (Φ). When a capital E subscript is added, it represents electric flux, which is a measure of the amount of electric field that passes through a defined area.
What is the equation for electric flux of a uniform electric field through a two-dimensional area?
-The equation for electric flux (Φ_E) of a uniform electric field through a two-dimensional area is Φ_E = E • A • cos(θ), where E is the electric field, A is the area, and θ is the angle between the direction of the electric field and the direction of the area.
Why is the electric flux a scalar and not a vector?
-Electric flux is a scalar because it results from the dot product of the electric field vector and the area vector, which gives a magnitude only, without direction.
What is the unit for electric flux given the units for electric field and area?
-The units for electric flux are newtons times square meters divided by coulombs (N⋅m²/C), given that the electric field is in newtons per coulomb (N/C) and area is in square meters (m²).
How does the net electric flux through a closed surface relate to the charge enclosed by the surface?
-According to Gauss' law, the net electric flux through a closed surface equals the charge enclosed by the surface divided by the permittivity of free space, regardless of the size or shape of the surface, as long as the enclosed charge is constant.
What is the significance of choosing a Gaussian surface for calculating electric flux?
-A Gaussian surface is chosen to simplify the calculation of electric flux by ensuring that the electric field is either perpendicular or parallel to the surface, which makes the cosine of the angle between the field and the area either 1, 0, or -1, simplifying the integral.
How does the electric field caused by a point charge behave as you move away from the charge?
-The electric field caused by a point charge decreases as you move farther from the point charge, following an inverse-square law, meaning the field strength is inversely proportional to the square of the distance from the charge.
What is the electric field surrounding an infinitely large, thin plane of positive charges with uniform surface charge density?
-The electric field surrounding an infinitely large, thin plane of positive charges with uniform surface charge density is uniform in magnitude and directed away from the plane, with its strength equal to the surface charge density divided by the quantity two times the permittivity of free space (σ/(2ε₀)).
How does the presence of an equal magnitude, parallel, infinite plane of negative charges affect the electric field between and outside two parallel planes of charges?
-Outside the two parallel, oppositely charged planes, the electric fields cancel each other out, resulting in a net electric field of zero. Between the two planes, the electric fields add up, doubling the electric field strength of a single plane (2σ/ε₀).
What is the electric field outside a uniformly charged sphere, and how does it compare to that of a point charge?
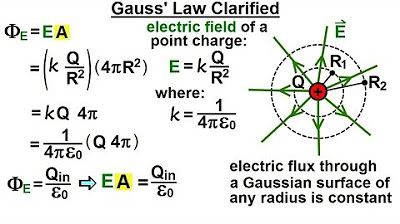
-Outside a uniformly charged sphere, the electric field is the same as if the sphere were a point charge, with the field strength given by the Coulomb's law formula (kQ/r²), where k is the Coulomb's constant, Q is the total charge, and r is the distance from the center of the sphere.
Outlines
📚 Introduction to Electric Flux and Gauss' Law
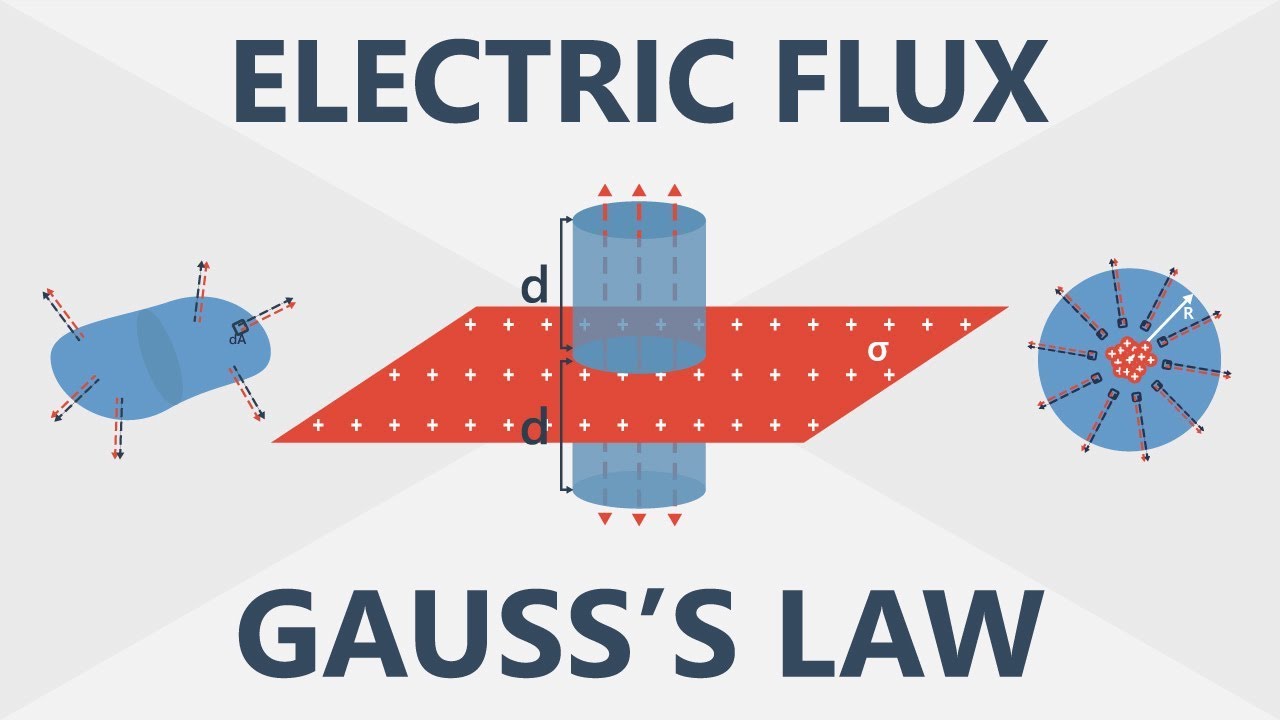
The script begins with an introduction to electric flux and Gauss' law in the context of AP Physics C: Electricity and Magnetism. It explains that electric flux is a measure of the electric field passing through a defined area and is symbolized by uppercase Phi with a subscript E. The equation for electric flux through a uniform electric field is discussed, highlighting its similarity to the work equation in physics. The script also delves into the concept of a closed surface and its relevance to calculating net electric flux, using a right triangular box as an example to illustrate the process.
🔍 Calculating Electric Flux Through Various Surfaces
This paragraph explores the calculation of electric flux through different surfaces of a right triangular box, considering the angle between the electric field and the area vector. It explains that the electric flux is negative when the electric field is entering a closed surface and positive when it is exiting. The discussion includes the flux through the rectangular sides, triangular sides, and the top surface of the box, leading to the conclusion that the net electric flux through the closed surface sums up to zero.
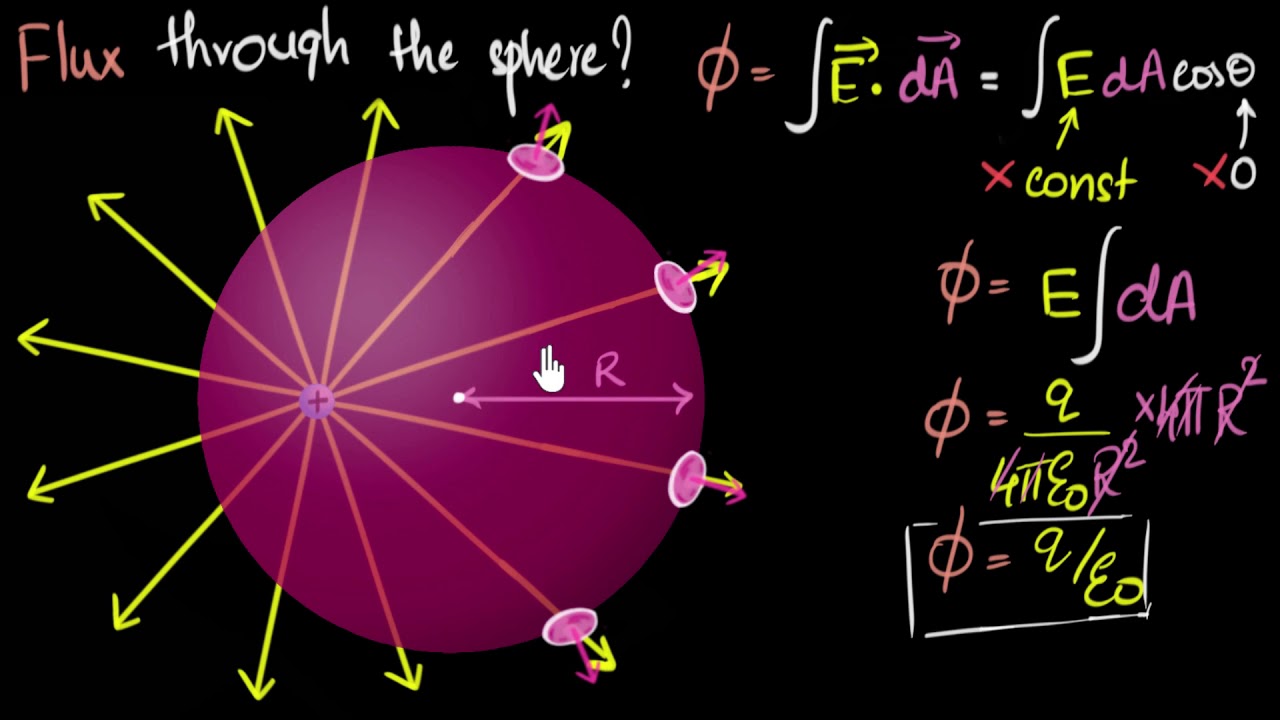
🌐 Gauss' Law and Electric Flux Through a Sphere
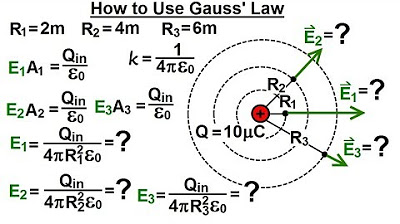
The script moves on to apply Gauss' law to calculate the electric flux through a sphere concentric with a point charge. It clarifies that the electric field around a point charge is not uniform, necessitating an integral approach. The electric field is constant at a constant radius, allowing for simplification using Coulomb's law. The final result demonstrates that the electric flux through the sphere is equal to the charge of the point charge divided by the permittivity of free space, regardless of the sphere's radius, showcasing Gauss' law in action.
📘 Advanced Gauss' Law Applications
This section delves deeper into the specifics of using Gauss' law, emphasizing the selection of Gaussian surfaces that simplify calculations due to the electric field's alignment with the surface normals. The script discusses the independence of electric flux from the size of the Gaussian surface when the enclosed charge is constant. It also introduces the concept of charge densities and their application in solving for electric fields using Gauss' law, specifically for symmetric charge distributions.
🌌 Electric Field Around an Infinite Charged Plane
The script presents an example of using Gauss' law to determine the electric field surrounding an infinitely large, thin plane of positive charges with uniform surface charge density. It explains the selection of an appropriate Gaussian surface—a cylinder with a horizontal axis—and the rationale behind it. The calculation leads to the conclusion that the electric field is uniform and independent of the distance from the plane, highlighting the principles of Gauss' law in symmetrical charge distributions.
🔄 Electric Field Between Two Parallel Charged Planes
Building on the previous example, this paragraph considers the scenario of adding an equal magnitude, parallel, infinite plane of negative charges to the positively charged plane. The script describes the resulting electric fields and their interactions, leading to the conclusion that the electric field outside the two parallel planes is zero, while the field between the planes doubles that of a single plane, reinforcing the concept of superposition in electric fields.
🏺 Electric Field of a Uniformly Charged Sphere
The final paragraph reviews the concept that outside a uniformly charged sphere, the electric field behaves as if the sphere were a point charge. The script uses Gauss' law to prove this by comparing the electric field at a distance from a charged sphere to that of a point charge, showing that the electric field diminishes with the square of the distance from the center, regardless of the sphere's size, thus concluding the review of electric flux and Gauss' law.
Mindmap
Keywords
💡Electric Flux
💡Gauss' Law
💡Uniform Electric Field
💡Right Triangular Box
💡Coulomb's Law
💡Permittivity of Free Space
💡Surface Charge Density
💡Infinitely Large Plane of Charges
💡Gaussian Surface
💡Maxwell's Equations
💡Symmetric Charge Distributions
Highlights
Electric flux is defined as the effect that appears to pass through a surface, which does not necessarily need to move.
The symbol for electric flux is uppercase Phi (Φ), and it measures the amount of electric field passing through a defined area.
The formula for electric flux through a two-dimensional area is Φ_E = E • A cos(θ), where E and A are vectors and θ is the angle between them.
Area vectors are always directed outward from a closed surface, similar to the direction of angular velocity in rotation.
Electric flux is a scalar and its units are newtons times square meters divided by coulombs (N⋅m²/C).
The net electric flux through a closed surface can be determined by summing the flux through each side of a geometric shape.
In the example of a right triangular box, the net electric flux is zero due to symmetry and the enclosed charge being zero.
Gauss' law is demonstrated through the electric flux passing through a sphere concentric to a point charge, which equals the charge divided by the permittivity of free space.
Gauss' law states that the electric flux through a closed surface equals the enclosed charge divided by the permittivity of free space.
The choice of Gaussian surface shape is crucial for simplifying calculations, as it should align with the electric field direction.
For an infinitely large, thin plane of positive charges, the electric field surrounding it is uniform and independent of distance from the plane.
Between two parallel planes of equal magnitude but opposite sign charges, the electric field is doubled compared to a single plane.
AP Physics C: Electricity and Magnetism guidelines specify that Gauss' law should be used for highly symmetrical charge distributions only.
Outside a uniformly charged sphere, the electric field is the same as if the sphere were a point charge, regardless of the sphere's size.
The electric field inside a conductor is zero, while inside an insulator it depends on the radius and charge distribution.
The motion of a charged particle in a constant electric field is analogous to projectile motion under gravity.
The review concludes with an emphasis on the importance of imagination and practice in visualizing and understanding electric flux and Gauss' law.
Transcripts
Browse More Related Video
Gauss law of electricity | Electrostatics | Physics | Khan Academy
Physics 37.1 Gauss's Law Understood (3 of 29) What is Gauss' Law?
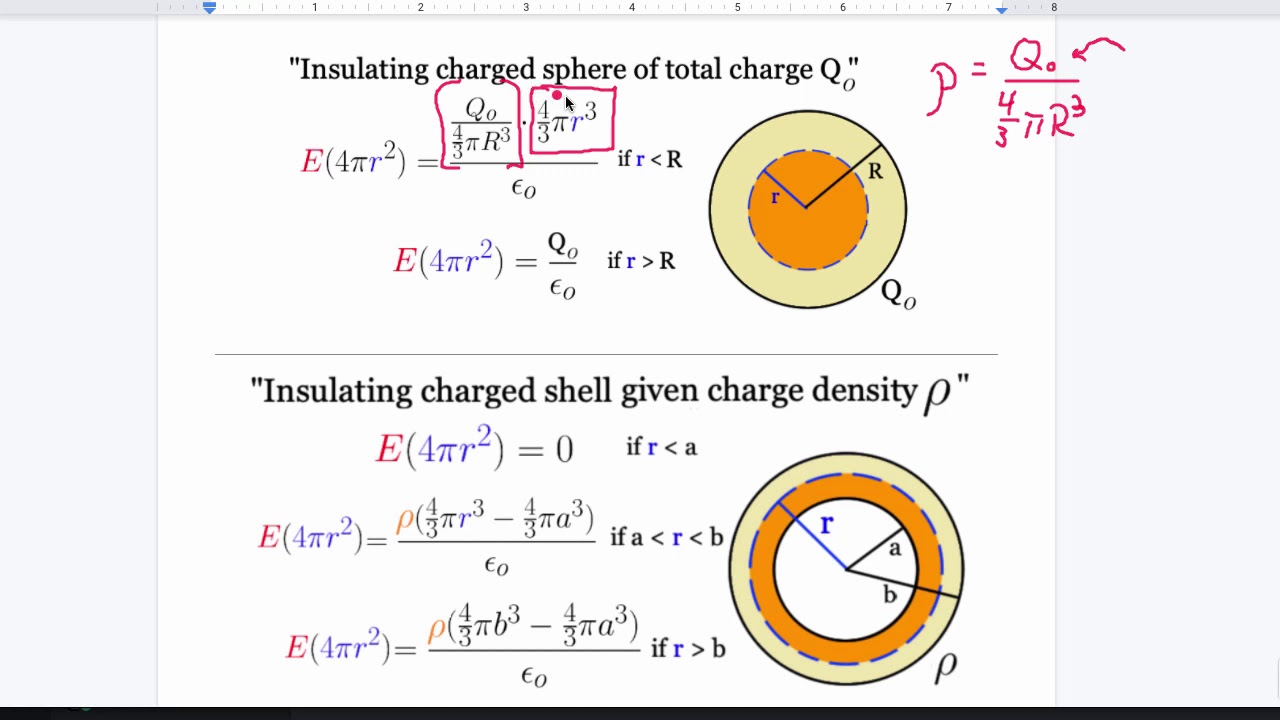
Ultimate Gauss' Law review
Physics 37.1 Gauss's Law Understood (4 of 29) Gauss' Law Clarified
Electric Flux and Gauss’s Law | Electronics Basics #6
Physics 37.1 Gauss's Law Understood (5 of 29) How to Use Gauss' Law
5.0 / 5 (0 votes)
Thanks for rating: