Graphing Conic Sections Part 1: Circles
TLDRThe script discusses conic sections, specifically circles, which are formed by slicing a cone with a plane. Circles have an eccentricity of 0 and are defined as the set of points equidistant from a center point. The equation of a circle can be derived using the distance formula and involves terms containing X, Y, the center point H and K, and the radius R, all squared. The script shows how to graph circles given their equations, determine equations from their graphs, and manipulate equations into standard form to enable graphing. It contrasts the simplicity of circles versus other conic sections like ellipses, and concludes by transitioning into a discussion of ellipses next.
Takeaways
- 👨🏫 Conic sections are important two-dimensional figures derived from slicing a three-dimensional right circular double-napped cone in different ways.
- 📈 A circle is formed when a plane cuts through a cone parallel to the base, while ellipses, parabolas, and hyperbolas are formed under different cutting conditions.
- 🔬 Eccentricity is a parameter that measures the deviation of a conic section from being perfectly circular, with circles having an eccentricity of zero.
- 📍 Circles are defined as the set of all points equidistant from a fixed point (the center), with this distance being the radius.
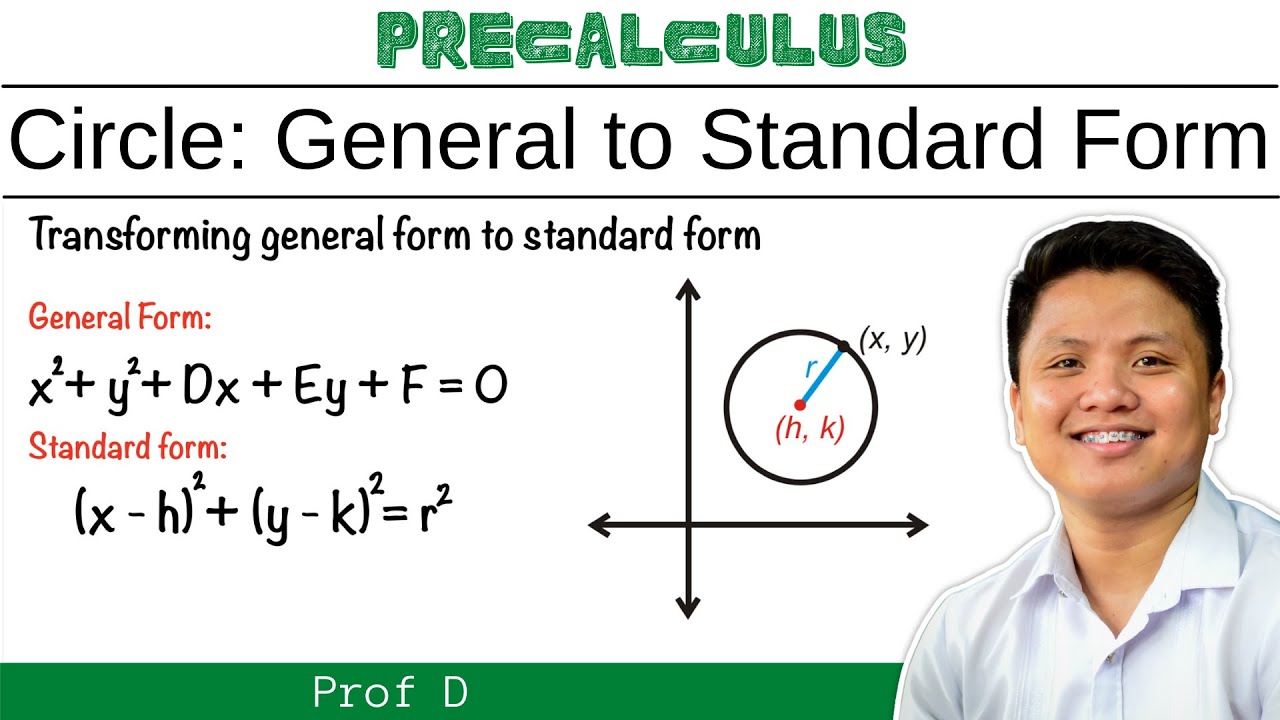
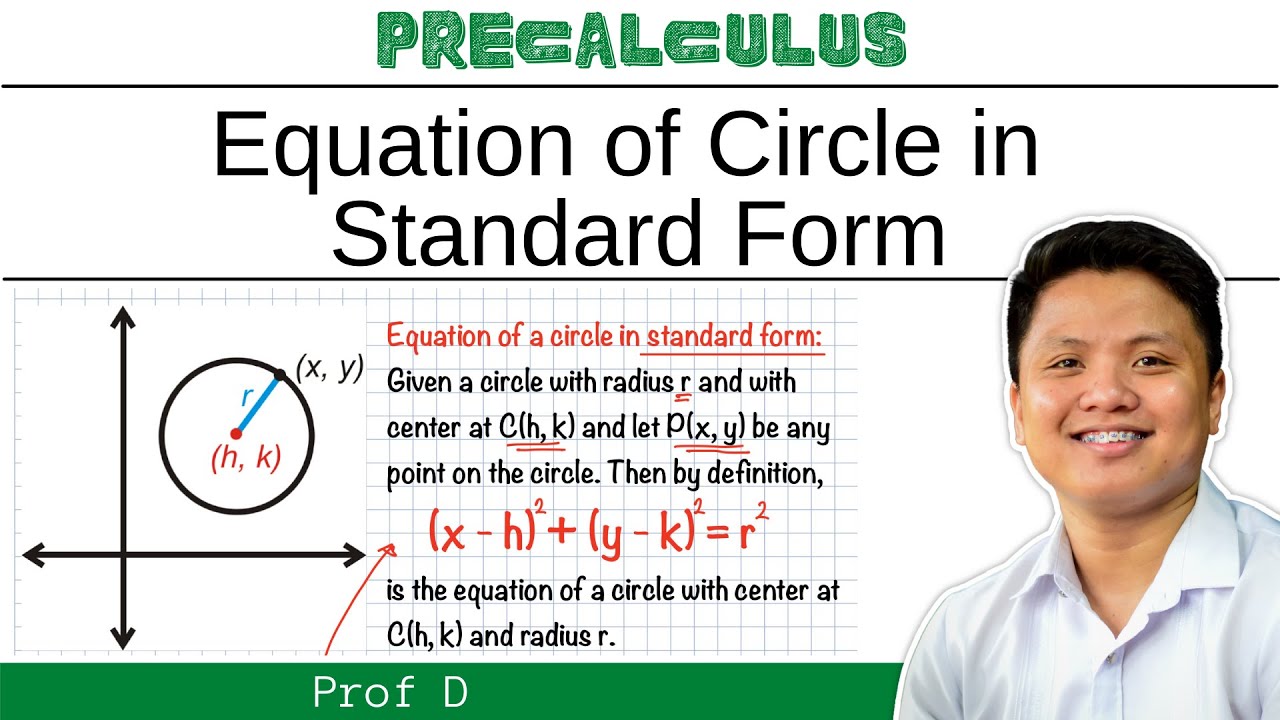
- 📊 The standard form equation of a circle in a coordinate plane involves squaring the distances from any point on the circle to the center (HK).
- 📚 The distance formula is applied to derive the circle's equation by squaring both sides of the equation involving the radius (R) and center (HK).
- 🛠 Graphing a circle can start from simple equations like X squared plus Y squared equals nine, indicating a circle centered at the origin with radius 3.
- 💾 For circles with centers not at the origin, the equation includes terms (X minus H) and (Y minus K) to represent the center (HK).
- 📝 Deriving a circle's equation from its graph involves locating the center for HK values and measuring the radius directly.
- 📏 Completing the square is a technique used to rearrange and simplify circle equations into standard form for easier graphing and analysis.
Q & A
What are conic sections and where does the name come from?
-Conic sections are circles, ellipses, parabolas and hyperbolas. They are called 'conic sections' because they can be formed by slicing a cone at different angles.
How is eccentricity used to describe different conic sections?
-Eccentricity describes how much a conic section deviates from a perfect circle. A circle has eccentricity 0. An ellipse has eccentricity between 0 and 1. A parabola has eccentricity exactly 1. A hyperbola has eccentricity greater than 1.
What is the algebraic definition of a circle?
-A circle is defined algebraically using the distance formula. For any point (X,Y) on the circle, the distance from that point to the center (H,K) equals the radius R.
What is the standard form equation for a circle and what does each term represent?
-The standard form is (X - H)2 + (Y - K)2 = R2. H and K represent the coordinates of the center. R represents the radius.
If a circle equation has no H and K terms, what does that mean about the center?
-If there are no H and K terms, that means the center is at the origin (0, 0).
How can you find the center and radius of a circle from its equation?
-To find the center, look at the terms with X and Y to see the values of H and K. To find the radius, take the square root of the number on the right side of the equation.
How can you derive the equation of a circle if you are only given its graph?
-Locate the center point to get H and K. Then measure the distance from the center to any point on the circle to get the radius R. Plug these values into the standard form equation.
When might you need to use completing the square to put a circle equation into standard form?
-If the equation has extra terms with X and Y, you may need to complete the square for each variable to isolate the (X - H)2 and (Y - K)2 terms.
What are some differences between graphs of circles and other conic sections like ellipses?
-Circles are symmetric about their center point. Ellipses can be stretched into oval shapes. Parabolas and hyperbolas are open-ended and not enclosed like circles and ellipses.
What are some real-world applications of circles and other conic sections?
-Conic sections model many natural phenomena and technological designs - for example, planetary orbits (ellipses), bridges (parabolas), flashlights or satellite dishes (parabolas), nuclear explosions (hyperbolas).
Outlines
📐 Introduction to Conic Sections and Circles
This paragraph introduces conic sections, a class of figures derived from a cone, including circles, ellipses, parabolas, and hyperbolas. It explains that these shapes are formed by slicing a cone with a plane at various angles and positions. The concept of eccentricity is introduced to measure the deviation of these sections from a perfect circle, with different values indicating different conic sections. The paragraph then focuses on circles, defining them as points equidistant from a center, and introduces the algebraic representation of a circle using the distance formula. It elaborates on the standard form equation of a circle and explains how to graph circles from equations, with examples to illustrate the process. The paragraph ends with an insight into deriving the equation of a circle from its graph.
🔍 Graphing Circles: Standard Form and Equation Manipulation
This paragraph delves into the process of graphing circles by manipulating their algebraic equations into a standard form. It begins by presenting a complex equation and explains how to rearrange and complete the square for both x and y terms to achieve the standard form. The paragraph emphasizes the importance of completing the square, a technique previously covered, and provides a step-by-step guide to apply this technique to both x and y terms. It demonstrates this through an example, resulting in a circle equation in standard form with identifiable center and radius. The paragraph concludes by summarizing the skills learned: representing circles graphically, writing their equations in standard form, and manipulating equations for graphing. It ends with a teaser about moving on to a more complex conic section, the ellipse, and includes a check for comprehension.
Mindmap
Keywords
💡conic sections
💡eccentricity
💡radius
💡center
💡distance formula
💡standard form
💡complete the square
💡ellipse
💡graph
💡manipulate
Highlights
The transcript discusses using neural networks for natural language processing tasks.
Word embeddings are introduced as a way to capture semantic meaning and represent words numerically.
Different embedding techniques like Word2Vec and GloVe are explained.
The transformer architecture is presented as a novel way to handle sequence modeling.
Attention mechanisms are described as allowing models to focus on relevant parts of the input.
Pre-trained language models like BERT and GPT are discussed as a breakthrough in NLP.
Fine-tuning techniques are introduced to adapt these models to specific tasks.
The application to question answering is outlined as a use case.
Challenges like bias in data and models are raised as important issues.
Ethical considerations in NLP systems design are emphasized.
Future directions like multimodal learning and causal modeling are suggested.
The potential of NLP to advance medicine, education and accessibility is highlighted.
Limitations of current methods and need for interpretability are noted.
The talk concludes by recapitulating key innovations covered.
Q&A session focuses on practical implementation challenges and advice.
Transcripts
Browse More Related Video
5.0 / 5 (0 votes)
Thanks for rating: