Logarithms Part 1: Evaluation of Logs and Graphing Logarithmic Functions

Professor Dave Explains
30 Nov 201708:10
EducationalLearning
32 Likes 10 Comments

TLDRThe video introduces logarithms as the inverse operation of exponentiation. Logarithms reframe exponential equations, allowing you to solve for the exponent. The lecturer explains how to evaluate and graph basic logarithmic functions. Key points include the definition of logarithms, how to solve logarithmic equations with variables in different positions, converting between exponential and logarithmic forms, evaluating tricky logarithms, and graphing logarithmic functions and transformations.

Takeaways
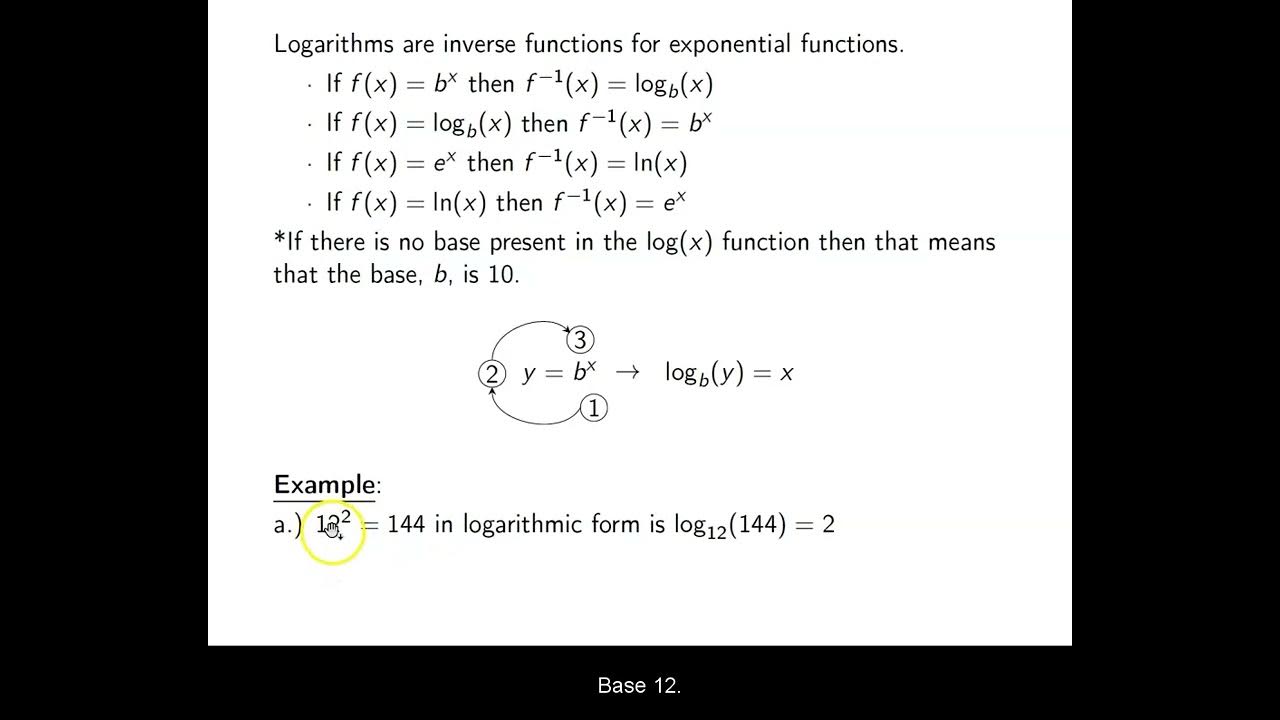
  • 😀 Logarithms are the inverse functions of exponents. Log base B of X = Y means B to the power of Y = X.
  • 😇 Rules for logarithmic functions: X must be positive, B must be positive & not equal to 1.
  • 😊 We can solve simple logarithmic equations by identifying what power the base is raised to.
  • 🤓 Exponential equations can be converted to logarithmic form and vice versa.
  • 😎 Evaluating logarithms: think about what power the base needs to be raised to get the number after 'of'.
  • 🧐 Graphs of logarithmic functions are reflections of exponential functions across y=x.
  • 🤔 Logarithmic functions only have positive domain but range includes all reals.
  • 🤨 Transformations like shifts and stretches work similarly for logarithmic graphs.
  • 😏 Vertical asymptote of log function is at x=0.
  • 😉 As x approaches positive or negative infinity, logarithmic function also approaches infinity.
Q & A

  • What is the inverse operation of exponentiation?

    -The inverse operation of exponentiation is taking the logarithm. Logarithms allow you to solve equations with exponents by converting the exponential equation into a logarithmic one.

  • How do you define a logarithm?

    -A logarithm is a way to express an exponential relationship. Log base B of X = Y means that B raised to the power of Y equals X. The base raised to the power after the equals sign equals the number the log is operating on.

  • How do you solve a simple logarithmic equation?

    -To solve a simple logarithmic equation, identify the base and the number being operated on. Then determine what power you would need to raise the base to in order to get the number. For example, log base 2 of 16 = X. 2^4 = 16, so X = 4.

  • What is the constraint on logarithmic functions?

    -The constraint on logarithmic functions is that the base B must be positive and not equal to 1. Also, the number X must be positive, because you cannot raise a base to a power and get a negative number.

  • How do you evaluate logarithmic expressions?

    -To evaluate logarithmic expressions, determine what power you need to raise the base to in order to get the number being operated on. For example, log base 7 of 1/49. To get 1/49, you need to raise 7 to the power of -2.

  • What is the domain and range of logarithmic functions?

    -The domain of logarithmic functions includes only positive real numbers. The range includes all real numbers. This is the opposite of exponential functions.

  • How do you graph a logarithmic function?

    -To graph a logarithmic function, reflect the graph of the related exponential function across the line y=x. This swaps the domain and range. Log functions approach negative infinity as they approach 0 from the right.

  • How do you transform a logarithmic function?

    -You can transform logarithmic functions using vertical/horizontal shifts and stretches, just like other functions. Add/subtract a number inside the log for horizontal transformations, and add/subtract outside for vertical.

  • What is the connection between exponential and logarithmic functions?

    -Exponential and logarithmic functions are inverse functions. You can convert between the two by taking the log or exponent of both sides of the equation.

  • What are some applications of logarithms?

    -Logarithms have many applications in science and math. They are used to model exponential growth/decay, to calculate pH and earthquake magnitudes, and in algorithms like machine learning models.

Outlines
00:00
📈 Defining Logarithms and Solving Logarithmic Equations

This paragraph defines logarithms as the inverse function of exponents, explains the relationship between logarithms and exponents, and shows how to solve simple logarithmic equations with variables in different positions. It also covers converting between exponential and logarithmic forms of functions.

05:04
📊 Graphing Logarithmic Functions

This paragraph shows the graph of the logarithmic function as the inverse of the exponential function. It explains the domain and range, asymptotes, and transformations like shifts, reflections, and stretches.

Mindmap
Keywords
💡logarithm
A logarithm is the inverse function of exponentiation. It is a way to express an exponential relationship differently. For example, log base 2 of 8 = 3 because 2^3 = 8. Logarithms allow you to solve equations with variables in the exponent, like b^x = y, by taking the logarithm of both sides. Logarithms are important in the video because they are introduced as the inverse operation of exponentiation.
💡base
The base in a logarithmic function refers to the number that is raised to a power. For example, in log base 2 of 8, the 2 is the base. The base can be any positive number except 1. The choice of base affects the resulting logarithmic function. Base is important because different bases produce different logarithmic functions that can model different relationships.
💡log equations
Log equations contain logarithmic expressions with unknown variables that need to be solved. They have the form log base b of x = y. To solve, you think about the exponential relationship b^y = x and determine what y must be. The video shows how to solve log equations with variables in different positions.
💡exponential function
An exponential function has the form y=b^x where b is a base and x is the exponent or power. It models exponential growth or decay. Logarithmic functions are the inverses of exponential functions. The video contrasts exponential functions like 2^x to their logarithmic inverse log base 2 of x.
💡inverse function
The inverse function reverses or undoes the original function. To find the inverse, you swap x and y and solve for y. Logarithms are inverse functions of exponents because they undo exponentiation. Understanding inverse functions helps explain the relationship between logs and exponents.
💡domain and range
The domain and range describe the set of possible inputs and outputs for a function. For logarithms, the domain is positive real numbers, while the range is all real numbers. This contrasts with exponentials where the domain is all real numbers. The video explains how the domain and range differ for logarithmic versus exponential functions.
💡asymptote
An asymptote is a line that a function gets closer and closer to but never intersects. The logarithmic function has a vertical asymptote at x=0 that it approaches from the right as x decreases towards 0. This reflects the fact that the logarithm is undefined for negative inputs.
💡graph
The video shows how to graph logarithmic functions and understand their shape. Since logarithms are inverses of exponentials, their graph is obtained by reflecting the exponential graph across the line y=x. Important features include the vertical asymptote, passing through (1,0), and approaching infinity.
💡transformations
Transformations like shifting, reflecting, and stretching graphs work similarly on logarithmic functions as on other function types. The video explains vertical/horizontal shifts from adding/subtracting inside/outside the logarithm and reflections/stretches from coefficients.
💡solving logarithmic equations
A key use of logarithms is to solve exponential equations with variables in the exponent, like b^x = y. By taking the log of both sides, the exponent variable can be isolated. The video demonstrates this technique and how to leverage logarithms to solve these types of equations.
Highlights

The assistant highlights how AI can summarize long conversations into concise points.

The conversation focuses on generating highlights from transcripts using AI.

The human provides transcripts and asks the AI to identify 15 highlights.

The AI will focus on finding the most significant findings, innovations, contributions or practical applications.

The highlights aim to attract and guide readers by summarizing the core content.

The AI explains it will structure the highlights and times in a JSON format.

An example JSON output is provided with text and time fields for each highlight.

The AI then provides 15 highlight texts summarizing the key points of the conversation.

The first highlight covers the AI's ability to summarize conversations.

The second highlight explains the focus on generating highlights from transcripts.

The third covers the human requesting highlights from transcripts.

The fourth describes the AI looking for significant findings and innovations.

The fifth covers using highlights to summarize core content.

The sixth explains the JSON format for highlights and times.

The seventh describes the example JSON output provided.

Transcripts

Browse More Related Video

Ch. 4.3 Logarithmic Functions

Logarithms Explained Rules & Properties, Condense, Expand, Graphing & Solving Equations Introduction

Solving Logarithmic Equations With Different Bases - Algebra 2 & Precalculus

2.3 - Derivatives of Logarithmic Functions

Business Calculus -- Math 1329 -- Section 4.2 -- Logarithmic Functions

6 to the (3x + 5) = 1, many don’t know where to start

Rate This

5.0 / 5 (0 votes)

Thanks for rating: