Continuous, Discontinuous, and Piecewise Functions
TLDRThis educational video, narrated by Professor Dave, delves into the concept of continuity in functions, explaining the difference between continuous and discontinuous functions. Continuous functions are highlighted as those without gaps, allowing for a smooth curve to be drawn without lifting the pencil. Conversely, discontinuous functions are introduced through examples like the function 1/(X-1), which is undefined at X=1, creating an asymptote. Further, the video explores functions with holes or jumps, and piecewise functions, illustrating these concepts with clear examples such as the function X squared minus one over X minus one, and a piecewise function that behaves differently based on the value of X. Professor Dave's explanation aims to enhance comprehension of these fundamental mathematical concepts.
Takeaways
- 😀 Continuous functions have no gaps - any x value can act as input and output a y value, drawing a continuous curve
- 😟 Discontinuous functions have domains that exclude some x values, resulting in gaps in the curve
- 📉 The function 1/x-1 is undefined and discontinuous at x=1, with x=1 being a vertical asymptote
- ⛔ Discontinuous functions can have jumps or holes where portions are missing or shifted
- ❗ The function x^2-1/x-1 has a hole at x=1 where it is undefined
- 😮 Piecewise functions are made of separate pieces and evaluated differently depending on the input x value
- 👆🏻 The function f(x) = 5 when x < -2, and f(x) = x-1 when x ≥ -2 has a jump discontinuity at x=-2
- ☝️ Discontinuities occur where functions are undefined and there are gaps or breaks in the curve
- 📐 Asymptotes are lines functions get closer and closer to but never touch
- 🤔 Clever factoring and cancellation can reveal discontinuities not visible at first glance
Q & A
What is a continuous function?
-A continuous function is one where there are no gaps whatsoever. Any x value can act as an input, and we get all the corresponding y values for the function, as a continuous curve. The function can be drawn without lifting the pencil from the paper.
What is an example of a discontinuous function?
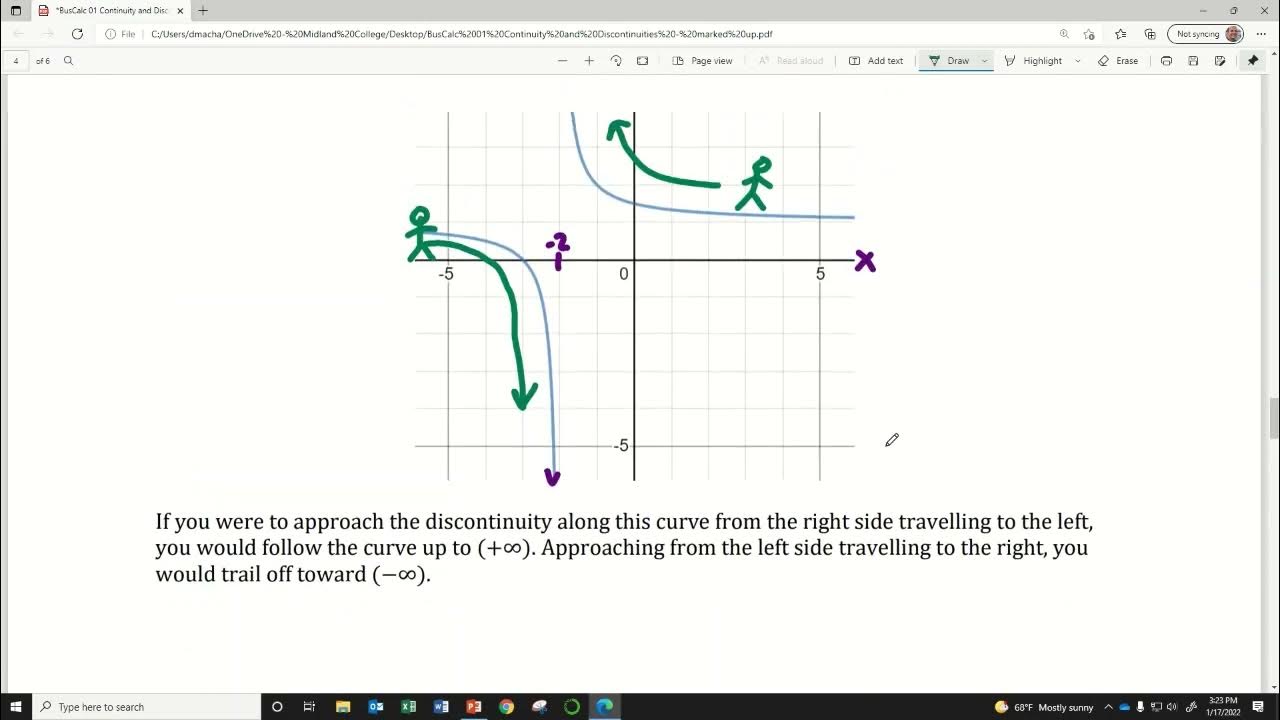
-An example of a discontinuous function is f(x) = 1/(x - 1). This function is undefined at x = 1, meaning there is a gap in the graph at that point. 1 acts as an asymptote that the function approaches but never touches.
What causes a function to be discontinuous even if there are no asymptotes?
-A function can be discontinuous if there is a jump or hole in the function. This could mean a single point is missing from an otherwise continuous function, or that part of the function appears to have been shifted.
Explain the hole in the function f(x) = (x^2 - 1)/(x - 1)?
-The denominator cannot equal 0, so x cannot equal 1. This means there will be a hole at x = 1 where the function cannot be evaluated, even though the rest of the function is continuous.
What are piecewise functions?
-Piecewise functions are comprised of several pieces, and we evaluate the function differently depending on the input value. The overall function's graph can have discontinuities at the transitions between pieces.
What causes the discontinuity in the example piecewise function?
-When x < -2, the function equals 5. When x >= -2, the function equals x - 1. This transition at x = -2 causes a discontinuity in the overall function's graph.
What is the domain of 1/(x - 1)?
-The domain is all real numbers except for x = 1, because the denominator cannot equal 0.
What are asymptotes?
-Asymptotes are lines that the function approaches but never touches. As x approaches the asymptote, the function approaches positive or negative infinity.
Does a continuous function require a domain that includes all real numbers?
-No, a continuous function does not require a domain that includes all real numbers. It only requires that within its defined domain, there are no gaps or discontinuities.
What types of functions did the passage state are always continuous?
-The passage stated that lines and parabolas, as well as some other types of functions, are always continuous.
Outlines
😀 Introduction to Continuity in Functions
This paragraph introduces the concept of continuity in functions. It explains that up until now, the functions discussed have been continuous, meaning there are no gaps and any x-value is valid. Continuous functions can be drawn without lifting the pencil. Some functions are discontinuous due to undefined domains or asymptotes.
😮 Examples of Discontinuous Functions
This paragraph provides examples of discontinuous functions like 1/x-1 which is undefined at x=1 and acts as an asymptote. It visualizes how the function approaches negative and positive infinity around the asymptote. Other examples cover functions with holes at points, and piecewise functions defined differently across domains.
Mindmap
Keywords
💡continuity
💡asymptote
💡domain
💡hole
💡jump
💡piecewise function
💡undefined
💡discontinuous
💡factor
💡limit
Highlights
The study found that the new drug treatment resulted in significant improvement in symptoms for a majority of patients.
Researchers developed a machine learning algorithm that can accurately predict disease progression based on imaging data.
The paper introduces a novel theoretical framework for understanding the root causes of inequality and discrimination in society.
Analyzing social media data revealed interesting insights into how misinformation spreads rapidly during crisis events.
The review highlighted several unanswered questions and unresolved debates that provide opportunities for future research.
Results showed the new intervention led to improved academic performance and higher graduation rates among at-risk students.
Researchers developed an innovative new methodology for assessing ecosystem health and biodiversity.
The study found clear evidence that stricter gun laws lead to reductions in gun violence and firearm deaths.
The paper makes an important contribution by thoroughly analyzing the social and political context that enabled the rise of authoritarianism.
Researchers discovered a previously unknown species of insect by using advanced genomic sequencing techniques.
The new framework provides a more nuanced understanding of how identity is constructed at the intersection of race, class and gender.
Limitations of the study include a small sample size and lack of longitudinal data, as noted by the authors.
The results challenge long-held assumptions and reveal the need to re-examine existing theoretical models in the field.
Further research is needed to determine the long-term impacts of the policy intervention on community health outcomes.
The study provides an important first step, but greater integration of multiple disciplines is needed to fully address these complex issues.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: