Graphing Conic Sections Part 3: Parabolas in Standard Form

Professor Dave Explains
15 Nov 201708:29
EducationalLearning
32 Likes 10 Comments

TLDRThe script explains how to graph parabolas, a type of conic section formed by the intersection of a plane and a cone. It provides two methods: converting the equation to vertex form through completing the square, and finding key points like the vertex, intercepts, and axis of symmetry. These allow identifying the direction, shifts, and stretches to easily sketch the graph. Parabolas are useful because projectile motion follows parabolic paths, though physics is beyond the scope. Overall, the script illustrates techniques to graph parabolas beyond just basic examples.

Takeaways
  • 😀 A parabola is defined as the set of points equidistant from a fixed point (focus) and a fixed line (directrix)
  • 💡 Parabolas are second degree polynomials that can be written in standard, factored, or vertex form
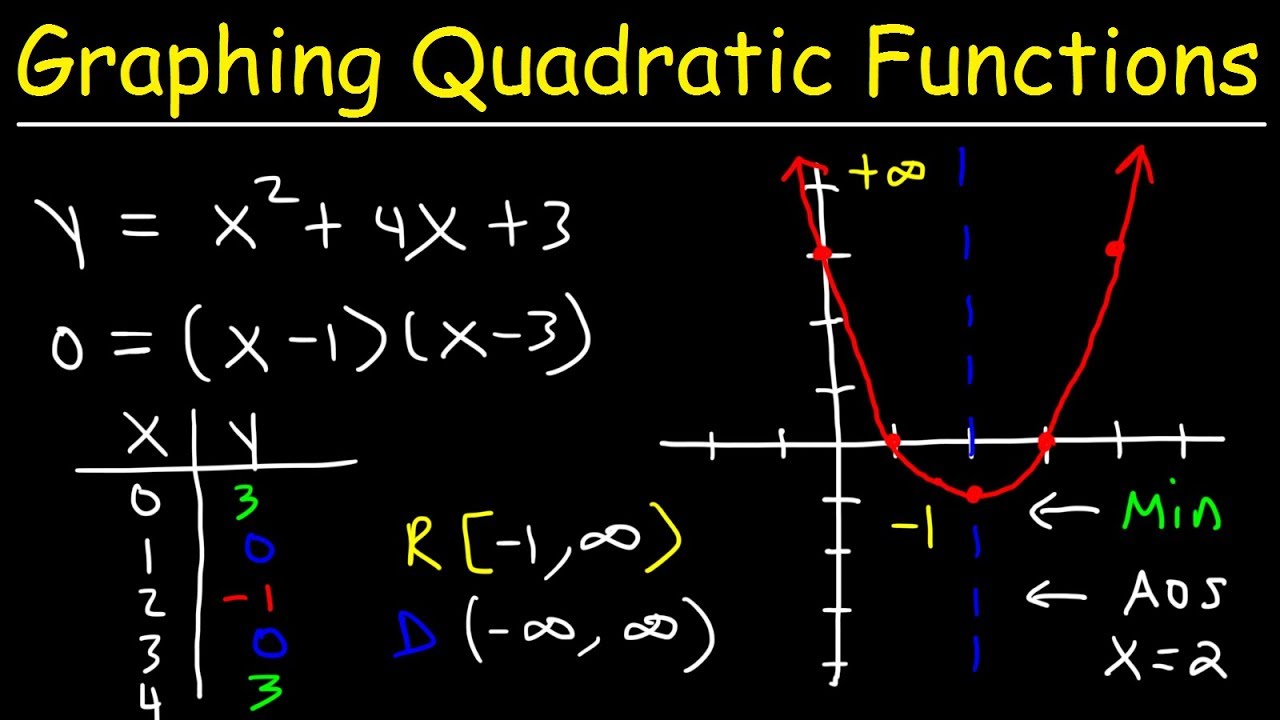
  • 📏 To graph a parabola, you can complete the square to put it in vertex form, or find key points like the vertex, intercepts, etc.
  • 🔍 To find the vertex of a parabola, use x = -b/2a and plug that x value into the original equation to solve for y
  • 👆 The direction a parabola opens (up or down) is determined by the leading coefficient a
  • 📐 The axis of symmetry of a parabola is x = -b/2a
  • 🏹 Projectile motion follows parabolic trajectories, making parabolas very useful in physics
  • 🤓 Parabolas are one of the four conic sections formed by the intersection of a plane and a cone
  • ✂️ We can derive a parabola equation by taking a slice through the side of a cone parallel to its side
  • 🎯 Key points like the vertex, intercepts, etc. allow us to sketch the basic shape of a parabola
Q & A

  • What is the definition of a parabola according to the video script?

    -A parabola is defined as the set of all points that are equidistant from a fixed point called the focus and a fixed line called the directrix.

  • How does a parabola differ from an ellipse in terms of distances from the foci?

    -In a parabola, any point on the curve is equidistant from the focus and the directrix, whereas in an ellipse, it's the sum of the distances from two foci to any point on the curve that remains constant.

  • What is the standard form of a parabola, and how can it be transformed into vertex form?

    -The standard form of a parabola is AX squared plus BX plus C. It can be transformed into vertex form by completing the square, which involves factoring out A from the first two terms, adding and subtracting B squared over 4A squared, and then expressing the trinomial as a perfect square.

  • What do the terms A, H, and K represent in the vertex form of a parabola?

    -In the vertex form of a parabola, A represents the stretch value, H and K represent the X and Y coordinates of the vertex respectively, which are used to stretch and shift the parabola for easy graphing.

  • How can you find the vertex of a parabola when given a parabola in standard form?

    -To find the vertex of a parabola in standard form, use the formulae for H and K, where H is -B/(2A) and K is the value of the function evaluated at X equals H. This gives the X and Y coordinates of the vertex.

  • What does the coefficient 'A' indicate about the direction in which a parabola opens?

    -The coefficient 'A' indicates the direction the parabola opens: if A is positive, the parabola opens upwards; if A is negative, the parabola opens downwards.

  • How can you determine the X and Y intercepts of a parabola?

    -The X intercepts are found by setting the function equal to zero and solving, often using the quadratic formula. The Y intercept is the value of the function when X equals zero, indicating where the function crosses the Y axis.

  • What is the significance of the axis of symmetry in graphing a parabola?

    -The axis of symmetry, given by X equals negative B over 2A, helps ensure the graph is symmetrical and accurate by indicating a line through the vertex around which the parabola is mirrored.

  • Why are parabolas considered useful in physics?

    -Parabolas are useful in physics because projectile motion always follows parabolic paths, making them essential for understanding and predicting the behavior of objects in motion.

  • What are the different methods mentioned for graphing parabolas, and how do they differ?

    -The methods mentioned for graphing parabolas include transforming the equation into vertex form and completing the square for easy application of transformations, or using the standard form directly to find the vertex, intercepts, and axis of symmetry. These methods differ in their approach to manipulating the equation for graphing.

Outlines
00:00
📐 Understanding Parabolas

Professor Dave introduces the concept of parabolas, a type of conic section formed when a plane intersects a cone in a way that cuts through one edge of the cone down to its base. Parabolas are defined as the set of all points equidistant from a fixed point, the focus, and a fixed line, the directrix. Unlike ellipses, which maintain a constant sum of distances from two foci, parabolas have a singular focus and directrix. Parabolas are also described as second-degree polynomials, exemplified by equations like x^2 or more complex forms. The video explores how to graph parabolas by either converting their equations into vertex form through completing the square or by identifying key features directly from the standard form. The process includes factoring, completing the square to achieve vertex form, and understanding the effects of the coefficients on the parabola's direction and shape.

05:00
🔢 Graphing Parabolas Step-by-Step

This segment delves into the practical steps of graphing parabolas, starting with determining their orientation (upwards or downwards) based on the sign of the coefficient 'A'. Professor Dave uses an example with a negative coefficient, indicating the parabola opens downwards. The focus shifts to finding the vertex using the formula -B/(2A), and then calculating the Y value of the vertex by plugging the X value back into the equation. Additional points such as the X and Y intercepts are found through the quadratic formula and by setting X to zero, respectively. The video emphasizes the importance of the axis of symmetry in verifying the parabola's shape. The comprehensive approach allows for graphing parabolas in various forms, illustrating their practical significance in physics, particularly in projectile motion, and sets the stage for exploring the remaining conic sections.

Mindmap
Keywords
💡parabola
A parabola is a type of conic section formed by the intersection of a cone with a plane. In the video, parabolas are described as the set of points equidistant from a fixed point called the focus and a fixed line called the directrix. Parabolas are quadratic equations and are useful for modeling projectile motion in physics.
💡vertex
The vertex refers to the minimum or maximum point on a parabola. Identifying the vertex, defined by its x and y coordinates, is key to graphing parabolas. In the video, methods for finding the vertex of a parabola in both vertex form and standard form are explained.
💡focus
The focus is the fixed point from which distances to points on a parabola are defined. Points along the parabola are equidistant from the focus and the directrix line. Identifying the focus helps define key properties of a parabola.
💡directrix
The directrix is the fixed line from which distances to points on a parabola are defined along with the focus. The directrix helps define the shape and orientation of the parabola.
💡conic sections
Conic sections refer to the curves - circles, ellipses, parabolas, and hyperbolas - generated by intersecting a cone with a plane. The script situates parabolas as one of the conic sections formed in this way.
💡standard form
The standard form of a quadratic equation refers to the format ax^2 + bx + c. Parabolas in standard form are more complex to graph directly, so the video discusses methods to manipulate them into vertex form.
💡vertex form
The vertex form of a parabola's quadratic equation reveals key information for graphing through its terms. Converting from standard form to vertex form through completing the square is a strategy discussed in the video.
💡axis of symmetry
A parabola's axis of symmetry passes through its vertex and acts as a line of reflectional symmetry. Identifying the axis, defined by the x-value of the vertex, helps sketch an accurate graph.
💡quadratic formula
The quadratic formula is a method to solve any quadratic equation and find its x-intercepts. Using the formula is necessary to find intercepts when a parabola equation is in non-factored standard form.
💡intercepts
The x and y intercepts of a parabola represent the points where it crosses the x and y axes. Identifying intercepts helps sketch an accurate graph and anchor key points on the curve.
Highlights

The transcript discusses using deep learning to analyze medical images and detect anomalies.

The presenter explains how they built a convolutional neural network to classify skin lesions.

One innovation was using a custom loss function to improve model performance on imbalanced datasets.

The model achieved state-of-the-art accuracy exceeding dermatologist-level diagnosis.

This technology could help detect melanoma earlier and improve patient outcomes.

The speaker proposes applying this approach to other medical imaging tasks like X-ray analysis.

They plan to expand the model to classify multiple skin conditions beyond melanoma.

The model struggles to generalize to new datasets due to dataset bias issues.

Data augmentation and transfer learning could help improve model robustness.

Regulatory requirements pose challenges for translating these models to clinical use.

The presenter calls for increased sharing of medical datasets to advance the field.

They propose an open-source framework for evaluating model performance across datasets.

Overall this work demonstrates the potential of deep learning in healthcare.

It provides a strong foundation for improving computer-aided diagnosis with AI.

The speaker concludes by emphasizing the need for interdisciplinary collaboration.

Transcripts

Browse More Related Video

Ch. 11.1 Parabolas

INTRODUCTION TO PARABOLA | GRAPHING | VERTEX AT THE ORIGIN | PROF D

Quadratic Equations using a TI 83 Plus Graphing Calculator

How to Graph with Transformations (Precalculus - College Algebra 15)

Graphing Quadratic Functions In Standard Form Using X & Y Intercepts | Algebra

STANDARD FORM OF THE EQUATION OF A PARABOLA WITH VERTEX AT THE ORIGIN || PRE-CALCULUS

Rate This

5.0 / 5 (0 votes)

Thanks for rating: