Gauss law logical proof (any closed surface) | Electric charges & fields | Physics | Khan Academy
TLDRThis video script delves into the mathematical proof of Gauss's Law for electric fields, demonstrating that the total electric flux through any closed surface equals the total charge enclosed divided by the permittivity of free space (ε₀). The script starts with the case of a spherical surface and extends the proof to any arbitrary shape, using a method inspired by Richard Feynman. It also addresses how to prove the law for multiple charges and the principle that external charges do not contribute to the flux, showcasing the elegance and universality of Gauss's Law in physics.
Takeaways
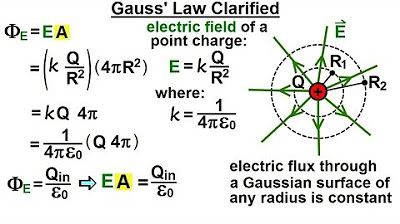
- 🌐 Gauss's Law states that the total electric flux through any closed surface is equal to the total charge enclosed divided by the permittivity of free space (ε₀).
- 📊 The electric flux through a sphere due to a charge at its center is given by the formula: Φ = Q / ε₀, where Q is the charge and ε₀ is the permittivity of free space.
- 🔍 Regardless of the shape of a closed surface, if it encloses a charge, the electric flux through the surface remains Q / ε₀.
- 🤔 The goal of the video is to mathematically prove Gauss's Law for any random shape, not just for spheres.
- 📘 The proof starts by considering a tiny sphere around a point charge and then comparing the flux through this sphere to the flux through a small piece of a larger, arbitrary-shaped surface.
- 📐 The electric field due to a point charge is radial and decreases as 1/r², where r is the distance from the charge.
- 📈 The perpendicular area of a surface element increases with the square of the distance from the charge, compensating for the decrease in the electric field strength.
- 🔗 By dividing both the sphere and the arbitrary surface into tiny pieces, the proof shows that the flux through each corresponding piece is the same due to the cancellation of the 1/r² factor.
- 🌀 Superposition principle is used to extend the proof to multiple charges, where the total flux is the sum of the individual flux contributions from each charge.
- 🚫 Charges outside the closed surface do not contribute to the flux through the surface, which is also proven by considering the flux through tiny pieces of the surface.
- 🎓 The video concludes by emphasizing the elegance of Gauss's Law and its proof, which is one of the presenter's favorite proofs in physics.
Q & A
What is the electric flux through a closed surface with a charge at its center?
-The electric flux through a closed surface with a charge at its center is given by the formula \( \Phi = \frac{q}{\epsilon_0} \), where \( q \) is the charge and \( \epsilon_0 \) is the permittivity of free space.
Why is the electric flux through any closed surface the same regardless of the shape?
-The electric flux through any closed surface remains the same because it is determined by the total charge enclosed by the surface and the permittivity of free space, not the shape of the surface.
What is the significance of Gauss's Law in electromagnetism?
-Gauss's Law is significant because it relates the electric flux through a closed surface to the total charge enclosed by that surface, providing a fundamental principle for calculating electric fields.
How is the electric field due to a point charge described in the script?
-The electric field due to a point charge is described as \( E = \frac{kq}{r^2} \), where \( k \) is Coulomb's constant, \( q \) is the charge, and \( r \) is the distance from the charge.
What is the method used in the script to prove Gauss's Law for any random shape?
-The method used in the script involves dividing the surface into tiny pieces and proving that the flux through each piece of the random shape is equal to the flux through a corresponding piece of a sphere of the same radius.
How does the script suggest comparing the electric field at different points on a surface?
-The script suggests comparing the electric field at different points by considering the distances from the charge and using the principle that the electric field decreases as one over the square of the distance.
What is the role of the perpendicular area in calculating the electric flux through a surface?
-The perpendicular area is crucial in calculating the electric flux because the flux is the product of the electric field and the perpendicular area at that point, which must be integrated over the entire surface.
How does the script explain the concept of superposition in the context of multiple charges?
-The script explains the concept of superposition by stating that the total flux due to multiple charges is the sum of the fluxes due to each individual charge, which can be proven by considering the flux due to each charge separately and then adding them together.
What does the script say about the contribution of charges outside the closed surface to the electric flux?
-The script states that charges outside the closed surface do not contribute to the electric flux through the surface, as the flux entering and exiting the surface due to these external charges cancels out.
How does the script use geometry to simplify the calculation of the perpendicular area in a non-perpendicular scenario?
-The script uses geometry by recognizing that the area and the charge form a cone, allowing the calculation of the perpendicular area by considering the ratio of the areas of similar triangles formed by the cone.
What is the final conclusion of the script regarding Gauss's Law?
-The final conclusion of the script is that Gauss's Law is proven to be true for any shape, stating that the flux through a closed surface equals the total charge inside divided by the permittivity of free space, regardless of the charges' positions or the shape of the surface.
Outlines
🔋 Gauss's Law and Electric Flux
The script introduces Gauss's Law, which quantifies the electric flux through a closed surface as the total charge enclosed divided by the permittivity of free space (epsilon naught). It explains that this relationship holds true regardless of the shape of the surface or the position of the charge within it. The video aims to mathematically prove this law not just for a sphere but for any random shape, using a method inspired by Richard Feynman. The explanation begins with a recap of the proof for a spherical surface and then discusses the challenge of extending this proof to irregular shapes due to varying electric fields and non-perpendicular areas.
📐 Proving Gauss's Law for Irregular Shapes
This paragraph delves into the method of proving Gauss's Law for irregular shapes by comparing the electric flux through a tiny sphere centered at the charge to that through a piece of the irregular surface. The script suggests dividing both the sphere and the irregular surface into infinitesimal pieces and demonstrating that the flux through corresponding pieces on the sphere and the irregular surface are equal. It then explains how to calculate the electric field and the perpendicular area for these tiny pieces, emphasizing the importance of the geometry of the situation and the application of the inverse square law for electric fields.
🔗 Flux Cancellation and Superposition Principle
The script concludes by addressing the application of Gauss's Law to multiple charges and the superposition principle, which states that the total electric flux through a closed surface is the sum of the fluxes due to individual charges. It also discusses how to prove that charges outside the closed surface do not contribute to the flux, using the same technique of dividing the surface into tiny pieces and showing that the flux entering equals the flux exiting, thus proving the contribution from external charges to be zero. The paragraph wraps up by reiterating the proof of Gauss's Law and expressing admiration for the elegance of the proof in physics.
Mindmap
Keywords
💡Electric Flux
💡Gauss's Law
💡Permittivity of Free Space (ε₀)
💡Electric Field
💡Charge (q)
💡Superposition Principle
💡Integration
💡Cone of Flux
💡Similar Triangles
💡Infinitesimals
💡Radial Electric Field
Highlights
Electric flux through any closed surface is constant and equals the total charge enclosed divided by epsilon naught, regardless of the shape of the surface.
Gauss's Law can be mathematically proven for any random shape, not just for spheres.
The electric field due to a point charge is uniform over the surface of a sphere, simplifying the flux calculation.
For a sphere, the flux is calculated by multiplying the electric field by the perpendicular area and integrating over the surface.
The electric field from a point charge is inversely proportional to the square of the distance from the charge.
The area of a sphere is perpendicular to the electric field lines, simplifying the calculation of flux through the sphere.
The total flux through a sphere is the charge divided by epsilon naught, a result of the area and electric field cancellation.
Richard Feynman's method involves imagining a tiny sphere within the larger, irregularly shaped surface to prove Gauss's Law.
Dividing the sphere and the large surface into tiny pieces allows for comparison of flux through each piece.
Flux through a tiny piece of the large surface is equal to the flux through the corresponding piece of the tiny sphere.
The electric field decreases with the square of the distance from the charge, as per the inverse square law.
The area perpendicular to the electric field can be calculated using geometric principles and the concept of similar triangles.
The product of the decrease in electric field and the increase in perpendicular area results in a constant flux through corresponding pieces.
Gauss's Law can be extended to multiple charges by summing the individual flux contributions due to each charge.
The superposition principle allows for the calculation of total flux due to multiple charges within the closed surface.
Charges outside the closed surface do not contribute to the flux, which can be proven by dividing the surface into tiny pieces and comparing entering and exiting flux.
The proof of Gauss's Law demonstrates that the flux through a closed surface equals the total charge inside divided by epsilon naught, a fundamental principle in physics.
Transcripts
Browse More Related Video
Electric Flux and Gauss’s Law | Electronics Basics #6
Physics 37.1 Gauss's Law Understood (3 of 29) What is Gauss' Law?
Ultimate Gauss' Law review
Gauss law of electricity | Electrostatics | Physics | Khan Academy

What is Flux? + an Introduction to Gauss Law (Electromagnetism – Physics)
Physics 37.1 Gauss's Law Understood (4 of 29) Gauss' Law Clarified
5.0 / 5 (0 votes)
Thanks for rating: