Proving the Pythagorean Theorem
TLDRIn this geometry lesson, Professor Dave provides an elegant proof of the Pythagorean theorem using basic facts about areas of shapes. By arranging four identical right triangles to form two squares, calculating and equating their areas, and applying algebraic techniques, the theorem A^2 + B^2 = C^2 emerges. Dave hopes this visually and symbolically satisfying derivation gives students warm, positive feelings about math as a discipline built on logical proofs regarding geometric relationships.
Takeaways
- 😀 The Pythagorean theorem states that in a right triangle, the square of the hypotenuse is equal to the sum of the squares of the other two sides.
- 👍 This video provides an elegant geometric proof of the Pythagorean theorem using area calculations.
- 🔼 It starts by constructing one large square using the sum of the legs of a right triangle.
- 🔽 It then constructs a smaller square using the hypotenuse.
- 💡 The area of the large square equals the sum of the areas of the four identical triangles and the small square.
- ✏️ By calculating and equating areas, the theorem follows algebraically after cancellations.
- 📐 There are other visual proofs using shapes and rearrangements to demonstrate the theorem.
- 🌟 The elegant nature of geometric proofs gives math an aesthetic appeal.
- 🧮 The FOIL method for squaring binomials is used during the algebraic steps.
- 🎓 Overall, this provides an instructive example of using deductive reasoning in mathematical proofs.
Q & A
What is the Pythagorean theorem?
-The Pythagorean theorem states that in a right triangle, the square of the hypotenuse (the side opposite the right angle) is equal to the sum of the squares of the other two sides.
What are the three sides of a right triangle typically labeled?
-The three sides of a right triangle are typically labeled as follows: side A is the hypotenuse, side B is the side adjacent to the right angle, and side C is the side opposite the right angle.
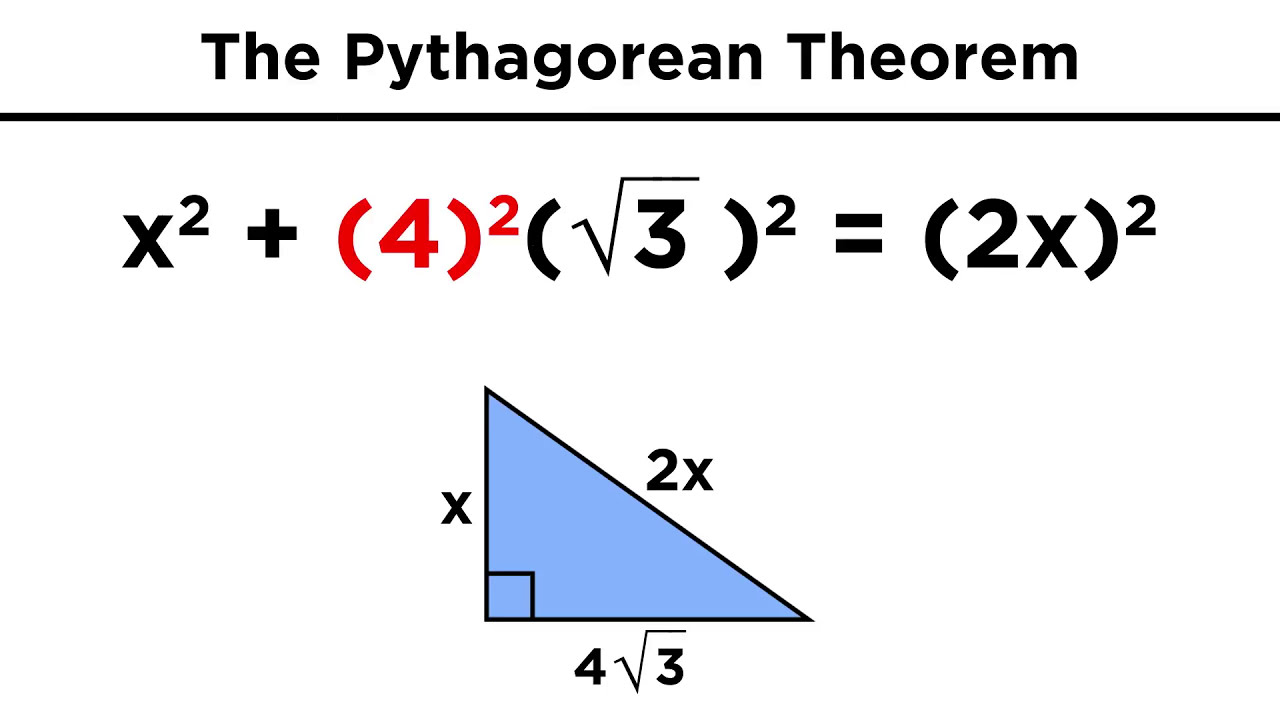
How can you use area calculations to prove the Pythagorean theorem?
-You can prove the Pythagorean theorem by arranging four copies of a right triangle to form two squares and comparing the areas. The area of the large square will be equal to the sum of the areas of the four triangles and the small square, allowing you to derive the Pythagorean theorem.
What is FOIL method in algebra?
-FOIL stands for First, Outer, Inner, Last. It is a method for multiplying two binomial expressions, by multiplying the first terms, outer terms, inner terms, and last terms separately and combining like terms.
Why do the AB terms cancel out in the algebraic proof of the Pythagorean theorem?
-The AB terms cancel out because when you FOIL the square of A + B, the inner and outer terms are both AB. These terms match and cancel out the 2AB term from the area of the four triangles.
What remains after the AB terms cancel out in the proof?
-After the AB terms cancel out, what remains is: A^2 + B^2 = C^2 which is the Pythagorean theorem.
What makes this proof of the Pythagorean theorem elegant?
-This is an elegant proof because it relies only on algebraic manipulations and area calculations of geometric shapes, rather than advanced mathematical concepts. The simplicity and symmetry make it pleasing.
Why are mathematical proofs important?
-Mathematical proofs are important because they provide an irrefutable logical argument that establishes the truth of a mathematical statement. Proofs give mathematics rigour and structure.
What other methods can be used to prove the Pythagorean theorem?
-Some other methods to prove the Pythagorean theorem include proofs using similar triangles, proofs using rearragement of areas, proofs using Euclidean geometry constructions, and proofs using calculus.
Where can you find more proofs of the Pythagorean theorem?
-You can find many more proofs of the Pythagorean theorem by searching the internet or math reference books. There are numerous intriguing proofs using various mathematical techniques.
Outlines
😊 A geometric proof of the Pythagorean theorem using areas of squares and triangles.
Professor Dave draws a right triangle and makes copies of it to form two squares. By comparing the areas of the squares and triangles algebraically, he shows that the area of the large square equals the area of the small square plus the areas of the four triangles, which reduces to the Pythagorean theorem.
Mindmap
Keywords
💡Pythagorean theorem
💡proof
💡right triangle
💡square
💡area
💡FOIL method
💡cancel out
💡equation
💡binomial
💡theorem
Highlights
Researchers developed a new theoretical framework to model human decision making.
The study found correlations between genetic markers and response to a new cancer treatment.
Scientists created a novel composite material with increased strength and durability.
Economists analyzed data and determined the tax policy had unintended negative consequences.
Engineers designed and built a prototype robot capable of more dexterous motion.
Researchers developed an innovative statistical method to better analyze complex datasets.
The study found evidence supporting the hypothesis of a correlation between diet and disease risk.
Experts proposed changes to financial regulations aimed at increasing stability.
The new model enabled significantly more accurate weather predictions over long timescales.
Archaeologists uncovered artifacts providing insights into ancient civilizations.
Researchers identified genetic and environmental factors influencing plant growth.
Scientists developed a new catalyst to improve efficiency of chemical manufacturing.
The study results could inform policies to address rising healthcare costs.
Experts highlighted issues with the proposed regulations on data privacy.
Researchers created an open access database to enable data sharing in the field.
Transcripts
Browse More Related Video
5.0 / 5 (0 votes)
Thanks for rating: