Alternating series test | Series | AP Calculus BC | Khan Academy
TLDRThe video script provides an in-depth explanation of the Alternating Series Test, a mathematical tool used to determine the convergence of infinite series. It breaks down the test's criteria, including the importance of a decreasing sequence and the limit of the sequence's terms approaching zero. The narrator illustrates the concept with a concrete example, demonstrating how the series of negative one to the power of N plus one over N converges, contrasting with the divergent Harmonic Series. The script also hints at other tests like the Limit Comparison Test, emphasizing the power of the Alternating Series Test in proving convergence.
Takeaways
- 📚 The script introduces the Alternating Series Test, a method to determine the convergence of an infinite series.
- 🔍 The series in question is expressed as the sum from N=K to infinity of A sub N, which can be rewritten with an alternating sign.
- 📉 The condition for the series to converge is that A sub N can be represented as (-1)^(N+1) * B sub N, where B sub N is non-negative and decreasing.
- 🎯 The two additional conditions for convergence are that the limit of B sub N as N approaches infinity is zero, and B sub N is a decreasing sequence.
- 🌐 The script applies the Alternating Series Test to a concrete example: the series from N=1 to infinity of (-1)^(N+1) / N.
- 🔢 The example series is analyzed step by step, showing how each term fits the criteria for the Alternating Series Test.
- 📉 The B sub N for the example is 1/N, which is verified to be greater than or equal to zero and a decreasing sequence.
- 📊 The limit of 1/N as N approaches infinity is shown to be zero, satisfying one of the convergence conditions.
- 📈 The series from N=1 to infinity of (-1)^(N+1) / N is concluded to converge based on the test.
- 🚫 The script clarifies that not passing the Alternating Series Test does not imply divergence; it only means that this test cannot prove convergence.
- 🔑 The Alternating Series Test is a powerful tool for proving convergence, in contrast to the Divergence Test which is used to show a series diverges.
Q & A
What is the purpose of the Alternating Series Test?
-The purpose of the Alternating Series Test is to determine whether an infinite series with alternating signs converges.
What is the general form of the series that the Alternating Series Test can be applied to?
-The Alternating Series Test can be applied to a series of the form \( \sum_{N=K}^{\infty} (-1)^N B_N \) where \( B_N \) is greater than or equal to zero for all relevant N, and \( (-1)^N \) represents the alternating sign.
What are the two additional conditions required for the Alternating Series Test to confirm the convergence of a series?
-The two additional conditions are: 1) The limit of \( B_N \) as N approaches infinity is zero, and 2) \( B_N \) forms a decreasing sequence.
Why is it important that \( B_N \) is a decreasing sequence in the Alternating Series Test?
-It is important because a decreasing sequence of positive terms ensures that the terms of the series are getting smaller as N increases, which is a necessary condition for the series to converge.
Can the Alternating Series Test be used to prove divergence of a series?
-No, the Alternating Series Test is only useful for proving convergence. If a series does not pass the test, it does not necessarily mean it diverges; it just means that this test cannot be used to prove its convergence.
What is an example of a series that the script applies the Alternating Series Test to?
-The script applies the Alternating Series Test to the series \( \sum_{N=1}^{\infty} \frac{(-1)^{N+1}}{N} \).
How does the script rewrite the general term \( A_N \) of the series for the Alternating Series Test?
-The script rewrites \( A_N \) as \( (-1)^{N+1} \times \frac{1}{N} \), where \( \frac{1}{N} \) is the \( B_N \) term in the test.
What is the limit of \( B_N \) as N approaches infinity in the example provided in the script?
-The limit of \( B_N \), which is \( \frac{1}{N} \), as N approaches infinity is zero.
Why does the script mention the Harmonic Series in the context of the Alternating Series Test?
-The script mentions the Harmonic Series to illustrate the difference between a series that diverges (the Harmonic Series without the alternating signs) and one that converges (the series with alternating signs that was tested).
What other technique is hinted at in the script that could be used to prove the convergence of the example series?
-The script hints at the Limit Comparison Test as another technique that could potentially be used to prove the convergence of the example series.
What is the significance of the term \( (-1)^{N+1} \) in the context of the Alternating Series Test?
-The term \( (-1)^{N+1} \) is significant because it ensures the series has alternating signs, which is a prerequisite for applying the Alternating Series Test.
Outlines
📚 Alternating Series Test Explanation
The narrator introduces the Alternating Series Test, a method for determining the convergence of an infinite series with alternating signs. The series is defined as a sum starting from N=K to infinity of A sub N, which can be rewritten as negative one to the power of N times B sub N, where B sub N is non-negative and forms a decreasing sequence. Two additional conditions are required for convergence: the limit of B sub N as N approaches infinity must be zero, and B sub N must be a decreasing sequence. The explanation is made concrete by applying the test to the series from N=1 to infinity of negative one to the power of N plus one over N, showing that it converges despite the Harmonic Series without the alternating signs not converging.
🔍 Further Analysis with Limit Comparison Test
The narrator mentions the possibility of using other techniques, such as the Limit Comparison Test, to prove the convergence of the series discussed. It is highlighted that the Alternating Series Test is a powerful tool for proving convergence, unlike the Divergence Test which is only useful for showing divergence when the limit of the terms does not approach zero. The narrator also clarifies that if a series does not pass the Alternating Series Test, it does not necessarily mean it diverges, just that the test cannot be used to prove its convergence.
Mindmap
Keywords
💡Alternating Series Test
💡Infinite Series
💡Convergence
💡Limit
💡Decreasing Sequence
💡B sub N
💡Negative One to the Power of N
💡Harmonic Series
💡Limit Comparison Test
💡Divergence Test
Highlights
Introduction to the Alternating Series Test for determining the convergence of infinite series.
Explanation of the conditions required for the Alternating Series Test: the series must be alternating, terms must approach zero, and the absolute values of terms must decrease monotonically.
Illustration of the Alternating Series Test using the series ∑(-1)^(N+1)/N from N=1 to infinity.
Rewriting the series to fit the Alternating Series Test criteria by expressing it as (-1)^(N+1) * (1/N).
Verification that B sub N (1/N) is greater than or equal to zero for all positive N.
Demonstration of the limit of B sub N (1/N) as N approaches infinity being equal to zero.
Confirmation that the sequence 1/N is a decreasing sequence as N increases.
Conclusion that the original series converges based on the satisfied conditions of the Alternating Series Test.
Comparison of the converging series with the diverging Harmonic Series to highlight the impact of alternating signs.
Mention of alternative techniques like the Limit Comparison Test for proving convergence.
Clarification that failure of the Alternating Series Test does not necessarily imply divergence.
Emphasis on the power of the Alternating Series Test as a tool for proving convergence.
Differentiation between the Alternating Series Test and the Divergence Test in terms of their utility and conclusions.
The importance of the test conditions: alternating signs, terms approaching zero, and monotonically decreasing absolute values.
The practical application of the test in analyzing the convergence of specific series.
The educational value of the test in understanding the behavior of infinite series.
The abstract nature of the test and its application to make the concept more concrete through examples.
Transcripts
Browse More Related Video
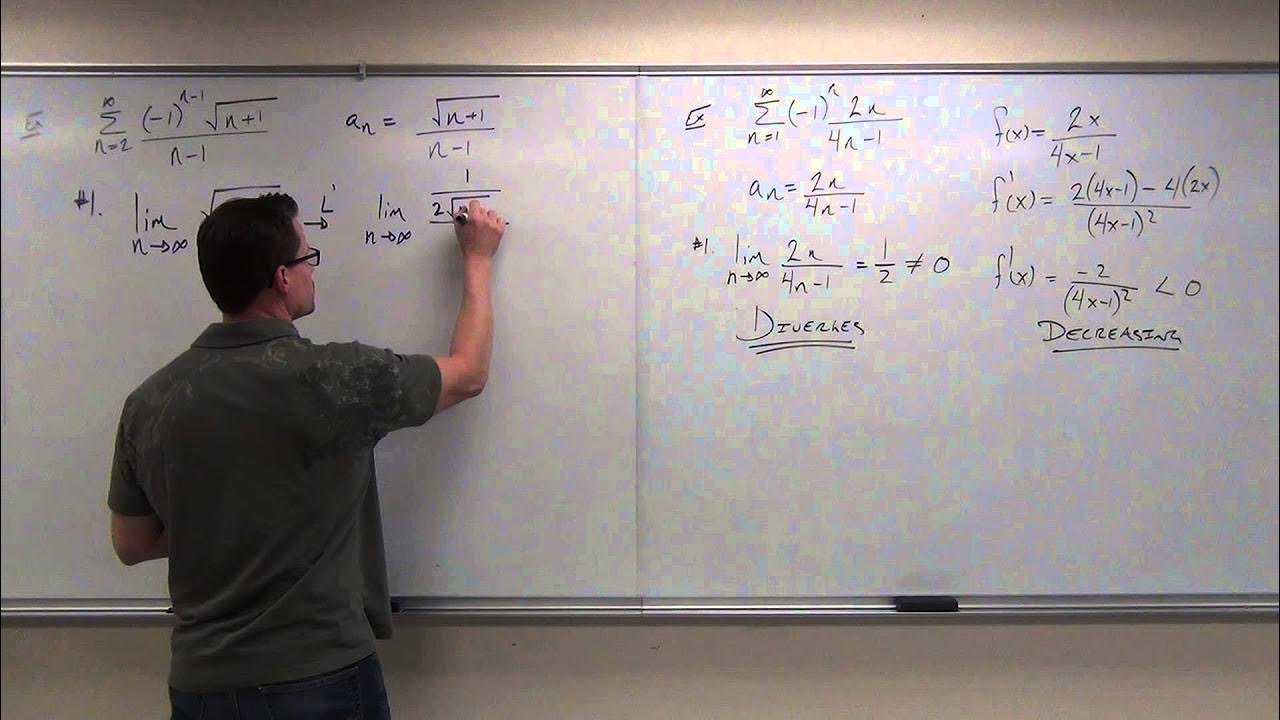
Calculus 2 Lecture 9.5: Showing Convergence With the Alternating Series Test, Finding Error of Sums

Calculus BC – 10.7 Alternating Series Test for Convergence
AP Calculus BC Lesson 10.7
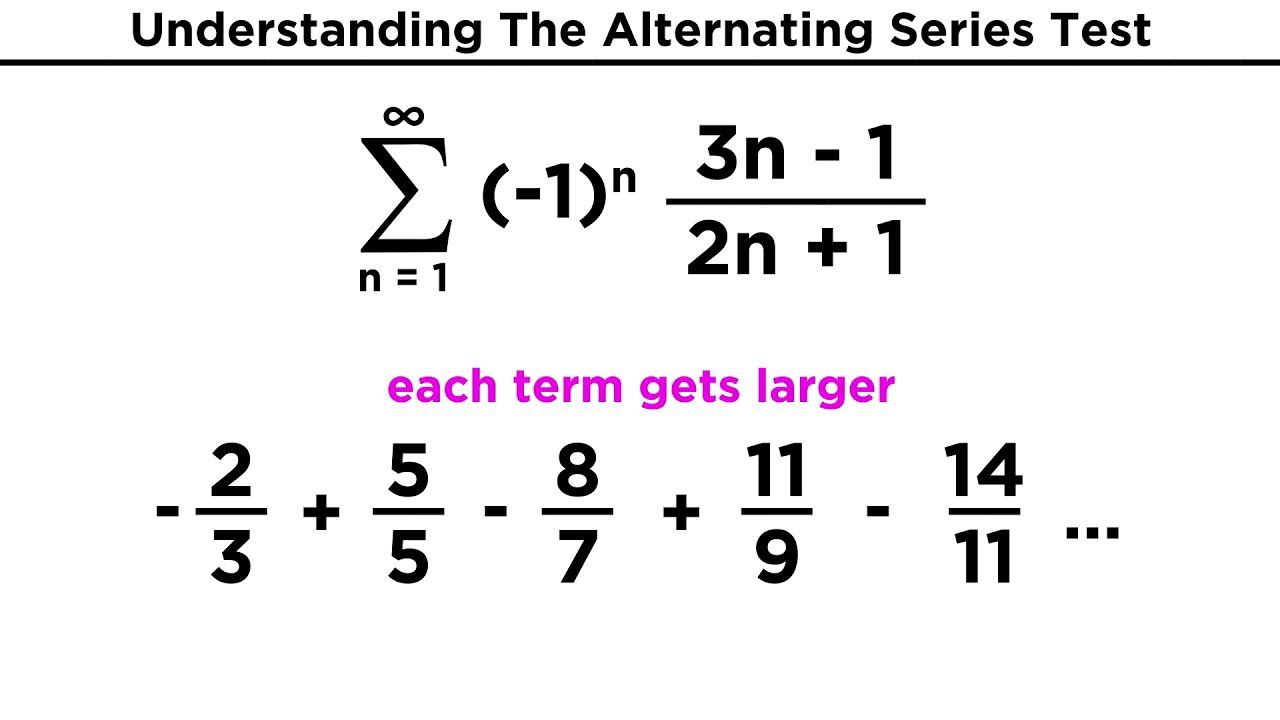
Alternating Series, Types of Convergence, and the Ratio Test

Conditional and Absolute Convergence for Infinite Series

The Root Test
5.0 / 5 (0 votes)
Thanks for rating: