The Root Test
TLDRThis lesson introduces the Root Test for determining the convergence of an infinite series. The test involves taking the nth root of the absolute value of the series' terms and examining the limit as n approaches infinity. If the limit is less than 1, the series converges; if greater than 1, it diverges; and if equal to 1, the test is inconclusive. The video provides step-by-step examples, demonstrating how to apply the Root Test to various series, including those with terms raised to the nth power and those with exponents containing n, concluding with the convergence or divergence of each series.
Takeaways
- 📚 The Root Test helps determine the convergence or divergence of an infinite series.
- ✏️ The Root Test states that if the limit of the nth root of the absolute value of a series' term (as n approaches infinity) is less than 1, the series converges.
- ❗ If the limit is greater than 1, the series diverges. If it equals 1, the test fails and another test must be used.
- 🔍 The Root Test is particularly useful when the series is raised to the nth power.
- ➗ The nth root of a number is the same as raising that number to the power of 1/n.
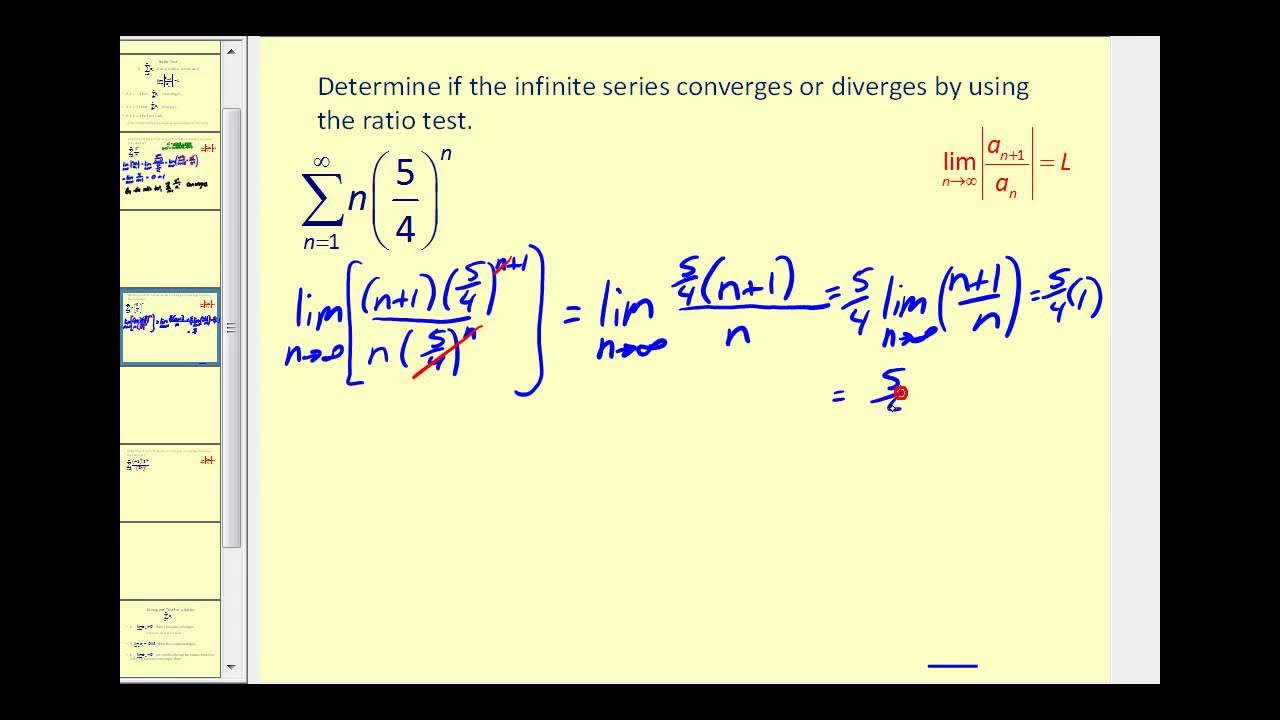
- 🔄 Example 1: For the series with the formula raised to the power of n, taking the nth root and evaluating the limit as n approaches infinity shows the series converges because the limit is 4/5, which is less than 1.
- 📉 Example 2: For the series where the exponents contain n, simplifying the exponents and evaluating the limit as n approaches infinity shows the series diverges because the limit is positive infinity, which is greater than 1.
- 🔢 Example 3: For the series where both the numerator and denominator contain n in the exponent, simplifying and evaluating the limit as n approaches infinity shows the series converges because the limit is 0, which is less than 1.
- 📐 Remember, powers raised to powers multiply the exponents.
- 🔎 When using the Root Test, always evaluate the limit as n approaches infinity to determine convergence or divergence.
Q & A
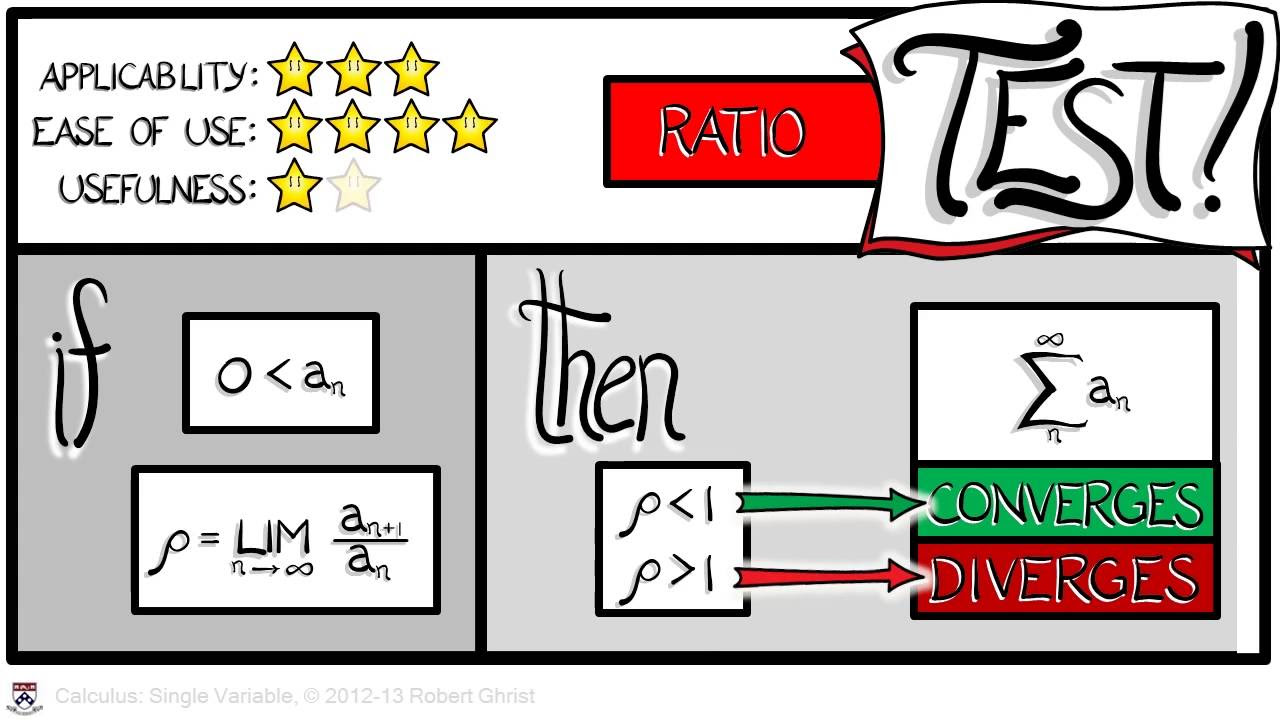
What is the Root Test for Convergence of an Infinite Series?
-The Root Test is a method used to determine the convergence of an infinite series. It states that if the limit as n approaches infinity of the nth root of the absolute value of the nth term of the series (a_sub_n) is less than 1, the series converges. If the limit is greater than 1, the series diverges. If the limit equals 1, the test is inconclusive, and a different test must be used.
What does it mean for the Root Test to be inconclusive?
-The Root Test is inconclusive if the limit of the nth root of the absolute value of the nth term equals 1. In this case, the test does not provide information about the convergence or divergence of the series, and another convergence test must be applied.
Why is the Root Test particularly useful when the series is raised to the nth power?
-The Root Test is especially helpful in cases where the terms of the series are raised to the nth power because the nth root can simplify the expression, making it easier to evaluate the limit of the nth root of the absolute value of the term.
How do you apply the Root Test to an infinite series?
-To apply the Root Test, you take the limit as n approaches infinity of the nth root of the absolute value of the nth term (a_sub_n). If this limit is less than 1, the series converges; if it's greater than 1, the series diverges; and if it's equal to 1, the test is inconclusive.
What is the significance of the leading coefficient in the limit calculation for the Root Test?
-When calculating the limit for the Root Test and the degree of the numerator and the denominator are the same, the limit is determined by the ratio of the leading coefficients. This simplifies the process of finding the limit, as only the leading terms need to be considered.
In the first example provided, why does taking the nth root simplify the expression?
-In the first example, the nth root simplifies the expression because the term is already raised to the nth power. Taking the nth root of something raised to the nth power results in the base of the expression, which in this case is (4n^2 + 1) / (5n^2 - 8).
What is the result of the first example's Root Test, and why does it indicate convergence?
-The result of the first example's Root Test is the limit as n approaches infinity of four-fifths (4/5), which is less than 1. This indicates that the series converges because the limit is less than 1 according to the Root Test criteria.
In the second example, why does the series diverge according to the Root Test?
-In the second example, the series diverges because the limit as n approaches infinity of the nth root of the absolute value of the term approaches positive infinity, which is greater than 1. This satisfies the condition for divergence in the Root Test.
What happens to the limit in the second example as n approaches infinity?
-In the second example, as n approaches infinity, the numerator approaches infinity while the denominator approaches a constant value (2 to the power of 1), resulting in a limit of positive infinity.
In the third example, why does the series converge according to the Root Test?
-The series in the third example converges because the limit as n approaches infinity of the nth root of the absolute value of the term equals zero, which is less than 1. This satisfies the condition for convergence in the Root Test.
What is the significance of the exponents in the third example for applying the Root Test?
-In the third example, the significance of the exponents is that they contain n, which allows for the application of the Root Test by raising the term to the power of one over n, simplifying the expression and making it easier to evaluate the limit.
Outlines
📚 Introduction to the Root Test for Series Convergence
This paragraph introduces the Root Test, a method for determining the convergence of an infinite series. It explains that if the limit of the nth root of the absolute value of the series' term (a sub n) approaches a value less than 1 as n goes to infinity, the series converges. Conversely, if the limit is greater than 1, the series diverges. If the limit equals 1, the test is inconclusive, and another test must be used. The paragraph emphasizes that the nth root of a term is equivalent to the term raised to the power of one over n, and provides an example where the series is already raised to the nth power, indicating the applicability of the Root Test.
🔍 Applying the Root Test with Series Examples
The second paragraph delves into applying the Root Test to specific series examples. It first presents a series with a term raised to the nth power and demonstrates the calculation of the limit of the nth root of the absolute value of the term. The limit is found to be the ratio of the leading coefficients of the numerator and denominator, which is less than 1, indicating convergence. The paragraph then discusses a series where the exponents contain n but are not raised to the nth power. By rewriting the term and applying the test, the limit is found to approach positive infinity, indicating divergence. The paragraph concludes with another example where both the numerator and denominator contain n as part of the exponent, setting the stage for the next step in the Root Test application.
Mindmap
Keywords
💡Root Test
💡Convergence
💡Divergence
💡nth Root
💡Absolute Value
💡Limit
💡Leading Coefficient
💡Infinite Series
💡Power
💡Exponent
💡Different Test
Highlights
The Root Test for Convergence of an Infinite Series is introduced.
The test is applicable when the series is generated by a sub N with a limit as N approaches infinity.
The limit L should be less than 1 for the series to converge.
If the limit L is greater than 1, the series diverges.
A limit L equal to 1 indicates the test fails and requires a different test.
The nth root of a is equivalent to a to the power of one over n.
The first example involves an infinite series with a formula raised to the power of n.
The nth root of a term raised to the nth power simplifies to the leading coefficient ratio.
The limit of the leading coefficients ratio determines the convergence of the series.
The second example does not have terms raised to the nth power but contains n in the exponents.
The root test can be applied by raising a sub n to the power of one over n.
Multiplying exponents simplifies the expression for the root test.
The limit approaches positive infinity, indicating divergence of the series.
The third example has both numerator and denominator with n in the exponents.
The limit of the third example equals zero, indicating convergence of the series.
The video concludes with a summary of the root test and its application.
Transcripts
5.0 / 5 (0 votes)
Thanks for rating: