AP Calculus BC Lesson 10.7

Elizabeth Fein

21 Jan 202316:07

EducationalLearning

32 Likes 10 Comments

TLDRThis video lesson delves into alternating series, explaining their defining characteristic of alternating signs between positive and negative terms. It provides examples of such series and illustrates how to determine their convergence or divergence using the Alternating Series Test. The test requires two conditions: the limit of the non-alternating part of the series must approach zero as n tends to infinity, and the terms must be non-increasing. The video also addresses the application of the test to various series and clarifies misconceptions about its use.

Takeaways

📌 Alternating series are mathematical series where the sign of the terms alternates between positive and negative.
🌟 Examples of alternating series include the series from n=1 to Infinity of (-1)^n * (1/n) and variations thereof.
🔍 The behavior of alternating series can be analyzed by plugging in different values of n to observe the pattern of terms.
📈 The harmonic series (1/1, 1/2, 1/3, 1/4, ...) is closely related to alternating series, with the main difference being the alternating signs.
🌐 The general form of an alternating series can be expressed as (-1)^n * (a_n), where a_n is the non-alternating part controlling the magnitude of terms.
🧐 The Alternating Series Test is a method to determine whether an alternating series converges or diverges.
🔢 For an alternating series to converge, two conditions must be met: lim(n→∞) a_n = 0 and a_n+1 ≤ a_n for all n, meaning the sequence a_n must be non-increasing.
📊 The convergence of a series can be demonstrated by taking the limit as n approaches Infinity of the non-alternating part a_n.
📉 To verify non-increase, one can analyze the trend of a_n by taking derivatives or simply observing if the sequence is decreasing.
🚫 If the degree of the numerator and the denominator of a series term are the same, the series may not converge by the Alternating Series Test and may need to be evaluated using other tests.
🔄 The Alternating Series Test can only be applied to series that are truly alternating; if the terms do not consistently alternate, the test is not applicable.

Q & A

What is an alternating series?
-An alternating series is a type of series in which the sign of the terms alternates between positive and negative.
Can you provide an example of an alternating series?
-An example of an alternating series is the series from n equals 1 to Infinity of (-1)^n times 1/n.
How does the alternating series resemble the harmonic series?
-The alternating series looks extremely similar to the harmonic series, with terms like 1, 1/2, 1/3, 1/4, etc., except that the sign of the terms alternates in the alternating series.
What is the Alternating Series Test, and how is it used to determine convergence or divergence of a series?
-The Alternating Series Test is a method used to determine whether an alternating series converges or diverges. It states that an alternating series converges if the limit as n approaches Infinity of the non-alternating part (a_n) is zero and a_n+1 is less than or equal to a_n for all n, meaning the sequence a_n must be non-increasing.
How can you verify if a sequence is non-increasing?
-To verify if a sequence is non-increasing, you can either take the derivative of the function and ensure it's negative for the values of interest, or simply observe the pattern of the sequence and ensure that each term is less than or equal to the previous term.
What happens when the degree of the numerator and the denominator of a term are the same in an alternating series?
-When the degree of the numerator and the denominator are the same, you take the leading coefficients for comparison. If the limit as n approaches Infinity of the non-alternating part (a_n) is not equal to zero, the series diverges by the nth term test, not the Alternating Series Test.
How does the presence of an oscillating function like cosine affect an alternating series?
-The presence of an oscillating function like cosine makes the terms of the series alternate between positive and negative, similar to (-1)^n, but with the function's values determining the sign of each term.
What are the two conditions that must be met for an alternating series to converge according to the Alternating Series Test?
-For an alternating series to converge, the limit as n approaches Infinity of the non-alternating part (a_n) must be zero, and a_n must be non-increasing (either decreasing or constant).
In the context of the Alternating Series Test, why can't a series with terms that are sometimes increasing be considered convergent?
-A series with terms that sometimes increase cannot be considered convergent by the Alternating Series Test because the series must be non-increasing to meet the test's conditions. Increasing terms violate this requirement.
How can you determine if a series converges using the Alternating Series Test for a series involving factorials?
-For a series involving factorials, you determine the limit as n approaches Infinity of the non-alternating part (1/n!) and verify that it is zero. Additionally, you must confirm that the sequence is non-increasing, which is true for 1/n! as the factorial increases with n.

Outlines

00:00

📘 Alternating Series Introduction and Examples

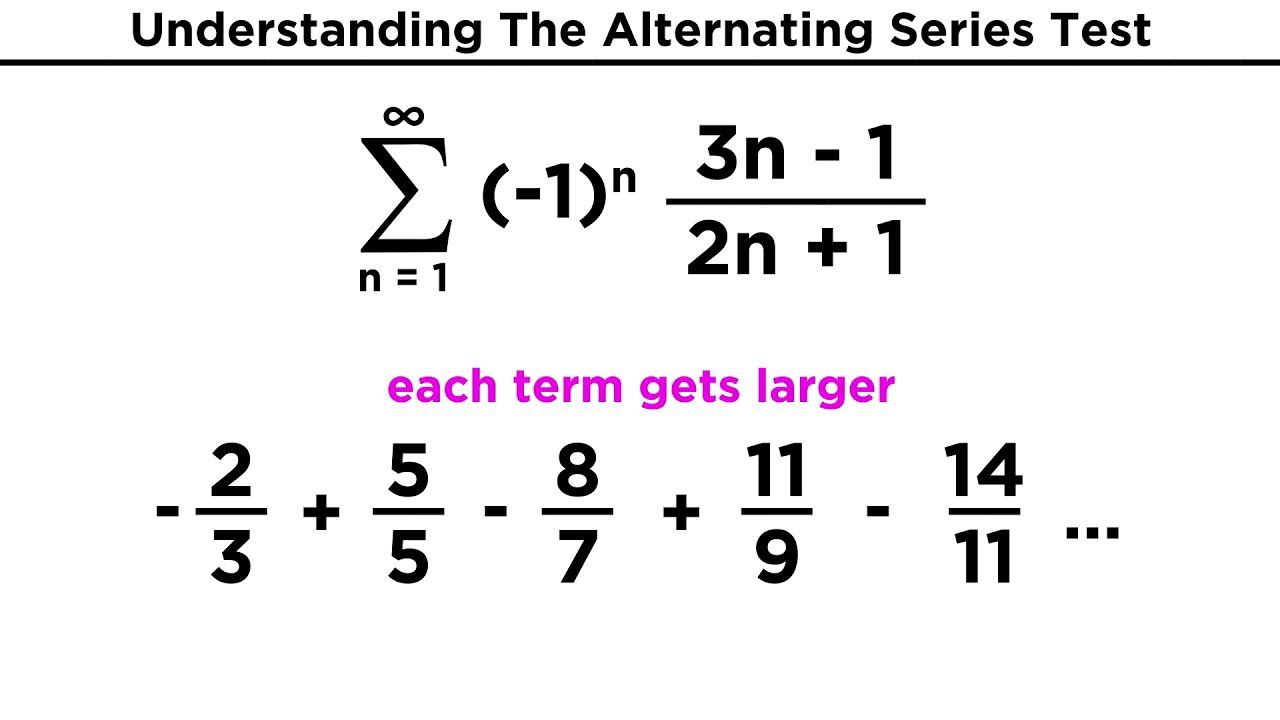
This paragraph introduces the concept of alternating series, where the sign of the terms alternates between positive and negative. It provides examples of alternating series, such as the series from n=1 to Infinity of (-1)^n/n, and explains how to calculate the terms by plugging in values for n. The paragraph also compares alternating series to the harmonic series and discusses the behavior of terms in these series. The concept of the alternating series test for convergence is introduced, with conditions explained for a series to converge: the non-alternating part of the series must approach zero as n approaches Infinity, and the sequence of non-alternating terms must be non-increasing.

05:02

📙 Applying the Alternating Series Test

This paragraph delves into applying the alternating series test to determine the convergence or divergence of various series. It explains how to identify the non-alternating part of the series and how to verify if it approaches zero as n approaches Infinity. The paragraph also discusses the need for the non-alternating terms to be non-increasing, which can be shown through derivatives or by inspection. Several examples are provided, with detailed explanations on how to apply the test and what the results indicate about the series' convergence or divergence.

10:03

📙 Multiple Choice Questions on Alternating Series Test

This paragraph presents multiple choice questions to test understanding of the alternating series test. It challenges the viewer to identify which series can have their convergence determined by the alternating series test based on the two conditions: the limit of the non-alternating part approaching zero and the non-increasing nature of the non-alternating terms. The paragraph also includes an explanation of why certain series cannot have their convergence determined by the alternating series test, either because the limit does not approach zero or because the terms are not non-increasing.

15:03

📘 Graphing to Determine Convergence

The final paragraph discusses the use of graphing to determine whether a series converges by the alternating series test. It highlights the importance of showing that the non-alternating part of the series approaches zero and that the sequence is non-increasing. The paragraph clarifies that while the graph may show the series approaching zero, if the sequence is not consistently non-increasing, the alternating series test cannot be applied to confirm convergence.

Mindmap

Keywords

💡Alternating Series

An alternating series is a type of mathematical series where the sign of each term alternates between positive and negative. This concept is central to the video, as it is used to describe and analyze various series, such as the series from n equals 1 to Infinity of (-1)^n times 1/n. The video provides examples and explains how to determine the convergence or divergence of such series using the alternating series test.

💡Convergence

In mathematics, convergence refers to the property of a sequence or series where the terms approach a certain value as the position in the sequence or series increases. The video focuses on determining the convergence of alternating series by examining if the series approaches a specific value or behaves in a predictable pattern. The alternating series test is introduced as a method to assess convergence.

💡Divergence

Divergence, in the context of series, indicates that the series does not approach a finite value. Instead, the terms of the series continue to increase without bound or oscillate indefinitely. The video explains how to determine if an alternating series diverges by using the alternating series test and checking if the conditions for convergence are not met.

💡Alternating Series Test

The alternating series test is a mathematical test used to determine whether an alternating series converges. It states that an alternating series converges if the limit of the non-alternating part of the series as n approaches infinity is zero, and if the sequence of the non-alternating parts is non-increasing. The video provides a detailed explanation and examples of how to apply this test to various series.

💡Non-increasing Sequence

A non-increasing sequence is one where each term is less than or equal to the previous term. In the context of the alternating series test, this means that the sequence of the absolute values of the non-alternating parts of the series does not increase as n gets larger. The video emphasizes that for an alternating series to converge, the non-alternating part of the series must form a non-increasing sequence.

💡Limit

In mathematics, a limit is the value that a function or sequence approaches as the input (or index) approaches some value. The video discusses taking the limit as n approaches infinity for the non-alternating part of an alternating series to determine if the series converges. The limit must equal zero for the alternating series test to be applicable.

💡Harmonic Series

The harmonic series is a divergent series where the terms are the reciprocals of the natural numbers (1, 1/2, 1/3, 1/4, etc.). The video compares the harmonic series to alternating series, noting that the harmonic series is similar to alternating series in that they both involve terms involving the reciprocal of n, but the sign of the terms in the alternating series changes with each term.

💡Cosine Function

The cosine function is a mathematical function that exhibits periodic behavior, oscillating between -1 and 1. In the video, the cosine function is used to create an alternating series by multiplying it with the term 1/n, resulting in the series Σ from n=1 to ∞ of cos(πn) * (1/n), which alternates signs because of the oscillating nature of the cosine function.

💡nth Term Test

The nth term test is a method used to determine the divergence of a series by examining the behavior of the nth term as n approaches infinity. If the nth term does not approach zero, the series is said to diverge. The video mentions the nth term test when discussing the divergence of a series with terms of the form n/(n+5), which does not approach zero as n increases.

💡Derivative

In calculus, a derivative represents the rate of change of a function with respect to its input variable. The video briefly mentions taking the derivative as a method to verify if a function is decreasing, which is relevant when applying the alternating series test to determine if a series is non-increasing.

💡Trigonometric Functions

Trigonometric functions, such as sine and cosine, are mathematical functions that relate the angles and sides of a triangle. In the video, the cosine function is used to create an alternating series, demonstrating how trigonometric functions can oscillate between positive and negative values, which is key for alternating series.

Highlights

Exploration of alternating series, where the sign of terms alternates between positive and negative.

Series example: from n=1 to Infinity of (-1)^n * (1/n), demonstrating the pattern of alternation.

Comparison to the harmonic series, with the key difference being the alternating sign in the alternating series.

Another alternating series example with (-1)^n + 1 as the alternating part and 1/n controlling the magnitude.

The series involving the cosine function, which oscillates between positive and negative, thus creating an alternating series.

Introduction of the alternating series test for determining convergence or divergence of alternating series.

Two conditions for the alternating series test: the limit of the non-alternating part must approach zero and the series must be non-increasing.

Example of determining convergence using the alternating series test for the series (-1)^n * (1/n).

Verification of the non-increasing nature of the series by examining the denominator's effect on the fraction's size.

Application of the alternating series test to other series, with specific examples provided for clarity.

Explanation of why certain series do not meet the criteria for the alternating series test, such as having a non-zero limit.

Use of calculus, specifically derivatives, as an alternative method to verify the decreasing nature of a series.

Multiple choice questions to test understanding of the alternating series test and its application.

Clarification that the alternating series test requires the series to be non-increasing, not strictly decreasing.

Discussion on the behavior of a series involving sine and cosine functions and their impact on the series' convergence.

Final multiple choice question assessing the ability to identify conditions under which the alternating series test can or cannot be applied.

Transcripts

Browse More Related Video

Calculus BC – 10.7 Alternating Series Test for Convergence

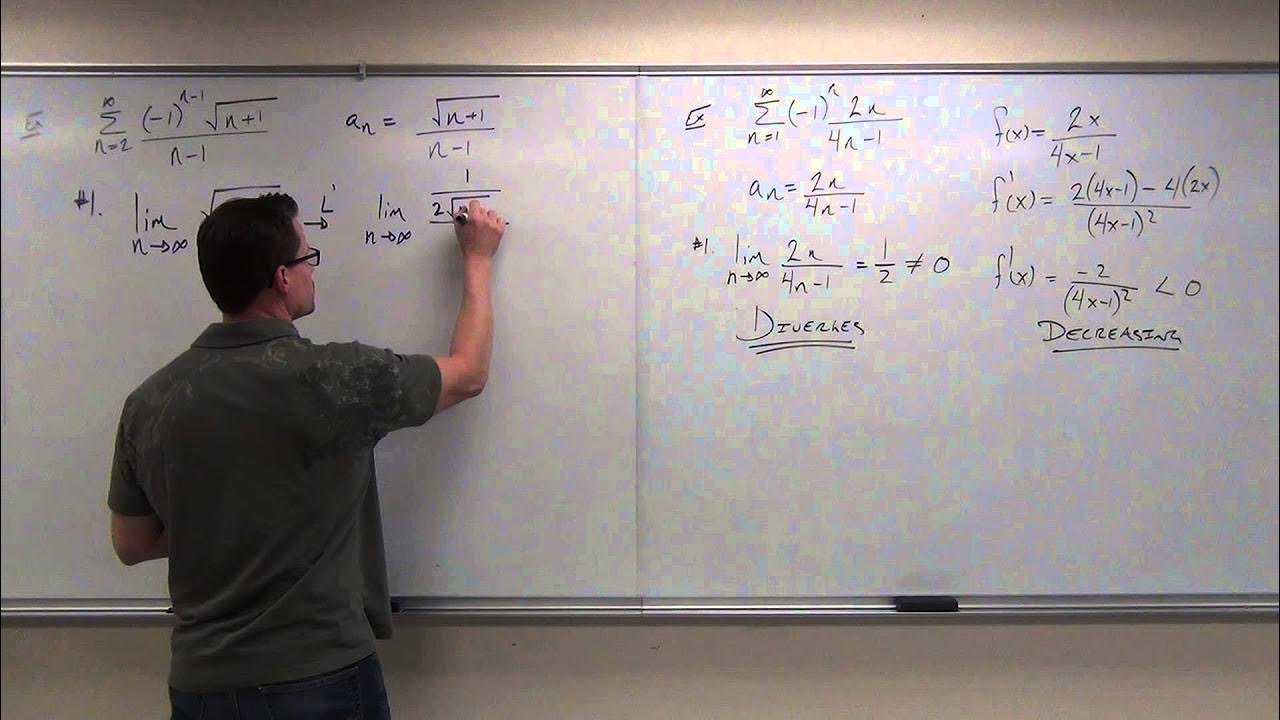
Calculus 2 Lecture 9.5: Showing Convergence With the Alternating Series Test, Finding Error of Sums

Alternating Series, Types of Convergence, and the Ratio Test

Alternating Series Test and Error for Alternating Series

Alternating Series

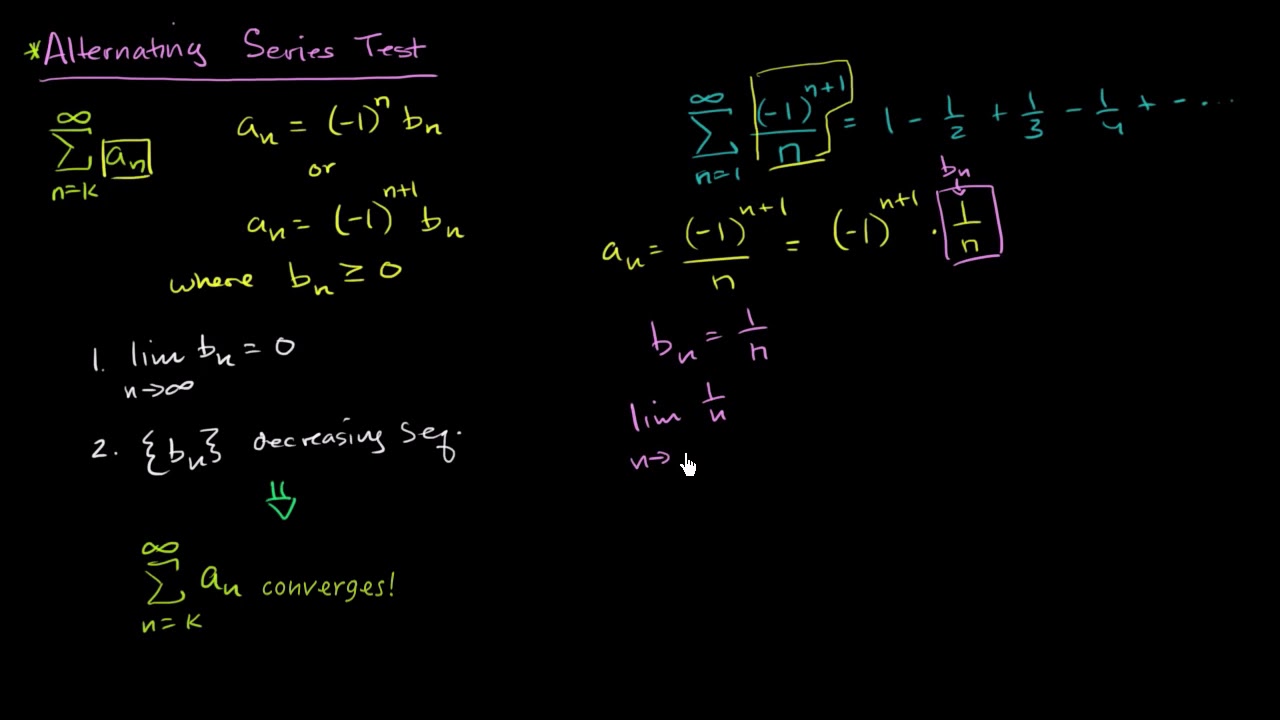
Alternating series test | Series | AP Calculus BC | Khan Academy