Math 119 Chapter 4 Part 1

The script begins with an introduction to Chapter 4, focusing on probabilities and their role in inferential statistics. It explains the concept of rare event rules, stating that if the probability of an observed event under a given assumption is very low, the assumption is likely incorrect. The instructor warns students that this section can be challenging and encourages them to seek help. The script then defines key terms such as 'event', 'simple event', and 'sample space', using the example of a woman's childbirth to illustrate these concepts. It further explains the difference between simple and non-simple events, emphasizing the importance of understanding the sample space for accurate probability calculations.

This paragraph delves into the three main approaches to calculating probability: relative frequency approximation, classical approach, and subjective approach. The relative frequency approach is based on the number of times an event occurs divided by the total number of trials. The classical approach requires equally likely outcomes and calculates the probability as the ratio of favorable outcomes to the total outcomes in the sample space. The subjective approach, on the other hand, estimates probability based on relevant circumstances and expert knowledge. Examples are provided to illustrate each approach, including tossing a coin, rolling a die, and predicting weather conditions.

The script presents an example to determine the sample space for the gender of three children in a family. It explains how to calculate the probability of having two boys out of three children using the classical approach, emphasizing the importance of identifying the number of favorable outcomes and the total number of outcomes in the sample space. The example also touches on the concept of sample space size and how it can grow exponentially with the number of events, highlighting the need to understand the size of the sample space for probability calculations.

This paragraph discusses the application of probability in sports, specifically using the example of NBA player Reggie Miller's free throw success rate. It demonstrates the use of the relative frequency approximation approach by calculating the probability of Miller making a free throw based on his career statistics. The summary emphasizes the high accuracy of Miller's free throws, suggesting that he is a reliable shooter from the free-throw line.

The script briefly mentions the probability of a car being struck by a meteorite, suggesting that this would be an example of using the subjective approach to probability due to the lack of concrete data on such rare events. It implies that the probability of such an event is extremely low, highlighting the use of expert knowledge and relevant circumstances in estimating probabilities for events with little historical data.

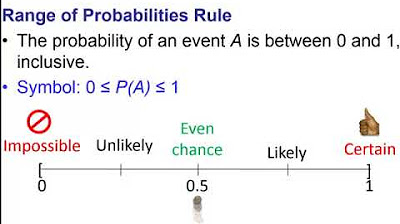
The Law of Large Numbers (LLN) is introduced, stating that as an event is repeated, the relative frequency of the event tends to approach the actual probability. The script uses the example of flipping a coin to illustrate how the true probability of the event (50/50 heads/tails) will eventually manifest over a large number of trials. It also discusses the boundaries of probability, which must fall between zero and one, inclusive. The concept of complementary events and the complement rule are explained, showing how to calculate the probability of an event not occurring by subtracting the event's probability from one.

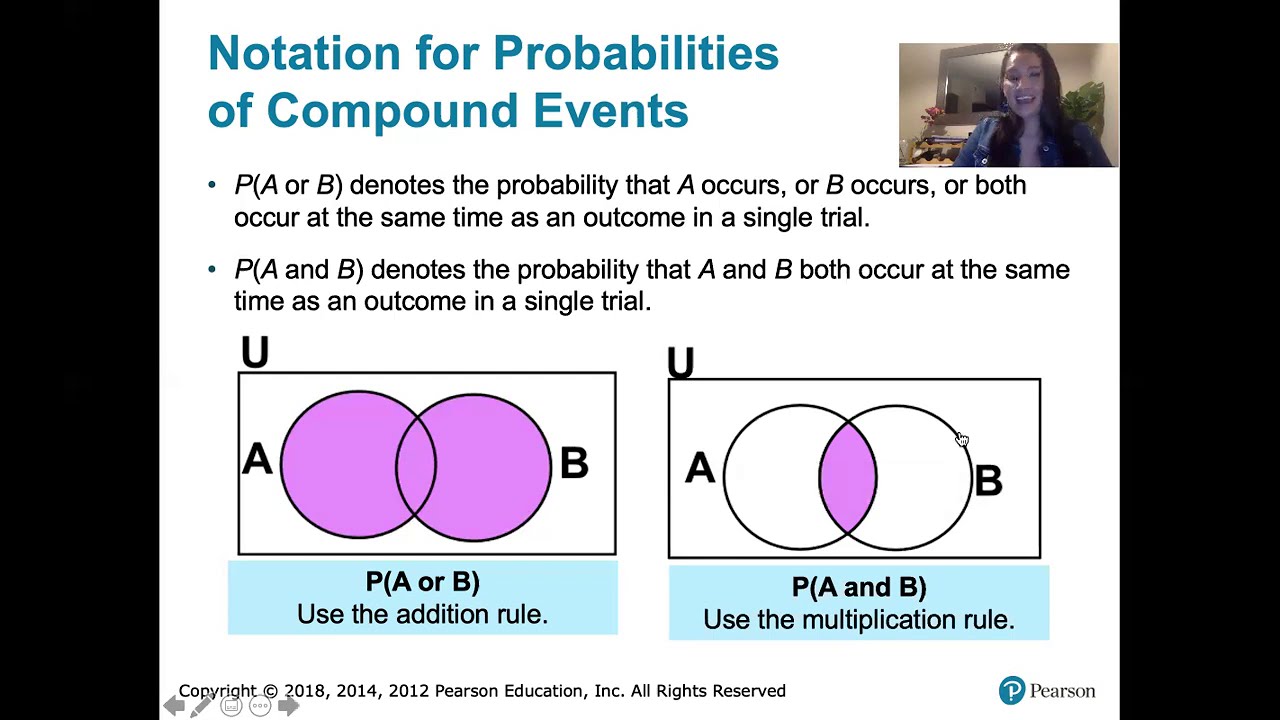
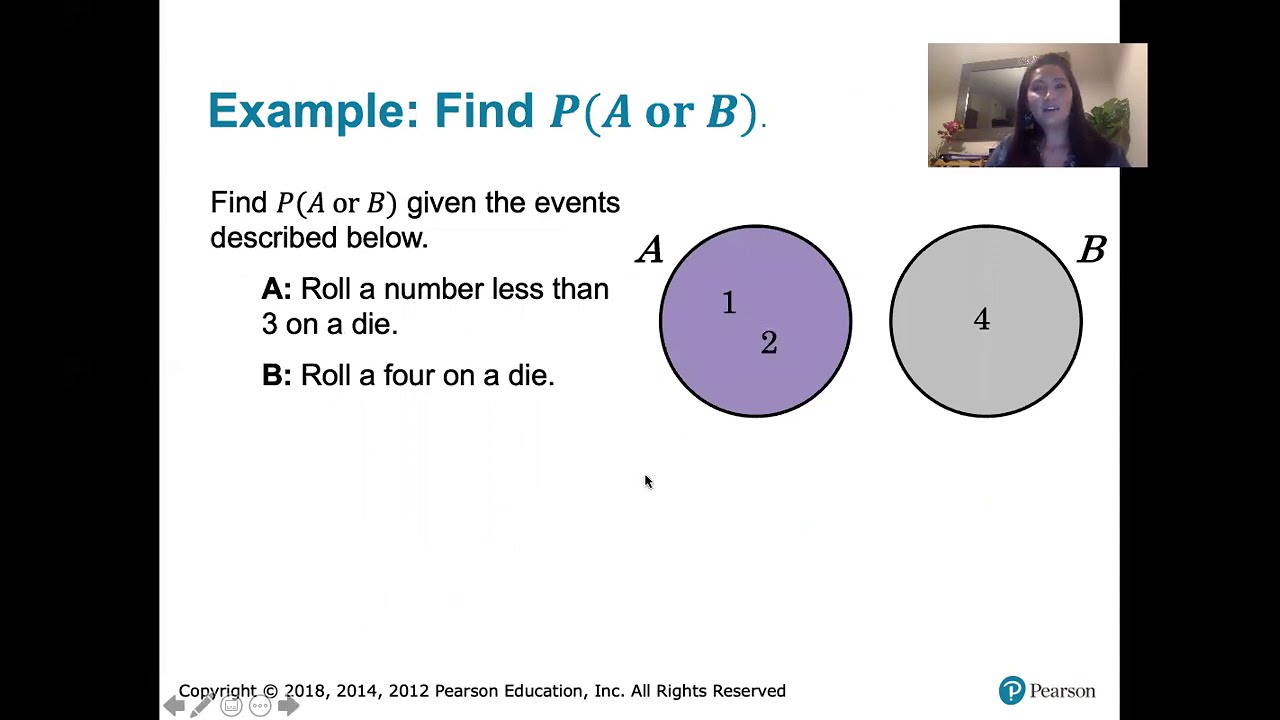
The Addition Rule for probability is explained, which is used to find the probability of either event A or event B occurring. The rule states that the probability of A or B is the sum of their individual probabilities minus the probability of both A and B occurring together (to avoid double counting). The script uses a Venn diagram to visually represent the concept and provides a real-world example involving pre-employment drug screening test results. It demonstrates how to calculate the probability of selecting a subject with a positive test result and the probability of selecting a subject who actually used drugs, as well as the combined probability using the Addition Rule.

The script discusses the scenario where events A and B are disjoint, meaning they cannot occur simultaneously. In such cases, the Addition Rule simplifies to the sum of the probabilities of A and B since there is no overlap to subtract. An example involving political affiliation is given, where the probability of an American being either a Republican or a Democrat is calculated by simply adding the individual probabilities of each affiliation, assuming they are mutually exclusive.

The instructor concludes the first video on probability, summarizing the key points covered and indicating that the chapter will be continued in subsequent videos. The summary includes a brief recap of the topics discussed, such as the different approaches to probability, the Law of Large Numbers, the Addition Rule, and the concept of disjoint events. The instructor thanks the viewers and expresses anticipation for further discussions in class.