Statistics Lecture 4.2: Introduction to Probability
TLDRThe video script delves into the concept of probability in statistics, distinguishing between descriptive and inferential statistics. It explains the basis of probability for decision-making, emphasizing that low probability indicates rare occurrences. Key terms like events, simple events, and sample space are introduced with examples, such as flipping a coin. The script further explores observed, classical, and subjective probabilities, using relatable examples like baseball statistics and card drawings. The importance of understanding these concepts is highlighted for accurate statistical analysis and prediction.
Takeaways
- 📊 Probability is a fundamental concept in statistics, serving as the basis for making decisions about data.
- 🎲 Low probability events are considered rare or unlikely to occur, guiding our expectations about their occurrence.
- 🔢 The vocabulary of statistics introduces key terms like events, simple events, and sample spaces to describe possible outcomes.
- 🎯 An 'event' is a collection of outcomes from a procedure, representing what you're looking for in your analysis.
- 🥌 A 'simple event' is a single, specific outcome from a procedure, such as getting heads in a coin flip.
- 🃏 The 'sample space' encompasses all possible outcomes of a procedure, like every potential result from flipping a coin.
- 🔄 Complementary events are mutually exclusive outcomes that together cover all possible outcomes of a procedure.
- 🔢 The probability of an event, plus the probability of its complement, always equals 1, representing the complete sample space.
- 📈 Observed probability is based on actual data from performed experiments or observations.
- 📐 Classical probability is theoretical, assuming each outcome has an equal chance of occurring, like the expected outcome of a fair coin flip.
- 🤔 Subjective probability is based on an individual's judgment or educated guess, without concrete data or observation.
Q & A
What is the main idea behind probability?
-The main idea behind probability is to make decisions about data based on the likelihood of certain outcomes occurring. It is a measure of how likely an event is to happen, with low probability events considered rare or unusual.
What is an 'event' in the context of statistics?
-In statistics, an 'event' is a collection of outcomes from a procedure. It refers to a specific outcome or set of outcomes that one is interested in when conducting a statistical analysis.
What is the difference between a 'simple event' and an 'event'?
-A 'simple event' is a single outcome from a procedure, whereas an 'event' is a collection of outcomes that represent what is being looked for in a statistical analysis. An event can be composed of one or more simple events.
What is the 'sample space' in statistics?
-The 'sample space' is the set of all possible outcomes that could result from a procedure. It includes every simple event that could occur when the procedure is performed.
How is 'observed probability' different from 'classical probability'?
-Observed probability is based on actual data collected from performing an experiment or observation, while classical probability is based on theoretical calculations assuming each outcome has an equal chance of occurring.
What is 'subjective probability'?
-Subjective probability is an individual's estimate of the likelihood of an event occurring based on personal judgment, experience, or available information. It is not based on actual data or theoretical calculations but rather on an educated guess.
How do you calculate the probability of an event?
-The probability of an event is calculated by dividing the number of times the event occurs by the total number of possible outcomes in the sample space.
What is the law of large numbers?
-The law of large numbers states that as the number of trials or observations increases, the observed probability of an event will get closer to the theoretical or classical probability.
What are 'complementary events'?
-Complementary events are two events that are mutually exclusive and together cover all possible outcomes of a sample space. The occurrence of one event implies the non-occurrence of the other, and vice versa.
What is the relationship between the probability of an event and its complement?
-The probability of an event plus the probability of its complement always equals 1, as they together cover all possible outcomes and represent a certain event occurring.
Outlines
📊 Introduction to Probability and Events
This paragraph introduces the concept of probability as a transition from descriptive to inferential statistics. It explains the basics of probability, stating that low probability events are rare and uses coin flipping as an example. The paragraph also introduces key vocabulary such as 'event', 'simple event', and 'sample space', setting the stage for further discussion on these terms.
🎲 Explaining Events and Simple Events
The speaker elaborates on the concepts of events and simple events using the example of flipping a coin. An event is a specific outcome that one is looking for, while a simple event is a single outcome from a procedure. The sample space is the set of all possible outcomes. The discussion includes the idea of listing all simple events for flipping a coin three times and understanding the relationship between events, simple events, and the sample space.
🔢 Calculating Simple and Total Events
This section delves into the calculation of simple and total events, particularly focusing on the number of ways an event can be accomplished. It uses the example of flipping a coin three times and finding the number of heads and tails. The speaker emphasizes understanding the difference between an event (what is being looked for) and simple events (the ways to accomplish that event), and how they relate to the sample space.
🎯 Understanding Procedures, Events, and Sample Spaces
The speaker clarifies the relationship between procedures, events, simple events, and sample spaces. A procedure is the action being performed, an event is a specific outcome of that procedure, a simple event is an individual outcome, and the sample space is the collection of all possible outcomes. The discussion includes examples of flipping a coin and rolling a die to illustrate these concepts and how they interrelate.
📈 Types of Probability
This paragraph introduces the three types of probability: observed, classical, and subjective. Observed probability is based on actual data from performed experiments, classical probability is based on theoretical equal chances of outcomes, and subjective probability is an estimate based on educated guesses or personal judgment. The speaker provides examples from everyday life, such as batting averages in baseball and doctors' estimates of health outcomes, to illustrate these types of probability.
🃏 Probability of Selecting a Heart from a Deck of Cards
The speaker calculates the classical probability of selecting a heart from a standard deck of cards. By dividing the number of hearts (13) by the total number of cards (52), the probability is found to be 0.25 or 25%. This example demonstrates how to calculate the probability of a simple event in a classical sense, assuming each card has an equal chance of being selected.
🏈 Peyton Manning's Pass Completion Probability
Using historical data, the speaker calculates the probability of Peyton Manning completing a pass. By dividing the number of completed passes (385) by the total number of passes attempted (528), the probability is approximately 72.9%. This example illustrates the calculation of observed probability, as it is based on actual performance data.
🃏 Probability of Selecting a Two from a Deck of Cards
The speaker calculates the classical probability of randomly selecting a two from a standard deck of cards. There are four twos in the deck, so the probability is 4 out of 52, or approximately 7.7%. This example shows how to calculate the probability of a simple event without conducting an actual experiment, based on the theoretical distribution of cards.
📊 Public Opinion on Cloning: An Observed Probability
The speaker discusses a poll on public opinion about cloning, where 91 out of 901 respondents viewed cloning positively. This is an example of observed probability, as it is based on actual data collected from a poll. The calculated probability is approximately 10.1%, indicating the likelihood of a randomly selected person from the poll sample having a positive view on cloning.
🤔 Subjective Probability and Complementary Events
The speaker introduces the concept of subjective probability, which is based on personal judgment rather than data or theory. An example is given where the speaker humorously estimates the probability of a bird pooping on a car. The speaker also introduces the idea of complementary events, which are mutually exclusive and together encompass all possible outcomes, such as rolling a five or not rolling a five on a die.
👶 Probability of Having Two Boys with Three Children
The speaker calculates the classical probability of having two boys if a couple has three children, assuming equal chances of having a boy or a girl. The sample space is listed with all possible gender combinations, and the probability is found by dividing the number of favorable outcomes (three ways to have two boys and one girl) by the total number of outcomes (eight total combinations). The probability is 37.5%, illustrating the calculation of classical probability based on theoretical chances.
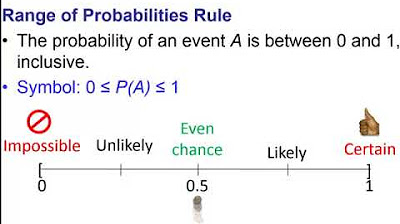
📐 Laws of Probability
The speaker discusses the laws of probability, emphasizing that probabilities always fall between 0 and 1, with 0 representing an impossible event and 1 representing a certain event. The law of large numbers is introduced, stating that as the number of trials increases, observed probability will approach classical probability. The speaker also explains the concept of complementary events and their probabilities summing up to 1, as they cover all possible outcomes.
Mindmap
Keywords
💡Probability
💡Event
💡Simple Event
💡Sample Space
💡Observed Probability
💡Classical Probability
💡Subjective Probability
💡Complementary Events
💡Law of Large Numbers
💡Mutually Exclusive
Highlights
Probability as the basis for making decisions about data
Understanding low probability as rare or unusual occurrences
Vocabulary related to probability, including 'event', 'simple event', and 'sample space'
An event being a collection of outcomes from a procedure
A simple event as a single, specific outcome
Sample space encompassing all possible outcomes of a procedure
Illustration of concepts through the example of flipping a coin
Procedures, events, simple events, and sample spaces explained using coin flipping
Understanding the difference between an event and simple events
Exploration of the types of probability: observed, classical, and subjective
Observed probability based on actual observations or experiments
Classical probability based on theoretical equal chances of outcomes
Subjective probability as an estimate based on an educated guess
The relationship between simple events and events, and how they relate to the sample space
The concept of complementary events and how they are mutually exclusive
The law of large numbers and how it relates to observed and classical probabilities
The probability of an event plus the probability of its complement equals one
Explanation of how to calculate probabilities using the formula P(A) = Number of favorable outcomes / Total number of outcomes
Discussion on the probability of selecting a heart from a standard deck of cards as a classical probability example
Calculation of the probability of Peyton Manning completing a pass using observed data
Transcripts
Browse More Related Video

Math 119 Chapter 4 Part 1

6.4.3 The Central Limit Theorem - The Rare Event Rule and the Central Limit Theorem

4.1.0 Basics of Probability - Lesson Overview, Key Concepts and Learning Outcomes
Chapter 4 Probability Part 1
Probability and Statistics Made Easy: Essential for Data Scientists

Tutorial 1- What Is Statistics And What Are Its Types In Hindi?
5.0 / 5 (0 votes)
Thanks for rating: