4.2.1 Addition and Multiplication Rules - Simple and Compound Events, and Their Probabilities
TLDRThis video script focuses on the concept of simple and compound events in probability theory. It defines simple events as specific outcomes that cannot be broken down further, while compound events combine two or more simple events. The script introduces the addition and multiplication rules for calculating probabilities of compound events, using examples such as consecutive births and die rolls to illustrate the concepts. It also explains the notation for the probability of 'A or B' and 'A and B', and how these relate to Venn diagrams, setting the stage for further lessons on the addition rule.
Takeaways
- 📚 The lesson focuses on understanding the difference between simple and compound events and their relation to probability rules.
- 🔍 Simple events are outcomes that cannot be broken down further, while compound events combine two or more simple events.
- 🎯 The addition and multiplication rules are essential for calculating the probabilities of compound events, which were previously computed using four different approaches for simple events.
- 👶 An example of a simple event is three consecutive births with two girls followed by a boy, as there's only one way this specific sequence can occur.
- 👨👧👦 Conversely, the result of two girls and a boy from three births is a compound event, as it can happen in three different ways.
- 🎲 Rolling a single die and getting a five is a simple event because there's only one outcome for this specific result.
- 🎯 Rolling an even number on a single die is a compound event because there are three possible outcomes (rolling a 2, 4, or 6).
- 📝 The notation for the probability of event A or B is represented differently from the probability of both A and B occurring simultaneously.
- 📈 The probability of A or B is calculated by adding the individual probabilities of A and B, considering all possible ways each can occur.
- 🔢 The probability of A and B occurring together is found by identifying the overlap in a Venn diagram and dividing by the total number of outcomes.
- 📚 The lesson will delve deeper into the addition rule in the next lesson and the multiplication rule later in the semester.
Q & A
What is the main goal of the lesson discussed in the video?
-The main goal is for viewers to be able to distinguish between simple and compound events and to understand how these events relate to the addition and multiplication rules for computing probabilities.
What are simple events according to the video?
-Simple events are outcomes or specific events that cannot be further broken down into simpler components.
How are compound events defined in the video?
-Compound events are events that combine two or more simple events and are not simple themselves.
What are the addition and multiplication rules mentioned in the video?
-The addition and multiplication rules are methods for finding probabilities of compound events, which are discussed in the lesson.
Can you provide an example of a simple event from the video?
-An example of a simple event is the outcome of two girls followed by a boy in three consecutive births, as there is only one way this specific sequence can occur.
How many ways can the result of two girls and a boy occur in three births according to the video?
-The result of two girls and a boy can occur in three different ways, making it a compound event.
What is an example of a simple event when rolling a single die?
-Rolling a five on a single die is a simple event because there is only one way to achieve this outcome.
What is an example of a compound event when rolling a single die?
-Rolling an even number is a compound event because it can occur in three different ways (rolling a two, four, or six).
What does the notation P(A or B) represent in the context of the video?
-P(A or B) represents the probability that event A occurs, or event B occurs, or both A and B occur at the same time in a single trial.
What does the notation P(A and B) represent in the video?
-P(A and B) represents the probability that both events A and B occur at the same time in a single trial.
How are the probabilities of compound events found using the addition rule as described in the video?
-The probabilities of compound events are found by adding up all the ways that event A can occur and all the ways that event B can occur, and then dividing by the total number of outcomes.
What will be the focus of the next video in the series?
-The next video will discuss learning outcome number two, which is not specified in the provided transcript.
Outlines
📚 Understanding Simple and Compound Events
This paragraph introduces the concepts of simple and compound events in probability theory. Simple events are outcomes that cannot be broken down further, such as rolling a five on a die. Compound events, on the other hand, are combinations of two or more simple events, like getting two girls followed by a boy in three consecutive births. The paragraph also explains the relevance of these concepts to the addition and multiplication rules for calculating probabilities, which will be discussed in subsequent lessons. Examples are provided to illustrate the differences between simple and compound events, and the importance of distinguishing between them is emphasized for understanding probability calculations.
📈 Probabilities of Compound Events and Notation
The second paragraph delves deeper into the concept of compound events, focusing on how to calculate their probabilities. It introduces the notation for the probability of either event A or B occurring, denoted as P(A or B), and explains it using a Venn diagram. The paragraph clarifies the difference between the probability of A or B and the probability of A and B occurring simultaneously, P(A and B), which will be calculated using the multiplication rule later in the course. The summary also touches on the importance of understanding these probabilities for accurate probability computations in various scenarios.
Mindmap
Keywords
💡Simple Events
💡Compound Events
💡Addition Rule
💡Multiplication Rule
💡Probability
💡Outcome
💡Venn Diagram
💡Mutually Exclusive Events
💡Independent Events
💡Total Number of Outcomes
💡Learning Outcome
Highlights
The video discusses the learning outcome from lesson 4.2, focusing on distinguishing between simple and compound events.
The goal is to understand the relationship between these events and the addition and multiplication rules for computing probabilities.
Simple events are defined as outcomes that cannot be further broken down into simpler components.
Compound events are events that combine two or more simple events and are not simple themselves.
Examples of simple and compound events are provided to illustrate the concepts.
The addition and multiplication rules are introduced as tools for finding probabilities of compound events.
A specific example of three consecutive births is used to explain simple events.
The possibility of different outcomes in three births of two girls and a boy is presented as a compound event.
Rolling a single die and the outcomes of rolling a five versus an even number are used to distinguish between simple and compound events.
Notation for the probability of event A or B is introduced, including its representation in a Venn diagram.
The probability of A and B occurring simultaneously is explained, with its notation and Venn diagram representation.
The addition rule for probabilities is mentioned as a method to be discussed in the next lesson.
The multiplication rule for finding the probability of both A and B occurring is introduced.
The video concludes with a summary of the discussion on compound events and a preview of the next learning outcome.
The importance of understanding the addition and multiplication rules for calculating probabilities of compound events is emphasized.
The video aims to equip viewers with the ability to apply these rules to various probability scenarios.
Transcripts
Browse More Related Video

4.1.1 Basics of Probability - Events, Simple Events, and Sample Spaces
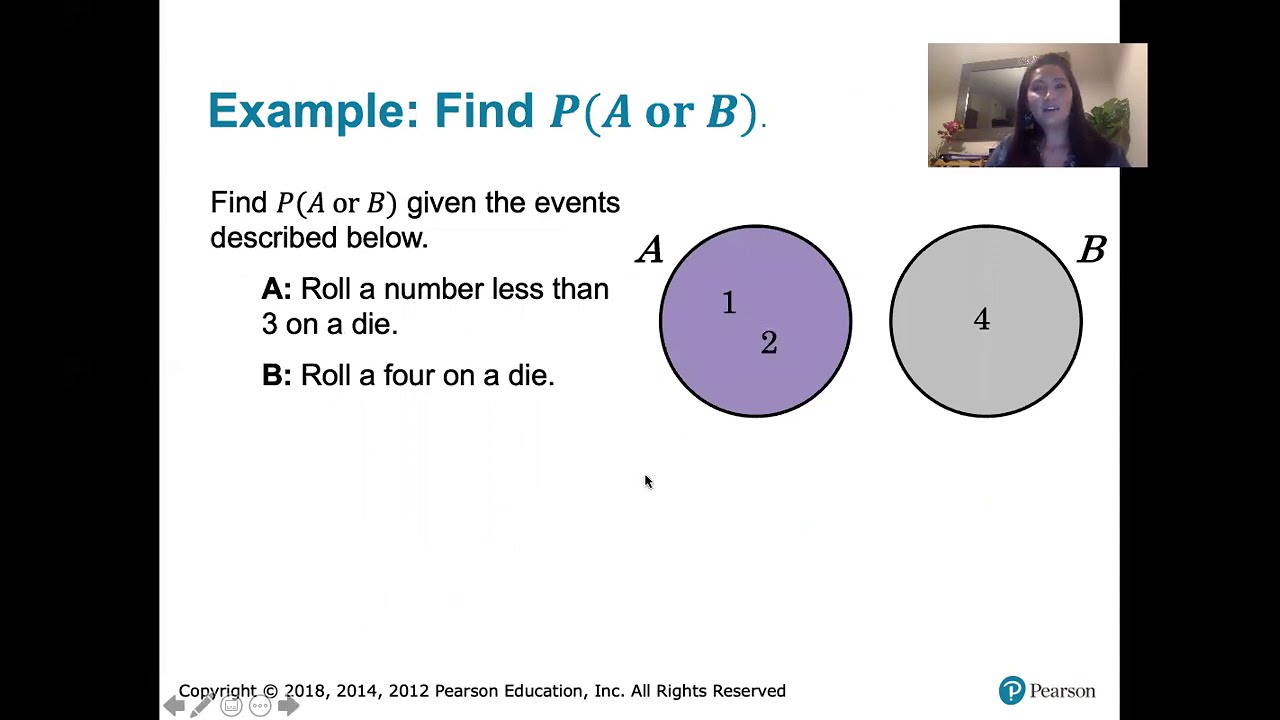
4.2.3 Addition and Multiplication Rules - Disjoint Events and Implications for Probability
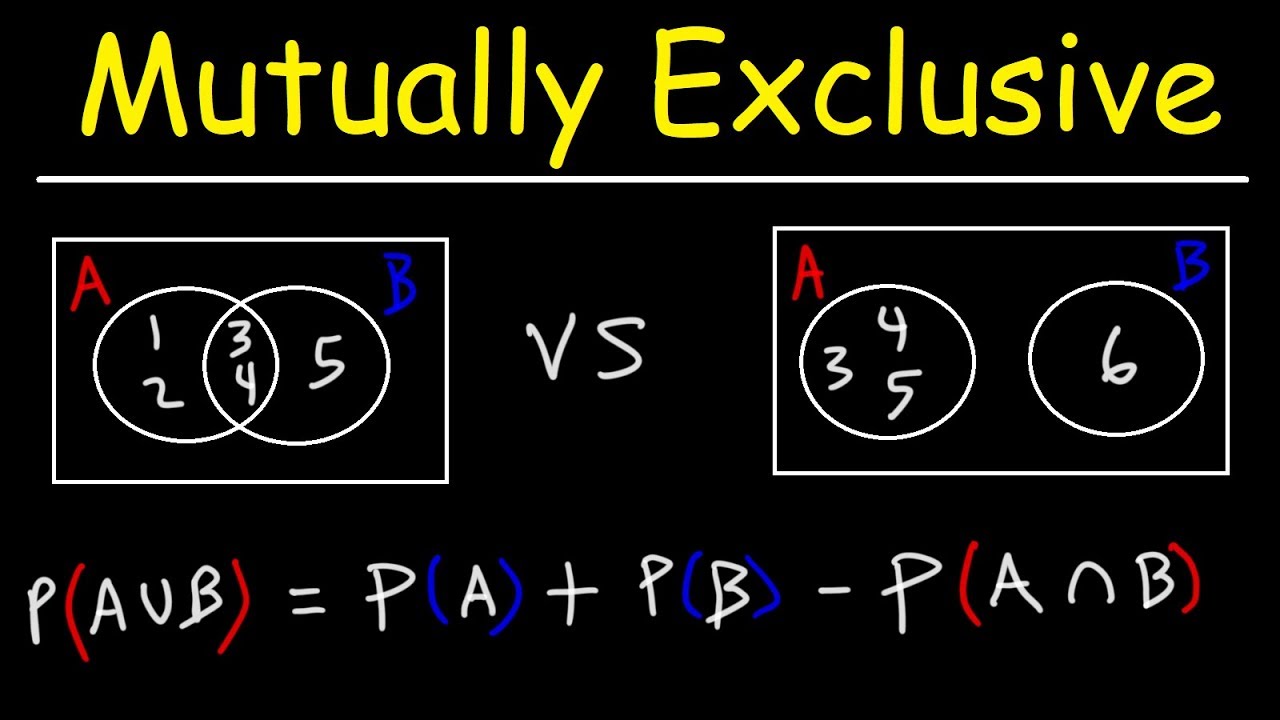
Probability of Mutually Exclusive Events With Venn Diagrams

Math 119 Chapter 4 Part 1
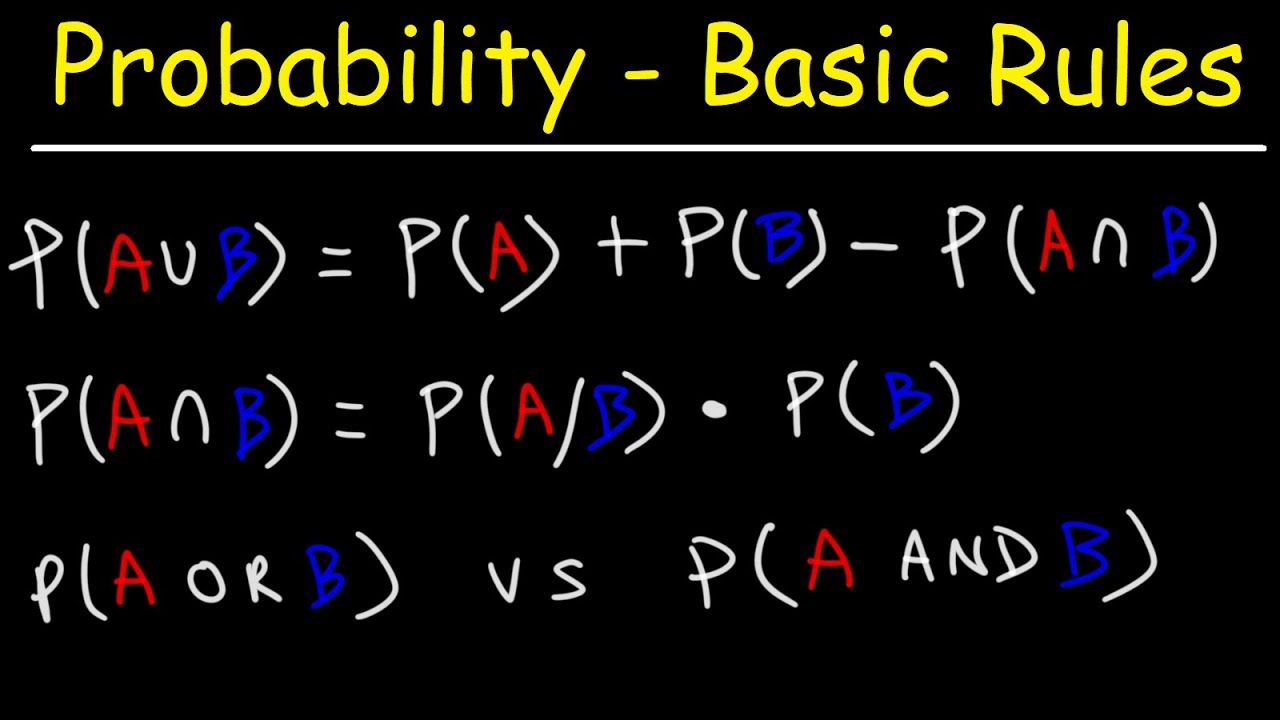
Multiplication & Addition Rule - Probability - Mutually Exclusive & Independent Events
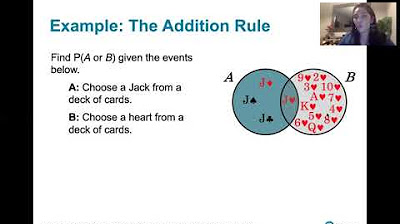
4.2.2 Addition and Multiplication Rules - The Addition Rule
5.0 / 5 (0 votes)
Thanks for rating: