4.1.0 Basics of Probability - Lesson Overview, Key Concepts and Learning Outcomes

Sasha Townsend - Tulsa
7 Oct 202003:46
EducationalLearning
32 Likes 10 Comments

TLDRThis video offers an insightful overview of Lesson 4.1, focusing on the fundamental concepts of probability in statistics. It delves into the role of probability, explains how to interpret probability values, and discusses odds in relation to probabilities. The lesson outlines five learning outcomes, including defining key terms, calculating probabilities using four common methods, understanding the law of large numbers, applying the rare event rule in inferential statistics, and comprehending odds in gambling contexts. The rare event rule, a crucial concept for the course, is highlighted for its relevance in subsequent chapters.

Takeaways
  • 📚 Overview of lesson 4.1 on basic concepts of probability.
  • 📖 The lesson is from Chapter 4 of the Essentials of Statistics, 6th edition by Mario Triola.
  • 📝 Focus on three sections of the chapter due to time constraints.
  • 🎲 Understanding the role of probability in statistics.
  • 🔢 Learning to interpret probability values, which range from 0 to 1.
  • 📊 Discussing the significance of small probabilities (e.g., 0.001) and large probabilities (e.g., 0.99).
  • 🔄 Introduction to important probability terms: events, simple events, and sample spaces.
  • 📈 Explanation of four common approaches to estimate or find the probability of an event.
  • 📐 Understanding the law of large numbers and its use in interpreting relative frequency approximations.
  • ♻️ Defining the complement of an event and finding its probability.
  • 📉 Introduction to the rare event rule for inferential statistics and its importance in the course.
  • 🎰 Explanation of odds, including odds against, odds in favor, and payoff odds, especially in gambling contexts.
Q & A

  • What is the main topic of lesson 4.1 in the video?

    -The main topic of lesson 4.1 is the basic concepts of probability.

  • Why are only three sections of the chapter on probability covered in the course?

    -Due to time constraints, only three sections of the chapter on probability are covered in the course.

  • Which textbook and edition is used for the slides in this lesson?

    -The slides are based on the Essentials of Statistics, sixth edition by Mario Triola.

  • What is the role of probability in statistics as discussed in the video?

    -Probability is used to understand and express the likelihood of events occurring, which is crucial in the field of statistics.

  • How are probability values interpreted?

    -Probability values are numbers between 0 and 1, where 0 means an event will not occur and 1 means an event will certainly occur. Values in between represent varying degrees of likelihood.

  • What does a probability value of 0.001 indicate?

    -A probability value of 0.001 indicates a very rare event.

  • What does a probability value of 0.99 indicate?

    -A probability value of 0.99 indicates an event that is very likely to occur.

  • What are the five learning outcomes for this lesson?

    -The five learning outcomes are: defining important terms related to probability, calculating probabilities, defining the complement of an event, stating the rare event rule for inferential statistics, and understanding odds and their relation to probabilities.

  • Why is the rare event rule important?

    -The rare event rule is important because it helps infer whether a certain number of successes is significantly high or low, which is crucial for understanding later chapters in the course.

  • What is the significance of understanding odds in this lesson?

    -Understanding odds is significant because it helps in interpreting probabilities in practical contexts, such as gambling, lotteries, and other situations involving chance.

Outlines
00:00
📚 Introduction to Lesson 4.1: Basic Concepts of Probability

This paragraph introduces Lesson 4.1 from the textbook 'Essentials of Statistics' by Mario Triola, focusing on fundamental probability concepts. It sets the stage for the chapter on probability within an hour course, noting that while there are many valuable sections, only three are covered due to time constraints. The lesson aims to define key terms related to probability, such as events and sample spaces, and to explain how to calculate probabilities using four common methods. Additionally, it previews the importance of understanding the rare event rule in inferential statistics, which will be a recurring theme throughout the course, and introduces the concept of odds in the context of gambling, which is particularly relevant to the local area.

Mindmap
Keywords
💡Probability
Probability is a measure of the likelihood that a specific event will occur. It is expressed as a number between 0 and 1, where 0 indicates an impossible event and 1 indicates a certain event. In the video, understanding probability is fundamental as it forms the basis of the lesson's focus on statistical analysis.
💡Events
In probability, an event is any outcome or combination of outcomes from a random experiment. The video distinguishes between simple events, which consist of a single outcome, and more complex events. This term is essential for defining the sample spaces and calculating probabilities.
💡Sample Spaces
A sample space is the set of all possible outcomes of a probability experiment. The video introduces this concept to help students understand how probabilities are calculated. Knowing the sample space is critical for determining the likelihood of different events.
💡Rare Event Rule
The rare event rule states that if an event has a very low probability of occurring under a given assumption, the occurrence of that event is a good indication that the assumption is not true. This concept is emphasized in the video as a key takeaway for interpreting statistical results throughout the course.
💡Law of Large Numbers
The law of large numbers is a principle that states as the number of trials in a probability experiment increases, the experimental probability of an event will get closer to the theoretical (true) probability. The video discusses this law to illustrate how repeated experiments help approximate probabilities more accurately.
💡Odds
Odds are a way of expressing the likelihood of an event happening in comparison to it not happening. The video explains different types of odds, such as odds against, odds in favor, and payoff odds, and relates them to probabilities, especially in gambling contexts like casinos and lotteries.
💡Complement of an Event
The complement of an event is the set of outcomes in the sample space that are not part of the event. The video covers how to calculate the probability of the complement, which is crucial for understanding the full range of possible outcomes in a probability experiment.
💡Relative Frequency Approximations
Relative frequency approximations involve using the frequency of an event occurring in past trials to estimate its probability. The video highlights this approach as one of the methods for calculating probabilities, linking it to the practical application of the law of large numbers.
💡Inferential Statistics
Inferential statistics involves using data from a sample to make inferences about a larger population. The video introduces the rare event rule in the context of inferential statistics, emphasizing its importance for making predictions and decisions based on statistical data.
💡Essentials of Statistics
This refers to the textbook 'Essentials of Statistics' by Mario Triola, which is the primary resource for the course. The video mentions that the content, particularly chapter 4, forms the basis of the lesson on probability, underscoring the structured approach to learning these concepts.
Highlights

Introduction to basic concepts of probability in Lesson 4.1.

Focus on three sections of Chapter 4 from 'Essentials of Statistics' by Mario Triola.

Understanding the role of probability in statistics.

Introduction to the rare event rule in statistics.

Learning to interpret probability values between 0 and 1.

Explanation of what a probability of 0 and 1 means.

Small probability values like 0.001 indicate very rare events.

Large probability values like 0.99 indicate events likely to occur.

Understanding odds and their relation to probabilities.

Defining important probability terms including events and sample spaces.

Learning to calculate probabilities using four common approaches.

Discussion of the law of large numbers and relative frequency approximations.

Defining and finding the probability of the complement of an event.

Introduction to the rare event rule for inferential statistics.

Application of the rare event rule to determine significantly high or low events.

Significance of the rare event rule in future chapters of the course.

Understanding odds against, odds in favor, and payoff odds.

Relevance of understanding odds in contexts like casinos and gambling.

Conclusion of the overview for Lesson 4.1 on the basics of probability.

Transcripts

Browse More Related Video

4.1.5 Basics of Probability - Odds and Their Relation to Probability

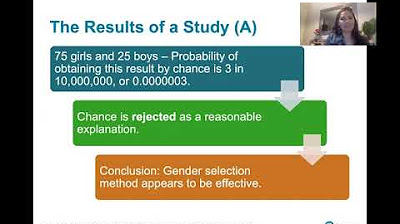
4.1.4 Basics of Probability - The Rare Event Rule of Inferential Statistics

Math 119 Chapter 4 Part 1

Introduction to Probability, Basic Overview - Sample Space, & Tree Diagrams

Statistics Lecture 4.2: Introduction to Probability

6.4.3 The Central Limit Theorem - The Rare Event Rule and the Central Limit Theorem

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Probability BasicsStatistics LessonEssentials of StatisticsMario TriolaRare Event RuleLikelihood InterpretationComplement EventsLaw of Large NumbersOdds UnderstandingGambling ConceptsInferential Statistics