4.1.2 Basics of Probability - Four Approaches to Find or Estimate Probabilities
TLDRIn this video, the speaker discusses four common approaches to estimating probabilities and interpreting relative frequency approximations. Using the 'law of large numbers,' they explain the core concepts of probability, including notation, interpreting probabilities, and different methods such as relative frequency approximation, classical approach, subjective probabilities, and simulations. Real-life examples, like skydiving deaths and rolling dice, are used to illustrate these concepts. The importance of understanding probabilities within the range of 0 to 1, along with common pitfalls, is emphasized to help viewers accurately interpret and estimate probabilities.
Takeaways
- 📚 The video discusses four common methods for estimating probabilities: relative frequency approximation, classical approach, subjective probability, and simulations.
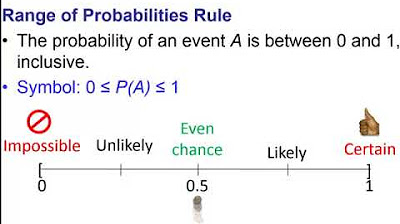
- 🎲 Probability is a measure of the likelihood of an event occurring, represented by numbers between 0 and 1, with 0 being impossible and 1 being certain.
- 📈 The relative frequency approximation estimates probability by dividing the number of times an event occurred by the total number of trials, which is based on past data and relies on the law of large numbers.
- 🎯 The classical approach to probability involves dividing the number of ways an event can occur by the total number of equally likely outcomes in the sample space.
- 🤔 Subjective probabilities are personal estimates based on intuition and understanding of the situation, without necessarily relying on empirical data.
- 🔮 Simulations estimate probabilities by mimicking the procedure of interest and counting the occurrences of the event in the simulated outcomes.
- 📉 Probabilities can never be negative or greater than one, as they represent a measure of likelihood and must fall within the range of 0 to 1.
- 🔄 The law of large numbers states that as the number of trials increases, the relative frequency of an event will tend to get closer to the actual probability.
- ❌ It's incorrect to assume that all outcomes are equally likely when using the classical approach; this should only be applied when all outcomes have the same chance of occurring.
- 📉 When calculating probabilities, it's common to express results as fractions, decimals, or percentages to understand the likelihood better.
- ⚠️ A common mistake is dividing the smaller number by the larger number when calculating probabilities, which is incorrect; the correct method is to divide the number of occurrences of the event by the total number of trials or observations.
Q & A
What are the four common approaches to estimating probabilities discussed in the video?
-The four common approaches to estimating probabilities discussed in the video are relative frequency approximation, classical approach, subjective probabilities, and simulations.
What is the notation typically used to denote a specific event in probability?
-The notation typically used to denote a specific event in probability is capital letters like A, B, and C. For example, P(A) represents the probability of event A occurring.
How does the video use the movie 'Dumb and Dumber' to explain the concept of probability?
-The video uses the famous line from 'Dumb and Dumber' where one character asks about the chances of a certain event happening, to illustrate the concept of probability as a measure of likelihood.
What is the range of values for probabilities and why is this range used?
-Probabilities range from 0 to 1 because they represent a measure of likelihood between the extremes of impossibility (0) and certainty (1). Negative probabilities and probabilities greater than one are not used because they do not make sense in the context of likelihood.
How does the law of large numbers relate to the relative frequency approximation of probability?
-The law of large numbers states that as a procedure is repeated, the relative frequency of an event occurring tends to approach the actual probability, which is the classical probability considering the number of ways an event can occur out of all possible outcomes.
What is the difference between the classical approach and the relative frequency approximation when estimating probabilities?
-The classical approach calculates probability based on the number of ways an event can occur (s) divided by the total number of possible outcomes (n), assuming all outcomes are equally likely. The relative frequency approximation estimates probability based on the number of times an event occurred divided by the number of trials conducted, using historical data.
Why is it inappropriate to use the classical approach to estimate the probability of dying during a skydiving jump?
-It is inappropriate to use the classical approach in this context because the outcomes (dying or surviving) are not equally likely, and various factors such as training, weather, and equipment condition influence the probability of an accident.
What is a subjective probability and how does it differ from the other methods of estimating probabilities?
-A subjective probability is an estimate made based on an individual's intuition and understanding of relevant circumstances. It differs from other methods as it is not based on historical data or theoretical calculations but rather on personal judgment.
How does the video explain the concept of a 'certain event' in terms of probability?
-The video explains a 'certain event' as an event that has a 100% chance of happening, which corresponds to a probability of 1. This means that the event is guaranteed to occur.
What is the significance of the law of large numbers in probability calculations and why is it important?
-The law of large numbers is significant because it provides a theoretical foundation for the relative frequency approximation, showing that as the number of trials increases, the relative frequency of an event will converge to its true probability. It is important because it validates the use of past data to estimate future probabilities.
Can you provide an example of a common mistake made when calculating probabilities and how to avoid it?
-A common mistake is dividing the number of occurrences of an event by the wrong total, such as dividing the number of texters by the number of non-texters instead of the total number of drivers. To avoid this, always divide the number of times an event occurred by the total number of trials or observations.
Outlines
📚 Introduction to Probability Concepts
The video begins with an introduction to learning outcome number two, focusing on understanding four common methods for estimating or finding probabilities. It uses a humorous reference from the movie 'Dumb and Dumber' to illustrate the concept of probability. The narrator explains the notation for probabilities, using capital letters to denote specific events, and emphasizes the importance of interpreting probabilities as measures of likelihood, typically represented by proportions between zero and one.
🎲 Understanding Probability Notation and Interpretations
This paragraph delves deeper into the notation and interpretation of probabilities. It discusses how probabilities are represented by fractions or decimals and how they can be thought of in terms of percentages. The video uses the example of the sun rising to illustrate the concept of likelihood and explains the difference between impossible, certain, and likely events, emphasizing that probabilities range from zero to one and cannot be negative or greater than one.
📈 Methods for Estimating Probabilities
The script outlines four methods for estimating probabilities: relative frequency approximation, classical approach, subjective probabilities, and simulations. The relative frequency approximation is based on past data, while the classical approach considers possible outcomes and their likelihood. Subjective probabilities are personal estimates based on intuition, and simulations provide an approximation by mimicking the actual procedure. The paragraph also clarifies that a zero probability does not necessarily mean an event is impossible.
🪂 Real-World Application: Skydiving Accidents
An example is provided to illustrate the use of the relative frequency approximation, using data on skydiving accidents. The video calculates the probability of dying during a skydiving jump based on the number of deaths out of the total number of jumps. It emphasizes that this is an approximation and relates the concept back to the law of large numbers, which suggests that as the number of trials increases, the relative frequency will approach the actual probability.
🎯 Classical Probability and Its Limitations
The script explains the classical approach to probability, which involves calculating the probability of an event based on the number of ways it can occur out of all possible outcomes, assuming equal likelihood. An example of rolling an odd number on a die is given. The video also discusses the inappropriateness of using the classical approach when outcomes are not equally likely, such as in the case of skydiving survival.
🔢 The Law of Large Numbers and Its Implications
The law of large numbers is introduced, stating that as a procedure is repeated, the relative frequency of an event will tend to approach the actual probability. The video uses the coin flipping example to clarify this concept. It also cautions against misinterpreting the law, emphasizing that it does not predict outcomes of individual trials but rather describes the behavior of probabilities over a large number of trials.
🚫 Avoiding Common Pitfalls in Probability Calculations
The script warns against common mistakes in calculating probabilities, such as assuming equally likely outcomes when they are not. It stresses the importance of considering relevant circumstances and not treating all outcomes as if they have the same probability of occurring. The video also touches on subjective probabilities and the use of simulations to estimate probabilities.
🤔 Considerations in Probability Estimation
This paragraph discusses the importance of considering various factors that may influence the probability of an event, such as preparation and external conditions. It advises against oversimplifying probabilities to a 50-50 chance without considering the complexity of real-world scenarios. The video also introduces the concept of subjective probability, where individuals estimate the likelihood of events based on their intuition and knowledge of relevant circumstances.
📉 Rounding Conventions and Probability Calculations
The video script concludes with a discussion on rounding conventions for probabilities, suggesting the use of exact fractions or decimals when possible, and rounding to three significant digits for longer decimals. It also highlights a common error in calculating probabilities, such as dividing the smaller number by the larger number without considering the total sample size, and provides a correct method for calculating the probability of a high school driver texting while driving based on a given study.
Mindmap
Keywords
💡Probability
💡Relative Frequency Approximation
💡Law of Large Numbers
💡Classical Approach to Probability
💡Subjective Probability
💡Simulation
💡Impossible Event
💡Certain Event
💡Proportion
💡Sample Space
Highlights
The video discusses four common methods for estimating probabilities and interpreting relative frequency approximations.
Probability is introduced as a measure of likelihood, with notation and examples provided.
The concept of relative frequency approximation is explained using the law of large numbers.
A humorous reference to the movie 'Dumb and Dumber' is used to illustrate the concept of unlikely probabilities.
The importance of distinguishing between probabilities represented as fractions or decimals and percentages is emphasized.
Examples of interpreting probabilities, such as impossible events having a probability of zero, are given.
The classical approach to probability is introduced, which involves calculating probabilities based on equally likely outcomes.
Subjective probabilities are explained as personal estimates based on intuition and understanding of relevant factors.
Simulations are presented as a method to estimate probabilities by mimicking the occurrence of events.
A real-world example of estimating the probability of death in skydiving using relative frequency approximation is discussed.
The law of large numbers is related to the convergence of relative frequency to actual probability with a large number of trials.
The difference between the classical approach and the relative frequency approximation is clarified with examples.
The inappropriateness of using the classical approach for events that are not equally likely, such as skydiving survival, is explained.
The importance of not misinterpreting the law of large numbers in relation to single events is highlighted.
The video addresses the common mistake of dividing the wrong numbers when calculating probabilities and provides the correct method.
Rounding conventions for expressing probabilities are discussed, with a preference for using three significant digits.
The video concludes with a summary of the lesson on estimating and interpreting probabilities.
Transcripts
Browse More Related Video

Math 119 Chapter 4 Part 1
Chapter 4 Probability Part 1

4.1.0 Basics of Probability - Lesson Overview, Key Concepts and Learning Outcomes

Random variables | Probability and Statistics | Khan Academy

Probability Part 2: Updating Your Beliefs with Bayes: Crash Course Statistics #14
Introduction to Probability, Basic Overview - Sample Space, & Tree Diagrams
5.0 / 5 (0 votes)
Thanks for rating: