4.2.3 Addition and Multiplication Rules - Disjoint Events and Implications for Probability
TLDRThis video script delves into the concept of disjoint or mutually exclusive events in probability theory, illustrating them with Venn diagrams and examples. It clarifies that such events cannot occur simultaneously, affecting the addition rule for calculating probabilities. The script uses scenarios like selecting genders for a clinical trial and rolling dice to explain the principles and their implications on the addition rule, emphasizing the zero probability of overlapping outcomes in disjoint events.
Takeaways
- 📚 The video discusses learning outcome number three from lesson 4.2, focusing on the concept of disjoint or mutually exclusive events.
- 🔍 Two events A and B are considered disjoint or mutually exclusive if they cannot occur simultaneously; there is no overlap in their Venn diagram.
- 🌐 The script provides examples of Venn diagrams to illustrate the concept of disjoint events, with one showing overlap and the other showing no overlap.
- 👤 An example of disjoint events is selecting a male or female for a clinical trial, as a person cannot be both genders in the traditional binary sense.
- 👩🎓 Another example contrasts disjoint events with non-disjoint events, such as selecting someone for a statistics course versus selecting a female, which can overlap.
- 🎲 The script uses the example of rolling a die to explain disjoint events, where rolling a number less than three and rolling a four have no outcomes in common.
- 🃏 It also discusses the example of choosing a jack or a heart from a deck of cards, where the jack of hearts represents an overlap, indicating non-disjoint events.
- ➕ The addition rule for probabilities is simplified for disjoint events, where the probability of A or B occurring is the sum of their individual probabilities without needing to subtract any overlap.
- ⚖️ The probability of non-disjoint events requires the traditional addition rule, which accounts for the overlap to avoid double-counting.
- 🧩 The script concludes with an example calculation of the probability of rolling a number less than three or a four on a die, demonstrating the application of the addition rule for disjoint events.
- 🔑 The takeaway emphasizes the importance of understanding whether events are disjoint to correctly apply the addition rule for calculating probabilities.
Q & A
What is the definition of mutually exclusive events?
-Mutually exclusive events, also known as disjoint events, are events that cannot occur at the same time. In a Venn diagram, there is no overlap between the sets representing these events.
How can you visually represent the concept of disjoint events using a Venn diagram?
-In a Venn diagram, disjoint events are represented by two separate circles that do not overlap. Each circle represents the set of outcomes for each event, and the lack of an overlapping section indicates that the events cannot happen simultaneously.
What is an example of mutually exclusive events in the context of gender selection?
-An example of mutually exclusive events is selecting someone for a clinical trial who is either male or female. Since an individual cannot be both male and female, these two events are mutually exclusive.
Why are the events of selecting someone taking a statistics course and selecting a female not mutually exclusive?
-These events are not mutually exclusive because there can be individuals who are both taking a statistics course and are female. The overlap of these two groups (female students taking statistics) means the events share common outcomes.
How does the outcome of rolling a die illustrate the concept of disjoint events?
-When rolling a die, the event of rolling a number less than three (1 or 2) and the event of rolling a four are disjoint because there is no outcome that satisfies both events simultaneously. The outcomes are 1, 2 for the first event and 4 for the second, with no overlap.
What is the relationship between choosing a jack from a deck of cards and choosing a heart?
-Choosing a jack and choosing a heart are not mutually exclusive events because there is a jack of hearts, which satisfies both conditions. The jack of hearts is part of the set of jacks and also part of the set of hearts, indicating an overlap.
What is the addition rule for calculating the probability of disjoint events?
-For disjoint events, the addition rule simplifies to adding the probabilities of each event. Since there is no overlap, the probability of either event A or event B occurring is the sum of their individual probabilities.
Why is there no need to subtract the probability of the intersection when calculating the probability of disjoint events?
-There is no need to subtract the probability of the intersection for disjoint events because there is no intersection; the events cannot occur at the same time, so there are no outcomes that belong to both sets.
How does the script use the example of rolling a die to explain the probability of disjoint events?
-The script uses the example of rolling a die where event A is rolling a number less than three and event B is rolling a four. Since these events are disjoint (no overlap), the probability of either event occurring is calculated by adding the probabilities of rolling a 1 or 2 (2/6) and rolling a 4 (1/6), resulting in a total probability of 3/6 or 1/2.
What is the implication of the addition rule for non-disjoint events?
-For non-disjoint events, the traditional addition rule must be used, which involves counting all outcomes in both events and then subtracting the outcomes that are counted twice (the intersection) before dividing by the total number of possible outcomes.
Outlines
📚 Understanding Disjoint and Mutually Exclusive Events
This paragraph introduces the concept of disjoint or mutually exclusive events in probability theory. It explains that two events, A and B, are considered disjoint if they cannot occur simultaneously, which is visually represented by a Venn diagram with no overlapping sections. The script provides examples to illustrate the concept, such as selecting a male or female for a clinical trial, which are disjoint due to the exclusivity of gender in the example. Another example contrasts this with selecting a student in a statistics course and a female, which are not disjoint because there can be an overlap, i.e., a female student in the statistics course. The paragraph also discusses the implications for the addition rule in probability when events are mutually exclusive, emphasizing that the probability of both events occurring together is zero, and the total probability is the sum of their individual probabilities without the need to subtract any overlap.
🎲 Consequences of Disjoint Events on Probability Calculations
The second paragraph delves into the consequences of disjoint events on the addition rule in probability. It clarifies that if events A and B are disjoint, the probability of their simultaneous occurrence is zero, simplifying the addition rule to the direct sum of their individual probabilities. The script uses the example of rolling a die to explain this concept, where rolling a number less than three and rolling a four are disjoint events. It demonstrates the calculation of the probability of either event occurring by adding the probabilities of rolling a one or a two (for event A) to the probability of rolling a four (for event B), resulting in a total probability of one-half or 1/2. The paragraph concludes by mentioning that this simplified addition rule is specific to disjoint events and that a traditional addition rule, which accounts for overlaps, is used for non-disjoint events.
Mindmap
Keywords
💡Disjoint Events
💡Mutually Exclusive
💡Venn Diagram
💡Addition Rule
💡Sample Space
💡Probability
💡Clinical Trial
💡Statistics Course
💡Deck of Cards
💡Complementary Events
Highlights
Definition of disjoint or mutually exclusive events is introduced, where two events cannot occur simultaneously.
Explanation of the Venn diagram concept, showing no overlap between two disjoint events.
Examples provided to illustrate the concept of non-disjoint events with overlapping possibilities.
Mutual exclusivity and disjoint events are synonymous, both implying no simultaneous occurrence.
Example of disjoint events: selecting a male or female for a clinical trial, based on traditional gender binary.
Example of non-disjoint events: selecting a person taking a statistics course and being female, as they can co-occur.
Rolling a die to demonstrate disjoint events: rolling less than three versus rolling a four, with no overlap.
Choosing a jack or a heart from a deck of cards as an example of non-disjoint events due to the jack of hearts.
Consequences for the addition rule when events are mutually exclusive, simplifying the probability calculation.
The addition rule for disjoint events simplifies to adding probabilities of individual events without overlap.
Traditional addition rule requires careful counting to avoid double-counting in non-disjoint events.
Application of the addition rule for disjoint events in calculating the probability of rolling a number less than three or a four on a die.
Explanation of how to calculate probabilities using the sample space and outcomes for disjoint events.
Final probability calculation example results in one half for the given disjoint events.
Discussion of the implications for the addition rule in the context of disjoint and mutually exclusive events.
Introduction of the next video topic, which will cover the rule of complementary events.
Transcripts
Browse More Related Video
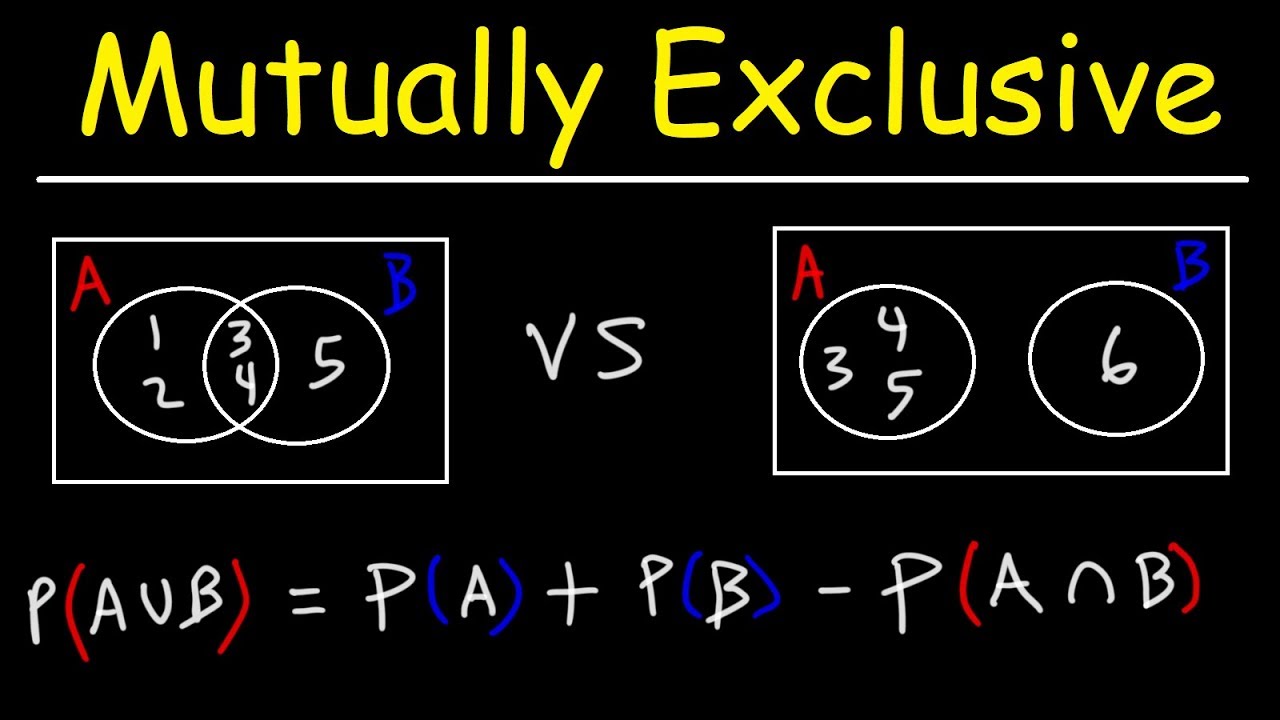
Probability of Mutually Exclusive Events With Venn Diagrams

Chapter 4 Probability Part 2
4.2.1 Addition and Multiplication Rules - Simple and Compound Events, and Their Probabilities

AP Stats Test Quick Review: Probability
"Mutually Exclusive" and "Independent" Events (...are VERY different things!)

Math 119 Chapter 4 Part 1
5.0 / 5 (0 votes)
Thanks for rating: