The Fundamental Theorem of Calculus: Redefining Integration

Professor Dave Explains
23 Apr 201809:37
EducationalLearning
32 Likes 10 Comments

TLDRThe script explains the fundamental theorem of calculus, which connects differentiation and integration as inverse mathematical operations. It introduces the notation and formal definition of integrals and antiderivatives. Through examples, it shows that finding the antiderivative undoes differentiation, allowing integration to be computed as evaluating antiderivatives over an interval rather than summing infinitesimal slices. This important realization enabled integral calculus to develop alongside differential calculus. The script concludes by formally stating the fundamental theorem, providing the foundation for applying integrals to evaluate areas under curves.

Takeaways
  • 😀 The fundamental theorem of calculus establishes the inverse relationship between differentiation and integration.
  • 😯 The integral sign ∫ represents the limit of sums of rectangles under a curve. The function f(x) is the integrand.
  • 🤓 To find an antiderivative F, reverse differentiate: increase the exponent by 1 and divide by the new exponent.
  • 😮 Evaluating a definite integral means evaluating the antiderivative at the upper limit minus the lower limit.
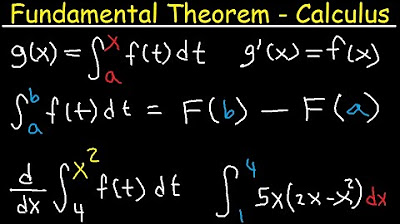
  • 🧐 The function g(x) represents the area under the curve up to x. Its derivative is the original function f(x).
  • 🤯 Differentiation and integration are inverse operations, like addition/subtraction or multiplication/division.
  • 👍🏻 The second part of the theorem gives the algorithm to evaluate integrals using antiderivatives.
  • 😊Integration is no longer about summing tiny rectangles, but evaluated antiderivatives.
  • 🙂 The function g(x) can represent the 'area so far' as x moves along the curve.
  • 😃 We can now evaluate definite integrals using antiderivatives rather than rectangles.
Q & A

  • What is the fundamental theorem of calculus?

    -The fundamental theorem of calculus establishes the integral and derivative as inverse operations, analogous to addition/subtraction or multiplication/division. It shows that differentiation and integration are connected.

  • What does the integral sign represent?

    -The integral sign represents the limit of a sum of rectangles used to find the area under a curve, introduced by Leibniz. It looks like a long S.

  • What is an antiderivative?

    -An antiderivative is a function that when differentiated gives you back the original function, similar to how squaring a square root gives you what's inside. F is the antiderivative of f if F' = f.

  • How do you compute an antiderivative?

    -To compute an antiderivative, use the opposite process of differentiation - increase the exponent by 1 and divide by the new exponent. For example, the antiderivative of x^2 is x^3/3.

  • What does it mean to evaluate a definite integral?

    -Evaluating a definite integral means to evaluate the antiderivative function at the upper limit of integration and subtract its evaluation at the lower limit. This gives the area under the curve over that interval.

  • What is the second part of the fundamental theorem?

    -The second part gives the algorithm to compute integrals - the integral from a to b of f(x) dx equals F(b) - F(a), where F is the antiderivative of f.

  • What is an indefinite integral?

    -An indefinite integral leaves the upper limit as a variable x rather than a number. This gives a function g(x) that represents the area under the curve up to that point, or the 'area so far'.

  • What is the significance of dx in an integral?

    -dx is required after the integrand function f(x) for the integral to make sense, but it has no independent meaning. It represents an infinitesimal difference.

  • Who are credited with developing integral calculus?

    -Isaac Newton and Gottfried Leibniz independently developed the foundations of integral calculus in the 17th century.

  • How did the fundamental theorem allow better understanding of integration?

    -The fundamental theorem showed integration to be the inverse of differentiation rather than an obsolete method of rectangles, allowing more computational approaches.

Outlines
00:00
😊 Introduction to the Fundamental Theorem of Calculus

Paragraph 1 introduces the fundamental theorem of calculus, which links differentiation and integration. It explains what integration is conceptually, in terms of finding the area under a curve. It then introduces the notation for definite integrals using the integral sign, limits of integration, the infinitesimal dx, and the concept of an antiderivative F. It shows how to compute integrals using antiderivatives over an interval from a to b. An example with the function x^2 from 0 to 1 is provided, giving a value of 1/3 which matches the geometrical understanding of area under a curve using rectangles.

05:05
😲 Integration and Differentiation as Inverse Operations

Paragraph 2 explains that differentiation and integration are inverse operations, just like addition/subtraction or multiplication/division. This inverse relationship is the essential truth of the fundamental theorem of calculus. It formally states the theorem for a continuous function f(t) integrated from a to x to give a function g(x). Taking the derivative of g(x) gives back f(x). The second part of the theorem is the integral evaluation rule using antiderivatives. This new understanding united integral and differential calculus.

Mindmap
Keywords
💡integral
An integral sign represents the limit of a sum of rectangles used to calculate the area under a curve. It is introduced as a new notation that is equivalent to the previous sum notation but more concise. Integrals represent the process of integration.
💡integration
Integration is the process of finding the area under a curve by adding up rectangles. It is the inverse operation of differentiation. The fundamental theorem of calculus shows that integration and differentiation are connected.
💡fundamental theorem of calculus
This theorem states that differentiation and integration are inverse operations, just like addition/subtraction or multiplication/division. It connects differential calculus and integral calculus. It shows that the derivative of the integral of a function is the original function.
💡antiderivative
The antiderivative of a function f(x) is another function F(x) such that the derivative of F(x) gives back f(x). So F(x) is the integral of f(x). This concept is key to evaluating definite integrals using limits of integration.
💡differentiation
Differentiation is the process of finding the derivative of a function, by bringing exponents down and reducing them by 1. Differentiation and integration are inverse operations.
💡continuity
For the fundamental theorem to hold, the function being integrated must be continuous over the interval of integration. Continuity means having no gaps or jumps.
💡limits of integration
The upper and lower numbers marked beside the integral sign denote the start and end points for the region of integration under the curve. This specifies the definite interval to integrate over.
💡definite integral
A definite integral evaluates the integral over a defined interval, from a lower limit to an upper limit. This gives a number value, representing the area under the curve over that interval.
💡indefinite integral
An indefinite integral contains variables for the limits of integration. This results in a function expressing the area under a curve up to a variable limit. The fundamental theorem connects definite and indefinite integrals.
💡inverse operations
Inverse operations are pairs of operations that “undo” each other, like addition/subtraction or multiplication/division. The fundamental theorem of calculus states that differentiation and integration are also inverse operations.
Highlights

Integration can be thought of as finding the area under a curve

The integral sign and dx notation were introduced to represent integration

An antiderivative is a function that gives the original function when differentiated

To find an antiderivative, reverse the differentiation process

Evaluating definite integrals involves evaluating antiderivatives over an interval

Differentiation and integration are inverse operations

The fundamental theorem of calculus connects differentiation and integration

Integration can be defined as an algorithm rather than approximating areas

The derivative of the integral of a function is that original function

The integral of f(x) equals the antiderivative F evaluated over the interval

We can think of the integral as the area so far under a curve

The fundamental theorem allows evaluating integrals without rectangles

We can now evaluate definite integrals using antiderivatives

The integral sign represents the limit of a sum of areas

Integration links differentiation and anti-differentiation

Transcripts

Browse More Related Video

Properties of Integrals and Evaluating Definite Integrals

Why Are Slope and Area Opposite: The Fundamental Theorem of Calculus

Calculus - Lesson 15 | Relation between Differentiation and Integration | Don't Memorise

Definite Integrals!

Business Calculus - Math 1329 - Section 5.3 - The Definite Integral and Fundamental Thm of Calculus

Fundamental Thereom of Calculus Explained - Part 1 & 2 Examples - Definite Integral

Rate This

5.0 / 5 (0 votes)

Thanks for rating: