Properties of Integrals and Evaluating Definite Integrals

Professor Dave Explains
25 Apr 201809:47
EducationalLearning
32 Likes 10 Comments

TLDRThe video explains key properties of integrals and walks through examples evaluating definite integrals of basic polynomial functions. It first highlights properties like switching integration limits, integrating over the same limit twice, integrating over adjacent intervals, pulling out constants, and integrating sums and differences of functions. It then evaluates antiderivatives, setting up integrals, plugging in limits of integration, and calculating areas under curves over specific intervals. The video emphasizes how integration builds on differentiation as an inverse operation and fundamental theorem of calculus to allow simpler calculation of areas and distances related to complex curvature.

Takeaways
  • 😀 Integration requires taking the antiderivative of a function
  • 😇 If we switch the limits of an integral, it changes sign but has the same magnitude
  • 🧐 An integral over the same limit equals zero
  • 🤓 Integrating a sum equals the sum of integrals over the same region
  • 👍🏻 Constants can be pulled out of integrals
  • 💡 Positive areas under a curve yield positive integrals, negative areas yield negative integrals
  • 📝 Simple polynomials can be integrated by finding their antiderivatives
  • 😮 Fractions inside integrals can be divided out
  • 🤯 Any integer exponent adds 1 upon antidifferentiating
  • ✅ Prior to calculus, finding areas under curves was only for brilliant mathematicians
Q & A

  • What is the relationship between differentiation and integration?

    -Differentiation and integration are inverse operations. Integration requires taking the antiderivative of a function.

  • What happens when you switch the limits of integration of a definite integral?

    -When you switch the limits of integration of a definite integral, you get the same integral but with the opposite sign. This is because you are integrating in the opposite direction.

  • When will a definite integral equal zero?

    -A definite integral will equal zero when the upper and lower limits of integration are the same number. This represents the area under a single point, which has no area.

  • How can you calculate the integral of a constant function?

    -To calculate the integral of a constant function c, take c times the difference between the upper and lower limits of integration. For example, the integral of 3 from 1 to 5 is 3*(5-1) = 12.

  • How do you calculate an integral when part of the function is above the x-axis and part is below?

    -Take the integral of the part above the x-axis and subtract the integral of the part below the x-axis. The result will be the net area between the function and the x-axis.

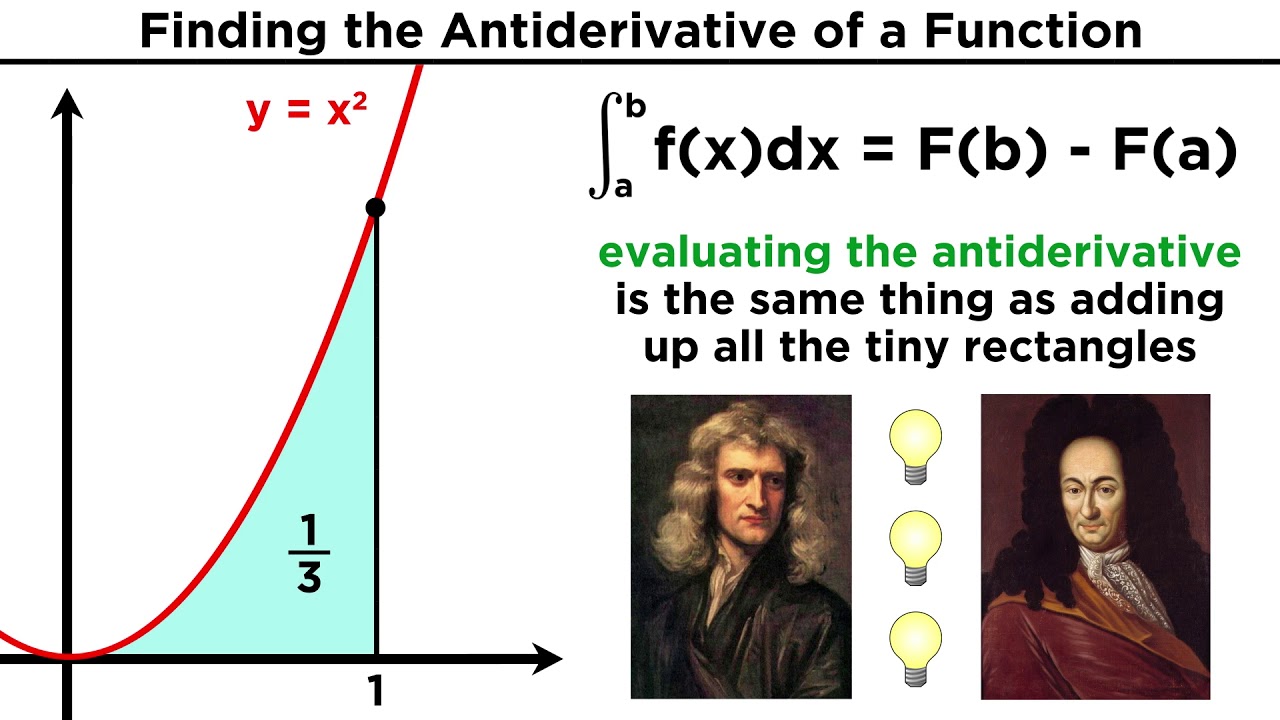
  • What is the fundamental theorem of calculus?

    -The fundamental theorem of calculus states that differentiation and integration are inverse operations. It connects differentiation and integration allowing the calculation of integrals by using antiderivatives.

  • What are some basic properties of integrals?

    -Basic properties of integrals include: 1) Switching limits changes the sign, 2) Equal limits give zero, 3) Breaking integrals into pieces and summing, 4) Pulling out constants, 5) Summing integrals of constituent functions.

  • What is an easy way to integrate a polynomial function?

    -To integrate a polynomial function, take the antiderivative by adding 1 to each exponent, dividing by the new exponent, and substituting in the limits of integration.

  • Why is integration important in mathematics?

    -Integration allows the calculation of areas, volumes, central points and many important mathematical quantities. It is a fundamental tool in mathematics and physics.

  • What are some strategies for evaluating more complex integrals?

    -Strategies for complex integrals include: integration by parts, trigonometric substitution, partial fractions, and numerical integration.

Outlines
00:00
📝 Overview of Integration Properties and Simple Examples

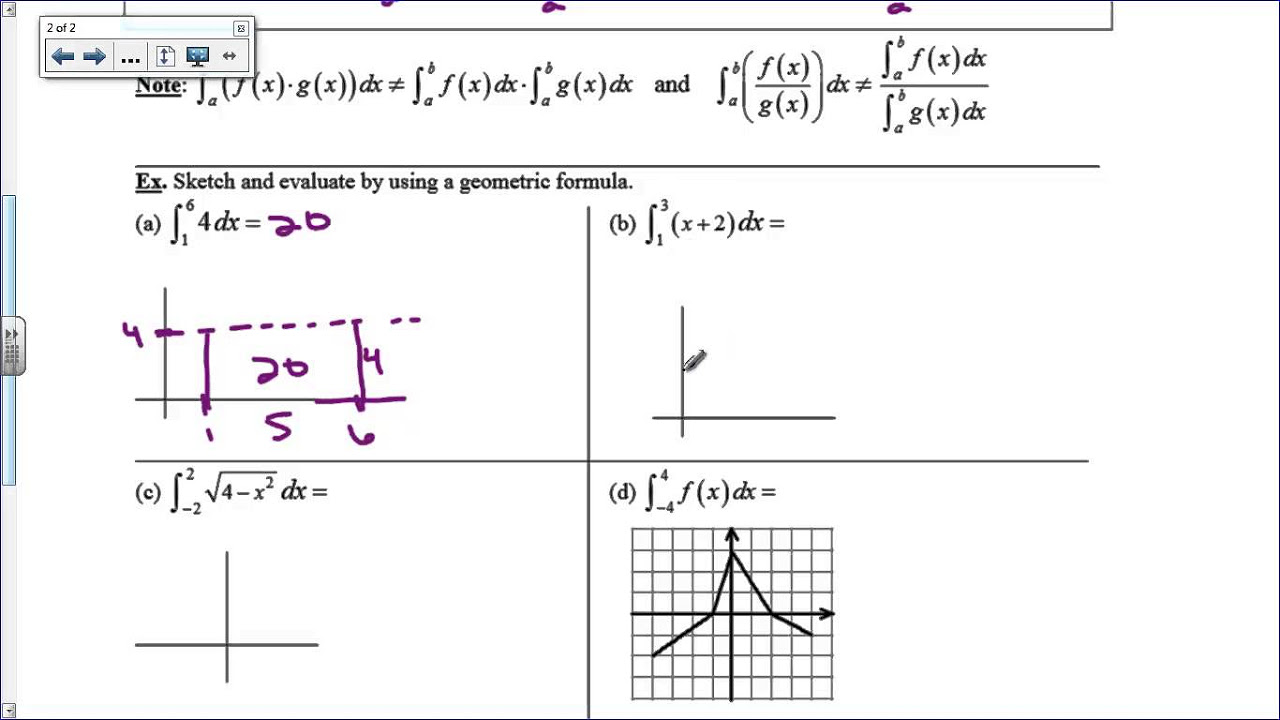
This paragraph provides an overview of some key properties of integrals, including reversing the limits of integration, integrating over the same limit equals zero, summing adjacent integrals, pulling constants out of integrals, positive/negative areas, etc. It then works through some simple examples of integrating polynomials by taking antiderivatives and evaluating at the limits.

05:01
😊 More Challenging Integral Examples

This paragraph provides some additional examples of evaluating definite integrals, involving square roots, fractions/exponents, etc. It highlights how integration allows easily finding areas that would have been very complex prior to the fundamental theorem of calculus. It ends by noting that integration will become more difficult but first checks comprehension of the concepts covered.

Mindmap
Keywords
💡integral
An integral represents the area under a curve on a graph. Integrals allow you to find the area between a function and the x-axis over a specific interval. In the video, integrals are presented as the inverse operation of differentiation, allowing you to go backwards from the derivative to the original function.
💡antiderivative
The antiderivative is the inverse function of the derivative, obtained by integrating. For example, the antiderivative of 2x is x^2. In the context of integrals, you first take the antiderivative of a function and then evaluate it between limits to find areas under curves.
💡limits of integration
The limits of integration define the interval over which you are integrating or finding the area under a curve. The video explains various properties when changing or combining limits of integration.
💡fundamental theorem of calculus
This important theorem connects differentiation and integration, showing that integration is the inverse process of differentiation. It provides the foundation for setting up and evaluating definite integrals.
💡polynomial
A polynomial is a function consisting of terms with increasing integer powers, like 2x^2 + x + 1. The video starts by evaluating integrals of basic polynomial functions, starting with quadratic and cubic polynomials.
💡exponent
An exponent indicates repeated multiplication, such as x^2 = x*x. The video shows techniques for taking integrals using rules of exponents to simplify functions.
💡area under a curve
A major application of integrals is to find the area between a curve and the x-axis over an interval. The video explains how this area calculation connects to evaluating integrals.
💡antiderivative
The antiderivative is the inverse function of the derivative, obtained by integrating. For example, the antiderivative of 2x is x^2. In the context of integrals, you first take the antiderivative of a function and then evaluate it between limits to find areas under curves.
💡algorithm
The video refers to the process of evaluating integrals as a simple algorithm - following the mathematical steps systematically allows even beginners to evaluate integrals and find areas under curves.
💡curvature
Curvature refers to how much a geometric object deviates from being flat, for example the curvature of a circle. Prior to calculus, analyzing curved shapes was very difficult. The fundamental theorem of calculus provided a breakthrough.
Highlights

Integration requires taking the antiderivative of a function.

For basic functions, this is easy to do, but it gets extremely complicated as the functions get more complex.

If we switch the limits of integration, this will be the same as the first integral but negative.

If both of the limits of integration are the same number, the integral will be equal to zero.

The sum of these integrals is equal to the integral of the function over the whole interval.

If the interval of a function that is being integrated is above the x-axis, its integral will be positive.

Let's integrate simple polynomials. This involves taking the antiderivative of a function.

Prior to the fundamental theorem of calculus, finding areas and distances associated with curvature was incredibly complex.

Now with this simple algorithm of finding the antiderivative, anyone can do it.

Integration will get much harder than this, but before moving forward, let’s check comprehension.

If we are taking the integral of a constant times some function, we can just pull the constant out of the integral.

The integral of a sum of functions over some interval is equal to the sum of their integrals over the same interval.

Remember we are still just adding one to the exponent to get x to the negative one, and then we divide by that exponent.

Hopefully after computing some simple integrals, we can see the immense power of this method.

Now don't get too cocky, integration will get much harder than this.

Transcripts

Browse More Related Video

The Fundamental Theorem of Calculus: Redefining Integration

Definite Integral Calculus Examples, Integration - Basic Introduction, Practice Problems

Calculus AB Unit 6 Review

Riemann Sums and Definite Integrals

Evaluating Indefinite Integrals

Business Calculus - Math 1329 - Section 5.3 - The Definite Integral and Fundamental Thm of Calculus

Rate This

5.0 / 5 (0 votes)

Thanks for rating: