Calculus - Lesson 15 | Relation between Differentiation and Integration | Don't Memorise
TLDRThis educational video explores the interconnected nature of differentiation and integration in calculus. It begins by explaining the derivative as the instantaneous rate of change and the integral as the area under a curve. The video then illustrates how these concepts are inversely related, using the example of a car's distance traveled over time. The main advantage discussed is the simplification of finding integrals through the relationship between the two processes. The script introduces the Fundamental Theorem of Calculus, which states that the integral of a derivative returns the original function, allowing for easier computation of integrals by evaluating the antiderivative at the limits of integration. The video concludes by encouraging viewers to find the antiderivative of a given function to utilize this theorem effectively.
Takeaways
- 📚 Differentiation and integration are two fundamental concepts in calculus, with differentiation providing the rate of change and integration providing the area under a curve.
- 🔄 The process of differentiation and integration are inverse operations, with one being the reverse of the other.
- 🚗 The script uses a car's motion as an example to illustrate the relationship between distance traveled (an integral concept) and speed (a derivative concept).
- 📈 The derivative of a distance function (like t^2) gives the instantaneous speed of the car at any time 'T', which is 2t in this case.
- 📉 The integral of the speed function (like 2t) can be found by considering the area under the curve, which in the example forms a triangle.
- 🧩 The area under the speed function's graph represents the total distance traveled by the car, showing the direct link between the integral and the original distance function.
- ♻️ Integration of the derivative of a function returns the original function, demonstrating the reversibility of the two operations.
- 📚 The Fundamental Theorem of Calculus is introduced, which states that the integral of a derivative function is the original function evaluated at the limits of integration.
- 🔢 This theorem simplifies the process of integration, as it allows finding the integral by simply evaluating the antiderivative at the integration limits.
- 🔑 An antiderivative, or indefinite integral, of a function is a function whose derivative is the original function, and it's crucial for applying the Fundamental Theorem of Calculus.
- 🔍 The script encourages viewers to think about and find the antiderivative of a given function, fostering engagement and deeper understanding.
Q & A
What is the relationship between differentiation and integration?
-Differentiation and integration are related as opposite processes in calculus. Differentiation finds the instantaneous rate of change of a function, while integration calculates the area under the curve of a function. They are connected such that the integral of the derivative of a function will yield the original function, up to a constant for indefinite integrals.
How is the derivative of a function related to the instantaneous rate of change?
-The derivative of a function at a specific point represents the instantaneous rate of change of that function at that point. It tells us how quickly the function's value is changing at that particular moment in time.
What does the integral of a function represent?
-The integral of a function represents the area under the curve of the function. It can also be interpreted as the accumulated quantity resulting from the variable of integration over a given interval.
Why is finding the integral of a function by dividing the area into rectangles and taking the limit cumbersome?
-This method is cumbersome because it involves a multi-step process of approximating the area under the curve with rectangles, summing these areas, and then taking the limit as the width of the rectangles approaches zero, which can be complex and time-consuming.
How does the concept of the Fundamental Theorem of Calculus simplify the process of finding integrals?
-The Fundamental Theorem of Calculus allows us to find the integral of a function by simply evaluating the antiderivative (or indefinite integral) of the function at the limits of integration and taking the difference, rather than having to perform the limit process of summing areas.
What is the function of time 'Y' in the context of the car traveling on a road?
-In the context of the car traveling on a road, 'Y' represents the distance traveled by the car as a function of time 'T'. It is used to model how far the car has gone at any given time.
Can you explain the example given in the script about the car's speed and distance traveled?
-The example illustrates that if the distance traveled by the car is given by the function Y = t^2, then the speed of the car at any instant 'T' is the derivative of this distance function, which is S = 2T. The integral of the speed function from 0 to T1 gives the distance traveled in time T1, showing the relationship between integration and differentiation.
What is the significance of the area under the speed function graph in the context of the car example?
-The area under the speed function graph represents the distance traveled by the car. This is because the speed function, when integrated over a time interval, gives the total distance covered during that interval.
What is the function 'F of T' mentioned in the script, and how is it related to its derivative 'f prime of T'?
-The function 'F of T' is an antiderivative of the function 'f prime of T'. When you differentiate 'F of T', you get 'f prime of T', and when you integrate 'f prime of T', you get back 'F of T', demonstrating the inverse relationship between differentiation and integration.
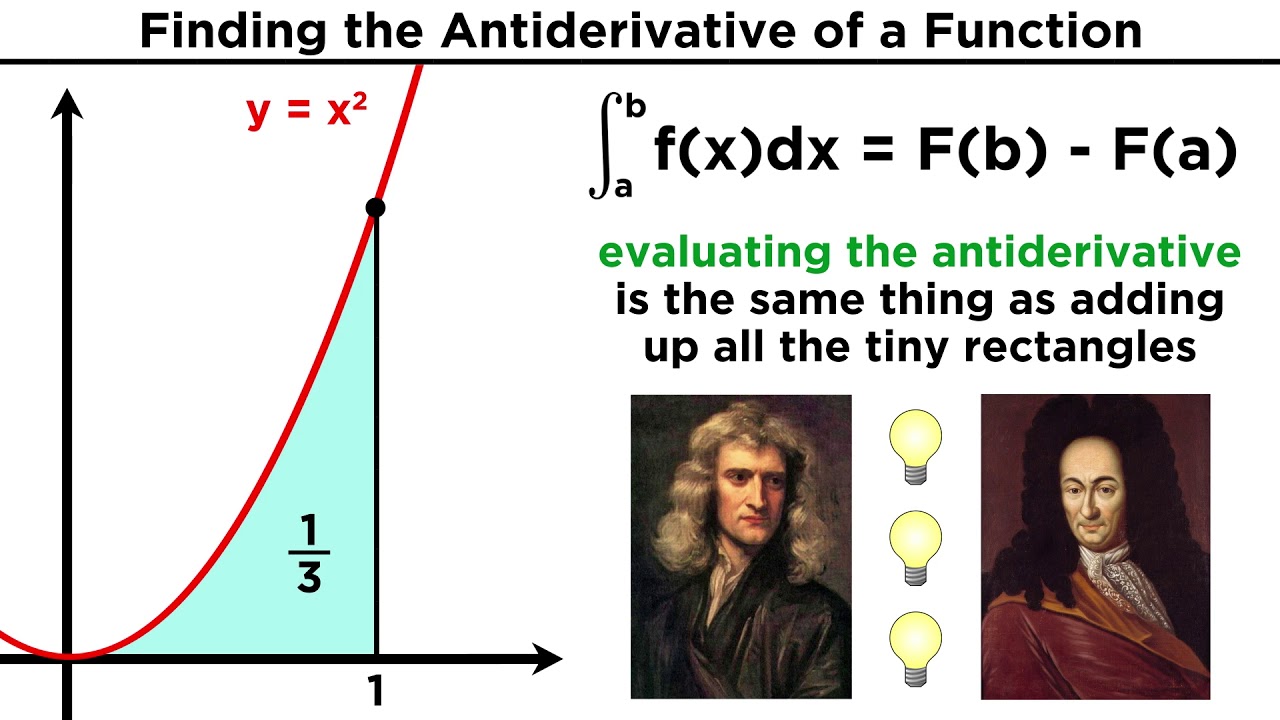
How does the Fundamental Theorem of Calculus apply to the integral of the function '2T' from 'A' to 'B'?
-According to the Fundamental Theorem of Calculus, the integral of the function '2T' from 'A' to 'B' is equal to the antiderivative of '2T' evaluated at 'B' minus the antiderivative evaluated at 'A'. In this case, it would be 'B^2 - A^2' because the antiderivative of '2T' is 'T^2'.
What is the term for a function 'Capital G of X' whose derivative is 'G of X'?
-A function 'Capital G of X' whose derivative is 'G of X' is called the antiderivative or the indefinite integral of the function 'G of X'.
Outlines
📚 Introduction to Differentiation and Integration
The script introduces the fundamental concepts of differentiation and integration in calculus. Differentiation is the process of finding the derivative of a function, which represents the instantaneous rate of change, while integration is the process of finding the area under the curve of a function. The script explains that these two operations, though seemingly distinct, are actually inverses of each other. This relationship simplifies the process of finding integrals, as the integral of a function's derivative can be found by reversing the differentiation process. The script uses the analogy of a car's distance traveled and its speed to illustrate this concept, showing how the derivative of the distance function gives the speed, and integrating the speed function gives back the distance function.
📈 The Fundamental Theorem of Calculus
This paragraph delves into the practical application of the relationship between differentiation and integration, introducing the Fundamental Theorem of Calculus. The theorem states that the integral of a function's derivative is equal to the original function evaluated at the limits of integration, minus the original function evaluated at the lower limit. This allows for the calculation of definite integrals without the need for the traditional area-under-the-curve method. The script provides an example using the function '2T', showing how to find the integral from 'A' to 'B' by evaluating the function at these points and taking the difference. The concept of an anti-derivative or indefinite integral is also introduced, which is a function whose derivative is the original function of interest. The script concludes by encouraging viewers to think about how to find such an anti-derivative for a given function and to stay tuned for future content.
Mindmap
Keywords
💡Differentiation
💡Integration
💡Instantaneous Rate of Change
💡Area Under the Graph
💡Limit
💡Function
💡Derivative
💡Integral
💡Fundamental Theorem of Calculus
💡Anti-derivative
Highlights
Differentiation and integration are fundamental concepts in calculus.
Derivative represents the instantaneous rate of change of a function.
Integral represents the area under the curve of a function.
Differentiation and integration are related and act as opposite processes.
The integral of a function can be found by summing areas of rectangles under the curve.
The process of finding an integral can be lengthy and cumbersome.
The relationship between differentiation and integration simplifies finding integrals.
Example: The distance traveled by a car is a function of time squared.
The speed of the car at any instant is the derivative of the distance function.
The integral of the speed function gives the distance traveled.
The area under the speed graph corresponds to the distance traveled by the car.
Integration and differentiation are inverse operations.
Integration of a derived function returns the original function.
The Fundamental Theorem of Calculus simplifies finding integrals.
The integral of a function's derivative is the original function evaluated at limits.
The Fundamental Theorem of Calculus allows for easy calculation of integrals.
Finding an anti-derivative or indefinite integral is key to applying the theorem.
The next video will explore anti-derivatives and their application.
Transcripts
Browse More Related Video

Why Are Slope and Area Opposite: The Fundamental Theorem of Calculus

Calculus - Lesson 16 | Indefinite and Definite Integrals | Don't Memorise

Derivatives of Integrals (w/ Chain Rule)

An Intuitive Understanding of the Fundamental Theorem of Calculus

Calculus 1 - Integration: Proof of the Fundamental Theorem of Calculus
The Fundamental Theorem of Calculus: Redefining Integration
5.0 / 5 (0 votes)
Thanks for rating: