Video 86 - Theorema Egregium
TLDRThis video delves into the theorema egregium, a fundamental concept in tensor calculus linking the Riemann curvature tensor with the extrinsic curvature. The presenter explains the derivation process, starting with the commutative operation on the shift tensor and leading to the Kodasi equation and the theorema egregium itself. The significance of these relationships is highlighted, emphasizing the intrinsic nature of Gaussian curvature, which can be determined solely from the metric tensor without any extrinsic elements. The video concludes with insights into the implications of these findings for understanding the intrinsic properties of manifolds.
Takeaways
- 📚 The video discusses the development of the 'Theorema Egregium', a fundamental theorem in tensor calculus that connects the Riemann curvature tensor with the extrinsic curvature tensor.
- 🔍 The commutative operation is applied to the shift tensor, leading to an expanded expression that is crucial for deriving the theorema egregium.
- 📉 The script explains the process of using the covariant derivative and the shift tensor to establish relationships that are essential for the theorem.
- 📌 The video references previous content, such as the normal component of the curvature tensor and its relation to the shift tensor, which is vital for understanding the derivation.
- 🔗 The script introduces the Kodama equation, which is derived from the normal projection of the commutative operation on the shift tensor.
- 📐 The Theorema Egregium is highlighted as a remarkable theorem because it shows that the Riemann curvature tensor, an intrinsic object, can be related to extrinsic curvature information.
- 🌐 The theorem demonstrates that the curvature of a manifold is an intrinsic property and can be determined solely from the metric tensor, without reference to the manifold's embedding in space.
- 📈 The Gaussian curvature is identified as an invariant scalar expression, which is derived from the determinant of the curvature tensor with mixed indices.
- 📝 The video concludes with the derivation of tensor expressions that are more suitable for use in tensor calculus, emphasizing the intrinsic nature of curvature.
- 🧠 The script emphasizes the importance of understanding that while the Riemann tensor can be determined from the metric tensor, the converse is not true, indicating the existence of isometric manifolds with different embeddings but the same intrinsic curvature.
- 🌀 The video uses the analogy of a contact lens to illustrate how the intrinsic curvature of a surface does not change with its shape, as long as the metric tensor remains unchanged.
Q & A
What is the main focus of video number 86 in the series on tensor calculus?
-The main focus of the video is to develop the theorema egregium, which establishes the connection between the Riemann tensor and the extrinsic curvature tensor.
What is the significance of the theorema egregium in the context of the video?
-The theorema egregium is significant because it connects the extrinsic curvature information with the intrinsic curvature information of a manifold, showing that the Riemann tensor carries information about the curvature of the manifold.
What does the commutative operation on the shift tensor lead to in the video?
-The commutative operation on the shift tensor leads to the expansion of the operation and the development of terms involving the Riemann tensor and the extrinsic curvature tensor.
What does the term 'surface index omega' refer to in the video script?
-In the script, 'surface index omega' refers to the index used in tensor operations that is specific to the surface being analyzed, as opposed to an ambient index that pertains to the surrounding space.
How does the video script describe the relationship between the covariant derivative of the shift tensor and the curvature tensor?
-The script describes the relationship by stating that the covariant derivative with respect to beta of the shift tensor is equal to the normal component times the curvature tensor with the appropriate indexes.
What is the Khadasi equation mentioned in the video, and what is its significance?
-The Khadasi equation is a result derived from the normal projection of the commutative operation on the shift tensor. Its significance lies in the fact that it is symmetric with respect to the first two indexes, indicating a total symmetry in the expression.
What does the term 'Weingarten's formula' refer to in the context of the video?
-In the video, 'Weingarten's formula' refers to the relationship that the covariant derivative of the normal component is equal to the negative value of the shift tensor contracted with the curvature tensor.
How does the video script explain the intrinsic nature of the Gaussian curvature?
-The script explains that the Gaussian curvature is an intrinsic property because it can be calculated directly from the metric tensor without any reference to extrinsic elements, proving that it is an intrinsic characteristic of the manifold.
What is the relationship between the determinant of the curvature tensor and the Gaussian curvature as explained in the video?
-The video explains that the determinant of the curvature tensor with both indexes in the lower position is equal to the Gaussian curvature, showing that the Gaussian curvature is an invariant expression derived from the curvature tensor.
How does the video script connect the extrinsic curvature tensor with the intrinsic curvature tensor through the theorema egregium?
-The script connects the two by showing that the Riemann tensor, which is an intrinsic object derived from the metric tensor, is equal to an expression involving the extrinsic curvature tensor, thus linking the curvature information from both perspectives.
What are the implications of the theorema egregium for the understanding of isometric manifolds?
-The theorema egregium implies that different surfaces can have the same metric tensor and therefore the same intrinsic curvature, even if they are not embedded or curved in the same way. This is the concept of isometric manifolds.
What did Gauss himself say about the theorema egregium, as quoted in the video?
-Gauss stated that if a curved surface is developed on any other surface, the measure of curvature at each point remains unchanged, highlighting the intrinsic nature of curvature as described by the theorema egregium.
Outlines
📚 Introduction to Theorema Egregium
This paragraph introduces the topic of Theorema Egregium, a fundamental concept in tensor calculus that establishes a relationship between the Riemann curvature tensor and the extrinsic curvature tensor. The explanation begins with the application of the commutative operation to the shift tensor and expands on the meaning of this operation. The process involves using the rules from a previous video, identifying the need for a separate term for each surface index, and recognizing the negative term due to the position of the index omega. The paragraph also revisits an expression from video 74, which relates the covariant derivative of the shift tensor to the normal component and the curvature tensor, and demonstrates how to replace and rearrange terms to simplify the expression using the product rule.
🔍 Deriving the Kodama Equation and Theorema Egregium
The second paragraph continues the exploration of tensor calculus by focusing on finding the normal and surface projections of the equation derived in the previous paragraph. The normal projection involves contracting both sides of the equation with the normal vector, leading to the Kodama equation. This equation is significant as it is symmetric with respect to the first two indices, indicating that the expression is invariant under the swap of these indices. The paragraph also discusses the surface projection, which is achieved by contracting both sides with the inverse shift tensor. This process results in the Theorema Egregium, a remarkable theorem that connects extrinsic curvature information with intrinsic curvature, demonstrating that the Riemann tensor carries information about the manifold's curvature.
🌐 The Significance of Theorema Egregium
This paragraph delves into the significance of the Theorema Egregium, highlighting its importance in understanding the curvature of a manifold. The theorem shows that the Riemann tensor, an intrinsic object derived from the metric tensor, carries information about the curvature of the manifold. It contrasts this with the extrinsic curvature tensor, which is derived from the embedding of the manifold in the ambient space. The discussion then narrows down to two dimensions, examining a specific component of the Riemann tensor and relating it to the determinant of a 2x2 matrix, which is a scalar expression of the curvature tensor. The paragraph concludes by emphasizing that the Gaussian curvature, derived from the Riemann tensor, is an intrinsic property of the manifold.
📘 Gaussian Curvature and Intrinsic Properties
The fourth paragraph further explains the intrinsic nature of Gaussian curvature, which can be computed directly from the metric tensor without any reference to extrinsic elements. It discusses how the Gaussian curvature is an invariant scalar expression and relates it to the determinant of the curvature tensor with mixed indices. The paragraph also introduces the concept of isometric manifolds, which have the same metric tensor but different embeddings, such as a plane and a cylinder. It uses the analogy of a contact lens to illustrate that bending a surface does not change its metric tensor or Gaussian curvature, emphasizing the theorem's remarkable property that curvature is an intrinsic measure.
🔗 Tensor Calculus and Gaussian Curvature Expressions
This paragraph builds upon the previous discussions to derive additional relationships suitable for tensor calculus. It starts by simplifying the expression for the Riemann tensor in two dimensions and then generalizes it to eliminate hard-coded references, making it more adaptable for tensor calculus. The paragraph introduces the concept of Levi-Civita symbols and their relationship with the Riemann tensor, leading to an expression that relates the Gaussian curvature to the determinant of the curvature tensor with both indices in the upper and lower positions. It concludes by discussing the symmetries of the Riemann tensor and presenting a tensor expression that captures the intrinsic curvature information.
🏁 Recap and Final Thoughts on Theorema Egregium
The final paragraph provides a recap of the video's main points, summarizing the key takeaways from the exploration of tensor calculus and the Theorema Egregium. It reiterates the significance of the Kodama equation and the Theorema Egregium, emphasizing the intrinsic nature of Gaussian curvature and its derivation from the Riemann tensor. The paragraph also reflects on the implications of the theorem, noting that while the Riemann tensor provides information about the curvature, it does not lead to a unique solution regarding the surface's embedding in the ambient space. It concludes with a quote from Gauss, highlighting the theorem's most remarkable aspect: the invariance of curvature under surface development.
Mindmap
Keywords
💡Theorema Egregium
💡Riemann Tensor
💡Extrinsic Curvature Tensor
💡Covariant Derivative
💡Shift Tensor
💡Curvature Tensor
💡Kodasi Equation
💡Gaussian Curvature
💡Levi-Civita Symbol
💡Metric Tensor
💡Tensor Calculus
Highlights
Introduction to the development of the theorema egregium, a key relationship between the Riemann tensor and the extrinsic curvature tensor.
Application of the commutative operation to the shift tensor to expand the full meaning of the operation.
Explanation of the need for a separate term for each surface index and the significance of the negative term due to the lower position of omega.
Utilization of the covariant derivative of the shift tensor in relation to the normal component and curvature tensor.
Derivation of the expression involving the covariant derivative and the normal component using the results from video number 74.
Application of the product rule to expand the product of terms in the equation.
Introduction of the Kodasi equation, highlighting its symmetry with respect to the first two indexes.
Discussion on the significance of the Kodasi equation and its implications for the curvature tensor.
Exploration of the normal and surface projections of the equation to find important relationships.
Use of Weingarten's formula to replace expressions in the equation with alternative forms.
Derivation of the theorema egregium, emphasizing its remarkable nature in connecting extrinsic and intrinsic curvature information.
Explanation of the theorema egregium's significance in showing that the Riemann tensor carries information about the manifold's curvature.
Demonstration of how the Gaussian curvature can be computed directly from the metric tensor, proving it as an intrinsic property.
Derivation of additional relationships suitable for tensor calculus equations from the curvature tensor values.
Introduction of the expression that captures the relationship between the Riemann tensor and the Gaussian curvature in two dimensions.
Discussion on the limitations of knowing the Riemann tensor, such as the possibility of isometric manifolds with different embeddings.
Final thoughts on the theorema egregium, emphasizing the intrinsic nature of Gaussian curvature and its independence from extrinsic elements.
Quote from Gauss highlighting the theorem's significance in the context of curvature measurement.
Transcripts
Browse More Related Video
Tensor Calculus Lecture 8e: The Riemann Christoffel Tensor & Gauss's Remarkable Theorem
Video 81 - Intrinsic Curvature

Tensor Calculus Lecture 11b: Gauss' Theorema Egregium, Part 2
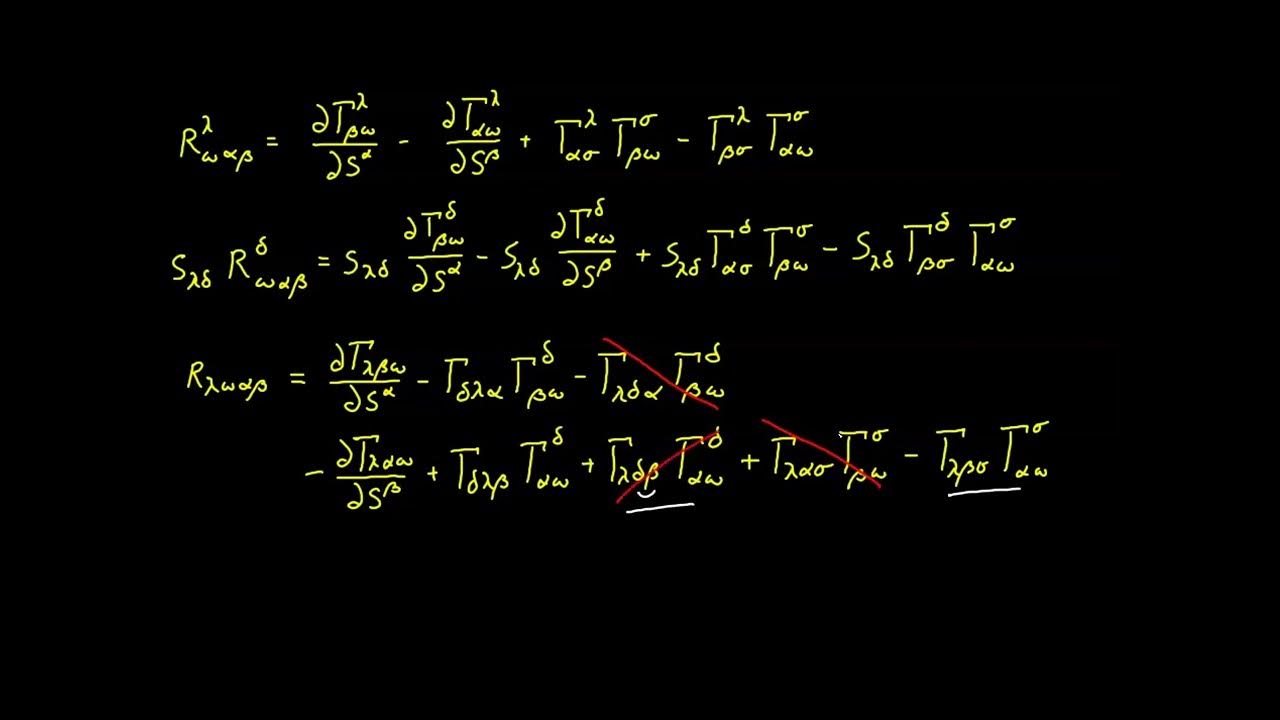
Video 82 - Riemann Christoffel Tensor
Video 92 - Curves Embedded in Surfaces
Tensor Calculus Lecture 8: Embedded Surfaces and the Curvature Tensor
5.0 / 5 (0 votes)
Thanks for rating: