Video 81 - Intrinsic Curvature
TLDRThis video script delves into the intrinsic properties of surface curvature, contrasting the extrinsic curvature tensor approach with a focus on how curvature can be understood from the surface's own measurements. It uses the analogy of two-dimensional 'flatland' creatures to illustrate how discrepancies between calculated and measured values of circles and triangles can reveal the surface's intrinsic curvature. The script also explores the concept of geodesics and the impossibility of parallel lines in positively curved spaces, setting the stage for a detailed exploration of the Riemann curvature tensor in upcoming videos.
Takeaways
- 📚 The video series is exploring tensor calculus with a focus on re-examining surface curvature from an intrinsic perspective rather than using the extrinsic curvature tensor.
- 🔍 The extrinsic approach to curvature involves analyzing the surface from the outside in, using concepts like the ambient space, tangent planes, and normal vectors.
- 🌐 The intrinsic approach, in contrast, focuses on the surface's internal properties, allowing for measurements of distance, area, and angles without any external information.
- 🧐 The script introduces the idea that Gaussian curvature can be derived intrinsically, suggesting that it is a property of the surface itself and not dependent on its embedding in space.
- 🤔 The video uses the example of 'Flatland' creatures to illustrate how intrinsic curvature can be detected by observing discrepancies between calculated and measured values of geometric figures on the surface.
- 📏 It demonstrates that on a curved surface, the actual circumference and area of a circle do not match the values predicted by the formulas derived from plane geometry.
- 📐 The sum of the angles in a triangle and the relationships between side lengths, as given by the law of cosines, can differ from Euclidean expectations when the surface is curved.
- 🚶♂️ The script discusses the concept of geodesics, which are the shortest paths between two points on a curved surface and appear straight to inhabitants of the surface.
- 🤖 The video uses the example of Bert and Ernie walking parallel paths to show that on a positively curved surface, such paths will eventually intersect, contrary to Euclidean geometry.
- 📘 The script points out that Euclid's fifth postulate, which assumes a unique parallel line through a point not on a given line, only holds true in flat spaces and not in curved manifolds.
- 🔬 The video promises a detailed exploration of intrinsic curvature in upcoming episodes, starting with the introduction of the Riemann curvature tensor.
Q & A
What is the main focus of the video series on tensor calculus?
-The main focus of the video series is to re-examine surface curvature from a different perspective, specifically looking at how Gaussian curvature can be derived directly from the intrinsic properties of the surface itself, rather than using the curvature tensor.
What does the term 'extrinsic' refer to in the context of the video?
-In the context of the video, 'extrinsic' refers to properties or measurements that are originating from the outside of the surface being studied, such as the ambient space, tangent plane, normal vector, and the curvature tensor.
How does the video illustrate the concept of extrinsic curvature?
-The video illustrates the concept of extrinsic curvature by using the example of a spherical surface and how it is analyzed from an external viewpoint, including the use of a tangent plane, normal vector, and basis vectors to define the curvature tensor.
What is the difference between extrinsic and intrinsic curvature?
-Extrinsic curvature is analyzed from an external viewpoint, considering how the surface is embedded in the ambient space. In contrast, intrinsic curvature is derived from the surface's own properties without any reference to the external space.
Why did Gauss suspect that Gaussian curvature could be an intrinsic property?
-Gauss suspected that Gaussian curvature could be an intrinsic property because it does not depend on the orientation of the normal, unlike the principal curvatures and mean curvature, which led him to question whether it could be derived without reference to any extrinsic object.
What is the significance of the two-dimensional flatland creatures in the video?
-The two-dimensional flatland creatures are used as a metaphor to illustrate how beings living on a surface might perceive and measure curvature without any knowledge of the third dimension or the ambient space.
How do Bert and Ernie's measurements of a circle on the surface differ from the expected values in a flat plane?
-Bert and Ernie find that as they measure larger circles on the surface, the actual circumference and area deviate from the calculated values based on the formula for a circle in a flat plane, indicating that they do not live on a flat surface.
What is the significance of the triangle example in the video?
-The triangle example demonstrates how the sum of the angles and the lengths of the sides deviate from the expected values in Euclidean geometry when the triangle is large enough to reveal the curvature of the surface.
What is a geodesic in the context of the video?
-A geodesic is a line that is straight within the manifold, meaning it goes from one point to another without turning right or left, following the curvature of the surface.
Why do Bert and Ernie's parallel paths eventually intersect on a curved surface?
-Bert and Ernie's paths intersect on a curved surface because, in a positively curved space like a sphere, it is impossible to draw a line through a point that is parallel to a given line, contrary to Euclid's fifth postulate which only holds true in flat spaces.
What does the video suggest about the limitations of Euclidean geometry in the context of curved surfaces?
-The video suggests that Euclidean geometry, which is based on the assumption of a flat space, has limitations when applied to curved surfaces, as the principles such as the sum of angles in a triangle being 180 degrees or the law of cosines do not hold true on curved manifolds.
Outlines
📚 Introduction to Intrinsic Surface Curvature
This paragraph introduces the concept of re-examining surface curvature from an intrinsic perspective, contrasting it with the extrinsic approach previously discussed. The intrinsic approach focuses on the properties of the surface itself, without reliance on the surrounding space. The video aims to show that Gaussian curvature can be derived directly from the surface's intrinsic properties, rather than using the extrinsic curvature tensor. The extrinsic curvature tensor is defined using external objects like the tangent plane and normal vector, which are related to the surface's embedding in the ambient space. The paragraph also introduces the idea that Gaussian curvature is an intrinsic property, suggesting that it can be understood without reference to any extrinsic objects, and sets the stage for an intrinsic analysis of surface curvature in subsequent videos.
🌐 Exploring Curvature Through Flatland Creatures
This paragraph uses the analogy of two-dimensional creatures, Bert and Ernie, living on a spherical surface to illustrate the intrinsic perspective of curvature. The creatures can measure distances, angles, and areas on the surface but are unaware of the third dimension or the ambient space. The video script describes how these creatures would measure a circle's circumference and area, expecting results based on the formulas for a flat plane. However, as the circle grows larger, discrepancies between the expected and actual measurements emerge, indicating the presence of curvature. The script explains that the deviation from the expected Euclidean results is a clue to the intrinsic curvature of the surface, with positive curvature leading to calculated values larger than actual measurements and vice versa for negative curvature.
🔍 Investigating Triangle Properties on Curved Surfaces
The script explores the properties of triangles on curved surfaces, specifically focusing on the sum of angles and the law of cosines. Initially, the triangle appears to conform to Euclidean geometry, with angles summing to 180 degrees and the law of cosines accurately predicting side lengths. However, as the triangle is enlarged, deviations occur: the angles sum to more than 180 degrees, and the law of cosines no longer matches the actual measurements. This section emphasizes that in a curved manifold, the local properties can appear Euclidean, but as the scale increases, the intrinsic curvature becomes evident, leading to deviations from flat space geometry. The use of geodesics, the shortest paths between points on the surface, is highlighted to explain why the angles in a large triangle on a sphere add up to more than 180 degrees.
🛤️ Parallel Lines and the Geometry of Curved Spaces
This paragraph discusses the concept of parallel lines in the context of curved spaces, using the example of Bert and Ernie walking on a curved surface. In Euclidean geometry, parallel lines never intersect, but on a curved surface, such as a sphere, this is not the case. The script shows that even when the characters attempt to walk in parallel paths, their paths eventually intersect due to the curvature of the surface. This leads to a discussion of Euclid's fifth postulate, which states that through a point not on a given line, there is exactly one line parallel to the given line. The script explains that this postulate only holds true in flat spaces and that in curved spaces, the concept of parallel lines must be redefined. The video concludes by emphasizing the differences between flat and curved spaces and the implications for geometric proofs and theorems.
Mindmap
Keywords
💡Tensor Calculus
💡Gaussian Curvature
💡Intrinsic Properties
💡Extrinsic Curvature Tensor
💡Principal Curvatures
💡Mean Curvature
💡Flatland Creatures
💡Geodesics
💡Euclidean Geometry
💡Riemann Curvature Tensor
Highlights
Introduction to re-examining surface curvature from an intrinsic perspective rather than using the curvature tensor.
Explaining the concept of extrinsic curvature, which involves analyzing curvature from the outside in, using ambient space.
Differentiating between extrinsic and intrinsic properties of a surface, with the latter being independent of the surface's embedding in ambient space.
Illustration of the curvature tensor as an extrinsic object, defined in terms of external objects like the tangent plane and normal vector.
Gaussian curvature identified as an intrinsic property, which can be derived without reference to any extrinsic object.
The idea of analyzing curvature from a 'bug's eye view', focusing only on measurements on the surface itself.
Qualitative illustration of how two-dimensional creatures (Bert and Ernie) on a surface can deduce the intrinsic curvature through measurements.
Demonstration of the discrepancy between calculated and measured values of a circle's circumference and area on a curved surface.
Explanation of how the deviation between calculated and actual measurements can indicate the presence of curvature.
The concept of geodesics as the shortest path between two points on a curved surface, appearing straight to inhabitants of the surface.
Observation that the sum of angles in a triangle on a curved surface deviates from the Euclidean geometry's 180 degrees.
Discussion on the law of cosines and its deviation when applied to a triangle on a curved surface.
The impossibility of maintaining parallel paths on a positively curved surface, leading to their intersection.
Euclid's fifth postulate challenged by the properties of curved spaces, where unique parallel lines do not exist.
The unique solution for parallel lines in flat spaces contrasted with the multiple solutions in negatively curved spaces.
Insight into how intrinsic measurements can reveal the nature of the space without extrinsic information.
Introduction of the Riemann curvature tensor as a tool for detailed analysis of intrinsic curvature in upcoming videos.
Transcripts
Browse More Related Video
Tensor Calculus Lecture 8: Embedded Surfaces and the Curvature Tensor
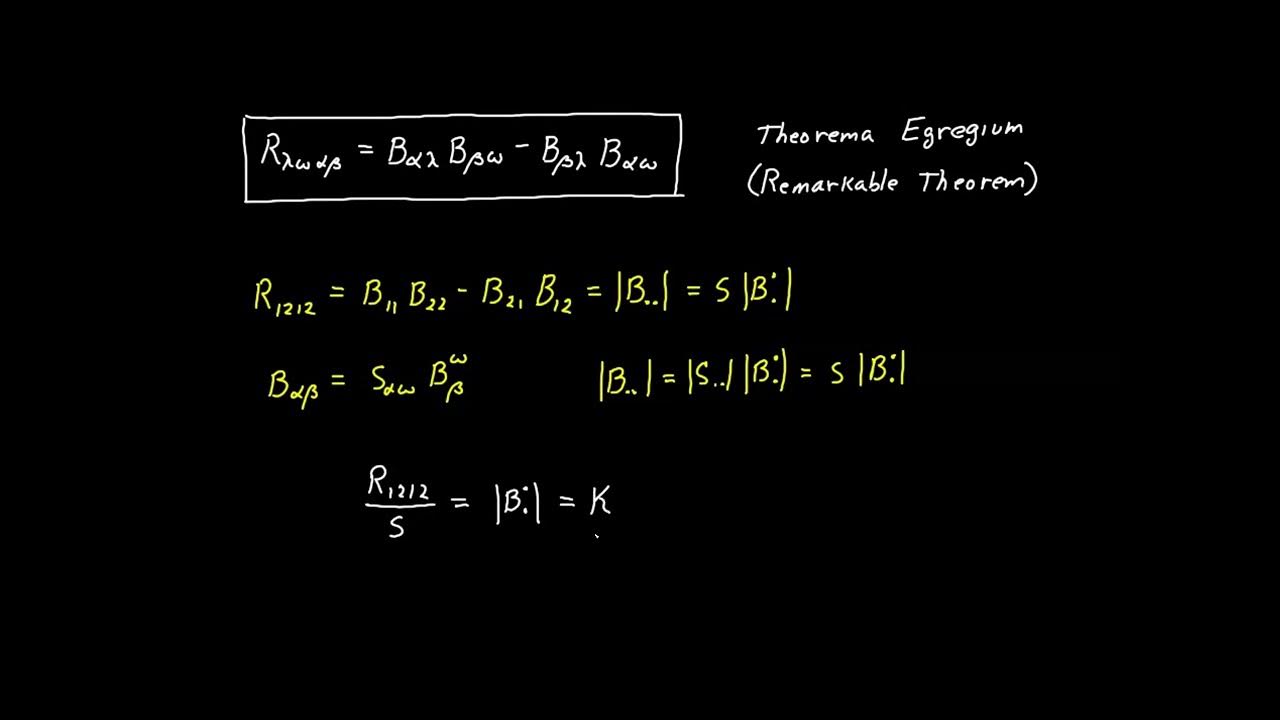
Video 86 - Theorema Egregium
Tensor Calculus Lecture 8e: The Riemann Christoffel Tensor & Gauss's Remarkable Theorem
Non-Euclidean Geometry Explained - Hyperbolica Devlog #1
Tensor Calculus 24: Ricci Tensor Geometric Meaning (Sectional Curvature)
Tensor Calculus 22: Riemann Curvature Tensor Geometric Meaning (Holonomy + Geodesic Deviation)
5.0 / 5 (0 votes)
Thanks for rating: