Tensor Calculus Lecture 11b: Gauss' Theorema Egregium, Part 2

MathTheBeautiful

3 Jun 201423:08

EducationalLearning

32 Likes 10 Comments

TLDRIn this educational video, the presenter embarks on a detailed exploration of Gauss's Theorema Egregium, focusing on its derivation in the context of Euclidean and Riemannian spaces. The script delves into the intricacies of the curvature tensor, the Kedazi relationship, and the derivation of the Gauss-Kedazi equation, which elegantly links the ambient and surface Riemann curvature tensors. The presenter guides viewers through an intricate mathematical derivation, emphasizing the geometric intuition and beauty of the final equations, culminating in a celebration of the elegant and meaningful results of the Gauss-Kedazi theorem.

Takeaways

📚 The lecture focuses on deriving Gauss's Theorema Egregium, a fundamental theorem in differential geometry that relates the intrinsic curvature of a surface to the extrinsic curvature of its embedding in space.
🌐 The script discusses the formulation of Gauss's theorem in the context of Euclidean space and hints at its generalization to Riemannian spaces.
🔍 The Kähler symmetry is highlighted, which states that the Riemann curvature tensor is symmetric in the first two indices, a property that holds only in Euclidean space.
🔄 The derivation involves the use of the commutator of covariant derivatives and the projection of tensors, which is a key technique in the proof.
📉 The script introduces a trick to relate the surface and ambient Riemann curvature tensors by considering one as a projection of the other, simplifying the derivation process.
📝 The derivation process involves applying the product rule and chain rule to the commutator, leading to a series of terms that need to be carefully managed.
📌 The script emphasizes the importance of not assuming the ambient space is flat (i.e., not assuming the ambient Christoffel symbols vanish) for a more general derivation.
⚖️ The final result is a combined relationship known as the Gauss-Kodazzi equation, which encapsulates both the intrinsic and extrinsic curvature properties.
📑 The script provides a method to extract the Kähler equation and Gauss's Theorema Egregium from the more general Gauss-Kodazzi equation by appropriate contractions.
🎯 The lecture concludes with the extraction of Gauss's Theorema Egregium, showcasing the beauty and elegance of the mathematical process and its geometric intuition.
👨‍🏫 The instructor expresses enthusiasm for the derivation process, encouraging students to appreciate the beauty of the mathematical dance of indices and the meaningful results it yields.

Q & A

What is Gauss's Theorem discussed in the script?
-Gauss's Theorem, as discussed in the script, is a remarkable theorem in differential geometry that relates the curvature of a surface to the curvature of the space in which it is embedded. It is formulated in the context of a Euclidean ambient space but can also be generalized to a Riemannian ambient space.
What are the conditions for the Kádár-Thomas relationship to hold?
-The Kádár-Thomas relationship holds when the ambient space is Euclidean. It states that the curvature tensor is symmetric in all its indices, which is a specific form of the relationship that does not necessarily hold in non-Euclidean spaces.
What is the significance of the Kádár-Thomas relationship in the script?
-The Kádár-Thomas relationship is significant because it provides a condition under which the curvature tensor is completely symmetric with respect to all of its indices. This is a key insight in understanding the properties of the curvature tensor in Euclidean spaces.
What is the role of the ambient space in the derivation of Gauss's Theorem?
-In the derivation of Gauss's Theorem, the ambient space plays a crucial role as it provides the context in which the surface is embedded. The properties of the ambient space, such as whether it is Euclidean or Riemannian, influence the form and the validity of the theorem.
What is the difference between the derivation of Gauss's Theorem in a Euclidean ambient space and a Riemannian ambient space?
-In a Euclidean ambient space, certain simplifications occur, such as the vanishing of the ambient Christoffel symbols, which makes the derivation more straightforward. In contrast, in a Riemannian ambient space, these symbols do not necessarily vanish, and the derivation must account for their presence, making the process more complex.
What is the trick mentioned in the script for relating the surface and ambient Riemann curvature tensors?
-The trick involves defining one tensor as a projection of the other and then applying a commutator to a product of these tensors. This allows the use of two independent identities in the same relationship, which helps to derive the relationship between the surface and ambient Riemann curvature tensors.
What is the Gauss-Kádár-Thomas equation mentioned in the script?
-The Gauss-Kádár-Thomas equation is a more general equation that combines both Gauss's Theorem and the Kádár-Thomas relationship. It provides a unified framework for understanding the relationship between the curvature of a surface and the curvature of the space in which it is embedded.
How can one extract the Kádár relationship from the Gauss-Kádár-Thomas equation?
-To extract the Kádár relationship, one should take the Gauss-Kádár-Thomas equation and contract both sides with the normal vector to the surface, effectively removing the terms that are orthogonal to the surface and leaving the Kádár relationship.
How can one derive Gauss's Theorem from the Gauss-Kádár-Thomas equation?
-To derive Gauss's Theorem from the Gauss-Kádár-Thomas equation, one should dot both sides of the equation with the metric tensor, which effectively projects the equation onto the tangent space of the surface, yielding Gauss's Theorem.
What is the significance of the combined Gauss-Kádár-Thomas equation in understanding the geometry of surfaces?
-The combined Gauss-Kádár-Thomas equation is significant as it encapsulates both the normal and tangential components of the relationship between a surface and its ambient space. It provides a comprehensive understanding of how the curvature of a surface is influenced by the curvature of the space in which it is embedded.

Outlines

00:00

📚 Introduction to Gauss's Theorem Derivation

The video begins with an introduction to the derivation of Gauss's theorem in the context of Euclidean and non-Euclidean ambient spaces. The presenter explains the symmetric properties of the curvature tensor and introduces the Kadasdi equation, which is valid only in Euclidean spaces. The main goal is to establish the relationship between the ambient and surface Riemann curvature tensors in a more general setting, assuming the ambient space to be Riemannian. The presenter outlines a strategy to relate the surface Christoffel symbols to the ambient Riemann curvature tensor, hinting at a derivation that involves the use of both tensors and their derivatives.

05:00

🔍 Commutator Application and Chain Rule Utilization

In this segment, the presenter embarks on a detailed calculation by applying a commutator to a product, which involves the use of the chain rule and the surface derivative. The process leads to the expansion of several terms, including the application of the product rule and the fundamental formula relating the normal vector, the curvature tensor, and the Christoffel symbols. The presenter simplifies the expression by considering the ambient space to be Euclidean, which allows certain terms to vanish due to the properties of the ambient Christoffel symbols.

10:07

📉 Symmetry in Commutator Terms and Derivation Progress

The video continues with the analysis of the commutator terms, highlighting their lack of individual symmetry in alpha and beta but showing how they combine to form a symmetric expression when considering the commutator as a whole. The presenter then focuses on the left-hand side of the equation, which involves the surface Riemann curvature tensor, and demonstrates how the terms can be manipulated to reveal the relationship between the surface and ambient tensors. The process involves careful index management and the application of geometric principles.

15:07

🎯 Extracting Gauss's Theorem and Kadasdi Relationship

The presenter achieves a significant milestone by deriving a combined relationship, referred to as the Gauss-Kadasdi equation, which encapsulates both Gauss's theorem and the Kadasdi relationship. This is done by considering the normal component of the equation and demonstrating how to extract the Kadasdi relationship by contracting with the normal vector. The presenter then outlines the steps to derive Gauss's theorem from the combined equation by dotting both sides with the appropriate indices and simplifying the expression.

20:09

🏆 Conclusion and Reflection on the Derivation Process

In the final part of the video, the presenter reflects on the exhilarating process of the derivation, emphasizing the beauty and meaningfulness of the mathematical relationships uncovered. The presenter expresses personal enjoyment in performing such calculations due to the geometric intuition they provide and the satisfaction of reaching a valuable conclusion. The video concludes with a promise to clean up some details in future lectures, leaving the audience with a sense of accomplishment and anticipation for further exploration.

Mindmap

Keywords

💡Gauss's Theorem

Gauss's Theorem, in the context of this video, likely refers to a fundamental theorem in differential geometry, which relates the intrinsic curvature of a surface to the extrinsic curvature of the surface within a higher-dimensional space. The video discusses the formulation of this theorem in the case of a Euclidean ambient space and its generalization to a Riemannian ambient space. The theorem is central to the video's theme of exploring the relationship between the ambient and surface curvature tensors.

💡Euclidean Space

Euclidean space is a mathematical setting where the geometry is defined by the Pythagorean theorem. It is a space of three dimensions where the shortest distance between two points is a straight line. In the video, the speaker discusses how Gauss's theorem is formulated in the simpler case of Euclidean space before delving into the more complex scenario of non-Euclidean spaces.

💡Riemannian Space

A Riemannian space is a type of smooth manifold where each point has a notion of distance defined in a way that is consistent with the properties of Euclidean space but can be generalized to higher dimensions or non-flat geometries. The video mentions that the derivation of Gauss's theorem will be in a more general setting, assuming the ambient space is Riemannian, which allows for a broader application of the theorem.

💡Curvature Tensor

The curvature tensor is a mathematical object that describes the curvature of a space or a surface. In differential geometry, it is used to express how much the space deviates from being flat. The video discusses the properties of the curvature tensor, such as its symmetry, and how it plays a key role in Gauss's theorem.

💡Christoffel Symbols

Christoffel symbols are used in differential geometry to express the covariant derivative, which generalizes the directional derivative to curved spaces. They are related to the way in which the metric tensor changes from point to point on a manifold. The video mentions Christoffel symbols in the context of deriving the relationship between the ambient and surface tensors.

💡Kodazzi Equation

The Kodazzi equation is a relation in differential geometry that connects the extrinsic curvature of a surface with its intrinsic curvature. The video script mentions this equation as a sibling to Gauss's theorem, indicating that they arise simultaneously and are related to the symmetry of the curvature tensor.

💡Commutator

In mathematics, a commutator is an expression that measures the difference between two actions performed in sequence. In the context of the video, the commutator is applied to tensor products to derive relationships between the surface and ambient curvature tensors. The script describes a method of using the commutator to relate these tensors in a more general setting.

💡Surface Laplacian

The surface Laplacian is a differential operator that generalizes the Laplacian operator to surfaces. It is used to study the properties of functions defined on a surface. The video script refers to previous exercises where the relationship between the ambient and surface Laplacians was discussed, indicating its relevance to the theme of the video.

💡Projection

In the context of the video, projection refers to the mathematical operation of taking a vector from one space and mapping it onto a subspace in a way that preserves the shortest distance to the subspace. The script describes a method where one tensor is defined as the projection of another, which is a key step in deriving the Gauss-Kodazzi equation.

💡Gauss-Kodazzi Equation

The Gauss-Kodazzi equation is a more general equation that combines both Gauss's theorem and the Kodazzi equation. The video script describes deriving this equation and then extracting the individual equations from it. This combined equation is a significant result that encapsulates the relationship between the surface and ambient spaces.

Highlights

Introduction to Gauss's remarkable theorem and its formulation in Euclidean space, contrasted with the more complex non-Euclidean case.

Explanation of the symmetry of the curvature tensor in Euclidean space and the introduction of the Kedazi relationship.

Derivation approach: assuming the ambient space is Riemannian rather than Euclidean, highlighting the flexibility in the derivation process.

Explanation of how Gauss's theorem and the Kedazi relationship relate the ambient and surface Riemann-Christoffel tensors.

Discussion on previously derived relationships, such as the connection between the ambient and surface Laplacians and Christoffel symbols.

Introduction to a 'trick' for using identities in the derivation, involving projections of tensors.

Step-by-step derivation process, starting with the application of a commutator to a product, leading to the relationship between the surface and ambient Riemann-Christoffel tensors.

Explanation of the cancellation of terms in the commutator due to symmetry and the assumptions made about the ambient space.

Introduction to the Gauss-Kedazi equation, a general relationship combining both Gauss's theorem and the Kedazi relationship.

Deriving the Kedazi relationship from the Gauss-Kedazi equation by contracting with the normal vector, simplifying to show the equation equals zero.

Deriving Gauss's theorem from the Gauss-Kedazi equation by projecting onto the tangent space and simplifying indices.

Emphasis on the beauty and satisfaction of the derivation process, despite its complexity and the historical context of Gauss's work.

Conclusion with a sense of accomplishment, highlighting the meaningful geometric intuition gained from the derivation.

The speaker's personal reflection on the enjoyment and significance of performing the calculation multiple times.

Announcement of the intention to clean up and revisit previous topics in the following lectures before moving on to more complex calculus.

Transcripts

Browse More Related Video

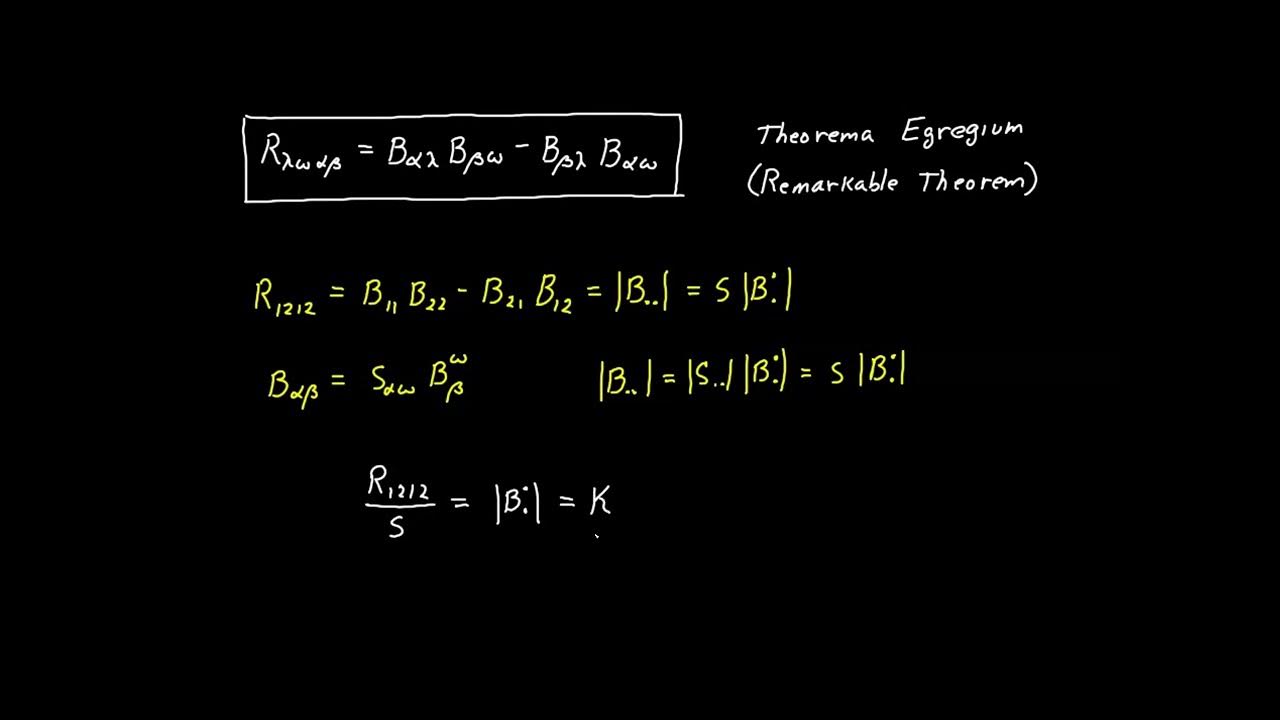
Video 86 - Theorema Egregium

Tensor Calculus Lecture 8e: The Riemann Christoffel Tensor & Gauss's Remarkable Theorem

Tensor Calculus Lecture 11a: Gauss' Theorema Egregium, Part 1

Tensor Calculus 22: Riemann Curvature Tensor Geometric Meaning (Holonomy + Geodesic Deviation)

Video 99 - Holonomy Part 2

Tensor Calculus Lecture 14d: Non-hypersurfaces - Relationship Among Curvature Tensors 1

Related Tags

Gauss's Theorems Euclidean Space Riemann Space Geometric Intuition Calculus Christoffel Symbols Curvature Tensor Kedazi Relationship Surface Laplacian Ambient Space