Tensor Calculus Lecture 8e: The Riemann Christoffel Tensor & Gauss's Remarkable Theorem
TLDRThis script delves into the Riemann Christoffel tensor, a fundamental concept in differential geometry with profound implications in general relativity. It discusses the tensor's intrinsic nature, its distinction from the curvature tensor, and its calculation from the metric tensor. The presentation explores the tensor's symmetries and introduces Gaussian curvature, linking it to the curvature tensor through Gauss's Theorema Egregium. The script concludes with the geometric interpretation of Gaussian curvature and its significance in understanding the curvature of two-dimensional surfaces.
Takeaways
- 📚 The Riemann-Christoffel tensor is intrinsic and can be calculated using the metric tensor and its partial derivatives without reference to an embedding space.
- 🔍 The fundamental difference between the Riemann-Christoffel tensor and the curvature tensor is that the former is intrinsic, while the latter is extrinsic and depends on the embedding space's coordinate basis and normal.
- 🤔 The Riemann-Christoffel tensor arises from the question of whether covariant derivatives commute when applied to tensors, leading to the discovery of this tensor when they do not.
- 🔗 Gauss's Theorema Egregium is a highlight of the lecture, relating the intrinsic Riemann-Christoffel tensor to the extrinsic curvature tensor in a remarkable way.
- 📏 In two dimensions, the Riemann-Christoffel tensor has only one degree of freedom due to its symmetries, simplifying its properties and implications for the geometry of surfaces.
- 📘 The Gaussian curvature is an invariant that can be derived from the Riemann-Christoffel tensor and is a key concept for two-dimensional surfaces, reflecting the surface's intrinsic geometry.
- 🌐 Gaussian curvature is intrinsic and unaffected by how the surface is embedded in the ambient space, making it a fundamental measure of curvature for a surface.
- 🔍 The integral of Gaussian curvature over a closed surface is a topological invariant, depending only on the surface's genus, as explained by the Gauss-Bonnet theorem.
- 📉 The Riemann-Christoffel tensor vanishes in Euclidean coordinates because covariant derivatives commute and the Christoffel symbols are zero, but this is not necessarily true on curved surfaces.
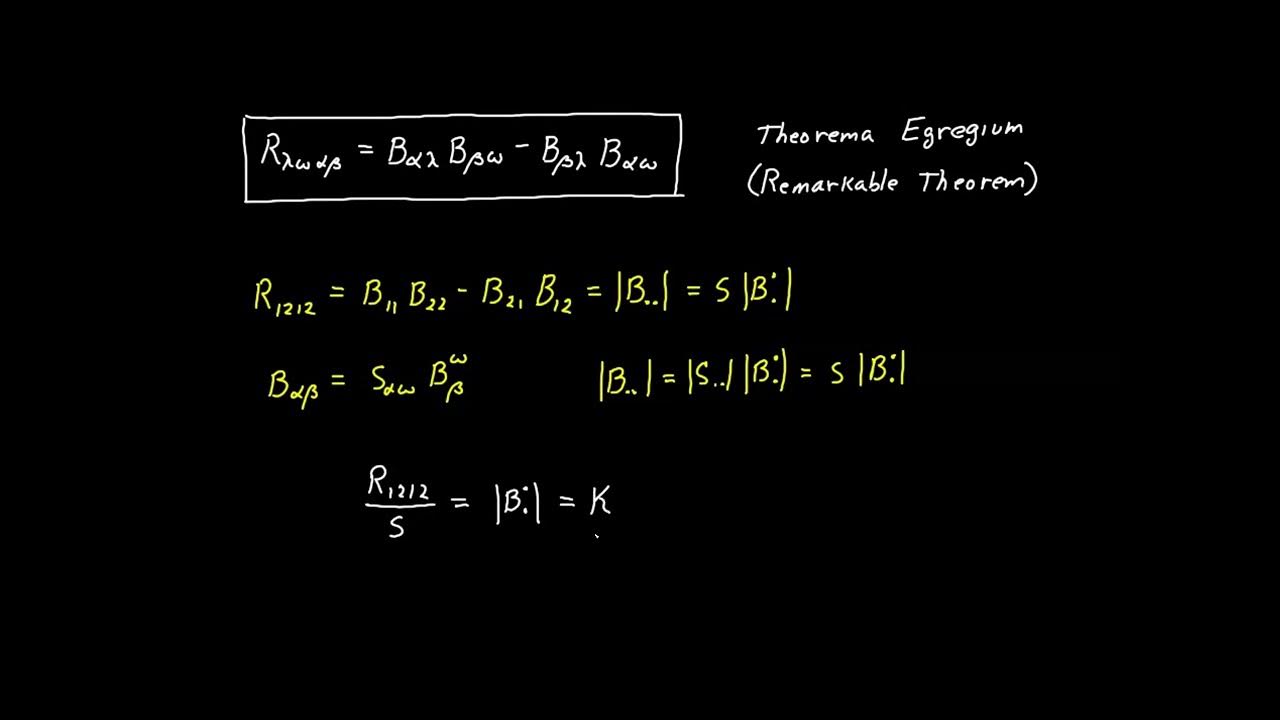
- 🌀 The relationship between the Riemann-Christoffel tensor and Gaussian curvature is expressed through Gauss's Theorema Egregium, showing that the intrinsic curvature can be described by the determinant of a specific form of the curvature tensor.
- 📐 For a sphere, the Gaussian curvature is related to its radius, demonstrating the power of tensor calculus in determining geometric properties from algebraic expressions.
Q & A
What is the Riemann-Christoffel tensor and why is it significant in the context of relativity?
-The Riemann-Christoffel tensor is a measure of the curvature of spacetime in the context of general relativity. It is significant because it describes the intrinsic curvature of a surface without any reference to an embedding in a larger space, making it a fundamental object in the study of the geometry of spacetime.
How is the Riemann-Christoffel tensor different from the curvature tensor discussed in the previous lectures?
-The Riemann-Christoffel tensor is intrinsic, meaning it can be calculated using only the metric tensor and its partial derivatives with respect to the surface variables, without any reference to how the surface is embedded in an ambient space. In contrast, the curvature tensor discussed in previous lectures is extrinsic and depends on the coordinate basis and normal, which are related to the embedding of the surface in a larger space.
What is the fundamental difference between intrinsic and extrinsic properties of a tensor?
-Intrinsic properties of a tensor can be calculated without any reference to an external space or embedding, relying solely on the properties of the space itself. Extrinsic properties, on the other hand, depend on the way the space is embedded in a larger space or the relationship between the space and its surroundings.
What is the relationship between the Riemann-Christoffel tensor and Gauss's theorema egregium?
-Gauss's theorema egregium is a remarkable relationship that links the intrinsic Riemann-Christoffel tensor to the extrinsic curvature tensor. It shows that the Gaussian curvature, which is derived from the Riemann-Christoffel tensor, can be expressed as a combination of the curvature tensor, demonstrating a deep connection between intrinsic and extrinsic curvature properties.
Why is the Riemann-Christoffel tensor able to be calculated without the need for a coordinate basis or normal?
-The Riemann-Christoffel tensor is able to be calculated without a coordinate basis or normal because it is constructed from the metric tensor and its derivatives, which are intrinsic to the surface. This allows for the calculation of curvature based solely on the distances and angles measured within the surface itself, independent of any external embedding.
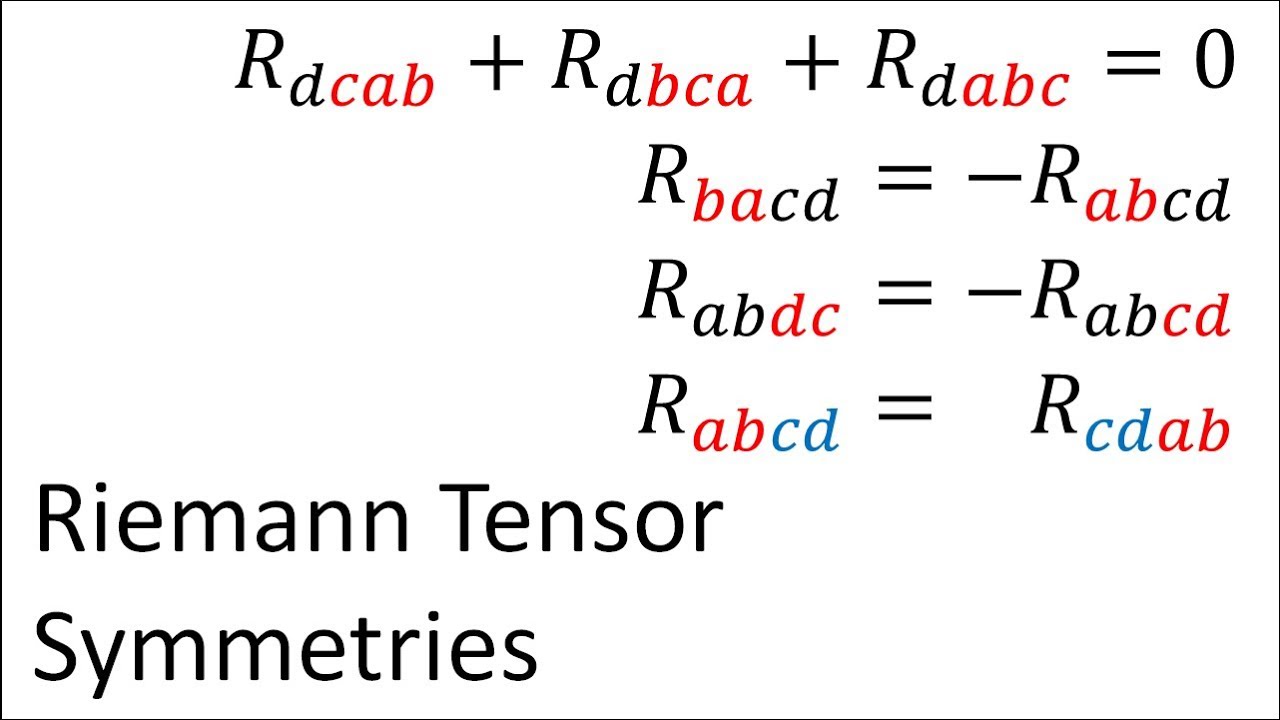
What are the symmetries of the Riemann-Christoffel tensor and how do they simplify its degrees of freedom in two dimensions?
-The Riemann-Christoffel tensor has several symmetries, including skew-symmetry in the first two indices and the last two indices, and a cyclic symmetry when swapping the pairs of indices. In two dimensions, these symmetries reduce the number of independent components to a single degree of freedom, which is the curvature scalar for the surface.
What is the significance of the Gaussian curvature in two-dimensional surfaces?
-Gaussian curvature is an intrinsic invariant that describes the curvature of a two-dimensional surface. It is significant because it is a measure of the total curvature of the surface, which is independent of the surface's shape and depends only on its topological properties, such as the number of handles.
How does the Gaussian curvature relate to the curvature tensor in the context of Gauss's theorema egregium?
-In Gauss's theorema egregium, the Gaussian curvature is shown to be equal to the determinant of a certain form of the curvature tensor with one index raised. This relationship demonstrates that the intrinsic curvature of a surface can be expressed in terms of the extrinsic curvature tensor, highlighting a profound connection between intrinsic and extrinsic geometric properties.
Why is the Riemann-Christoffel tensor zero in Euclidean space?
-The Riemann-Christoffel tensor is zero in Euclidean space because the covariant derivatives commute in this space, and the metric tensor is represented by the identity matrix at all points. This results in the Christoffel symbols, which are used to calculate the tensor, being zero, and thus the tensor itself is zero.
What is the physical interpretation of the Riemann-Christoffel tensor in the context of general relativity?
-In the context of general relativity, the Riemann-Christoffel tensor represents the curvature of spacetime. It encodes the way in which the geometry of spacetime is influenced by the presence of mass and energy, affecting the motion of particles and light as they traverse the curved spacetime.
Outlines
📚 Introduction to Riemann Christoffel Tensor
The script begins with an introduction to the Riemann Christoffel tensor, an intrinsic object in differential geometry with significant applications in the theory of relativity. The tensor is unique in that it can be calculated using only the metric tensor and its partial derivatives, without any reference to the surface's embedding in a larger space. This makes it a fundamental tool for describing the curvature of a space, independent of the coordinate system used. The lecturer hints at the tensor's relationship with Gauss's Theorema Egregium, a highlight of the intrinsic versus extrinsic properties of curvature.
🔍 Exploring the Riemann Christoffel Tensor's Properties
This paragraph delves deeper into the properties of the Riemann Christoffel tensor, discussing its intrinsic nature and how it arises from the question of whether covariant derivatives commute. The lecturer explains the tensor's form and its significance in the context of tensor calculus, emphasizing that while the expression for the tensor is complex, its properties are what truly matter. The paragraph also addresses why the tensor is considered a tensor, applying the quotient theorem to demonstrate its tensorial nature.
🌐 Vanishing of the Riemann Christoffel Tensor in Euclidean Space
The script explains why the Riemann Christoffel tensor vanishes in Euclidean space, providing two arguments. The first argument is based on the tensor's evaluation in Cartesian coordinates, where the Christoffel symbols are zero, leading to the tensor's disappearance. The second argument involves the expression for the Christoffel symbol in terms of basis elements, which, when plugged into the tensor's expression, results in cancellation of terms. The paragraph also discusses the limitations of these proofs in non-Euclidean contexts, such as on a sphere, where Cartesian coordinates may not exist.
📉 Non-Vanishing of the Riemann Christoffel Tensor on Curved Surfaces
The lecturer contemplates the possibility that the Riemann Christoffel tensor may not vanish on certain curved surfaces, such as a sphere, where Cartesian coordinates do not exist. The paragraph explores the implications of this for the tensor's intrinsic curvature properties and discusses the conditions under which the tensor would vanish, such as on surfaces that can be unwrapped into a flat sheet without changing distances, like a cylinder or a cone.
🔗 Symmetries of the Riemann Christoffel Tensor
This section of the script discusses the symmetries inherent in the Riemann Christoffel tensor, which are crucial for understanding its properties. The lecturer identifies two key symmetries: the skew-symmetry in the first two indices and a second symmetry that arises from swapping pairs of indices. These symmetries are foundational for understanding the tensor's behavior and its role in describing curvature.
📏 Gaussian Curvature and Its Intrinsic Nature
The script introduces Gaussian curvature as an invariant that arises from the Riemann Christoffel tensor in two-dimensional spaces. The lecturer explains how Gaussian curvature is intrinsic to the surface and can be computed from the tensor, highlighting its importance as a measure of curvature that is unaffected by the surface's embedding in a larger space. The paragraph also touches on the geometric interpretation of Gaussian curvature and its significance in the Gauss-Bonnet theorem.
🧩 Gauss's Theorema Egregium and the Curvature Tensor
The lecturer presents Gauss's Theorema Egregium, a remarkable theorem that links the intrinsic Gaussian curvature to the extrinsic curvature tensor. The script explores the beauty and elegance of this relationship, showing how the curvature tensor, which describes the surface's embedding in space, can be related to the intrinsic Riemann Christoffel tensor through a specific combination of its components.
📚 Analytical Expression of Gauss's Theorema Egregium
This part of the script focuses on deriving an analytical expression for Gauss's Theorema Egregium, demonstrating that Gaussian curvature is equivalent to the determinant of a specific form of the curvature tensor. The lecturer guides through the algebraic manipulations, emphasizing the importance of tensor properties in this derivation and showcasing the power of tensor notation in understanding and expressing complex geometric relationships.
🌕 Application of Gauss's Theorema Egregium to the Sphere
In conclusion, the script applies the insights from Gauss's Theorema Egregium to the specific case of a sphere, illustrating how the theorem can be used to calculate the Gaussian curvature of a sphere and relate it to the sphere's radius. The lecturer reflects on the significance of the tensor framework in enabling this understanding and the elegance of the mathematical structure that underpins the theory of curvature.
Mindmap
Keywords
💡Riemann Christoffel Tensor
💡Curvature Tensor
💡Gauss's Theorema Egregium
💡Covariant Derivative
💡Metric Tensor
💡Euclidean Space
💡Gaussian Curvature
💡Christoffel Symbols
💡Tensor
💡Einstein Summation Convention
💡Geodesic
Highlights
Introduction to the Riemann Christoffel tensor, an intrinsic object in the field of relativity.
The Riemann Christoffel tensor can be calculated using the metric tensor and its partial derivatives, without reference to the surface's embedding in ambient space.
Fundamental difference between the Riemann Christoffel tensor and the curvature tensor in terms of intrinsic vs. extrinsic properties.
Gauss's Theorema Egregium, a remarkable relationship between the Riemann Christoffel tensor and Gaussian curvature.
Exploration of the Riemann Christoffel tensor's role in the question of whether surface derivatives commute.
Derivation of the Riemann Christoffel tensor from the non-commutativity of covariant derivatives.
Explanation of why the Riemann Christoffel tensor is considered a tensor despite its complex form.
Vanishing of the Riemann Christoffel tensor in Euclidean coordinates due to the properties of Cartesian coordinates.
Discussion on the limitations of proofs involving the Riemann Christoffel tensor in non-Euclidean geometries.
Properties of the Riemann Christoffel tensor, including skew-symmetry and its implications.
Restriction to two dimensions and the simplification of the Riemann Christoffel tensor's degrees of freedom.
Introduction of Gaussian curvature as an intrinsic invariant derived from the Riemann Christoffel tensor.
Gauss's Theorema Egregium in two dimensions, relating Gaussian curvature to the determinant of the curvature tensor.
Geometric interpretation and significance of Gaussian curvature in characterizing the intrinsic curvature of a surface.
Gauss-Bonnet theorem's implication on the total curvature of a closed surface and its independence of shape.
Tensor notation and its importance in deriving and understanding the properties of the Riemann Christoffel tensor and Gaussian curvature.
Practical applications of the Riemann Christoffel tensor in the study of relativity and curvature of space-time.
Summary of the analytical journey from understanding the Riemann Christoffel tensor to Gauss's Theorema Egregium and its geometric insights.
Transcripts
Browse More Related Video
Video 86 - Theorema Egregium
Tensor Calculus 23: Riemann Curvature Tensor Components and Symmetries
Tensor Calculus 22: Riemann Curvature Tensor Geometric Meaning (Holonomy + Geodesic Deviation)
Tensor Calculus Lecture 8: Embedded Surfaces and the Curvature Tensor
Tensor Calculus Lecture 8c: The Curvature Tensor On The Sphere Of Radius R
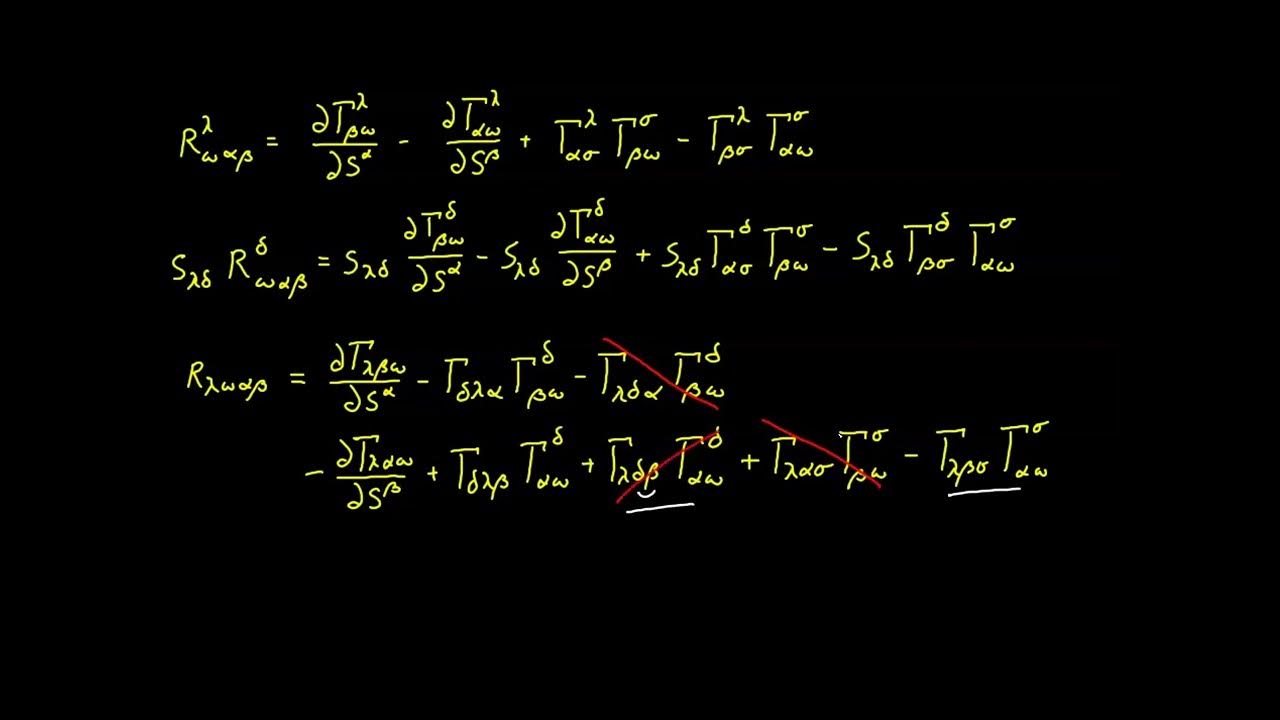
Video 82 - Riemann Christoffel Tensor
5.0 / 5 (0 votes)
Thanks for rating: