Magnetic (AP Physics SuperCram Review)

We Are Showboat
11 May 201204:40
EducationalLearning
32 Likes 10 Comments

TLDRThis script delves into the fundamental principles of magnetic forces on point charges and current-carrying wires, emphasizing the role of the right-hand rule in determining force direction. It explains the maximum force scenario at a 90° angle between velocity and magnetic field, and how to calculate the force using the formula F = qvB sin(Θ). The script also covers the circular motion of charges in a magnetic field, the formula for magnetic field strength around a wire, and the concept of magnetic flux and induced EMF. It concludes with Lenz's law, illustrating how induced currents oppose changes in magnetic flux.

Takeaways
  • 🧲 The magnetic force on a point charge is given by the formula F = qvB sin(θ), where q is the charge, v is the velocity, B is the magnetic field, and θ is the angle between the velocity and the magnetic field.
  • 👐 To determine the direction of the force on a point charge, use the right-hand rule: thumb points in the direction of velocity, index finger in the direction of the magnetic field, and middle finger in the direction of the force on a positive charge.
  • 🔄 When the angle θ is 90°, the force is maximized because sin(θ) is 1.
  • 📏 For a current-carrying wire, the force can be calculated using F = IBL sin(θ), where I is the current and L is the length of the wire.
  • 🔁 The magnetic force causes a charge moving perpendicular to a magnetic field to move in a circular path. The radius of this path is given by r = mv / (qB).
  • ⚡ The magnetic force cannot do work because it is always perpendicular to the velocity of the charge.
  • 🔄 A long straight wire creates a circular magnetic field around it. The direction of this field can be found using another right-hand rule: thumb in the direction of the current and fingers curl in the direction of the magnetic field.
  • 🔬 The magnetic field strength around a wire can be calculated using B = (μI) / (2πr), where μ is the permeability constant, I is the current, and r is the distance from the wire.
  • 🧲 Bar magnets have a north and south pole, with magnetic field lines emanating from the north pole and entering the south pole.
  • ⚡ Magnetic flux is given by Φ = BA cos(θ), and a change in magnetic flux over time induces an electromotive force (EMF). The induced current will oppose the change in flux according to Lenz's Law.
Q & A

  • What is the formula for the magnetic force on a point charge?

    -The magnetic force on a point charge is given by the formula F = qvB sin(Theta), where q is the charge, v is the speed of the charge, B is the magnetic field, and Theta is the angle between the direction of the velocity and the magnetic field.

  • What happens to the magnetic force when the angle Theta is 90 degrees?

    -When the angle Theta is 90 degrees, the force is at its maximum because sin(90°) is 1.

  • How do you use the right-hand rule to find the direction of the magnetic force on a positive charge?

    -To use the right-hand rule, point your thumb in the direction of the velocity, your index finger in the direction of the magnetic field, and your middle finger will point in the direction of the force on a positive charge.

  • How can you find the force on a current-carrying wire?

    -For a current-carrying wire, point your thumb in the direction of the current. The force on the wire can be found using the formula F = ILB sin(Theta), where I is the current, L is the length of the wire, and B is the magnetic field.

  • What is the significance of the magnetic force being perpendicular to the velocity?

    -The significance is that the magnetic force cannot do any work since it is always perpendicular to the velocity of the charge.

  • How do you find the direction of the magnetic field created by a current-carrying wire?

    -Use the right-hand rule: point your thumb in the direction of the current, and your fingers will curl around in the direction of the magnetic field.

  • What is the formula for the magnetic field created by a long straight wire?

    -The magnetic field B created by a long straight wire is given by the formula B = (mu * I) / (2 * pi * R), where mu is a constant (4π x 10^-7 T*m/A), I is the current, and R is the distance from the wire.

  • How do magnetic field lines behave around a bar magnet?

    -Magnetic field lines come out of the North Pole and go into the South Pole of a bar magnet.

  • What happens when a negative charge moves through a magnetic field?

    -When a negative charge moves through a magnetic field, the direction of the force is opposite to that of a positive charge. Use the right-hand rule for a positive charge and then reverse the direction for a negative charge.

  • What is magnetic flux, and how is it calculated?

    -Magnetic flux is given by the formula Φ = B * A * cos(Theta), where B is the magnetic field, A is the area of the loop, and Theta is the angle between the magnetic field and the normal to the loop.

  • What does Lenz's Law state about induced currents?

    -Lenz's Law states that the direction of the induced current will always be such that it opposes the change in magnetic flux that produced it.

Outlines
00:00
🧲 Magnetic Force on a Point Charge

This paragraph explains the formula for the magnetic force on a point charge, which is given by \( qvB \sin(\Theta) \), where \( q \) is the charge, \( v \) is the velocity, \( B \) is the magnetic field, and \( \Theta \) is the angle between the velocity and the magnetic field. The force is maximized when \( \Theta \) is 90°. The right-hand rule is introduced to determine the direction of the force on a positive charge and a current-carrying wire. Additionally, the formula for the force on a wire, \( F = BIL \), is provided, where \( I \) is the current, \( L \) is the length of the wire, and \( B \) is the magnetic field. The paragraph also discusses the circular motion of a charge in a magnetic field and the formula to find the radius of that circle, \( R = \frac{mv}{qB} \).

Mindmap
Keywords
💡Magnetic Force
The magnetic force is the force exerted on a charged particle moving through a magnetic field. It is given by the formula F = qvB sin(θ), where q is the charge, v is the velocity, B is the magnetic field, and θ is the angle between the velocity and the magnetic field. In the video, the magnetic force is discussed as a central concept for understanding how charged particles interact with magnetic fields.
💡Right Hand Rule
The right hand rule is a mnemonic used to determine the direction of the magnetic force on a positive charge moving in a magnetic field. By pointing the thumb in the direction of velocity, the index finger in the direction of the magnetic field, the middle finger then points in the direction of the force. The video explains this rule to help visualize the interaction between magnetic fields and moving charges.
💡Magnetic Field (B)
A magnetic field is a vector field surrounding a magnetic material or a moving electric charge, within which the force of magnetism acts. The strength and direction of the magnetic field at a point in space are represented by the magnetic field vector B. The video discusses how magnetic fields are created by currents and how they influence other charges in their vicinity.
💡Current-Carrying Wire
A current-carrying wire generates a magnetic field around it. The direction of this field can be determined using the right hand rule, with the thumb pointing in the direction of the current and the fingers curling around the wire in the direction of the magnetic field. In the video, this concept is explained as part of understanding how magnetic fields are generated and interact with other currents.
💡Magnetic Flux
Magnetic flux refers to the total magnetic field passing through a given area, typically a loop of wire. It is given by the formula Φ = B * A * cos(θ), where B is the magnetic field, A is the area, and θ is the angle between the field and the perpendicular to the area. The video covers how changes in magnetic flux can induce an electromotive force (EMF) in a loop.
💡Induced EMF
Induced EMF (Electromotive Force) occurs when there is a change in magnetic flux through a loop of wire, according to Faraday's Law of Induction. This induced EMF drives a current through the wire. The video explains how altering the magnetic field, the area of the loop, or the angle between them can induce a voltage in the wire.
💡Lenz's Law
Lenz's Law states that the direction of the induced current will always oppose the change in magnetic flux that caused it. This is a consequence of the conservation of energy and is used to determine the direction of the induced current in response to changing magnetic fields. The video uses Lenz's Law to explain the direction of induced currents in various scenarios.
💡Velocity (v)
Velocity refers to the speed and direction of a moving charge within a magnetic field. The velocity of the charge, in combination with the magnetic field and the charge's magnitude, determines the magnitude and direction of the magnetic force acting on it. The video discusses velocity as a key factor in calculating the magnetic force on a moving charge.
💡Magnetic Field Direction
The direction of a magnetic field is the direction that a north pole of a compass needle would point if placed in the field. Magnetic field lines extend from the north pole of a magnet to the south pole. The video explains how the direction of the magnetic field is crucial in determining the force on a moving charge and the direction of induced currents.
💡Circular Motion in Magnetic Field
When a charged particle moves perpendicularly to a magnetic field, it experiences a force that causes it to move in a circular path. The radius of this path is determined by the balance between the magnetic force and the centripetal force, given by the formula r = mv/qB. The video describes this concept in the context of how magnetic forces influence the trajectory of charged particles.
Highlights

The magnetic force on a point charge is given by qvb sin θ, where q is the charge, v is the speed, B is the magnetic field, and θ is the angle between the velocity and the magnetic field.

When the angle θ is 90°, the force is at its maximum because sin θ is 1.

The right-hand rule is used to find the direction of the force on a point charge: thumb for velocity, pointy finger for magnetic field, and middle finger for force on a positive charge.

For a positive charge with velocity to the right and magnetic field into the page, the force is upwards.

For a current-carrying wire, the right-hand rule is used similarly: thumb in the direction of the current and fingers curling in the direction of the magnetic field.

The formula F = ILB sin θ is used to find the size of the force on a current-carrying wire, where I is the current, L is the length of the wire, and B is the magnetic field.

If the velocity of the charge is perpendicular to the magnetic field, the charge will move in a circle due to the magnetic force.

The radius of the circular path of the charge can be found using the formula r = mv / (qB).

The magnetic force can never do any work since it is always perpendicular to the velocity.

A long straight wire creates a circular magnetic field around it, which can be determined using another right-hand rule.

The size of the magnetic field created by a wire is given by μI / (2πr), where μ is a constant, I is the current, and r is the distance from the wire.

Magnetic field lines emerge from the north pole of a magnet and enter the south pole.

A compass aligns with the direction of the magnetic field.

The magnetic flux through a loop is given by BA cos θ, and changes in magnetic flux induce an EMF.

Lenz's law states that the direction of the induced current will always oppose the change in magnetic flux.

If the magnetic flux increases, the induced current creates a magnetic field in the opposite direction. If the flux decreases, it creates a magnetic field in the same direction.

Transcripts

Browse More Related Video

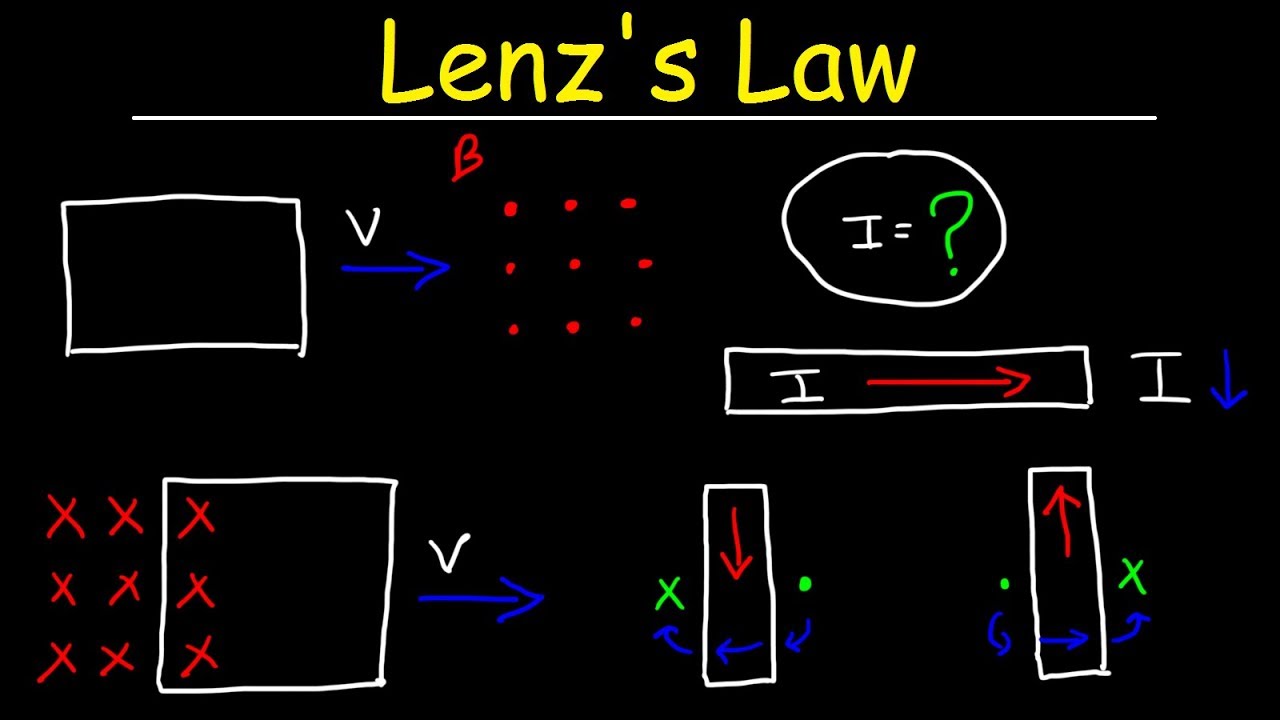
Lenz's Law, Right Hand Rule, Induced Current, Electromagnetic Induction - Physics

Magnetism, Magnetic Field Force, Right Hand Rule, Ampere's Law, Torque, Solenoid, Physics Problems

Magnetic Force on a Current Carrying Wire

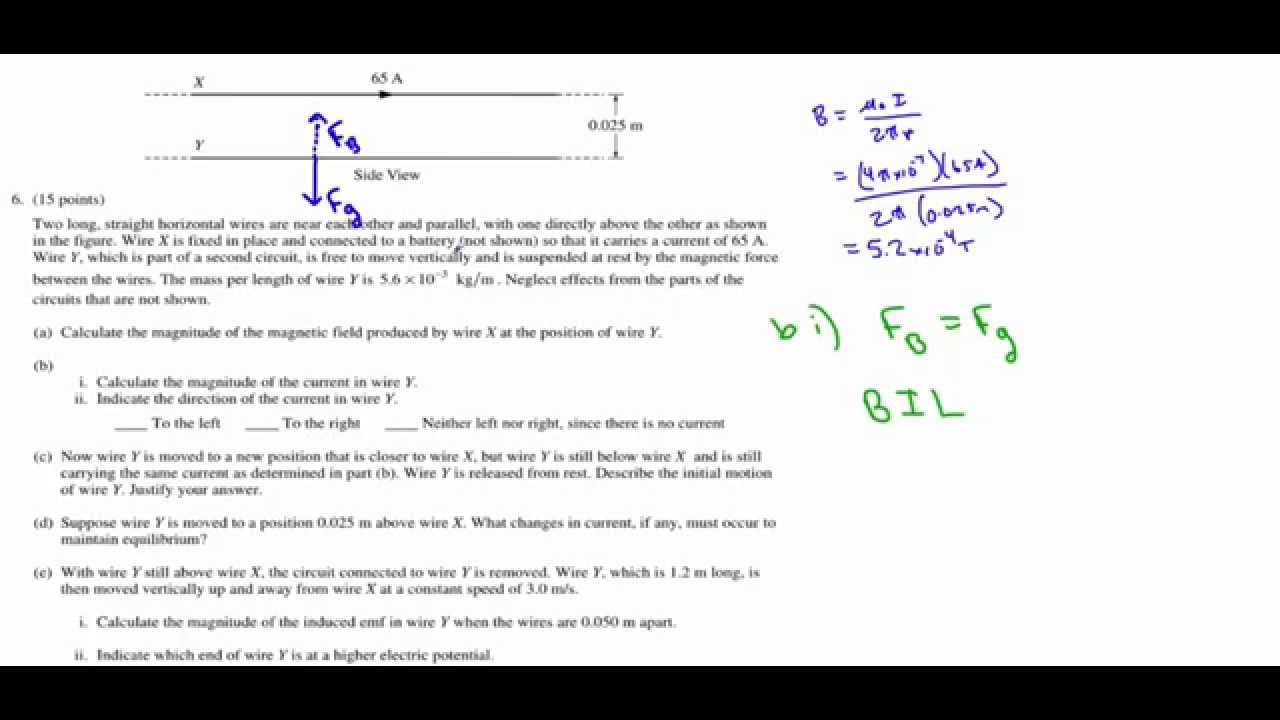
AP Physics B 2013 Question 6 - Electromagnetism

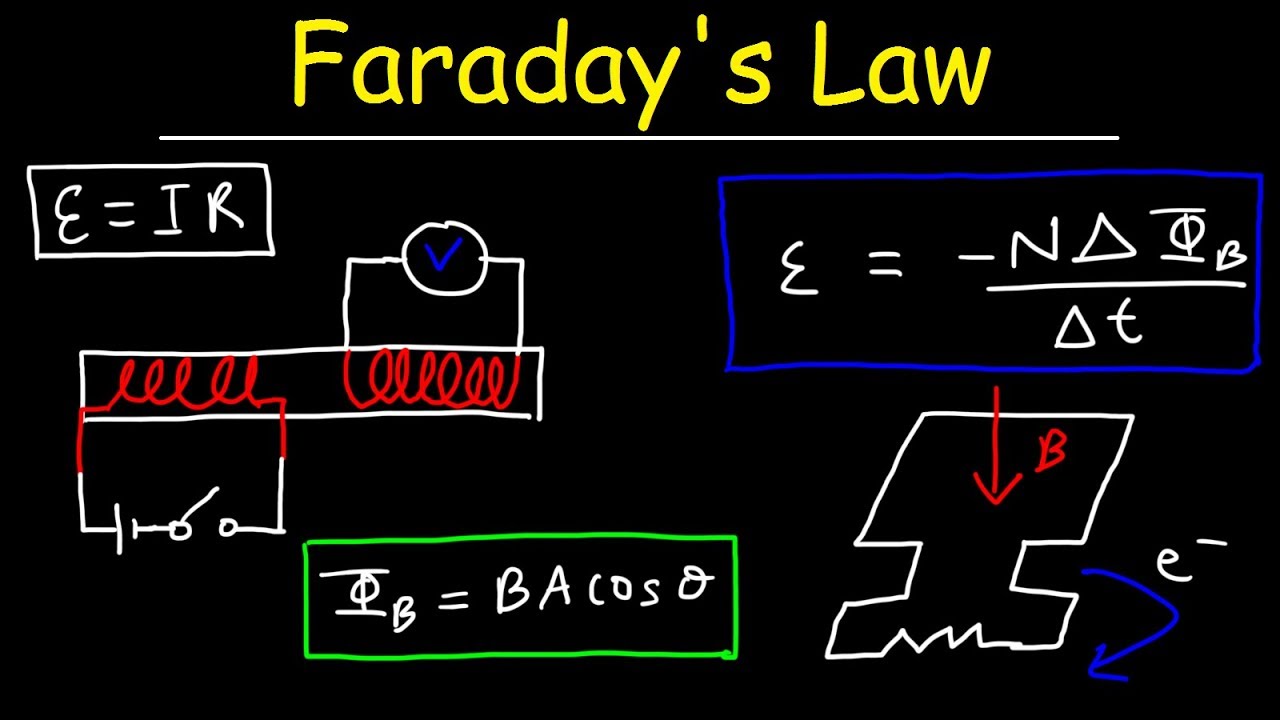
Faraday's Law of Electromagnetic Induction, Magnetic Flux & Induced EMF - Physics & Electromagnetism

EMF and flux explained

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Magnetic ForceElectromagnetismPoint ChargeRight Hand RuleMagnetic FieldCurrent Carrying WireInduced EMFMagnetic FluxLorentz ForcePhysics EducationElectrical Engineering