AP Physics B 2013 Question 6 - Electromagnetism
TLDRThis script dissects a 2013 AP Physics B exam question involving magnetic fields and induced EMF. It explains calculating the magnetic field around a current-carrying wire, determining the current in a second wire suspended by magnetic force, and analyzing the motion of a wire when brought closer to the first. The script also covers the induced EMF when a wire moves through a magnetic field, using Faraday's law of electromagnetic induction and the right-hand rule to determine electric potential.
Takeaways
- 🔌 The problem involves two long, straight wires with currents flowing through them, and it's a part of the 2013 AP Physics B exam.
- 🧲 The magnetic field produced by the top wire at the position of the bottom wire is calculated using the formula \( B = \frac{{\mu_0 I}}{{2 \pi r}} \).
- 📏 Given: current in the top wire (65 A), distance between wires (0.025 m), and permeability of free space (4π x 10^-7 T·m/A).
- 📐 The magnetic field at the bottom wire due to the top wire is found to be \( 5.2 \times 10^{-4} \) T.
- ⚖️ The bottom wire is in equilibrium, meaning the magnetic force must balance the weight of the wire.
- 🔄 The current in the bottom wire is found using the equilibrium condition \( BIL = mg \), where m/L is the mass per unit length.
- 💡 Calculations yield that the current in the bottom wire is approximately 108 A.
- 🔀 The direction of the current in the bottom wire must be the same as the top wire for them to attract each other, thus it flows to the right.
- 🏃 When the bottom wire is moved closer to the top wire, the increased magnetic force causes it to accelerate towards the top wire.
- 🧲 If the bottom wire is placed above the top wire, to maintain equilibrium, the currents must be anti-parallel to repel each other.
- 💡 When the bottom wire moves up at a constant speed in the magnetic field, the induced EMF is calculated using \( \text{EMF} = BLv \).
- ⚡ The induced EMF is found to be \( 9.4 \times 10^{-4} \) V when the bottom wire is 0.05 m away from the top wire.
- 🔋 Using the right-hand rule, the direction of the force on moving charges in the wire helps determine that the right end of the wire is at a higher potential.
Q & A
What is the current in the top wire (wire X) mentioned in the problem?
-The current in the top wire (wire X) is 65 amps.
What is the distance between wire X and wire Y?
-The distance between wire X and wire Y is 0.025 meters.
What formula is used to calculate the magnetic field produced by wire X at the position of wire Y?
-The formula used is B = (μ₀ * I) / (2π * r), where μ₀ is the permeability of free space, I is the current, and r is the distance from the wire.
What is the value of the magnetic field produced by wire X at the position of wire Y?
-The magnetic field produced by wire X at the position of wire Y is 5.2 * 10^-4 Tesla.
How do you determine the magnitude of the current in wire Y?
-The magnitude of the current in wire Y is determined by equating the magnetic force to the weight of wire Y, and using the formula I = (m * g) / (B * L), where m is mass per unit length, g is the gravitational acceleration, B is the magnetic field, and L is the length of the wire.
What is the calculated magnitude of the current in wire Y?
-The calculated magnitude of the current in wire Y is approximately 108 amps.
In which direction does the current in wire Y flow to maintain equilibrium?
-The current in wire Y flows in the same direction as the current in wire X, which is to the right.
What happens to wire Y if it is moved closer to wire X and released from rest?
-If wire Y is moved closer to wire X and released from rest, it will accelerate towards wire X because the magnetic force becomes larger due to the decreased distance.
What changes are necessary to maintain equilibrium if wire Y is moved above wire X?
-To maintain equilibrium when wire Y is moved above wire X, the current in wire Y must flow in the opposite direction (to the left) to create a repulsive force.
What is the induced EMF in wire Y when it is moved up at a constant speed of 3 m/s?
-The induced EMF in wire Y when moved up at a constant speed of 3 m/s is 9.4 * 10^-4 volts.
Which end of wire Y is at a higher electric potential when it is moved up at a constant speed?
-The right end of wire Y is at a higher electric potential.
How is the direction of the force on the moving wire Y determined?
-The direction of the force on the moving wire Y is determined using the right-hand rule, considering the direction of motion, the magnetic field, and the resulting force direction on the wire.
Outlines
🔬 Calculating the Magnetic Field Produced by Wire X
This paragraph discusses the calculation of the magnetic field produced by a wire (Wire X) at a point 0.025 meters away from it. The current flowing through Wire X is 65 amps. The formula used for the magnetic field (B) is B = μ₀I / 2πr, where μ₀ (the permeability of free space) is 4π x 10⁻⁷ Tm/A, I is the current (65 A), and r is the distance (0.025 m). Substituting these values, the magnetic field at the specified location is calculated to be 5.2 x 10⁻⁴ Tesla.
📏 Determining the Current in Wire Y
This paragraph explains how to determine the current in Wire Y, which is suspended at rest below Wire X due to the magnetic force between the wires. The magnetic force must balance the weight of Wire Y for equilibrium. The formula used is F = BIL, where B is the magnetic field (5.2 x 10⁻⁴ T), I is the current, and L is the length of Wire Y. Given the mass per unit length (5.6 x 10⁻³ kg/m) and acceleration due to gravity (10 m/s²), the current in Wire Y is calculated using I = (mass per unit length x g) / (B x L), resulting in approximately 108 amps. The direction of the current in Wire Y is determined to be the same as that in Wire X, making the wires attractive and keeping Wire Y suspended.
🔄 Initial Motion of Wire Y When Moved Closer to Wire X
This paragraph describes the initial motion of Wire Y when it is moved closer to Wire X. Since the magnetic force between the wires depends inversely on the distance, moving Wire Y closer increases the force, making it greater than the weight of Wire Y. This results in a net force toward Wire X, causing Wire Y to accelerate toward Wire X.
🛠️ Adjusting for Equilibrium When Wire Y is Above Wire X
This paragraph discusses the changes needed to maintain equilibrium if Wire Y is moved above Wire X. Previously, the attractive force between the parallel currents in the same direction kept Wire Y suspended. Now, to maintain equilibrium with Wire Y above, the currents in the wires must be antiparallel to repel each other. This would provide an upward magnetic force to balance the downward gravitational force.
🚀 Induced EMF in Moving Wire Y
This paragraph focuses on the induced EMF in Wire Y when it is moved upward at a constant speed after being disconnected from its circuit. The wire, now 1.2 meters long and moving at 3 m/s, experiences a changing magnetic flux, inducing an EMF. Using the formula EMF = B L v, where B is the magnetic field (2.6 x 10⁻⁴ T, recalculated for the new distance), L is the length of the wire, and v is the velocity, the induced EMF is calculated to be 9.4 x 10⁻⁴ volts.
🔧 Determining the High Electric Potential End of Wire Y
This paragraph discusses identifying the higher electric potential end of Wire Y, considering the induced EMF. Using the right-hand rule for a moving conductor in a magnetic field, the direction of the induced current and thus the potential difference across the wire is determined. The wire moves up through a magnetic field pointing out of the page, leading to a force on the electrons to the right. Consequently, the right end of the wire becomes positive, and the left end negative.
Mindmap
Keywords
💡Magnetic Field
💡Current
💡Force
💡Equilibrium
💡Ampere
💡Tesla
💡Mass per Unit Length
💡Induced EMF
💡Right Hand Rule
💡Gravitational Force
Highlights
Problem involves two long, straight wires with currents and magnetic interactions.
Top wire carries a 65 A current; bottom wire is 0.025 meters away with given mass per unit length.
Equation for magnetic field produced by a wire: B = (μ₀I) / (2πr).
Calculation of the magnetic field 0.025 meters away from the top wire results in 5.2 x 10^-4 Tesla.
The magnetic force must equal the weight of the second wire for equilibrium.
Current in the second wire is found using the formula: I = (mg) / (BL), resulting in approximately 108 A.
Direction of current in the second wire must be the same as the first for attraction, hence to the right.
When moved closer to the top wire, the second wire will experience a greater magnetic force and accelerate towards the top wire.
If the second wire is moved above the first wire, their currents must be anti-parallel to maintain equilibrium.
With no current, the moving second wire will experience an induced EMF due to the changing magnetic flux.
Induced EMF calculated using B = 2.6 x 10^-4 T, L = 1.2 m, V = 3 m/s results in 9.4 x 10^-4 V.
Right-hand rule helps determine the direction of the induced current and the potential difference across the wire.
Explanation using Lenz's Law: the induced current creates a magnetic field opposing the change in flux.
High potential is on the right end of the wire, corresponding to the direction of the induced current.
Detailed analysis and application of electromagnetic theory in a practical exam setting.
Transcripts
Browse More Related Video
Magnetic (AP Physics SuperCram Review)

Magnetism, Magnetic Field Force, Right Hand Rule, Ampere's Law, Torque, Solenoid, Physics Problems
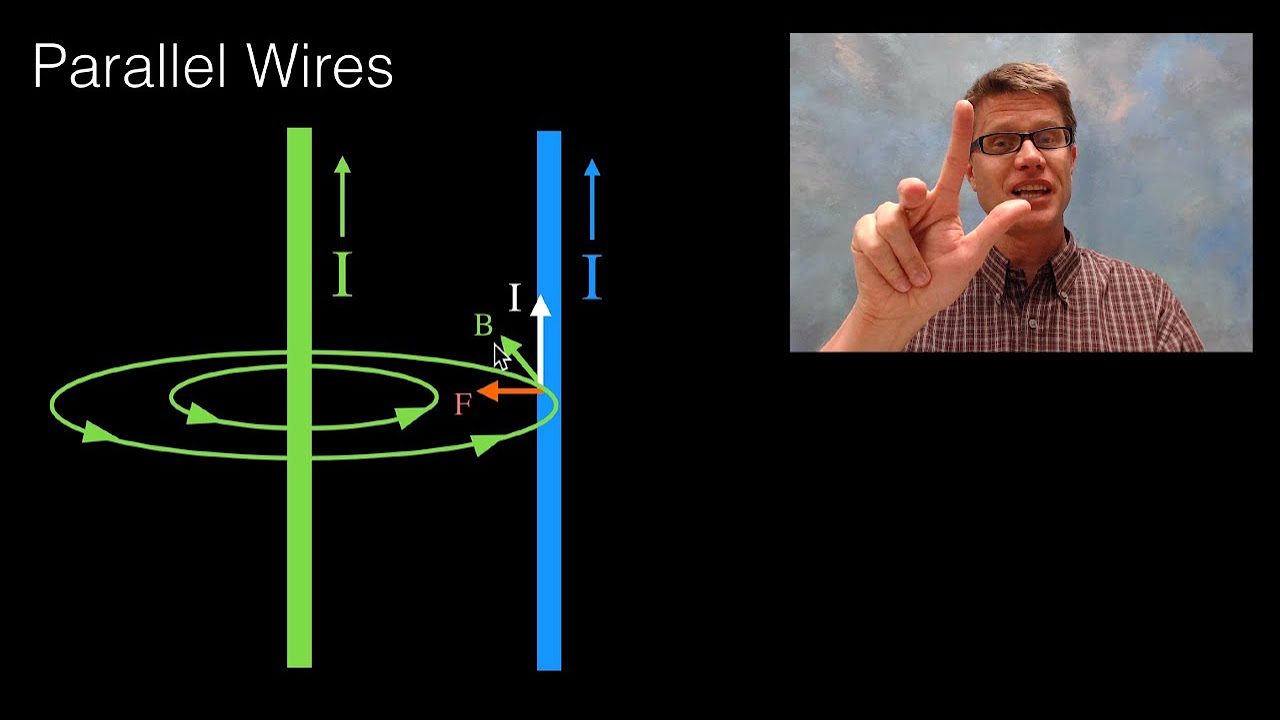
Magnetic Field of a Wire
Faraday's Law of Electromagnetic Induction, Magnetic Flux & Induced EMF - Physics & Electromagnetism
Faraday's & Lenz's Law of Electromagnetic Induction, Induced EMF, Magnetic Flux, Transformers
michael faraday | law of electromagnetic induction | faraday's law of induction
5.0 / 5 (0 votes)
Thanks for rating: