Polar to Cartesian | MIT 18.01SC Single Variable Calculus, Fall 2010

MIT OpenCourseWare
7 Jan 201108:41
EducationalLearning
32 Likes 10 Comments

TLDRIn this educational video, the instructor guides viewers through the process of converting polar coordinates to Cartesian coordinates for two given equations. The first equation, \( r^2 = 4r\cos(\theta) \), is transformed and simplified to reveal a circle with a radius of 2, centered at (2, 0). The second equation, \( r = 9\tan(\theta)\sec(\theta) \), is rewritten to express a parabola in Cartesian coordinates, with a stretch factor of 9. The video emphasizes the importance of recognizing the forms of these equations to identify the shapes of the curves they represent.

Takeaways
  • 🎓 Welcome back to recitation, focusing on relating polar and Cartesian coordinates.
  • ✍️ Problem 1: Convert r^2 = 4r cos θ to Cartesian coordinates.
  • 📏 Cartesian coordinates are represented as (x, y).
  • 📐 Convert r^2 = 4r cos θ to x^2 + y^2 = 4x.
  • 🔍 Solve x^2 + y^2 - 4x = 0 by completing the square.
  • ⭕ The equation (x - 2)^2 + y^2 = 4 represents a circle with center (2, 0) and radius 2.
  • ✍️ Problem 2: Convert r = 9 tan θ sec θ to Cartesian coordinates.
  • 🔍 Simplify r cos θ = 9 tan θ to x = 9 y/x.
  • 📐 Solve x^2 = 9y to describe a parabola.
  • ⚠️ Note: Consider domain restrictions for sec θ and tan θ.
Q & A

  • What is the main objective of the video?

    -The main objective of the video is to demonstrate the process of converting polar coordinates to Cartesian coordinates and describing the resulting curves.

  • What are the two polar equations given in the video?

    -The two polar equations given are r^2 = 4r cos(θ) and r = 9 tan(θ) sec(θ).

  • What is the Cartesian coordinate system also known as?

    -The Cartesian coordinate system is also known as the (x, y) coordinate system.

  • What is the first step in converting the first polar equation to Cartesian coordinates?

    -The first step is to replace r^2 with x^2 + y^2 and r cos(θ) with x.

  • Why is it not advisable to solve for y in the first equation?

    -Solving for y is not advisable because y might not be a function of x, and taking the square root could lead to loss of information about the curve.

  • What mathematical technique is used to simplify the first equation into a recognizable curve form?

    -The mathematical technique used is completing the square to transform the equation into a form that resembles a circle.

  • What is the resulting curve described by the first equation in Cartesian coordinates?

    -The resulting curve is a circle with a center at (2, 0) and a radius of 2.

  • How is secant theta related to cosine theta in the context of the second equation?

    -Secant theta is the reciprocal of cosine theta, which means sec(θ) = 1/cos(θ).

  • What is the relationship between the second polar equation and the Cartesian coordinate x?

    -The second polar equation can be rewritten as x = 9 * (y/x), which simplifies to x^2 = 9y in Cartesian coordinates.

  • What type of curve does the second equation represent in Cartesian coordinates?

    -The second equation represents a parabola that passes through the origin (0, 0) with a vertical or horizontal stretch factor of 9 or 1/9.

  • What potential issue is mentioned regarding the domain of the second equation?

    -The potential issue is that secant theta is not defined for all values of theta, which could affect the domain of the curve and what part of it is well-defined.

Outlines
00:00
📚 Polar to Cartesian Conversion and Curve Description

This paragraph introduces a mathematical problem that involves converting polar coordinates to Cartesian coordinates and describing the resulting curves. The instructor presents two equations: one involving r squared equals 4r cosine theta and the other r equals 9 tangent theta secant theta. The goal is to rewrite these in terms of x and y and to identify the shapes of the curves they represent. The instructor also advises on the importance of recognizing the curve's form and not just converting the coordinates.

05:01
🔍 Detailed Analysis of Polar Equations into Cartesian

The second paragraph delves into solving the given problem by converting the polar equations into Cartesian coordinates. The first equation is manipulated algebraically to reveal it represents a circle with a center at (2, 0) and a radius of 2. The process involves recognizing patterns that suggest completing the square, which leads to the circle's equation. The second equation is simplified by understanding the trigonometric identities involved, leading to the identification of a parabola that passes through the origin with a vertical or horizontal stretch factor of 9 or 1/9. The instructor also touches on the limitations of the domain for secant theta and the implications for the curve's definition.

Mindmap
Keywords
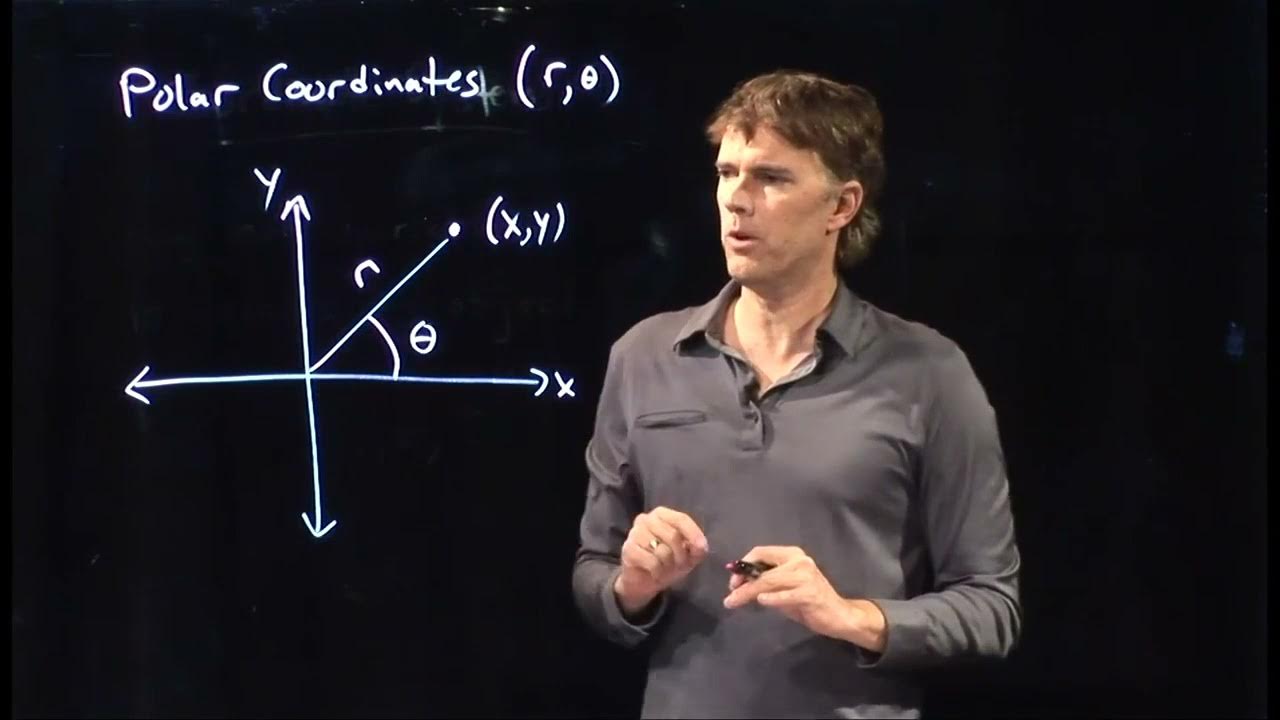
💡Polar coordinates
Polar coordinates are a two-dimensional coordinate system in which each point on a plane is determined by a distance from a reference point and an angle from a reference direction. In the video, the instructor uses polar coordinates to define the initial equations of the curves before converting them into Cartesian coordinates. The script mentions 'r squared equals 4r cosine theta' as an example of a polar coordinate equation.
💡Cartesian coordinates
Cartesian coordinates, also known as rectangular coordinates, are a system that specifies each point uniquely in a plane by a pair of numerical values, which are the distances to two fixed perpendicular directed lines from the point. The video's main theme revolves around converting equations from polar to Cartesian coordinates, which are expressed as (x, y) pairs, to describe the curves in a more familiar way.
💡Conversion
Conversion in the context of the video refers to the process of transforming equations from one coordinate system to another. The script discusses converting polar equations to Cartesian form, which is essential for understanding the geometric representation of the curves described by the equations.
💡Curve description
Curve description involves explaining the geometric properties and shapes of curves represented by mathematical equations. The video aims to not only convert equations into Cartesian coordinates but also to describe what kind of curves they represent, such as circles or parabolas, as seen in the script when the instructor describes the curve as a circle with a specific radius and center.
💡r squared
In polar coordinates, 'r squared' represents the square of the radial distance from the origin to a point on the curve. The script uses 'r squared equals 4r cosine theta' to introduce the concept of converting a polar equation into a recognizable Cartesian form, which eventually describes a circle.
💡Cosine theta
Cosine theta (cos θ) is a trigonometric function that relates the angle θ to the x-coordinate in polar coordinates. In the video, 'r cosine theta' is used to represent the x-coordinate, which is essential for converting the polar equation to Cartesian coordinates.
💡Completing the square
Completing the square is a mathematical technique used to solve quadratic equations or to rewrite them in a form that makes certain properties more evident. In the script, the instructor uses this technique to transform the equation into a perfect square, which helps identify the curve as a circle.
💡Circle
A circle is a geometric shape consisting of all points in a plane that are equidistant from a given point, called the center. The video identifies one of the converted equations as representing a circle with a specific center and radius, illustrating the process of curve description after coordinate conversion.
💡Tangent theta
Tangent theta (tan θ) is a trigonometric function that represents the ratio of the opposite side to the adjacent side in a right-angled triangle, which in the context of the video, relates to the y-coordinate over the x-coordinate in polar coordinates. The script uses 'r equals 9 tangent theta secant theta' to introduce the concept of converting this relationship into Cartesian coordinates.
💡Secant theta
Secant theta (sec θ) is the reciprocal of cosine theta and is another trigonometric function. In the video, the instructor explains that 'secant theta' can be used to convert the polar equation into Cartesian coordinates, which eventually describes a parabola.
💡Parabola
A parabola is a U-shaped curve that is defined as the set of all points equidistant from a fixed point (the focus) and a line (the directrix). The video describes the second curve as a parabola after converting the polar equation into Cartesian coordinates, showcasing another type of curve that can be represented mathematically.
Highlights

Introduction to converting polar to Cartesian coordinates, focusing on r^2 = 4r cos θ and r = 9 tan θ sec θ.

Explanation of how r^2 = x^2 + y^2 in Cartesian coordinates.

Identifying r cos θ as x in Cartesian coordinates.

The importance of manipulating equations to reveal recognizable forms, such as circles or parabolas.

Completing the square technique used to transform x^2 - 4x into (x - 2)^2.

Resulting equation (x - 2)^2 + y^2 = 4 represents a circle centered at (2, 0) with radius 2.

Converting r = 9 tan θ sec θ to Cartesian form using r cos θ = x and tan θ = y/x.

Final form x = 9y, indicating a parabola in Cartesian coordinates.

Discussion of domain limitations for sec θ and implications for defining the curve.

Emphasis on understanding the relationship between polar and Cartesian coordinates.

Avoiding loss of information by not taking square roots prematurely in the conversion process.

Recognition that sec θ being undefined at certain angles limits the defined domain of the curve.

Clarification that the goal is to illustrate coordinate conversions, not to fully explore all technicalities.

Highlighting that the exercises help understand coordinate systems and the appearance of curves.

Conclusion that the lesson demonstrates practical applications of converting polar equations to Cartesian form.

Transcripts

Browse More Related Video

Lesson 14 - Polar Equations (Calculus 2 Tutor)

Polar coordinates 2 | Parametric equations and polar coordinates | Precalculus | Khan Academy

Calculus 2: Polar Coordinates (Video #30) | Math with Professor V

Lec 33 | MIT 18.01 Single Variable Calculus, Fall 2007

How to Convert From Polar Equations to Rectangular Equations (Precalculus - Trigonometry 40)

Polar Coordinate System | Physics with Professor Matt Anderson | M3-02

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Polar CoordinatesCartesian CoordinatesMathematicsEducationalCurvesCircle EquationEllipseParabolaTrigonometryGeometryProblem Solving