Lesson 14 - Polar Equations (Calculus 2 Tutor)

Math and Science

18 Aug 201604:00

EducationalLearning

32 Likes 10 Comments

TLDRThis video tutorial introduces the concept of polar coordinates and equations, contrasting them with the more familiar Cartesian coordinates. It explains that while Cartesian coordinates use x and y values, polar coordinates are defined by the distance from the origin (r) and an angle (theta). The instructor illustrates this by presenting the polar equation for a circle, which is simply r = 2, highlighting that the radius remains constant regardless of the angle, thus forming a circle. The video aims to show how to visualize and convert between polar and rectangular formats, particularly for functions involving polar coordinates.

Takeaways

📚 The section discusses advanced calculus, specifically polar coordinates and polar equations.
📐 Polar coordinates are an alternative to Cartesian coordinates (x, y), where points are defined by a distance from the origin (r) and an angle (theta).
📈 In polar coordinates, functions are defined differently; instead of y = f(x), they are expressed as r = f(theta), where r varies with the angle theta.
🔄 The script introduces the concept of converting between polar and rectangular coordinate systems.
🎨 The video aims to show what polar coordinate graphs look like and how to interpret them.
📈 The analogy between Cartesian and polar functions is highlighted, with r taking the role of y in Cartesian coordinates.
📊 A key example given is the polar equation of a circle, which is r = 2, illustrating the simplicity of representing circles in polar coordinates.
🔄 The general form of a polar equation has r as a function of theta, but for a circle, r is a constant and does not depend on theta.
🔶 The script explains that the absence of theta in the equation for a circle means that r remains constant, forming a perfect circle.
🤔 The discussion hints at the potential for more complex polar equations that can trace out various shapes with circular symmetry or other patterns.

Q & A

What is the main topic discussed in this section of the advanced calculus tutorial?
-The main topic discussed in this section is polar coordinates and polar equations, with a focus on understanding how to represent and convert between polar and rectangular coordinate systems.
What is the difference between a function in Cartesian coordinates and a function in polar coordinates?
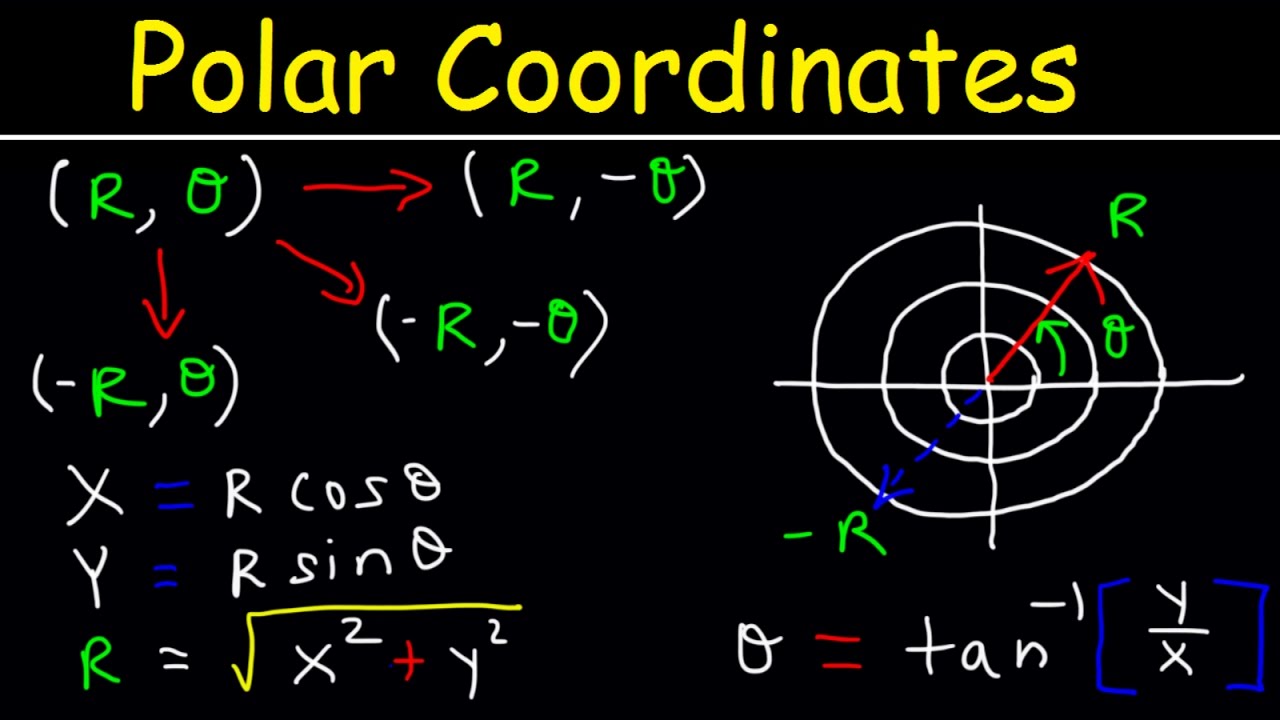
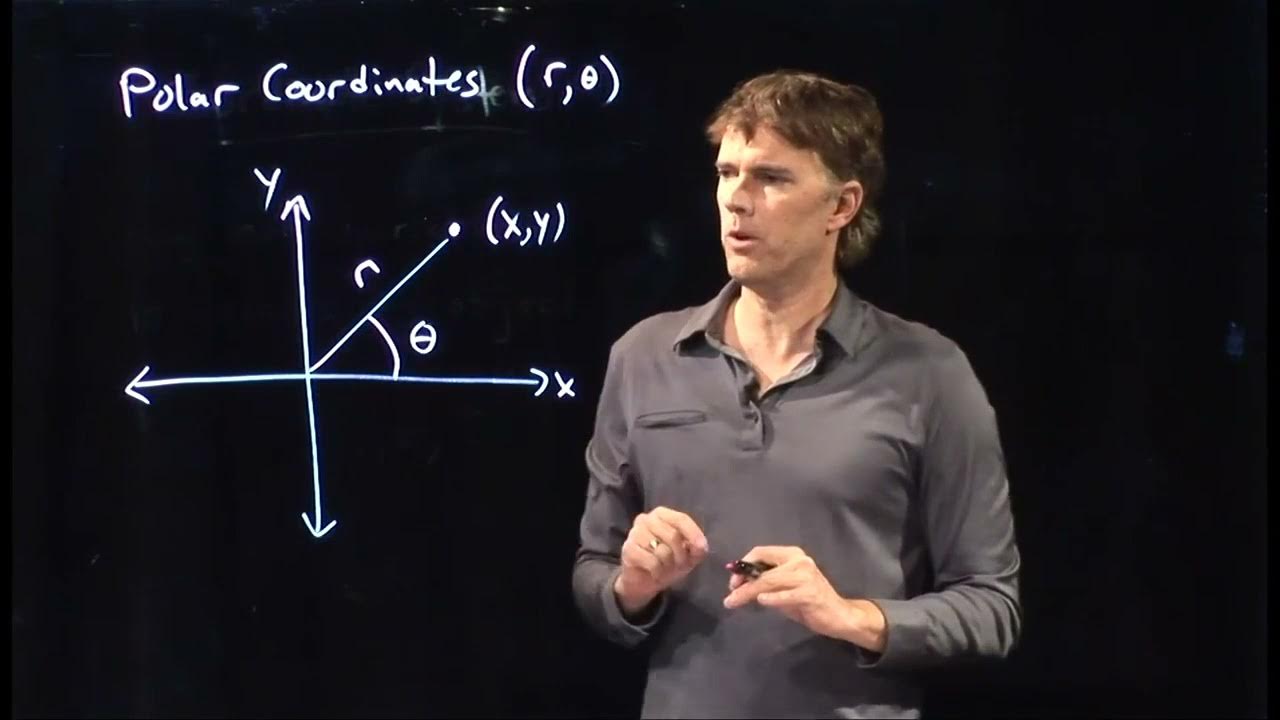
-In Cartesian coordinates, a function is typically represented as y = f(x), where y is a function of x. In polar coordinates, the function is represented as r = f(θ), where r, the distance from the origin, is a function of θ, the angle from the x-axis.
What is the significance of the angle θ in polar coordinates?
-The angle θ in polar coordinates represents the angle measured from the positive x-axis to the point in question. It is one of the two variables used to define a point in the polar coordinate system, the other being the radial distance r.
How does the script describe the process of converting from polar to rectangular coordinates?
-The script does not provide explicit steps for converting from polar to rectangular coordinates but suggests that it will discuss this process, indicating that it is an important aspect of understanding polar equations.
What is the polar equation for a circle as presented in the script?
-The polar equation for a circle given in the script is r = 2, which means that the distance from the origin (r) is constant, regardless of the angle θ, resulting in a circular shape.
Why are polar coordinates particularly useful for problems involving circles?
-Polar coordinates are particularly useful for problems involving circles because they simplify the representation of circular shapes. In polar coordinates, a circle with a radius r is represented by the simple equation r = constant, which is more straightforward than the Cartesian representation.
What does it mean if r is a constant in a polar equation?
-If r is a constant in a polar equation, it means that the distance from the origin does not change as the angle θ varies. This results in a shape that is symmetrical about the origin, such as a circle.
Can polar equations always be converted back to rectangular equations?
-Yes, polar equations can be converted back to rectangular equations, and vice versa. This conversion is useful for solving problems and understanding the relationship between different coordinate systems.
What is an example of a polar equation that does not exhibit circular symmetry?
-The script does not provide a specific example of a polar equation without circular symmetry. However, any polar equation where r is not a constant function of θ would not necessarily exhibit circular symmetry.
How does the script illustrate the concept of functions in polar coordinates?
-The script illustrates the concept of functions in polar coordinates by comparing them to functions in Cartesian coordinates and providing the example of a polar equation for a circle, emphasizing the role of r as a function of θ.

Outlines

00:00

📚 Introduction to Polar Coordinates and Equations

This paragraph introduces the concept of polar coordinates as an alternative to the Cartesian coordinate system. It explains that while Cartesian coordinates use x and y to define a point, polar coordinates use r (the distance from the origin) and theta (the angle measured from the x-axis). The paragraph also mentions that polar coordinates are particularly useful for problems involving circles due to their inherent circular symmetry. The speaker begins by contrasting regular functions in Cartesian coordinates, where y is a function of x, with functions in polar coordinates, where r is a function of theta. An introductory section is promised to show how to visualize these polar functions and convert between polar and rectangular formats.

Mindmap

Keywords

💡Advanced Calculus

Advanced Calculus is a higher-level branch of mathematics that deals with the study of functions, limits, derivatives, and integrals. In the context of the video, it signifies the complexity of the mathematical concepts being discussed, which are suitable for students who have a foundational understanding of calculus and are ready to delve into more complex topics such as polar coordinates and polar equations.

💡Polar Coordinates

Polar coordinates are a two-dimensional coordinate system where each point is determined by a distance from a reference point and an angle from a reference direction. In the video, polar coordinates are introduced as an alternative to the Cartesian coordinate system, allowing for a different perspective in analyzing and graphing functions, particularly those with circular symmetry.

💡Cartesian Coordinates

Cartesian Coordinates refer to the traditional coordinate system where points are defined by their x (horizontal) and y (vertical) values relative to two perpendicular axes. The video script contrasts Cartesian coordinates with polar coordinates, explaining that while Cartesian uses x and y values, polar coordinates use radial distance (r) and angular position (theta).

💡Function

In mathematics, a function is a relation between a set of inputs and a set of permissible outputs with the property that each input is related to exactly one output. The video script uses the concept of a function to transition from Cartesian to polar coordinates, explaining how a function can be defined differently in polar coordinates as r being a function of theta.

💡Theta (θ)

Theta, often symbolized by the Greek letter θ, represents the angle in polar coordinates measured from the positive x-axis. In the video, theta is one of the key components of polar coordinates, and it is used to define the angular position of a point in the polar coordinate system.

💡R (r)

In polar coordinates, r represents the radial distance from the origin (or pole) to the point in question. The video script explains that in polar equations, r can be a function of theta, which means the distance from the origin changes as the angle theta varies.

💡Polar Equations

Polar Equations are equations that describe the relationship between the radial distance (r) and the angle (theta) in polar coordinates. The video script discusses polar equations as a way to represent geometric shapes and patterns, especially those with circular symmetry, in a polar coordinate system.

💡Conversion

Conversion in the context of the video refers to the process of translating between Cartesian and polar coordinate systems. The script mentions the importance of understanding how to convert formats, which is crucial for solving problems that involve both coordinate systems.

💡Circular Symmetry

Circular Symmetry refers to the property of a shape or pattern that is symmetrical about a circle. The video script highlights that polar coordinates are particularly useful for problems involving circular symmetry because they can simplify the representation of such shapes.

💡Polar Graph

A Polar Graph is a graphical representation of a polar equation, where the radial distance (r) is plotted against the angle (theta). The video script introduces the concept of drawing polar graphs to visualize the shapes and patterns described by polar equations, such as the example of a circle where r is a constant.

Highlights

Introduction to polar coordinates as an alternative to Cartesian coordinates.

Explanation of Cartesian coordinates with function f(x, y).

Transition to discussing functions in polar coordinates.

Polar coordinates defined with r as a function of theta.

Analogous relationship between Cartesian and polar functions.

Description of r as the distance from the origin and theta as the angle from the x-axis.

Patterns traced in the XY plane with polar coordinates often exhibit circular symmetry.

Introduction to converting formats between polar and rectangular coordinates.

Example of a polar equation representing a circle.

Polar equation of a circle given as r = 2.

Explanation of r being constant in the polar equation for a circle.

Implication of r being constant results in a circular shape.

General behavior of r as a function of theta varies depending on the equation.

In the given circle example, r does not change with theta.

The simplicity of the polar equation for a circle.

Importance of polar coordinates in solving circular problems.

Transcripts

Browse More Related Video

Polar coordinates 2 | Parametric equations and polar coordinates | Precalculus | Khan Academy

Polar coordinates 1 | Parametric equations and polar coordinates | Precalculus | Khan Academy

Polar Coordinates Basic Introduction, Conversion to Rectangular, How to Plot Points, Negative R Valu

Polar Coordinate System | Physics with Professor Matt Anderson | M3-02

AP Calculus BC Lesson 9.7

Calculus 2: Polar Coordinates (Video #30) | Math with Professor V

Lesson 14 - Polar Equations (Calculus 2 Tutor)

Takeaways

Q & A

What is the main topic discussed in this section of the advanced calculus tutorial?

What is the difference between a function in Cartesian coordinates and a function in polar coordinates?

What is the significance of the angle θ in polar coordinates?

How does the script describe the process of converting from polar to rectangular coordinates?

What is the polar equation for a circle as presented in the script?

Why are polar coordinates particularly useful for problems involving circles?

What does it mean if r is a constant in a polar equation?

Can polar equations always be converted back to rectangular equations?

What is an example of a polar equation that does not exhibit circular symmetry?

How does the script illustrate the concept of functions in polar coordinates?