Video 49 - Voss Weyl Formula

Tensor Calculus
5 Jun 202214:11
EducationalLearning
32 Likes 10 Comments

TLDRIn this 49th installment of the tensor calculus series, the Vosvile formula is derived, offering a more practical alternative for calculating the divergence and Laplacian in various coordinate systems. The script begins by revisiting the covariant derivative and divergence expression, then introduces a new formula that simplifies the process by eliminating Christoffel symbols. The Vosvile formula is particularly useful for theoretical tensor calculus and for evaluating divergence and Laplacian in specific coordinate systems, making it more efficient with fewer terms involved. The video concludes with the application of this formula to simplify the evaluation of the Laplacian operator, setting the stage for the next video where these concepts will be applied to sample coordinate systems.

Takeaways
  • 📚 The video is part of a series on tensor calculus, focusing on deriving the Voss-Ziegler formula.
  • 🔍 The Voss-Ziegler formula provides an alternative method for evaluating divergence and Laplacian, which is more convenient in certain coordinate systems.
  • 📐 The script revisits the divergence of a vector and the covariant derivative, which was introduced in an earlier video.
  • 🔑 The video derives a new form for the divergence that eliminates the need for Christoffel symbols, simplifying the expression to three terms.
  • 🧩 The Voss-Ziegler formula is obtained by factoring out terms and applying the product rule to the partial derivative of a product of terms.
  • 🌐 The divergence formula is simplified to 1 over the square root of the metric tensor times the partial derivative of the product of the square root of the metric tensor and the vector component.
  • 📉 The new divergence expression is advantageous for specific coordinate systems, reducing the number of terms needed for calculation.
  • 🌀 The Laplacian operator is discussed as the divergence of the gradient's contravariant component, and its evaluation can also be simplified using the Voss-Ziegler formula.
  • 📝 The script emphasizes the importance of using the right formula for theoretical work in tensor calculus versus practical calculations in specific coordinate systems.
  • 🔄 The next video will apply these formulas to sample coordinate systems to demonstrate the divergence and Laplacian in those contexts.
Q & A

  • What is the main topic of this video in the series on tensor calculus?

    -The main topic of this video is the derivation of the Voss-Zhao formula, which provides an alternative method for evaluating the divergence and Laplacian in various coordinate systems.

  • Why was the divergence formula derived in video 28 not used for each sample coordinate system?

    -The divergence formula from video 28 was not used for each sample coordinate system because it is not particularly useful for that purpose. A more convenient formula, the Voss-Zhao formula, is introduced in this video for evaluating the divergence in sample coordinate systems.

  • What is the significance of the covariant derivative in the context of this video?

    -The covariant derivative is significant as it is the starting point for the discussion on the divergence of a vector field and is integral to the derivation of the Voss-Zhao formula.

  • How does the Voss-Zhao formula simplify the evaluation of the divergence?

    -The Voss-Zhao formula simplifies the evaluation of the divergence by eliminating the Christoffel symbols from the expression, reducing the number of terms needed to compute the divergence in a specific coordinate system.

  • What is the role of the volume element in the Voss-Zhao formula?

    -The volume element plays a crucial role in the Voss-Zhao formula as it is used to factor out terms and simplify the expression, making it easier to compute the divergence and Laplacian in various coordinate systems.

  • How does the video script relate the Voss-Zhao formula to the product rule in calculus?

    -The script relates the Voss-Zhao formula to the product rule by showing that the formula is equivalent to the partial derivative of a product of terms, specifically the square root of the metric tensor times the vector component.

  • What is the difference between the Voss-Zhao formula and the original divergence formula in terms of theoretical use?

    -The original divergence formula is more succinct and easier to work with theoretically when not dealing with a specific coordinate system, while the Voss-Zhao formula is more effective for evaluating the divergence in individual coordinate systems.

  • How does the script connect the Laplacian operator to the Voss-Zhao formula?

    -The script connects the Laplacian operator to the Voss-Zhao formula by explaining that the Laplacian is the divergence of the gradient's contravariant component, and thus the Voss-Zhao formula can be used to simplify the evaluation of the Laplacian.

  • What is the purpose of the next video in the series after this one?

    -The purpose of the next video is to apply the Voss-Zhao formula to each of the sample coordinate systems to see what the divergence and the Laplacian look like in those specific cases.

  • Why is the Voss-Zhao formula considered more convenient for evaluating the Laplacian in specific coordinate systems?

    -The Voss-Zhao formula is considered more convenient for evaluating the Laplacian in specific coordinate systems because it simplifies the expression to fewer terms compared to the original formula, making it easier to compute.

Outlines
00:00
📚 Introduction to the Vosvile Formula

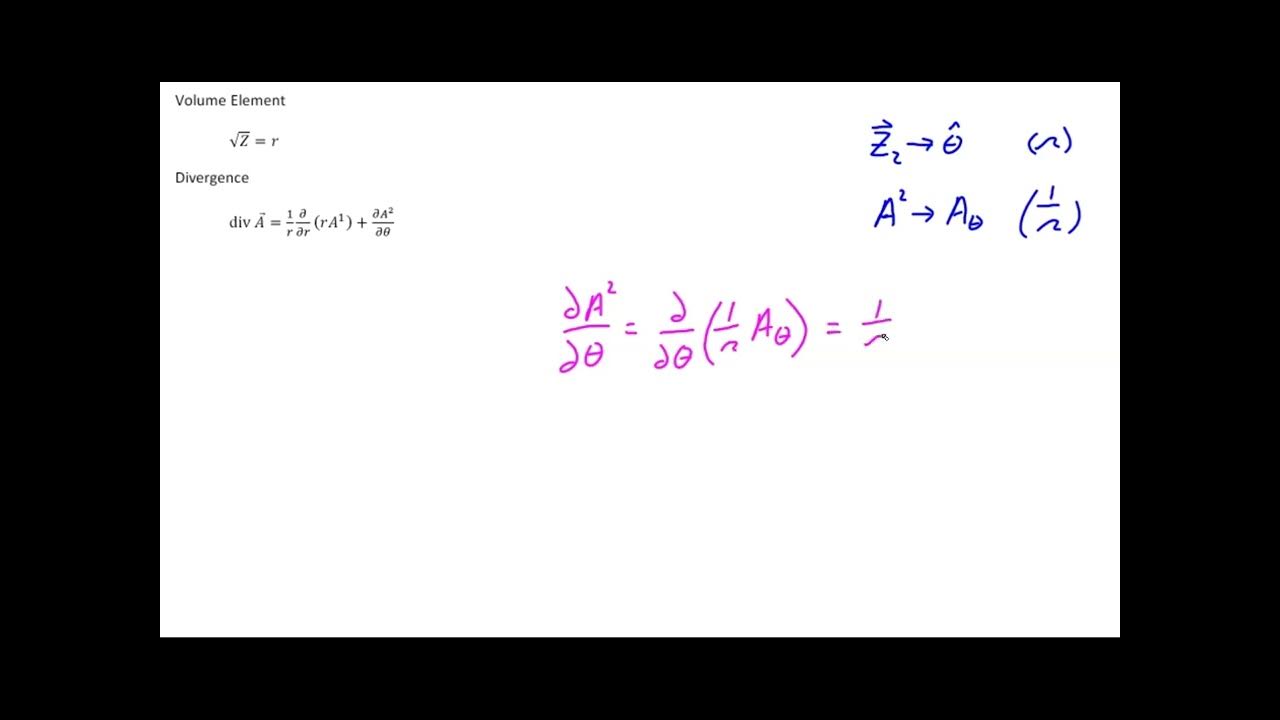
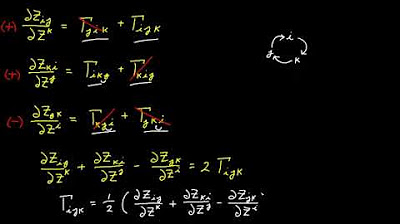
This paragraph introduces the Vosvile formula as an alternative method for evaluating the divergence and Laplacian in tensor calculus. The speaker recalls the development of the divergence expression in a previous video and explains that while the current formula is concise, it's not practical for calculating divergence across different coordinate systems. The paragraph sets the stage for deriving a new, more convenient form of the divergence, starting with the covariant derivative of a vector and incorporating Christoffel symbols.

05:02
🔍 Deriving the Vosvile Formula for Divergence

The speaker proceeds to derive the Vosvile formula by expanding the covariant derivative expression and rearranging terms to isolate the Christoffel symbol. By recognizing a pattern in the partial derivative of the volume element, the formula is simplified to eliminate the Christoffel symbol, resulting in a more straightforward expression for divergence. This new formula is highlighted for its practicality in calculating divergence within specific coordinate systems, contrasting it with the original formula's theoretical utility.

10:02
🌐 Applying the Vosvile Formula to the Laplacian Operator

Building on the Vosvile formula for divergence, the speaker explains its application to the Laplacian operator. The Laplacian is defined as the divergence of the gradient's contravariant component. By substituting the gradient's components into the Vosvile formula, a simplified expression for the Laplacian is achieved. The paragraph emphasizes the formula's convenience for evaluating the Laplacian in specific coordinate systems, despite its initial appearance of complexity, and contrasts it with the more theoretical, concise form used in tensor calculus equations.

Mindmap
Keywords
💡Tensor Calculus
Tensor calculus is a branch of mathematics that deals with the manipulation of tensors, which are generalizations of scalars, vectors, and higher-dimensional arrays. In the context of the video, tensor calculus is the overarching theme, as the script discusses the derivation of formulas related to the divergence and Laplacian within this mathematical framework. The video aims to provide an alternative method for evaluating these operations, emphasizing the importance of tensor calculus in advanced physics and engineering applications.
💡Vosvile Formula
The Vosvile formula, as introduced in the script, is an alternative expression for calculating the divergence of a vector field. It is presented as a more convenient formula for evaluating the divergence in various coordinate systems. The script derives this formula and contrasts it with the traditional method, highlighting its utility in simplifying calculations within the scope of tensor calculus.
💡Divergence
Divergence is a measure in vector calculus that describes the magnitude of a vector field's source or sink at a given point. In the script, the divergence of a vector field is initially discussed using a covariant derivative approach. The Vosvile formula is then derived to offer a more practical method for calculating divergence in specific coordinate systems, which is crucial for understanding fluid dynamics and electromagnetism.
💡Covariant Derivative
The covariant derivative is a generalization of the partial derivative that is used in tensor calculus to take into account the curvature of the space in which the tensor field is defined. In the video script, the covariant derivative is used to express the divergence of a vector field and is integral to the derivation of the Vosvile formula, illustrating its role in advanced mathematical physics.
💡Christoffel Symbols
Christoffel symbols are used in differential geometry and general relativity to describe the torsion and curvature of a manifold. In the context of the video, Christoffel symbols are part of the covariant derivative expression for the divergence. The script discusses how these symbols can be eliminated in the Vosvile formula, simplifying the calculation process.
💡Volume Element
A volume element in a mathematical context is a small portion of space that is used in integral calculus to represent the volume over which an integration is performed. In the script, the volume element is used in the derivation of the Vosvile formula, where it is manipulated to simplify the expression for the divergence, demonstrating its importance in the calculus of variations and related fields.
💡Partial Derivative
A partial derivative is a derivative of a function of multiple variables with respect to one of those variables, while the others are held constant. In the script, partial derivatives are used extensively in the derivation of the Vosvile formula and in the discussion of the divergence and Laplacian, showing their fundamental role in calculus and its applications.
💡Laplacian
The Laplacian is a differential operator that is used to measure the divergence of a gradient field. It is a second-order differential operator and is important in fields such as potential theory and fluid dynamics. In the video script, the Laplacian is discussed in relation to the covariant derivative and the Vosvile formula, highlighting its significance in tensor calculus.
💡Contravariant Component
In tensor calculus, a contravariant component is a component of a tensor that is raised to a power, as opposed to a covariant component, which is lowered. The script mentions contravariant components in the context of the gradient and the Laplacian, where they are used to construct expressions that are then simplified using the Vosvile formula.
💡Scalar Value
A scalar value is a single numerical value, as opposed to a vector or tensor, which has both magnitude and direction. In the script, scalar values are produced by contractions in tensor expressions, such as the covariant derivative, which is crucial for deriving scalar quantities like the divergence and Laplacian.
💡Product Rule
The product rule is a fundamental principle in calculus that states how to differentiate a product of two functions. In the script, the product rule is applied when taking the partial derivative of a product of terms, specifically in the context of the Vosvile formula, to simplify the expression for the divergence.
Highlights

Introduction of the Voss-Vial formula as an alternative method for evaluating divergence and Laplacian.

Explanation of the divergence of a vector field and its relation to the covariant derivative.

Derivation of an alternate form for the divergence that is more useful for sample coordinate systems.

Expansion of the covariant derivative of a vector component and its significance.

Renaming of dummy indexes in tensor calculus expressions for clarity.

Utilization of the partial derivative of the volume element in the derivation process.

Simplification of the Christoffel symbol to facilitate the evaluation of divergence.

Derivation of the Voss-Vial formula by applying the product rule to the divergence expression.

Comparison between the original divergence formula and the Voss-Vial formula in terms of convenience.

Discussion on the practicality of the Voss-Vial formula for specific coordinate systems.

Introduction of the Laplacian operator and its relation to the covariant derivative of the gradient.

Expansion of the Laplacian operator using the contravariant component of the gradient.

Application of the Voss-Vial formula to simplify the evaluation of the Laplacian.

Explanation of the advantages of using the Voss-Vial formula for theoretical tensor calculus expressions.

Comparison of the Laplacian's expression in different coordinate systems using the Voss-Vial formula.

Preview of the next video's content, which will apply these formulas to sample coordinate systems.

Transcripts

Browse More Related Video

Video 50 - Voss Weyl Examples

Video 30 - Christoffel Symbol Evaluation

Tensor Calculus Lecture 7d: The Voss-Weyl Formula

Video 31 - Christoffel Symbol Examples

Tensor Calculus for Physics Ep. 14 | Covariant Curl

Explicit Laplacian formula

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Tensor CalculusVoss-Vial FormulaDivergenceLaplacianCovariant DerivativeChristoffel SymbolVolume ElementPartial DerivativeVector FieldsMathematics Tutorial