Polar Coordinate System | Physics with Professor Matt Anderson | M3-02

Physics with Professor Matt Anderson
1 Nov 202104:15
EducationalLearning
32 Likes 10 Comments

TLDRIn this informative transcript, Professor Anderson introduces the concept of polar coordinates, a crucial coordinate system in physics, focusing on its two-dimensional application. He explains the transition from Cartesian coordinates to polar by defining a point in terms of its distance from the origin (r) and its angle (theta) relative to the x-axis. The relationship between Cartesian (x, y) and polar coordinates (r, theta) is established through trigonometric functions and Pythagorean theorem. Additionally, a sign convention for theta is discussed, where counterclockwise rotation is positive and clockwise is negative, providing a foundational understanding of polar coordinates.

Takeaways
  • 📍 The primary focus is on two-dimensional polar coordinates, which are an alternative to the Cartesian coordinate system for 2D motion analysis.
  • 🔢 In polar coordinates, the position of a point is defined by the radius (r) from the origin and the angle (θ) with respect to the x-axis.
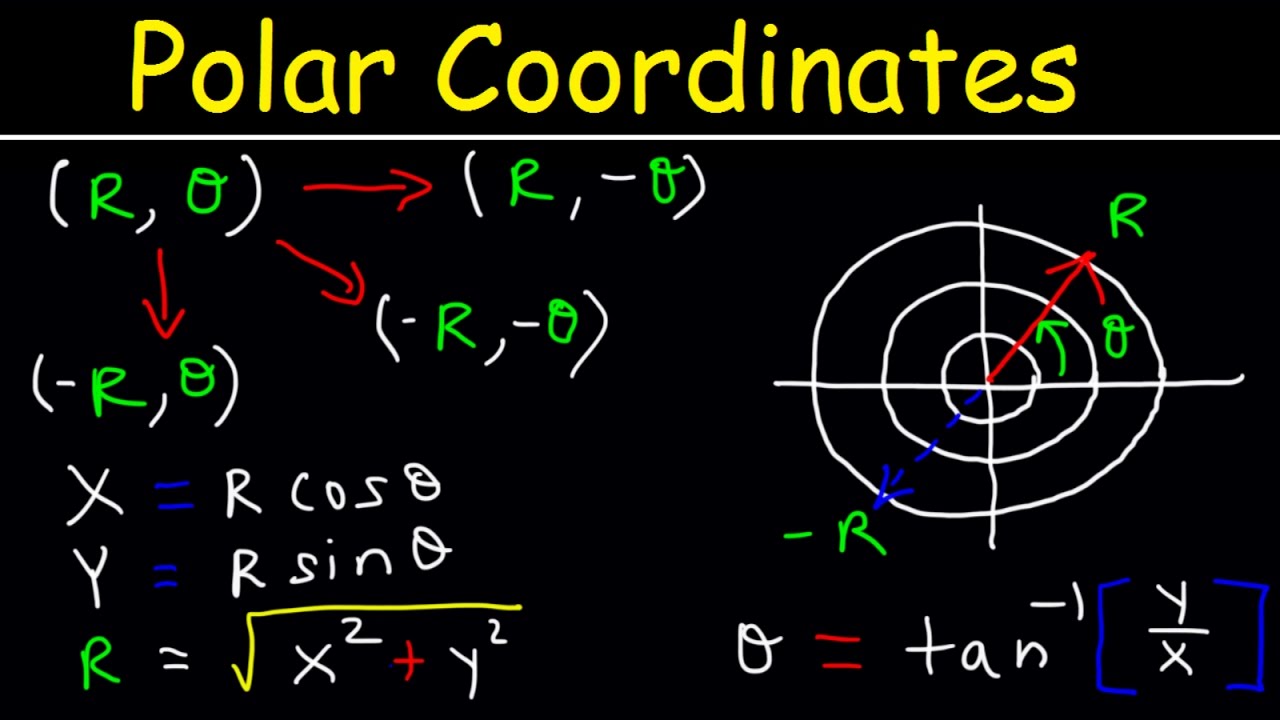
  • 📐 The relationship between Cartesian (x, y) and polar (r, θ) coordinates is given by the equations: x = r * cos(θ) and y = r * sin(θ).
  • 🌐 The tangent of the angle θ is represented by the ratio y/x in the context of the right triangle formed by the point, the origin, and the x-axis.
  • 🔍 Pythagoras' theorem relates the Cartesian and polar coordinates through the equation: r^2 = x^2 + y^2.
  • 📈 The sign convention for angles in polar coordinates is such that positive angles (counterclockwise rotation) are greater than zero, and negative angles (clockwise rotation) are less than zero.
  • 🌟 The trigonometric relationships for the right triangle in polar coordinates are fundamental for understanding the conversion between Cartesian and polar coordinate systems.
  • 👤 The script is part of a lecture by Professor Anderson, aiming to familiarize students with the concept and application of polar coordinates in physics.
  • 📚 The cylindrical and spherical coordinate systems are mentioned as higher-dimensional extensions of the polar coordinate system.
  • 🎓 Understanding the conversion between coordinate systems is crucial for advanced studies in physics and engineering.
Q & A

  • What is the primary focus of the coordinate system discussed in the transcript?

    -The primary focus is on two-dimensional polar coordinates, which are an alternative way to represent points in a plane other than the Cartesian coordinate system.

  • How many dimensions does the Cartesian coordinate system typically deal with in the context of the motion discussed in the transcript?

    -In the context of the motion discussed, the Cartesian coordinate system typically deals with two dimensions.

  • What are the two variables used to define a point in polar coordinates?

    -The two variables used to define a point in polar coordinates are the distance from the origin, denoted as r, and the angle with respect to the x-axis, denoted as theta.

  • What is the significance of the angle theta in polar coordinates?

    -Theta represents the orientation of the line connecting the point to the origin with respect to the x-axis, and it helps determine the location of the point on a circular path of a given radius.

  • How is the relationship between Cartesian coordinates (x, y) and polar coordinates (r, theta) expressed mathematically?

    -The relationship is expressed through the equations: x = r * cos(theta) and y = r * sin(theta), derived from the right triangle formed by the point, the origin, and the x-axis.

  • What is the Pythagorean relationship between r, x, and y in polar coordinates?

    -The Pythagorean relationship is given by the equation: r^2 = x^2 + y^2, which represents the hypotenuse of the right triangle formed by the polar coordinates and the Cartesian coordinates.

  • What is the significance of the tangent of theta in the context of the triangle formed by polar coordinates?

    -The tangent of theta is given by the ratio y/x, which is the slope of the line connecting the point to the origin and is a trigonometric function that relates the y-coordinate to the x-coordinate.

  • What is the sign convention for angles theta in polar coordinates?

    -The sign convention states that angles that go counterclockwise are positive (theta > 0), and angles that go clockwise are negative (theta < 0).

  • How can the cosine of theta be used in the context of the right triangle formed by polar coordinates?

    -The cosine of theta is used to find the x-coordinate of a point in polar coordinates, given by the equation x = r * cos(theta), where cos(theta) represents the adjacent side over the hypotenuse in the right triangle.

  • How can the sine of theta be used in the context of the right triangle formed by polar coordinates?

    -The sine of theta is used to find the y-coordinate of a point in polar coordinates, given by the equation y = r * sin(theta), where sin(theta) represents the opposite side over the hypotenuse in the right triangle.

  • What is the relevance of the trigonometric functions in the conversion between Cartesian and polar coordinates?

    -The trigonometric functions (cosine, sine, and tangent) are essential for converting between Cartesian (x, y) and polar (r, theta) coordinates, as they relate the position and orientation of a point in the plane using the right triangle formed by the point, the origin, and the x-axis.

Outlines
00:00
📐 Introduction to Polar Coordinates

In this paragraph, Professor Anderson introduces the concept of polar coordinates, emphasizing its relevance in two-dimensional motion analysis. He explains that while Cartesian coordinates are commonly used, polar coordinates offer an alternative representation focusing on distance (r) from the origin and orientation (theta) with respect to the x-axis. The paragraph outlines the relationship between Cartesian (x, y) and polar (r, theta) coordinates, highlighting the trigonometric functions that connect them: r cosine theta equals x, r sine theta equals y, and tangent of theta equals y/x. Additionally, the paragraph touches on the Pythagorean theorem's role in this context and the sign convention for angles, where counterclockwise rotation is positive and clockwise is negative.

Mindmap
Keywords
💡Polar Coordinates
Polar coordinates are a two-dimensional coordinate system in which each point is determined by a distance from a reference point, called the origin, and an angle from a reference direction, usually the positive x-axis. In the context of the video, polar coordinates are introduced as an alternative way to represent points in a Cartesian coordinate system, particularly useful for problems involving circular or radial symmetry.
💡Cartesian Coordinate System
The Cartesian coordinate system, also known as the直角坐标系 (rectangular coordinate system), is a coordinate system named after René Descartes. It is the most common system used in plane geometry, where each point is represented by an (x, y) coordinate pair, with x and y being the horizontal and vertical distances from a fixed point called the origin, respectively. In the video, the Cartesian system is contrasted with polar coordinates to highlight the differences in how points are represented.
💡Cylindrical Coordinate System
The cylindrical coordinate system is a three-dimensional coordinate system that extends the two-dimensional polar coordinate system. It is used to solve problems involving cylindrical symmetry, such as those related to cylindrical objects or waves. In the video, the cylindrical coordinate system is mentioned as one of the three coordinate systems important in physics, alongside the Cartesian and spherical coordinate systems.
💡Spherical Coordinate System
The spherical coordinate system is a three-dimensional coordinate system that is primarily used to solve problems involving spherical symmetry. It uses three coordinates: the radial distance from a fixed point (the origin), the polar angle (similar to theta in two dimensions but extended to three dimensions), and the azimuthal angle. The video mentions this system as one of the three coordinate systems crucial for advanced physics, suggesting its importance in three-dimensional spatial analysis.
💡r (radius)
In the context of polar coordinates, r represents the radial distance from the origin to a point in the plane. It is a scalar quantity that defines how far away the point is from the reference point without considering direction. The video explains that r alone is not sufficient to pinpoint a location since it only provides the distance, not the orientation.
💡theta (angle)
Theta (θ) is the angular coordinate in polar coordinates that represents the orientation of a point with respect to the reference direction, typically the positive x-axis. It is a measure in degrees or radians that describes the angle from the reference direction to the line segment connecting the origin and the point. In the video, theta is introduced as the second variable needed to fully describe a point in polar coordinates, complementing the radial distance r.
💡Trigonometric Relationships
Trigonometric relationships refer to the fundamental relationships between the angles and sides of a right triangle, which are encapsulated in the sine, cosine, and tangent functions. In the context of the video, these relationships are used to express the x and y Cartesian coordinates in terms of r and theta in polar coordinates. The professor explains that r cosine theta equals x, r sine theta equals y, and the tangent of theta is y over x.
💡Pythagorean Theorem
The Pythagorean theorem is a fundamental principle in geometry that states that in a right-angled triangle, the square of the length of the hypotenuse (the side opposite the right angle) is equal to the sum of the squares of the lengths of the other two sides. In the context of the video, the theorem is used to relate the polar coordinates r and theta to the Cartesian coordinates x and y, as it helps to establish the relationship between the radial distance and the components along the x and y axes.
💡Sign Convention
A sign convention is an agreed-upon set of rules for determining the signs of quantities in a mathematical or physical context. In the video, a specific sign convention is introduced for angles in polar coordinates, where angles measured counterclockwise from the positive x-axis are positive, and those measured clockwise are negative. This convention is crucial for correctly interpreting the orientation of points in polar coordinates.
💡Sokatoa
Sokatoa, mentioned in the video, appears to be a reference to the Pythagorean theorem, possibly due to a mispronunciation or a playful reference. The theorem is a fundamental concept in geometry and is used to relate the sides of a right-angled triangle. In the context of the video, it is used to derive the relationship between Cartesian and polar coordinates.
💡Transformation
Transformation in the context of the video refers to the process of converting one type of coordinate system into another. Specifically, it involves translating points represented in Cartesian coordinates to their corresponding polar coordinates and vice versa. This process is essential for solving problems that are more naturally described in polar coordinates, such as those involving circular or radial motion.
Highlights

Introduction to polar coordinates as a coordinate system distinct from Cartesian coordinates.

Focus on two-dimensional motion and its representation in polar coordinates.

Definition of polar coordinates using two variables: distance from the origin (r) and angle (theta).

Explanation of the need for two variables in polar coordinates to specify a point's location.

Technical distinction between polar coordinates and the related spherical coordinate system.

Correlation of polar coordinates with the spherical coordinate system in two dimensions.

Description of the relationship between Cartesian coordinates (x, y) and polar coordinates (r, theta).

Use of trigonometric functions (cosine, sine, and tangent) to relate Cartesian and polar coordinates.

Explanation of the sign convention for theta, with positive angles indicating counterclockwise rotation and negative angles indicating clockwise rotation.

Application of the Pythagorean theorem to derive the relationship between r, theta, x, and y.

Discussion of the practical applications of polar coordinates in physics and geometry.

Use of the term 'sohcahtoa' as a mnemonic for trigonometric relationships.

Engagement of the audience with a question about the cosine of theta.

Engagement of the audience with a question about the sine of theta.

Engagement of the audience with a question about the tangent of theta.

Explanation of how the orientation of a line in polar coordinates (theta) is determined relative to the x-axis.

Discussion on the typical convention for the signs of theta in polar coordinates.

Transcripts

Browse More Related Video

Polar coordinates 1 | Parametric equations and polar coordinates | Precalculus | Khan Academy

An Example Using Polar Coordinates | Physics with Professor Matt Anderson | M3-09

Lesson 14 - Polar Equations (Calculus 2 Tutor)

Calculus 2: Polar Coordinates (Video #30) | Math with Professor V

Polar coordinates 2 | Parametric equations and polar coordinates | Precalculus | Khan Academy

Polar Coordinates Basic Introduction, Conversion to Rectangular, How to Plot Points, Negative R Valu

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
CoordinateSystemsPolarCoordinatesTrigonometryPhysicsConceptsMathematics2DSpaceThetaOrientationXandYTrigonometricRelationshipsCartesianToPolar