Mean Value Theorem

RH Mathematics
6 Sept 202107:52
EducationalLearning
32 Likes 10 Comments

TLDRThis video script introduces the Mean Value Theorem and Rolle's Theorem in the context of calculus. It explains that if a function is differentiable on a closed interval with the same y-values at the interval's endpoints, there must be at least one point where the derivative is zero, as per Rolle's Theorem. The Mean Value Theorem extends this idea, stating that there's at least one point where the slope of the tangent line equals the average rate of change between two points. The script provides a visual example of these concepts without proving the theorems, promising proofs in a linked video. It concludes with a question about identifying points where the tangent line is parallel to the secant line in a graph.

Takeaways
  • 📚 The Mean Value Theorem (MVT) is discussed in the context of calculus, following the introduction of the derivative.
  • 📉 Rolle's Theorem is introduced as a precursor to MVT, stating that for a differentiable function on a closed interval with the same y-values at the endpoints, there must be at least one point where the derivative is zero.
  • 📈 The script provides a visual explanation of Rolle's Theorem through a graph, illustrating the concept of a 'flat spot' on the graph where the tangent is horizontal.
  • 🔍 The video script does not provide a proof of Rolle's Theorem but mentions that it will be linked in the video description for those interested.
  • 📐 The Mean Value Theorem is then explained as a generalization of Rolle's Theorem, applicable to functions that do not necessarily have the same y-values at the endpoints of a closed interval.
  • 📊 The MVT guarantees the existence of at least one point in the interval where the slope of the tangent line equals the average rate of change of the function over that interval.
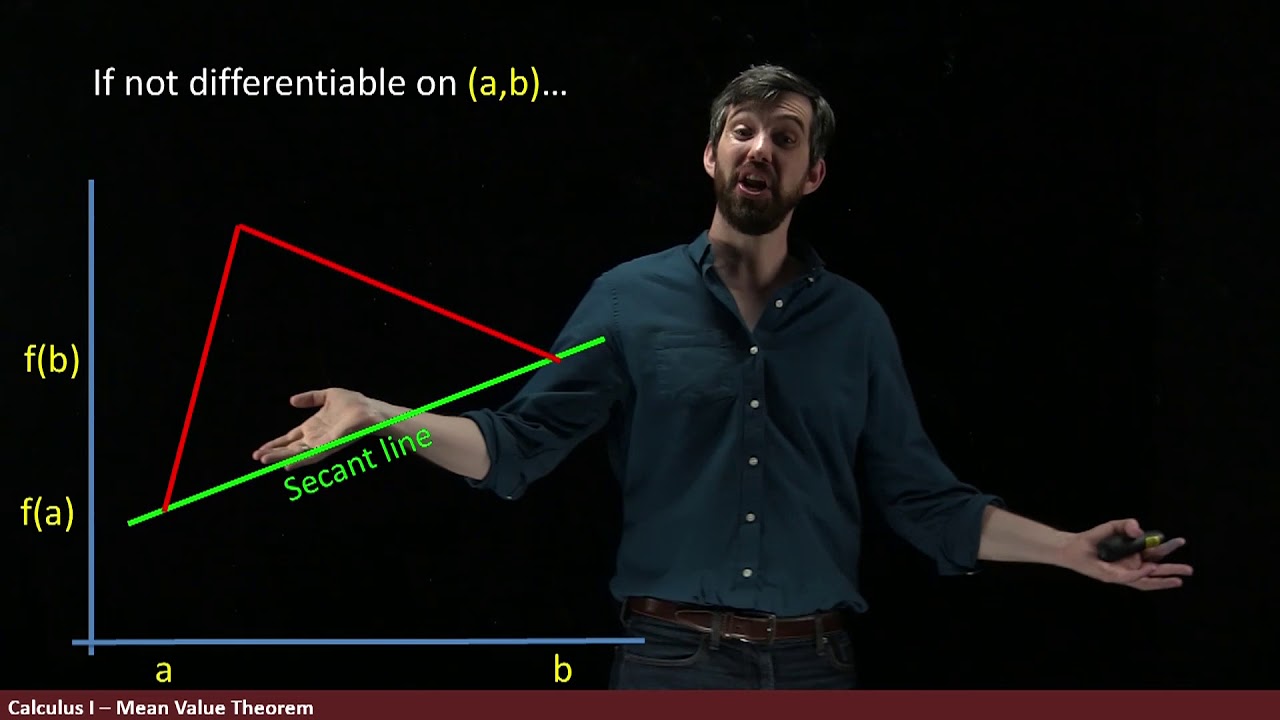
  • 📘 The script uses the secant line, the line connecting two points on the graph, to illustrate the average rate of change and its relation to the slope of the tangent line at some point c.
  • 📝 The Mean Value Theorem is not proven in the script, but the presenter assures that a proof will be available in another linked video.
  • 🔑 The script suggests a method for identifying intervals where the MVT might apply by looking for constant values of the function over an interval.
  • 📚 An example is given to demonstrate how to apply the MVT to a function, even without knowing the derivative rules, by visually identifying where the tangent line is parallel to the secant line.
  • 🔍 The final part of the script poses a question about the number of points satisfying the MVT for a given function graph, emphasizing understanding the theorem by its conclusion rather than its name.
Q & A

  • What is the Mean Value Theorem?

    -The Mean Value Theorem states that if a function is continuous on a closed interval [a, b] and differentiable on the open interval (a, b), then there exists at least one point 'c' in the interval (a, b) where the derivative of the function (the slope of the tangent line) is equal to the average rate of change of the function over the interval.

  • What is the condition for Rolle's Theorem to apply?

    -Rolle's Theorem applies to a differentiable function on a closed interval where the function values at the endpoints are the same, meaning f(a) = f(b).

  • What does it mean for a function to be differentiable?

    -A function is differentiable if it has a derivative at every point in its domain, implying it has a well-defined tangent line at each point without any vertical tangents, sharp corners, or discontinuities.

  • What is the algebraic expression for the conclusion of Rolle's Theorem?

    -The algebraic expression for Rolle's Theorem is f'(c) = 0, indicating there is at least one point 'c' in the interval (a, b) where the derivative of the function is zero.

  • How does the Mean Value Theorem relate to the secant line?

    -The Mean Value Theorem states that there is at least one point 'c' in the interval (a, b) where the slope of the tangent line to the function at 'c' is equal to the slope of the secant line connecting the points (a, f(a)) and (b, f(b)).

  • What is the average rate of change of a function over an interval?

    -The average rate of change of a function over an interval [a, b] is the difference in the function values at the endpoints divided by the difference in the x-values, expressed as (f(b) - f(a)) / (b - a).

  • Can the Mean Value Theorem be applied to a function that is not continuous?

    -No, the Mean Value Theorem requires the function to be continuous on the closed interval [a, b] and differentiable on the open interval (a, b).

  • What is the significance of the Mean Value Theorem in calculus?

    -The Mean Value Theorem is significant in calculus as it provides a connection between the derivative of a function and its average rate of change, ensuring the existence of a point where the instantaneous rate of change equals the average rate of change.

  • How can you identify a flat tangent on a graph of a differentiable function?

    -A flat tangent on a graph of a differentiable function can be identified by looking for a point where the derivative of the function is zero, indicating a horizontal tangent line.

  • What is the difference between the conclusion of Rolle's Theorem and the Mean Value Theorem?

    -While both theorems guarantee the existence of at least one point where the derivative has a certain value, Rolle's Theorem specifically states that this value is zero (f'(c) = 0) when the function values at the endpoints are the same, whereas the Mean Value Theorem states that the derivative at some point 'c' is equal to the average rate of change of the function over the interval.

Outlines
00:00
📚 Introduction to Rolle's Theorem

This paragraph introduces Rolle's Theorem, which is a fundamental concept in calculus. The theorem states that if a function is differentiable on a closed interval and has the same y-values at the endpoints, there must be at least one point 'c' within the interval where the derivative (slope) is zero, indicating a flat tangent. The presenter explains this with a visual example, illustrating the function's graph and the concept of a flat spot on the curve. The video also mentions that a proof of Rolle's Theorem will be provided in a separate video linked in the description.

05:01
📐 Mean Value Theorem: Understanding and Application

The second paragraph delves into the Mean Value Theorem, which is related to Rolle's Theorem but does not require the function values at the endpoints to be the same. The Mean Value Theorem guarantees that for a function defined on a closed interval, there exists at least one point 'c' where the slope of the tangent line equals the average rate of change over the interval, represented as f'(c) = (f(b) - f(a)) / (b - a). The presenter uses graphical examples to demonstrate this concept, showing how the secant line's slope can be parallel to the tangent line's slope at certain points. The video also hints at an example question related to the theorem, suggesting that understanding the theorem's conclusion is more important than its name for practical application.

Mindmap
Keywords
💡Mean Value Theorem
The Mean Value Theorem (MVT) is a fundamental concept in calculus that states if a function is continuous on a closed interval [a, b] and differentiable on the open interval (a, b), then there exists at least one point c in (a, b) where the derivative of the function at c is equal to the average rate of change of the function over the interval. In the video, the MVT is introduced to explain the relationship between the slope of the tangent line at some point c and the secant line connecting the endpoints of the interval.
💡Rolle's Theorem
Rolle's Theorem is a special case of the Mean Value Theorem that applies when a function is differentiable on the interval [a, b], continuous on [a, b], and when the function's values at the endpoints are equal, i.e., f(a) = f(b). The theorem states that there is at least one point c in (a, b) where the derivative of the function is zero. The script uses Rolle's Theorem to set the stage for the Mean Value Theorem, illustrating the concept with a function that has the same y-values at the edges of a closed interval.
💡Differentiable
A function is said to be differentiable at a point if it has a derivative at that point, meaning it has a non-vertical tangent and no sharp corners or discontinuities. In the context of the video, differentiability is a prerequisite for the Mean Value Theorem and Rolle's Theorem to hold true, as it ensures the function has a well-defined rate of change at every point in the interval.
💡Closed Interval
A closed interval in mathematics is an interval that includes its endpoints. In the video, the closed interval [a, b] is used to define the domain over which the function is continuous and differentiable, which is necessary for the application of both Rolle's Theorem and the Mean Value Theorem.
💡Open Interval
An open interval is an interval that does not include its endpoints. The video mentions the open interval (a, b) to specify the set of points where the function is differentiable, which is a condition for the Mean Value Theorem to apply, although the theorem itself guarantees the existence of a point within this interval where the derivative equals the average rate of change.
💡Derivative
The derivative of a function at a certain point represents the rate at which the function is changing at that point. It is a fundamental concept in calculus and is used in the video to describe the slope of the tangent line to the function at a point c, which is central to both Rolle's Theorem and the Mean Value Theorem.
💡Tangent Line
A tangent line is a straight line that touches a curve at a single point without crossing it. In the video, the tangent line is used to illustrate the instantaneous rate of change of the function at a specific point, which is compared to the average rate of change represented by the secant line in the context of the Mean Value Theorem.
💡Secant Line
A secant line is a straight line that intersects a curve at two or more points. The video explains that the slope of the secant line between the endpoints of an interval is the average rate of change of the function over that interval, and according to the Mean Value Theorem, there must be at least one point where the tangent line has the same slope as the secant line.
💡Average Rate of Change
The average rate of change of a function over an interval is calculated as the difference in the function's values at the endpoints of the interval divided by the difference in the x-values of those endpoints. In the video, this concept is used to describe the slope of the secant line and to compare it with the instantaneous rate of change at a point given by the derivative.
💡Flat Spot
A flat spot on a graph refers to a point where the derivative of the function is zero, indicating a horizontal tangent line. The video script mentions 'flat spot' in the context of Rolle's Theorem, where it is guaranteed that there is at least one such point on the graph of a differentiable function with equal y-values at the endpoints of a closed interval.
💡Table of Values
A table of values is a list that shows the input and output pairs of a function, which can be used to visualize the function's behavior. In the video, the script suggests using a table of values to identify intervals where the function's values are constant, which could indicate the presence of a flat spot and the application of Rolle's Theorem.
Highlights

Introduction to the Mean Value Theorem (MVT) and its connection to the derivative.

Rolle's Theorem as a prerequisite to MVT, stating the existence of at least one flat spot in a differentiable function on a closed interval with equal y-values at the edges.

Differentiability implies the absence of vertical tangents, sharp corners, and discontinuities.

Algebraic expression of Rolle's Theorem: f'(c) = 0 for some c between a and b.

Explanation of the possibility of multiple flat spots if the function is constant.

Announcement of a separate video for the proof of Rolle's Theorem.

Differentiation between Rolle's Theorem and MVT regarding the requirement of equal function values at endpoints.

MVT's guarantee of a value where the derivative equals the average rate of change over a closed interval.

Secant line definition and its slope calculation as the average rate of change.

MVT's assertion that there exists at least one c where the slope of the tangent line equals the slope of the secant line.

Illustration of the tangent line being parallel to the secant line in a graphical example.

Explanation of the potential for multiple points where the tangent line is parallel to the secant line.

Clarification that the Mean Value Theorem will not be proved in the video but linked in the description.

Introduction of an example involving a table of values to identify intervals with a flat tangent.

Demonstration of how to identify points where the derivative equals the average rate of change without knowing derivative rules.

A graphical example showing four instances where the tangent line is parallel to the secant line, satisfying MVT.

Conclusion of the video with a summary of the Mean Value Theorem and its practical application.

Transcripts

Browse More Related Video

Calculus 1 Lecture 3.2: A BRIEF Discussion of Rolle's Theorem and Mean-Value Theorem.

The MEAN Value Theorem is Actually Very Nice

Mean Value Theorem and Rolle's Theorem

Mean value theorem | Existence theorems | AP Calculus AB | Khan Academy

The Mean Value Theorem For Integrals: Average Value of a Function

2011 Calculus AB free response #4d | AP Calculus AB | Khan Academy

Rate This

5.0 / 5 (0 votes)

Thanks for rating:

Related Tags
Mean Value TheoremDerivative TheoryMathematicsEducational VideoCalculus ConceptsGraph AnalysisTangent LineSecant LineDifferentiable FunctionsRate of ChangeRolle's Theorem