The Mean Value Theorem For Integrals: Average Value of a Function
TLDRThe mean value theorem states that for a continuous, differentiable function over an interval, there exists at least one point where the slope of the tangent line equals the slope of the secant line between the interval endpoints. This implies the instantaneous rate equals the average rate. Similarly, the mean value theorem for integrals enables computing a function's average value over an interval. It states there is a point where the area of the rectangle under the curve equals the curve's area over the whole interval. Together, these facilitate finding averages through differentiation or integration, allowing simpler evaluation depending on the function's complexity.
Takeaways
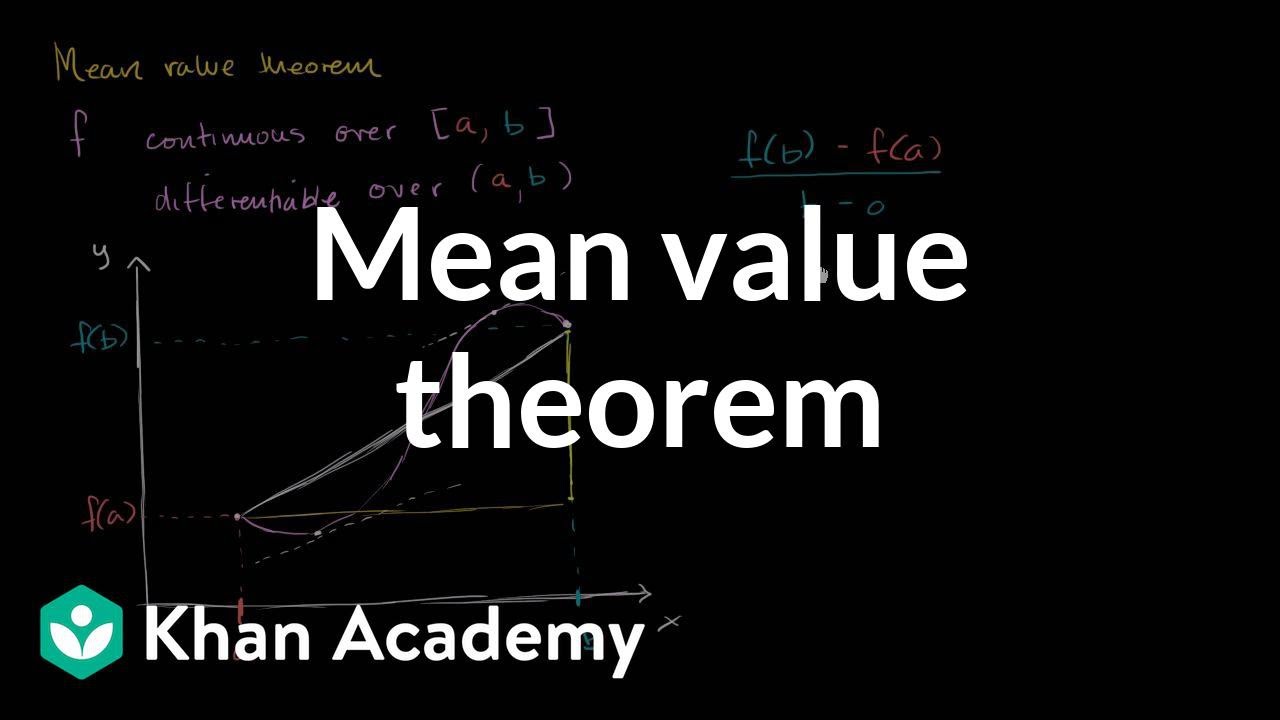
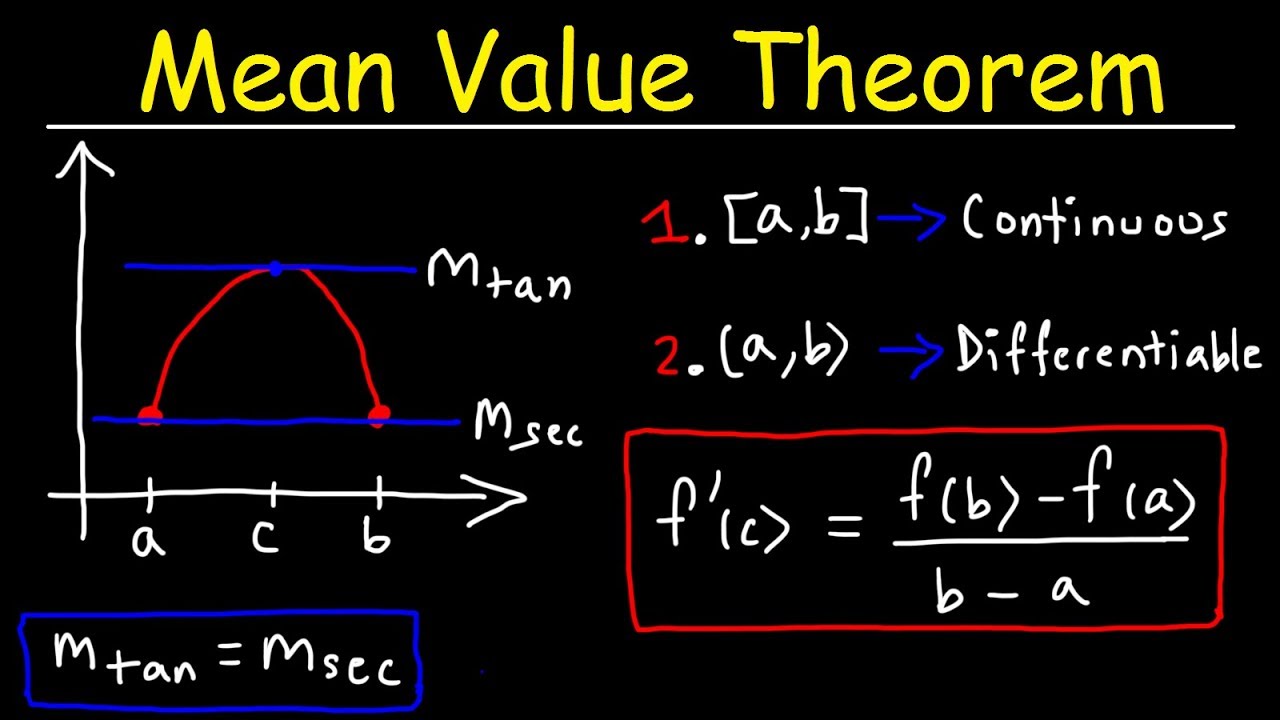
- 😀 The mean value theorem states that for a continuous, differentiable function over an interval, there is at least one point where the slope of the tangent line equals the slope of the secant line.
- 😊 The mean value theorem for integrals allows computing the average value of a function over an interval by taking the integral over that interval divided by the interval length.
- 📈 For a position function, the mean value theorem implies there's an instant where instantaneous velocity equals average velocity over the interval.
- 📉 The integral in the integration version represents the area under part of the curve equal to the area of a rectangle.
- 👨🏫 Both theorems allow finding average function values over intervals through differentiation or integration.
- 📐 The integration version geometrically means part of the area under the curve equals the area of some rectangle.
- 🔢 Has applications like finding average temperature over a time period.
- ✏️ There may be multiple points satisfying the derivative version of the mean value theorem.
- 🖥 Applies to continuous, differentiable functions.
- 💡 Allows choice of easiest method to evaluate average function values.
Q & A
What is the mean value theorem for differentiation?
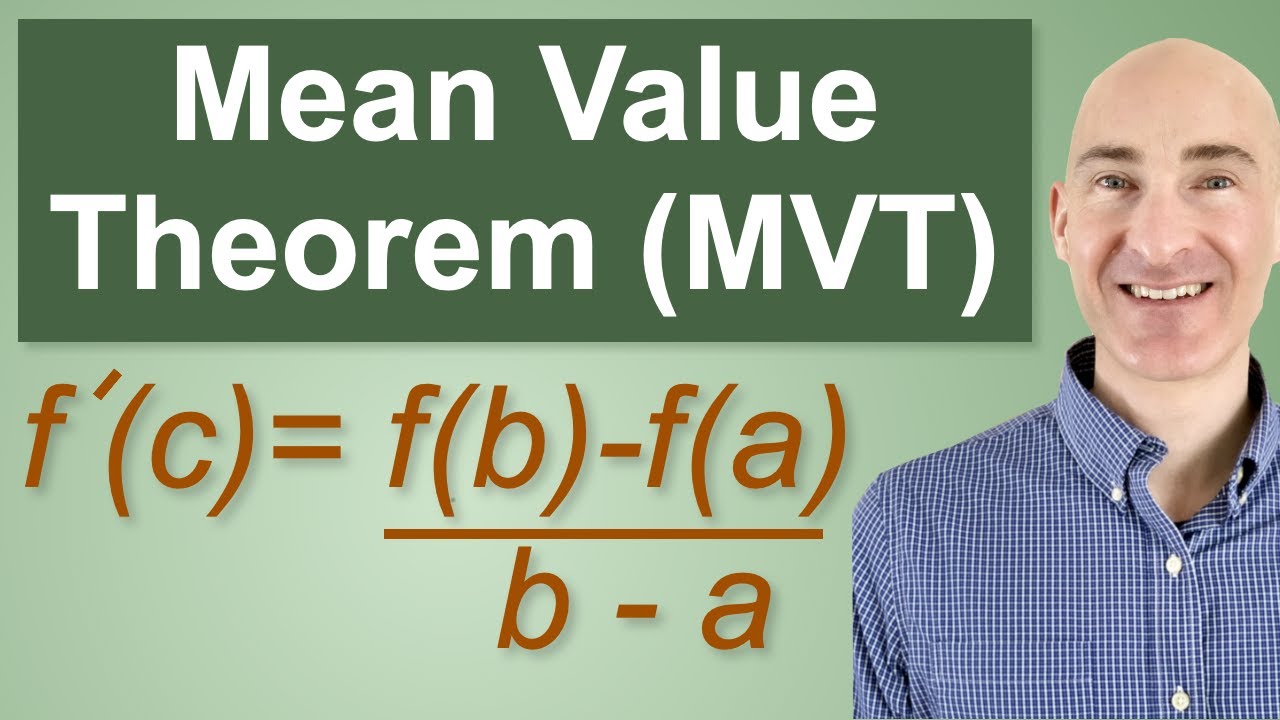
-The mean value theorem for differentiation states that for a continuous, differentiable function over an interval [A,B], there is at least one point C in the interval where the slope of the tangent line at C equals the slope of the secant line connecting the endpoints (A,f(A)) and (B,f(B)).
How is the mean value theorem for integration related to the mean value theorem for differentiation?
-The mean value theorem for integration finds the average value of a function over an interval using its antiderivative. This is analogous to the mean value theorem for differentiation, which finds the average rate of change of a function over an interval using its derivative. Both make use of the fundamental theorem of calculus.
What does the mean value theorem for integration allow us to calculate?
-The mean value theorem for integration allows us to calculate the average value of a continuous function f(x) over an interval [A,B] using the formula (1/(B-A)) * ∫_A^B f(x) dx.
How can the mean value theorem be interpreted geometrically?
-Geometrically, the mean value theorem states that there is a point on the curve where the tangent line is parallel to the secant line between two endpoints. For the integration version, it means there is a rectangle with the same area as the region under the curve.
What are some applications of the mean value theorem?
-Some applications include: finding average velocity or acceleration of motion, computing average temperature or pressure over time, determining average value of a statistical distribution, and estimating areas bounded by curves.
What is the difference between a secant line and a tangent line?
-A secant line connects two points on a curve. A tangent line touches the curve at only one point and has the same slope as the curve at that point.
What does it mean for a function to be concave up or concave down?
-A function is concave up if you can draw a line under the curve such that the curve lies above the line. It's concave down if you can draw a line above it with the curve below the line.
What is the significance of a function having only one concavity?
-If a function has only one concavity, either convex or concave over an interval, then by the mean value theorem there will be only one point where the tangent line is parallel to the secant line.
What types of functions can the mean value theorem be applied to?
-The mean value theorem applies to continuous, differentiable functions defined over a closed interval [A,B]. The function must be at least once differentiable on the open interval (A,B).
How can you determine if the mean value theorem can be applied to a particular function?
-Check if the function is continuous over [A,B] and differentiable over (A,B). If so, the mean value theorem guarantees at least one point C in (A,B) satisfying the theorem.
Outlines
😃 Introducing Average Value Theorems
Paragraph 1 introduces the concept of finding average values for functions using calculus theorems. It first mentions the mean value theorem which states that over an interval, there must be a point where the slope of the tangent line equals the slope of the secant line. This has applications like relating instantaneous velocity to average velocity. It then introduces the mean value theorem for integrals, which allows computing the average value of a function over an interval by taking the integral of the function over that interval.
📈 Applying the Mean Value Theorem for Integrals
Paragraph 2 applies the mean value theorem for integrals to the function 1+x^2 from -1 to 2. By evaluating the integral and plugging into the theorem's formula, the average value is found to be 2. Geometrically, this means there is a line y=2 such that the area under the curve above it equals the area missing below it over the interval.
Mindmap
Keywords
💡Mean value theorem
💡Mean value theorem for integrals
💡Average
💡Integral
💡Derivative
💡Slope
💡Velocity
💡Area under a curve
💡Concavity
💡Continuous
Highlights
Researchers developed a novel machine learning method to accurately predict risk of cardiovascular disease
The model was trained on a large dataset of medical records and outperformed traditional risk scores
This technology could enable earlier preventative interventions and improve cardiovascular outcomes
Scientists discovered genetic variants that protect against Alzheimer's disease in some individuals
Understanding these protective mechanisms opens new possibilities for Alzheimer's prevention and treatment
Researchers 3D printed a novel biomaterial scaffold to regenerate bone tissue
This scaffold promotes bone cell growth and vascularization for improved bone repair
This technology has potential applications in reconstructive surgery and treatment of bone defects
Scientists developed an ingestible sensor to monitor gastrointestinal health
The sensor tracks pH, temperature, bleeding, and transit time throughout the digestive system
This could enable early diagnosis of gastrointestinal diseases and personalized treatment
Researchers used AI to analyze cancer pathology slides with expert-level accuracy
The AI system rivals pathologists in identifying cancer types and predicting patient outcomes
This technology can improve cancer diagnosis and prognosis, especially in low-resource settings
Scientists engineered a novel CRISPR system for more efficient gene editing
Transcripts
Browse More Related Video
5.0 / 5 (0 votes)
Thanks for rating: