The MEAN Value Theorem is Actually Very Nice
TLDRThe video script introduces the Mean Value Theorem (MVT), a fundamental concept in calculus that is intuitively appealing. The MVT posits that for a 'nice' function—differentiable on an open interval and continuous on a closed interval including its endpoints—there exists at least one point where the slope of the tangent line equals the slope of the secant line between two endpoints. The script explains that the theorem's conditions are necessary, as illustrated by examples where the function is not differentiable or continuous, leading to the failure of the theorem. It also highlights a special case of the MVT known as Rolle's Theorem, which occurs when the function values at the endpoints are equal, implying that the derivative at some point within the interval is zero. The video emphasizes the importance of these theorems in mathematical proofs, often starting with Rolle's Theorem before generalizing to the MVT.
Takeaways
- 📌 The Mean Value Theorem is an intuitive theorem that states for a 'nice' function, there exists a tangent line with the same slope as the secant line between two points on the function's graph.
- 📐 The secant line's slope is calculated as the rise over run between two points a and b on the function, while the tangent line's slope is the derivative at a specific point c.
- 🔍 A 'nice' function, as per the theorem, must be differentiable on the open interval (a, b) and continuous on the closed interval [a, b].
- 🚫 If a function is not differentiable on the interval, the Mean Value Theorem does not apply, as illustrated by a function with a corner point.
- 🔗 Continuity on the closed interval is also necessary; without it, the theorem may fail, as shown by a function that breaks continuity at the endpoint.
- 📈 Rolle's Theorem is a special case of the Mean Value Theorem where the function values at the endpoints a and b are equal, implying the existence of a point c where the derivative is zero.
- 🔑 To prove the Mean Value Theorem, one typically starts with Rolle's Theorem, which is a simpler case, and then generalizes the proof.
- ⛰ The Mean Value Theorem is fundamental in calculus and is often used as a stepping stone for proving other more complex theorems.
- 🤔 The theorem prompts consideration of why certain conditions, such as differentiability and continuity, are necessary for its application.
- 📊 The graphical representation of the theorem helps in understanding the relationship between the secant line, the tangent line, and the function's behavior between two points.
- 🧩 The theorem guarantees the existence of at least one point c where the slope of the tangent line equals the slope of the secant line, given the function meets the criteria.
- ↔️ The theorem's conditions must be met for the function over the entire specified interval, not just at the endpoints or at a single point.
Q & A
What is the Mean Value Theorem?
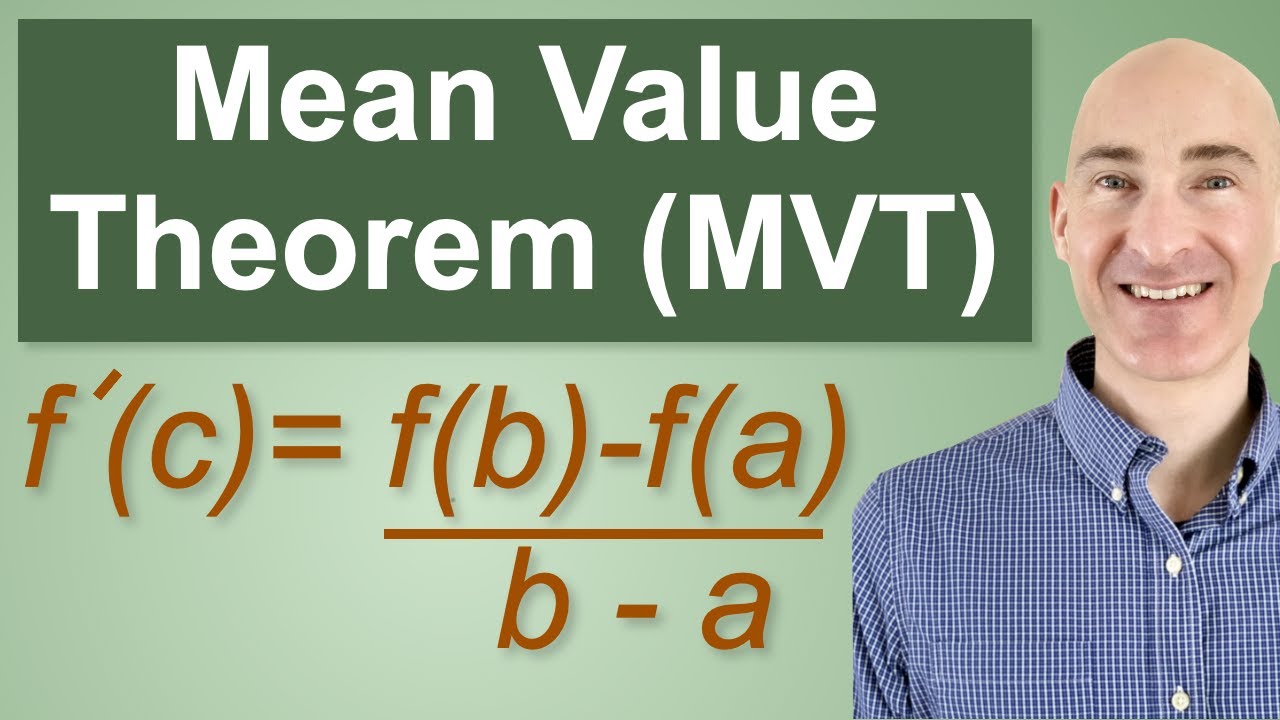
-The Mean Value Theorem states that, for a function that is differentiable on the open interval (a, b) and continuous on the closed interval [a, b], there exists at least one point 'c' in the interval (a, b) where the slope of the tangent line at 'c' is equal to the slope of the secant line between the points (a, f(a)) and (b, f(b)).
What are the conditions required for the Mean Value Theorem to apply?
-The conditions required for the Mean Value Theorem to apply are that the function must be differentiable on the open interval (a, b) and continuous on the closed interval [a, b].
What is the significance of the secant line in the Mean Value Theorem?
-The secant line is significant because it connects two points on the graph of a function, and its slope represents the average rate of change of the function between those two points. The Mean Value Theorem states that there is at least one point where the slope of the tangent line equals the slope of the secant line.
What is Rolle's Theorem, and how is it related to the Mean Value Theorem?
-Rolle's Theorem is a special case of the Mean Value Theorem that applies when the function has the same value at the endpoints, f(a) = f(b). It states that there exists at least one point 'c' in the open interval (a, b) where the derivative of the function is zero. Rolle's Theorem is often used to prove the Mean Value Theorem.
Why is differentiability required for the Mean Value Theorem to hold?
-Differentiability is required because the theorem involves the slope of the tangent line, which is the derivative of the function at a point. If the function is not differentiable at some point in the interval, the concept of a tangent line (and thus its slope) does not apply, making the theorem inapplicable.
Why is continuity required for the Mean Value Theorem?
-Continuity is required to ensure that the function behaves well over the interval [a, b], including at the endpoints. If the function is not continuous, it may have discontinuities that prevent the existence of a tangent line with the same slope as the secant line over the interval.
What happens if a function is not differentiable on the interval [a, b]?
-If a function is not differentiable on the interval [a, b], it may have corner points or cusps where the derivative does not exist. In such cases, the Mean Value Theorem does not apply because the concept of a tangent line (and its slope) is not defined at those points.
What is the geometric interpretation of the Mean Value Theorem?
-The geometric interpretation of the Mean Value Theorem is that for a 'nice' function (one that is differentiable and continuous on the given interval), there is at least one point on the graph where the tangent line is parallel to the secant line connecting the endpoints of the interval.
How does the Mean Value Theorem relate to the concept of instantaneous rate of change?
-The Mean Value Theorem relates to the concept of instantaneous rate of change by stating that there is at least one point within the interval where the instantaneous rate of change (the derivative) equals the average rate of change (the slope of the secant line) over that interval.
What is the role of the point 'c' in the Mean Value Theorem?
-The role of the point 'c' in the Mean Value Theorem is to be a specific point within the open interval (a, b) where the slope of the tangent line to the function at 'c' is equal to the slope of the secant line between the endpoints (a, f(a)) and (b, f(b)).
Why is it important to distinguish between the open interval (a, b) and the closed interval [a, b] in the Mean Value Theorem?
-The distinction is important because the function must be differentiable at every point within the open interval (a, b) for the concept of a tangent line to be valid, while continuity on the closed interval [a, b] ensures the function's behavior is well-defined at the endpoints, including any potential discontinuities.
Can the Mean Value Theorem be applied to any function, regardless of its shape?
-No, the Mean Value Theorem can only be applied to functions that are both differentiable on the open interval (a, b) and continuous on the closed interval [a, b]. Functions with discontinuities or non-differentiable points within the interval do not satisfy the conditions of the theorem.
Outlines
📐 Mean Value Theorem: Intuition and Basics
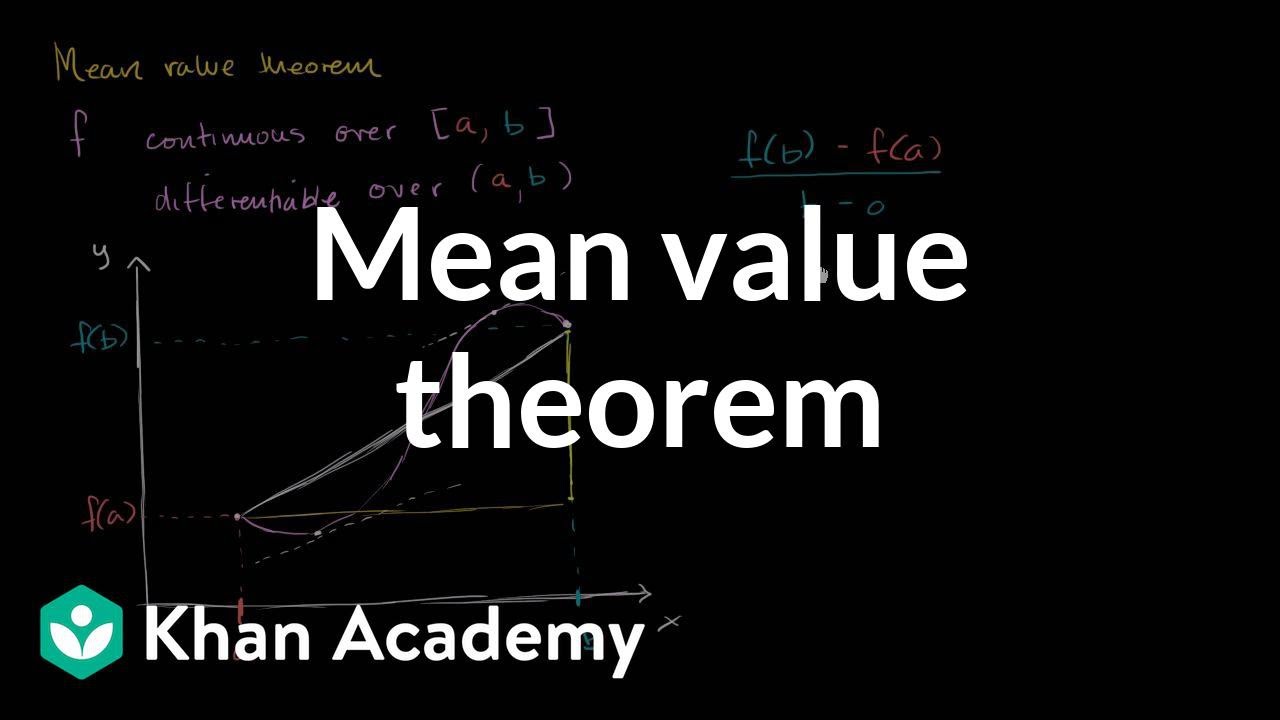
This paragraph introduces the Mean Value Theorem (MVT), a fundamental concept in calculus. The speaker uses a graphical approach to explain the theorem, which states that for a 'nice' function defined on an interval, there exists at least one point where the slope of the tangent line equals the slope of the secant line between the endpoints of the interval. The 'nice' function is characterized by being differentiable on the open interval and continuous on the closed interval, including the endpoints. The explanation includes the mathematical representation of the secant line's slope (F(b) - F(a)) / (b - a) and the tangent line's slope, which is the derivative at some point C within the interval. The importance of differentiability for the theorem's applicability is emphasized through an example of a non-differentiable function with a corner point.
🔍 Continuity and Differentiability: Conditions for MVT
The second paragraph delves into the necessity of the continuity and differentiability conditions for the Mean Value Theorem to hold. It discusses why the theorem does not apply to functions that are not differentiable over the interval, using a graphical example of a function with a corner point. The speaker then explores the requirement of continuity up to the endpoints, providing an example of a function that fails the theorem due to a discontinuity at the endpoint. The paragraph also introduces Rolle's Theorem as a special case of the MVT, where the function values at the endpoints are equal, implying that the tangent line at some point within the interval has a slope of zero. Rolle's Theorem is highlighted as a historically significant case that often serves as a stepping stone to proving the more general MVT.
Mindmap
Keywords
💡Mean Value Theorem
💡Secant Line
💡Tangent Line
💡Derivative
💡Differentiable
💡Continuous
💡Rolle's Theorem
💡Slope
💡Interval
💡Endpoint
💡Graphical Interpretation
Highlights
The Mean Value Theorem is an intuitive theorem that connects the slope of a secant line between two points on a function to the slope of a tangent line at some point within that interval.
The theorem applies to 'nice' functions, which are differentiable on an open interval and continuous on a closed interval, including the endpoints.
The slope of the secant line between points A and B is equivalent to the slope of the tangent line at some point C within the interval.
The derivative at point C is used to represent the slope of the tangent line.
The slope of the secant line is calculated as the rise over run between points A and B on the function.
Differentiability on the open interval (a, b) and continuity on the closed interval [a, b] are necessary conditions for the Mean Value Theorem to hold.
If a function has a corner point, it is not differentiable at that point, and the Mean Value Theorem does not apply.
Continuity on the closed interval [a, b] is required; without it, the secant line may not align with the graph of the function.
Rolle's Theorem is a special case of the Mean Value Theorem where the function values at the endpoints A and B are equal.
In Rolle's Theorem, it is guaranteed that there exists a point C where the derivative is zero, given the function's differentiability and continuity conditions.
The Mean Value Theorem is often proven by first establishing Rolle's Theorem and then generalizing the result.
The Mean Value Theorem is fundamental in mathematical proofs and analysis, often used as a stepping stone to more complex theorems.
The theorem provides a relationship between the average rate of change (secant line) and the instantaneous rate of change (tangent line) of a function.
The theorem is applicable to functions that are 'nice', meaning they have a well-behaved graph that allows for differentiation and continuity.
The existence of a tangent line with the same slope as the secant line is a key claim of the Mean Value Theorem.
The Mean Value Theorem is a powerful tool for understanding the behavior of functions and their rates of change.
The theorem's conditions ensure that the function's graph does not have abrupt changes that would invalidate the comparison between secant and tangent lines.
Understanding the Mean Value Theorem enhances the ability to analyze and predict the behavior of various mathematical functions.
Transcripts
Browse More Related Video

Mean Value Theorem and Rolle's Theorem

Calculus 1 Lecture 3.2: A BRIEF Discussion of Rolle's Theorem and Mean-Value Theorem.

AP Calculus Review Three Theorems You Must Know (EVT, IVT, MVT)

Mean Value Theorem
Mean Value Theorem with Example
Mean value theorem | Existence theorems | AP Calculus AB | Khan Academy
5.0 / 5 (0 votes)
Thanks for rating: