Mean value theorem | Existence theorems | AP Calculus AB | Khan Academy
TLDRThe Mean Value Theorem (MVT) is a fundamental concept in calculus that provides an intuitive understanding of a function's behavior over a closed interval. The theorem states that for a function that is continuous on the closed interval [a, b] and differentiable on the open interval (a, b), there exists at least one point 'c' within the interval (a, b) where the instantaneous rate of change equals the average rate of change over the interval. Visually, this is represented by the slope of the tangent line at point 'c' being the same as the slope of the secant line between points 'a' and 'b'. The theorem is significant as it establishes a relationship between the average change and the instantaneous change at some point within the interval, which is crucial for understanding the behavior of functions and their derivatives.
Takeaways
- 📊 The Mean Value Theorem (MVT) provides an intuitive understanding of the relationship between a function's average rate of change and its instantaneous rate of change over an interval.
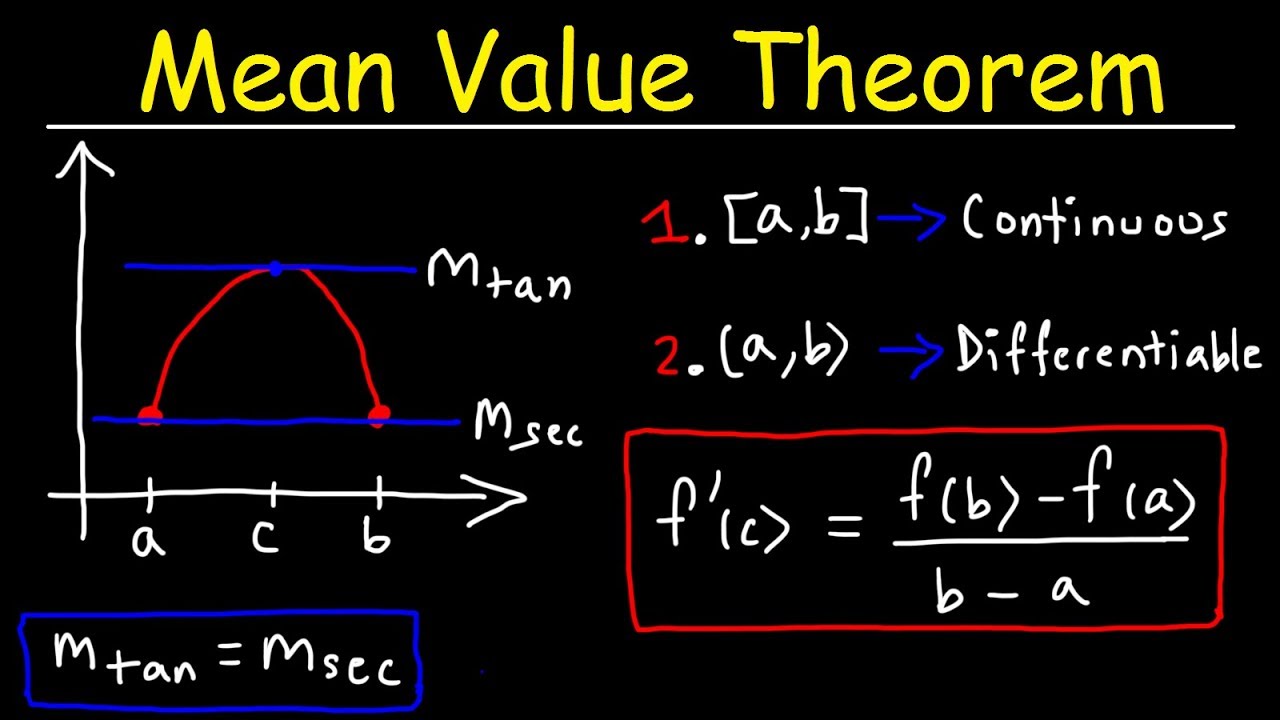
- 🔍 A function must be continuous over the closed interval [a, b] and differentiable over the open interval (a, b) to satisfy the conditions of the MVT.
- 🔑 The theorem states that there exists at least one point 'c' in the open interval (a, b) where the instantaneous rate of change equals the average rate of change over the interval.
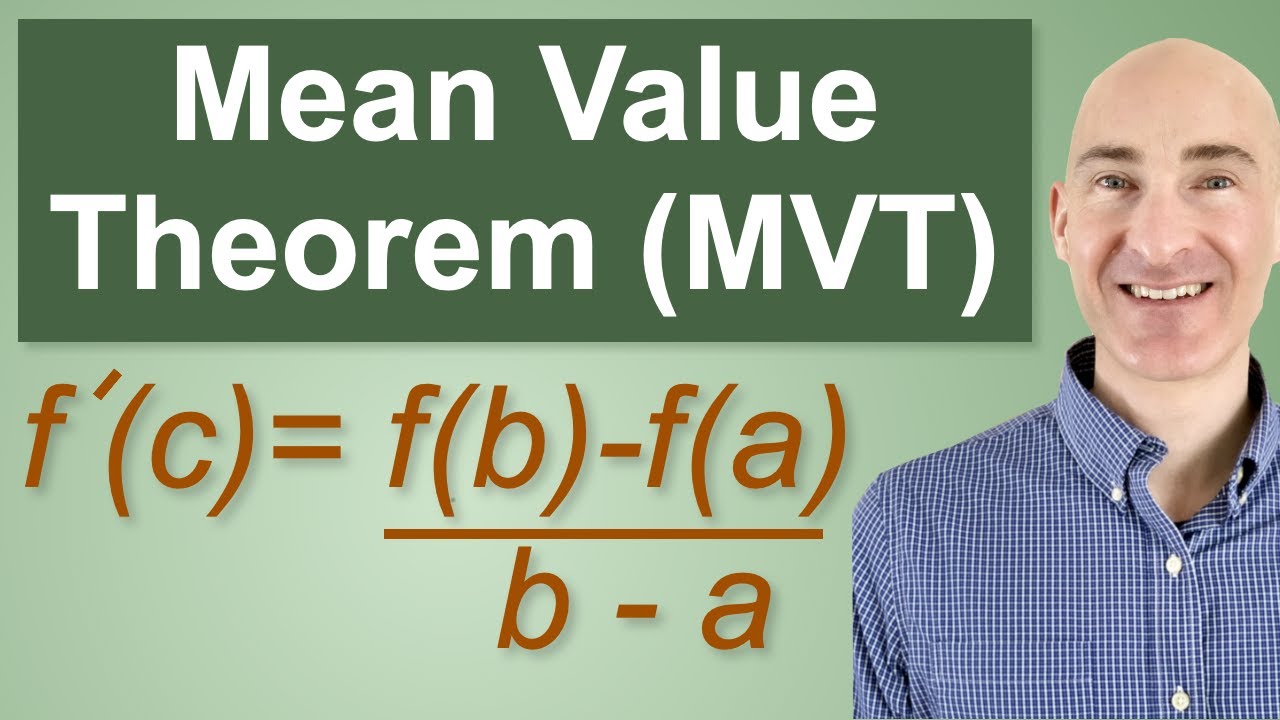
- 📐 The average rate of change is calculated as the slope of the secant line between points a and b, which is (f(b) - f(a)) / (b - a).
- 📈 The instantaneous rate of change at point 'c' is the slope of the tangent line to the function at that point, which is the derivative of the function at 'c'.
- 🌟 Visually, the MVT suggests that at some point 'c', the slope of the tangent line appears to match the slope of the secant line.
- 🎯 The MVT is a fundamental theorem in calculus that connects the concepts of differentiation and integration.
- ✅ The theorem guarantees the existence of a point 'c', but it does not provide the specific location or value of 'c'.
- 🤔 The MVT is often used to prove other theorems and solve problems in calculus, even though it may not be immediately obvious how it applies in certain contexts.
- 📚 Understanding the MVT requires parsing mathematical language and notation, which can be abstract but is essential for grasping the theorem's implications.
- 🚀 The MVT is a powerful tool for analyzing functions and is a cornerstone of real analysis and advanced calculus courses.
Q & A
What is the Mean Value Theorem?
-The Mean Value Theorem states that if a function is continuous on a closed interval [a, b] and differentiable on the open interval (a, b), then there exists a point c in the interval (a, b) such that the instantaneous rate of change at c is equal to the average rate of change over the interval [a, b].
What does it mean for a function to be continuous over a closed interval?
-A function is continuous over a closed interval if there are no gaps or jumps in the function within that interval. This includes the endpoints, meaning the function includes the points a and b.
What is the difference between a closed interval and an open interval?
-A closed interval includes its endpoints, denoted by square brackets [a, b]. An open interval does not include its endpoints, denoted by parentheses (a, b), allowing for the function to potentially be undefined or discontinuous at the endpoints.
What does it mean for a function to be differentiable over an open interval?
-A function is differentiable over an open interval if there is a defined derivative at every point within that interval. It implies the function has a slope at each point and does not have any undefined points or vertical tangents.
How is the average rate of change calculated over an interval?
-The average rate of change over an interval [a, b] is calculated as the change in y (f(b) - f(a)) divided by the change in x (b - a). This is also the slope of the secant line that connects the points (a, f(a)) and (b, f(b)).
What is the instantaneous rate of change at a point on a function?
-The instantaneous rate of change at a point on a function is the derivative of the function at that point, which represents the slope of the tangent line to the function at that specific point.
How does the Mean Value Theorem relate to the slope of the secant line and the tangent line?
-According to the Mean Value Theorem, there exists at least one point c in the interval (a, b) where the slope of the tangent line (instantaneous rate of change) is equal to the slope of the secant line (average rate of change).
What is the significance of the Mean Value Theorem in calculus?
-The Mean Value Theorem is significant because it provides a connection between the average rate of change over an interval and the instantaneous rate of change at some point within that interval. It is a fundamental theorem that underpins many other calculus concepts and applications.
Can the Mean Value Theorem be applied to any function?
-No, the Mean Value Theorem can only be applied to functions that are continuous on a closed interval and differentiable on the corresponding open interval. If a function fails to meet these conditions, the theorem does not guarantee the existence of a point where the average and instantaneous rates of change are equal.
What does the theorem imply about the behavior of a function within the interval (a, b)?
-The theorem implies that regardless of the shape of the function within the interval (a, b), there is at least one point where the function's instantaneous rate of change matches the average rate of change over the entire interval.
How can the Mean Value Theorem be visualized geometrically?
-Geometrically, the Mean Value Theorem can be visualized by drawing a function's graph with the interval [a, b] marked on the x-axis. The theorem states that there is at least one point where the slope of the tangent to the curve at that point (c, f(c)) is the same as the slope of the secant line between the points (a, f(a)) and (b, f(b)).
What is the role of the point 'c' in the Mean Value Theorem?
-The point 'c' is the value within the open interval (a, b) where the instantaneous rate of change of the function equals the average rate of change over the interval. It is a crucial element of the theorem as it demonstrates the existence of such a point, even though the specific location of 'c' may not be determined without further information.
Outlines
📐 Understanding the Mean Value Theorem
This paragraph introduces the Mean Value Theorem (MVT) by explaining its intuitive nature despite the mathematical terminology. The function f is considered, which is continuous over the closed interval [a, b] and differentiable over the open interval (a, b). The MVT states that there exists at least one point 'c' within the open interval where the instantaneous rate of change equals the average rate of change over the entire interval. The average rate of change is represented by the slope of the secant line between points a and b, and the instantaneous rate of change is the slope of the tangent line at point 'c'. The visual representation of this theorem is also discussed, showing how the slopes of the secant and tangent lines can be the same at some point 'c'.
🔍 Existence of a Point 'c' in the MVT
The second paragraph delves deeper into the MVT, emphasizing the existence of a point 'c' in the open interval (a, b) where the instantaneous rate of change matches the average rate of change. It reiterates the conditions for the theorem: the function must be continuous over the closed interval [a, b] and differentiable over the open interval (a, b). The mathematical representation of the average rate of change is also explained, which is the change in y (f(b) - f(a)) over the change in x (b - a). The paragraph concludes by noting that the theorem guarantees the presence of a point 'c' where the instantaneous slope equals the average slope, and hints at a future real-life example to illustrate the concept.
Mindmap
Keywords
💡Mean Value Theorem
💡Continuous Function
💡Differentiable Function
💡Closed Interval
💡Open Interval
💡Instantaneous Rate of Change
💡Average Rate of Change
💡Secant Line
💡Tangent Line
💡Derivative
💡Interval
Highlights
The Mean Value Theorem provides an intuitive understanding of how the average rate of change over an interval is related to the instantaneous rate of change at some point within that interval.
A function f is considered for the theorem, which must be continuous over the closed interval [a, b] and differentiable over the open interval (a, b).
The theorem states that there exists at least one point 'c' in the open interval (a, b) where the instantaneous rate of change equals the average rate of change over [a, b].
Visualizing the function helps in understanding that the instantaneous slope of the tangent line at some point 'c' matches the slope of the secant line between points a and b.
The average rate of change is calculated as the slope of the secant line, which is the change in y (f(b) - f(a)) over the change in x (b - a).
The Mean Value Theorem is applicable even if the function is not differentiable at the endpoints a or b.
The theorem is intuitive as it suggests that at some point within the interval, the instantaneous rate of change will be the same as the average rate over that interval.
The theorem is often illustrated geometrically by comparing the slope of the tangent line at a point 'c' to the slope of the secant line between a and b.
The Mean Value Theorem is a fundamental concept in calculus that connects the ideas of average and instantaneous rates of change.
The theorem can be mathematically expressed as the existence of a value 'c' such that the derivative at 'c' (f'(c)) equals the average rate of change over the interval.
The Mean Value Theorem is a powerful tool for understanding the behavior of differentiable functions without needing to know the explicit form of the function.
The theorem guarantees the existence of a point 'c' where the instantaneous rate of change is not just an approximation but exactly equals the average rate of change.
Understanding the Mean Value Theorem requires parsing mathematical notation and lingo, which once decoded, reveals the theorem's intuitive nature.
The theorem is applicable to a wide range of functions, providing a general principle for analyzing the relationship between points on a function's graph.
The Mean Value Theorem is not only a theoretical concept but also has practical applications in various fields where understanding rates of change is crucial.
The theorem's proof and application often involve advanced mathematical techniques, but its core idea can be grasped intuitively through graphical representation.
The Mean Value Theorem is a cornerstone in the study of calculus, helping to bridge the gap between the concepts of differentiation and integration.
Transcripts
Browse More Related Video

Calculus 1 Lecture 3.2: A BRIEF Discussion of Rolle's Theorem and Mean-Value Theorem.
Mean Value Theorem with Example
Mean Value Theorem
The Mean Value Theorem For Integrals: Average Value of a Function

Mean Value Theorem and Rolle's Theorem

AP Calculus Review Three Theorems You Must Know (EVT, IVT, MVT)
5.0 / 5 (0 votes)
Thanks for rating: